|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Ito K. — Encyclopedic Dictionary of Mathematics |
|
|
 |
| Предметный указатель |
Parameter(s) local canonical (for power series) 339.A
Parameter(s) local uniformizing (of a Riemann surface) 367.A
Parameter(s) location 396.I 400.E
Parameter(s) of regularity (of a Lebesgue measurable set) 380.D
Parameter(s) one- (group of transformations) 105.N
Parameter(s) one- (subgroup of a Lie group) 249.Q
Parameter(s) one-, semigroup of class ( ) 378.B ) 378.B
Parameter(s) scale 396.I 400.
Parameter(s) secondary 110.A
Parameter(s) selection 396.F
Parameter(s) time (of a stochastic process) 407.A
Parameter(s) transformation 396.I
Parameter(s), distinct system of 284.D
Parameter(s), Lagrange’s method of variation of 252.D
Parameter(s), regular system of 284.D
Parameter(s), system of 284.D
Parameter(s), transformation of 111.D
Parameter(s), true value of 398.A
Parametric function 102.A 399.A
Parametric programming 264.C
Parametric representation 165.C
Parametric representation (of a subspace of an affine space) 7.C
Parametric representation (of Feynman integrals) 146.B
Parametrically sustained vibration 318.B
Parametrix 189.C
Parametrix left 345.A
Parametrix right 345.A
Parametrized, effectively (at 0) 72.G
Parasyuk(Parasiuk), Ostap Stepanovich(1921-) 146.A
Paraxial ray 180.B
Paris, Jeffrey B.(1944-) 33.r
Parity check matrix 63.C
Parity check polynomial 63.D
Parity transformation 359.B
Parker, Ernest Tilden(1926-) 151.H 241.B
Parreau — Widom type 164.K
Parreau, Michel(1923-) 164.K 193.G 207.C 367.E
Parry, William(1934-) 136.C 136.r
Parseval equality 18.B 197.C
Parseval identity 18.B 159.A 160.C 192.K 220.B 220.C 220.E
Parseval, Marc Antoine(1755-1836) 18.B 159.A 160.C 160.H 192.M 197.C 220.B 220.C 220.E
Parshin, Aleksei Nikolaevich 118.E
Parsing 31.E
Part(s) (for a function algebra) 164.F
Part(s) connected 150.D
Part(s) cyclic (of an ergodic class) 260.B
Part(s) dissipative (of a state space) 260.B
Part(s) essential 260.I
Part(s) finite (of an integral) 125.C
Part(s) Gleason (for a function algebra) 164.F
Part(s) holomorphic (in a Laurent expansion) 198.D
Part(s) homogeneous (of a formal power series) 370.A
Part(s) imaginary 74.A
Part(s) integration by (for the D-integral) 100.G
Part(s) integration by (for the Riemann integral) 216.C
Part(s) integration by (for the Stieltjes integral) 94.C
Part(s) negative (of an element of a vector lattice) 310.B
Part(s) positive (of an element of a vector lattice) 310.B
Part(s) principal (of a differential operator) 112.A
Part(s) principal (of a Laurent expansion) 198.D
Part(s) principal (of a partial differential operator) 320.B
Part(s) purely contractive 251.N
Part(s) real 74.A
Part(s) semisimple (of a nonsingular matrix) 13.E
Part(s) semisimple (of an algebraic group) 13.E
Part(s) singular (of a Laurent expansion) 198.D
Part(s) unipotent (of a nonsingular matrix) 13.E
Part(s) unipotent (of an algebraic group) 13.E
Parthasarathy, Kalyanapuram Rangachari 213.F 341.r 374.r
Partial boundary operator 200.E
Partial capture 420.D
Partial correlation coefficient 397.J
Partial correlation coefficient sample 280.E
Partial de Rham system 274.G
Partial denominator (of an infinite continued fraction) 83.A
Partial derivative 106.F 106.K
Partial derivative nth-order 106.H
Partial derived functor 200.I
Partial differential 200.H
Partial differential coefficient 106.E
Partial differential equation(s) 313.A 320
Partial differential equation(s) (initial value problems) 321
Partial differential equation(s) (method of integration) 322
Partial differential equation(s) Fokker — Planck 115.A
Partial differential equation(s) hyperbolic 325
Partial differential equation(s) of elliptic type 323 App. Table
Partial differential equation(s) of hyperbolic type 325
Partial differential equation(s) of mixed type 326
Partial differential equation(s) of parabolic type 327
Partial differential equation(s) of the first order 324
Partial differential equation(s) solution, of the first order App. A Table
Partial differential equation(s) solution, of the second order App. A Table
Partial differential equation(s) system of, of order 1 (on a differentiable manifold) 428.F
Partial differential operator 112.A
Partial differentiation 106.F
Partial fraction App. A Table
Partial function 356.E
Partial graph 186.C
Partial mapping (of a mapping) 381.C
Partial numerator (of an infinite continued fraction) 83.A
Partial observation 405.C
Partial ordering 311A
Partial pivoting 302.B
Partial product 379.G
Partial quotient, nth 83.A
Partial recursive (in a partial recursive function) 356.E 356.F
Partial sum (of a series) 379.A
Partial sum diagonal (of a double series) 379.E
Partial summation, Abel 379.D
Partial wave 386.B
Partial wave expansion 375.E 386.B
Partial wave scattering amplitude 375.E
Partially balanced incomplete block design 102.J 406.J
Partially confounded (with blocks) 102.J
Partially conserved axial-vector currents 132.G
Partially differentiable (function) 106.F
Partially isometric (operator) 251.E
Partially ordered set 311.A
Particle(s) Bose 132.A
Particle(s) composite 132.A
Particle(s) elementary 132
Particle(s) Fermi 132.A
Particular solution (for a system of differential equations) 313.C
Particular solution (of a differential equation) 313.A
Particular solution (of partial differential equations) 320.C
Particular transformation (of  ) 248.R ) 248.R
Partition function 402.D
Partition function grand 402.D
Partition(s)  -independent 136.E -independent 136.E
Partition(s) (in ergodic theory) 136.E
Partition(s) (of a set) 381.D
Partition(s) (of a space) 425.L
Partition(s) (of an interval) 216.A
Partition(s) Markov (for an automorphism) 136.C
Partition(s) of numbers 328
Partition(s) of unity 425.R
Partition(s) of unity of class 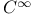 105.S 105.S
Partition(s) of unity subordinate to a covering 425.R
Partition(s) Pinsker 136.E
Partition(s) principal 66.H
Partition(s) upper semicontinuous 425.L
Partition(s), entropy of 136.E
Partition(s), independent sequence of 136.E
Partition(s), number of 177.D 328
Partitioning algorithm 215.E
Parzen, Emanuel(1929-) 421.D
Pascal configuration 78.K
Pascal line 78.K
Pascal theorem (in geometry) 155.E
Pascal theorem (in projective geometry) 343.E
| Pascal theorem (on conic sections) 78.K
Pascal triangle 330
Pascal, B. 329
Pascal, Blaise(1623-1662) 20 75.A 78.K 155.E 181 265 329 330 342.A 343.E
Pascal, Ernesto(1865-?) 15.r
Pascal, Etienne(1588-1651) 329
Pascal, limacon of 93.H
Pasch, Moritz(1843-1930) 155.B
Pasch’s axiom (in geometry) 155.B
Passive (state) 402.G
Passive boundary point 260.I
Passive boundary point dual 260.I
Passive completely 402.G
Passive network 282.C
Passive orthonomic system (of partial differential equations) 428.B
Passman, Donald S.(1940-) 151 .r
Past cone 258.A
Past history, independent of the 406.D
Pasta, J. 287.r
Pasternack, Joel 154.H
Pasting together the boundaries 114.F
Pastur, Leonid Andreevich 340.r
Path (in a Finsler space) 152.C
Path (in a graph) 186.F
Path (in a topological space) 148.C 170
Path (of a Markov process) 261.B
Path (of a stochastic process) 407.A
Path asymptotic (for a meromorphic function) 272.H
Path closed (in a graph) 186.F
Path closed (in a topological space) 170
Path closed, space of 202.C
Path critical 376
Path direct 186.F
Path direct closed 186.F
Path Euler (in a graph) 186.F
Path general geometry of 152.C
Path Hamilton 186.F
path integral 351.F
Path inverse 170
Path nontangential 333.B
Path of an integration (curvilinear integral) 94.D
Path sample 407.A
Path simple 186.F
Path space 148.C 261.B
Path Stolz (in a plane domain) 333.B
Path, projective geometry of 109
Path, quasi-independent of (response probability) 346.G
Path-component 79.B
Path-connected 79.B
Path-dependent, d-trial 346.G
Path-independent (response probability) 346.G
Pathological (space) 65.F
Pathwise uniqueness of solution 406.D
Patil, Ganapati P.(1934-) 374.r
Patodi, Vijay Kumar(1945-1976) 237.r 366.r 391.C 391.K 391.L 391.N 391.r
Pattern formation 263.D
Pauli approximation 351.G
Pauli principle 351.G
Pauli spin matrix 258.A 351.G
Pauli — Lubanski vector 258.D
Pauli, Wolfgang Ernst(1900-1958) 150.A 258.A 258.D 351.G 351.H 351.r 386.B
Pawley, G.Stuart(1937-) 92.F
Payoff 108.B 108.C 173.B
Payoff function 173.C
Pazy, Amnon(1936-) 162 286.X 378.F
PBIBD (partially balanced incomplete block design) 102.J
PC (predictor-corrector) method 303.E
PCT invariance 386.B
PCT theorem 386.B
Peak point 164.D
Peak point generalized 164.D
Peak set 164.D
Peak set generalized 164.D
Peano area (of a surface) 246.F
Peano continuum 93.D
Peano curves 93.J
Peano postulates 294.B
Peano, Giuseppe(1858-1932) 93.D 93.J 106.H 107.A 117.A 156.B 246.F 246.G 267 294.A 294.B 294.r 316.E 411.A
Pearcy, Carl Mark(1935-) 251.r
Pearl, Raymond(1879-1940) 263.A
Pears, Alan(1938-) 117.r
Pearson distribution 397.D
Pearson lemma, Neyman — 400.B
Pearson, D.B. 331.E 375.B
Pearson, Egon Sharpe(1895-1980) 400.B 401.B 401.F 401.r STR
Pearson, Karl(1857-1936) 40.B 174.r 374.r 397.D 401.E 403.C STR
Peclet number 116.B
Peclet, Jean Claude Eugene 116.B
Pedal curve 93.H
Pedersen, Gert Kjaergard(1940-) 36.K 36.r 212.C 308.r
Pederson, Roger N.(1930-) 438.C
Pedoe, Daniel(1910-) 343.r
Peetre, Jaak(1935-) 112.E 112.K 125.F 224.A 224.C 224.E 224.F 224.r
Peierls — Bogolyubov inequality 212.B
Peierls, Rudolf Ernst(1907-) 212.B
Peirce decomposition (of a Jordon algebra) 231.B
Peirce left decomposition (in a unitary ring) 368.F
Peirce right decomposition (in a unitary ring) 368.F
Peirce space 231.B
Peirce, Benjamin Osgood(1854-1914) App.A Table
Peirce, Benjamin(1809-1880) 231.B 368.F
Peirce, Charles Sanders(1839-1914) 156.B 411.A
Peixoto, Mauricio Matos(1921-) 126.A 126.H 126.I 126.M
Pelczynski theorem, Bessaga — 443.D
Peleczynski, Aleksander 37.L 37.r 68.M 443.D
Pell equation 118.A
Pell, John(1611-1685) 118.A
Penalized problems 440.B
Penalty method 292.E
Penalty term 440.B
Pencil algebraic 15.C
Pencil Lefschetz 16.U
Pencil linear 16.N
Pencil of conies 343.E
Pencil of hyperplanes (in a projective space) 343.B
Pencil of lines (in a projective plane) 343.B
Pencil of planes (in a 3-dimensional projective space) 343.B
Pencil of quadric hypersurfaces 343.E
Pencil of quadrics 343.E
Peninsula (in a Riemann surface) 272.J
Pentagamma function 174.B
Pentagon 155.F
Pentagonal number 4.D
Pentagonal number theorem 328
Pentaspherical coordinates 90.B
Pepis, Jozef(c.1922-c.1942) 97.*
Percolation process 340.D
Percolation process bond 340.D
Percolation process site 340.D
Peressini, Anthony L.(1934-) 310.r
Perfect  -(graph) 186.K -(graph) 186.K
Perfect  -(graph) 186.K -(graph) 186.K
Perfect (image) 180.A
Perfect additive functional 261.E
Perfect code 63.B
Perfect delay convention 51.F
Perfect field 149.H
Perfect fluid 205.B
Perfect image 425.CC
Perfect inverse image 425.CC
Perfect kernel (in potential theory) 338.E
Perfect mapping 425.W
Perfect mapping quasi- 425.CC
Perfect number 297.D
Perfect number of the second kind 297.D
Perfect set 425.O
Perfectly normal space 425.Q
Perfectly separable space 425.P
Perfectness theorem 186.K
Perigon, straight 139.D
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 )
) )
)  -independent
-independent
 -(graph)
-(graph)  -(graph)
-(graph)