|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Ito K. — Encyclopedic Dictionary of Mathematics |
|
|
 |
| Предметный указатель |
Optimal solution 255.A 264.B 292.A
Optimal solution basic 255.A
Optimal stopping 405.E
Optimality A- 102.E
Optimality D- 102.E
Optimality E- 102.E
Optimality, principle of 127. A
Optimization model 307.C
Optimum allocation 373.E
Optimum predictor, linear 395.D
Optional  -algebra 407.B -algebra 407.B
Optional (stochastic process) 407.B
Optional sampling 262.C
Optional sampling theorem 262.A
Orbit (= system of transitivity) 362.B
Orbit (of a dynamical system) 126.B
Orbit (of a permutation group) 151.H
Orbit (of a topological transformation group) 110.A 431.A
Orbit closed 126.D
Orbit determination 309.A
Orbit exceptional 431.C
Orbit principal 431.C
Orbit pseudo-,  - 126. J - 126. J
Orbit pseudo-, tracing property 126.J
Orbit singular 431.C
Orbit space (of a topological group) 431.A
Orbit type 431.A
Orbit type principal 431.C
Orbital angular momentum 351.E
Orbital elements, Kepler’s 309.B
Orbital stability (of a solution of a differential equation) 394.D
Orbitally stable 126.F
Ord, J.Keith 374.r
Order  , capacity of 169.C , capacity of 169.C
Order  , Cesaro method of summation of 379.M , Cesaro method of summation of 379.M
Order  , Hoelder condition of 84.A , Hoelder condition of 84.A
Order  , Lipschitz condition of 84.A , Lipschitz condition of 84.A
Order  , potential of 338.B , potential of 338.B
Order  , summable by Cesaro’s method of 379.M , summable by Cesaro’s method of 379.M
Order  -point of the fcth (of a holomorphic function) 198.C -point of the fcth (of a holomorphic function) 198.C
Order ( = a subring) 27.A
Order ( = order relation) 311.A
Order (of a covering) 425.R
Order (of a differential equation) 313.A 320.A
Order (of a differential operator) 112.A
Order (of a function defined by a Dirichlet series) 121.C
Order (of a function on an algebraic curve) 9.C
Order (of a generating point of a simple maximally overdetermined system) 274.H
Order (of a group) 190.C
Order (of a homomorphism of Abelian varieties) 3.C
Order (of a Lie algebra) 191.D
Order (of a meromorphic function) 272.C
Order (of a microdifferential operator) 274.F
Order (of a multistep method) 303.E
Order (of a plane algebraic curve) 9.B
Order (of a point in an ordinary curve) 93.C
Order (of a point with respect to a cycle) 99.D
Order (of a pole of a complex function) 198.D
Order (of a system of differential equations) 313.B
Order (of a transcendental entire function) 429.B
Order (of a zero point of a complex function) 198.C
Order (of an algebraic number field) 14.B
Order (of an element of a group) 190.C
Order (of an elliptic function) 134.E
Order (of an infinitesimal) 87.G
Order (of an infinity) 87.G
Order (of the precision of numerical solution) 303.B
Order 0, frame of 110.C
Order 1, family of frames of 110.B
Order 1, frame of 110.C
Order 2, frame of 110.B 110.C
Order 3, frame of 110.B 110.C
Order 4, frame of 110.B
Order at most (a function) 87.G
Order bounded 310.B
Order convergent sequence (in a vector lattice) 310.C
Order derivatives of higher App. A Table
Order d’Alembert’s method of reduction of 252.F
Order finite (distribution) 125.J
Order function (meromorphic function) 272.B
Order homomorphic (ordered sets) 311.E
Order homomorphism 311.E
Order ideal (of a vector lattice) 310.B
Order infinite (element in a group) 190.C
Order isomorphic (ordered sets) 311.E
Order isomorphism 311.E
Order k, coefficient of 110.A
Order k, converge in the mean of 173.B 342.D
Order k, invariants of 110.A
Order k, population moment of 396.C
Order k, principal components of 110.A
Order k, quantile of 341.H
Order k, Riesz method of summation 379.R
Order k, summable by Hoelder’s method of 379.M
Order k, summable by M. Riesz’s method of 379.R
Order left(of a g-lattice) 27.A
Order limit (in a vector lattice) 310.C
Order maximal (of a g-lattice) 27.A
Order of higher 87.G
Order of lower 87.G
Order of the nth 87.G
Order of the same 87.G
Order p, contravariant tensor field of 105.O
Order p, jet of 105.X
Order principal (fundamental theorem of) 14.C
Order principal (of an algebraic number field) 14.B
Order relation 311.A
Order right (of a g-lattice) 27.A
Order s, covariant tensor field of 105.O
Order small set of 436.G
Order space of line elements of higher 152.C
Order statistic 396.C
Order surface of the second 350.A
Order topology 425.C
Order type 312.A
Order zero point of the kth (of a holomorphic function) 198.C
Order zero point of the — kth (of a complex function) 198.D
Order, difference of the nth 104.A
Order-convergent (in a vector lattice) 310.C
Order-disorder transition 402.F
Order-preserving mapping 311.E
Order-preserving semigroup 286.Y
Ordered additive group 439.B
Ordered additive group totally 439.B
Ordered complex (of a semisimplicial complex) 70.E
Ordered field 149.N
Ordered field Archimedean 149.N
Ordered field Pythagorean 60.O
Ordered group 243.G
Ordered group lattice- 243.G
Ordered group totally 243.G
Ordered linear spaces 310.B
Ordered linear spaces lattice- 310.B
Ordered pair 33.B 381.B
Ordered set 311.A
Ordered set inductively 34.C
Ordered set lattice- 243.A
Ordered set linearly 311.A
Ordered set partially 311.A
Ordered set semi- 311.A
Ordered set totally 311. A
Ordered simplex (in a simplicial complex) 70.E
Ordered simplicial complex 70.C
Ordering 96.C 311.A
Ordering dual 311.A
Ordering duality principle for 311.A
Ordering lexicographic 311.G
Ordering lexicographic linear 248.M
Ordering linear 311.A
Ordering partial 311.A
| Ordering pre- 311.H
Ordering total 311.A
Ordering well- 311.C
Ordeshook, Peter C. 173.r
Ordinal numbers 312.B
Ordinal numbers admissible 356.G
Ordinal numbers constructive 81.B
Ordinal numbers countable 49.E
Ordinal numbers finite 312.B
Ordinal numbers hyperconstructive 81.E
Ordinal numbers initial 49.E
Ordinal numbers isolated 312.B
Ordinal numbers limit 312.B
Ordinal numbers of a higher number class 312.D
Ordinal numbers of the first, second, or third number class 312.D
Ordinal numbers strongly inaccessible 312.E
Ordinal numbers transfinite initial 49.E
Ordinal numbers transfmite 312.B
Ordinal numbers weakly inaccessible 312.E
Ordinal numbers, cardinality of 49.E
Ordinal product (of a family of ordered sets) 311.G
Ordinal scale 397.M
Ordinal sum (of a family of ordered sets) 311.G
Ordinary curve 93.C
Ordinary derivative (of a set function) 380.D
Ordinary differential equation(s) 313 App. Table
Ordinary differential equation(s) (asymptotic behavior of solutions) 314
Ordinary differential equation(s) (boundary value problems) 315
Ordinary differential equation(s) (initial value problems) 316
Ordinary differential equation(s) Euler linear App. A Table
Ordinary differential equation(s) higher-order App. A Table
Ordinary differential equation(s) homogeneous App. A Table
Ordinary differential equation(s) homogeneous (of higher order) App. A Table
Ordinary differential equation(s) linear 252 313.A
Ordinary differential equation(s) linear (global theory) 253
Ordinary differential equation(s) linear (local theory) 254
Ordinary differential equation(s) linear (of higher order) App. A Table
Ordinary differential equation(s) linear (of the first order) App. A. Table 14.I
Ordinary differential equation(s) linear (with constant coefficients) App. A Table
Ordinary differential equation(s) nonlinear 313.A
Ordinary differential equation(s) nonlinear (global theory) 288
Ordinary differential equation(s) nonlinear (local theory) 289
Ordinary differential equation(s), system of 313.B
Ordinary differential operator 112.A
Ordinary Dirichlet series 121.A
Ordinary double point (of a plane algebraic curve) 9.B
Ordinary element 191.I
Ordinary helicoid 111.I
Ordinary helix 111.F 114.F
Ordinary integral element 428.E
Ordinary integral manifold (of a differential ideal) 428.E
Ordinary lower derivative (of a set function) 380.D
Ordinary point (in hyperbolic geometry) 285.C
Ordinary point (of a curve) 93.G
Ordinary point (of an analytic set) 23.B
Ordinary point (of an ordinary curve) 93.C
Ordinary point (on a Riemann surface) 11.D
Ordinary representation (of a finite group) 362.G
Ordinary sense, derivable in the 380.D
Ordinary singularity (of an analytic function) 198.P
Ordinary singularity in the wider sense 198.P
Ordinary solution (of a differential ideal) 428.E
Ordinary upper derivative (of a set function) 380.D
Ordinate set 221.E
Ore, Oystein(1899-1968) 157.r 190.L
Oresme, Nicole(c.1320(30)-1382) 372
Orey, Steven(1928-) 260.J
Orientable (manifold) 105.F 201.N
Orientable (pseudomanifold) 65.B
Orientable fiber bundle 147.L
Orientable transversely 154.B
Orientation (of a contact element) 110. A
Orientation (of a manifold) 105.F 201.N
Orientation (of an affine space) 139.B
Orientation cohomology class 201.N
Orientation local (in an oriented manifold) 201.N
Orientation manifold 201.N
Orientation negative (of an oriented manifold) 105.F
Orientation opposite (of oriented atlases) 105.F
Orientation positive (of an oriented manifold) 105.F
Orientation same (of oriented atlases) 105.F
Orientation sheaf 201.R
Orientation-preserving mapping 99.A
Orientation-reversing mapping 99.A
Oriented atlas (of an orientable differentiable manifold) 105.F
Oriented cobordism class 114.H
Oriented cobordism group 114.H
Oriented differentiable structures, group of (on the combinatorial sphere) 114.I
Oriented element (in a covering manifold) 110.A
Oriented G-manifold 431.E
Oriented graph 186.B
Oriented manifold 105.F 201.N
Oriented manifold integrals over 105.T
Oriented pseudomanifold 65.B
Oriented pseudomanifold coherently 65.B
Oriented q-simplex 201.C
Oriented real hypershpere 76.A
Oriented segment 442.A
Oriented simplicial chain complex 201.C
Oriented singular r-simplex of class 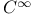 105.T 105.T
Oriented tangent line 76.B
Origin (of a Euclidean space) 140
Origin (of a projective frame) 343.C
Origin (of an affine space) 7.C
Orihara, Masae(1915-) 310.r
Orlicz space 168.B
Orlicz — Pettis theorem 443.D
Orlicz, Wladyslaw(1903-) 168.B 443.D
Ornstein — Uhlenbeck Brownian motion 45.I
Ornstein, Donald S.(1934-) 5.G 136.B 136.C 136.E—G 136.r 162 213.E 213.F
Ornstein, Leonard Salomon(1880-1941) 45.I
Ortega, James McDonogh(1932-) 301.r
Orthant, positive 89.G
Orthochronous 258.A
Orthocomplement (of a subspace of a linear space) 139.G
Orthogonal (block design) 102.J
Orthogonal (elements of a ring) 368.B
Orthogonal (functions) 317.A
Orthogonal (in a Hilbert space) 197.C
Orthogonal (in Euclidean geometry) 139.E 139.G
Orthogonal (linear subspaces) 256.G
Orthogonal array 102.L
Orthogonal complement (of a subset of a Hilbert space) 197.E
Orthogonal component (of an element of a linear space) 139.G
Orthogonal coordinate system adapted to (a flag) 139.E
Orthogonal curvilinear coordinate system App. A Table
Orthogonal curvilinear coordinates 90.C
Orthogonal expansion 317.A
Orthogonal for a finite sum 19.G
Orthogonal fractional factorial design 102.I
Orthogonal frame 111.B 139.E
Orthogonal frame bundle 364.A
Orthogonal frame bundle tangent 364.A
Orthogonal function(s) 317 App. Table
Orthogonal function(s) Haar system of 317.C
Orthogonal function(s) Rademacher system of 317.C
Orthogonal function(s), Walsh’s system of 317.C
Orthogonal group 60.I 139.B 151.I
Orthogonal group (over a noncommutative group) 60.O
Orthogonal group complex 60.I
Orthogonal group complex special 60.I
Orthogonal group infinite 202.V
Orthogonal group over K with respect to Q 60.K
Orthogonal group pair 422.I
Orthogonal group proper 60.I 258.A
Orthogonal group reduced 61.D
Orthogonal group special 60.I
Orthogonal k-frame (in 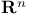 ) 199.B ) 199.B
Orthogonal matrix 269.J
Orthogonal matrix complex 269.J
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 -algebra
-algebra  -
-  -point of the fcth (of a holomorphic function)
-point of the fcth (of a holomorphic function) 
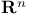 )
)