|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Ito K. — Encyclopedic Dictionary of Mathematics |
|
|
 |
| Предметный указатель |
Mechanics equilibrium statistical 402.A
Mechanics graphical 19.C
Mechanics Markov statistical 340.C
Mechanics Newtonian 271.A
Mechanics nonlinear 290.A
Mechanics quantum 351
Mechanics quantum statistical 402.A
Mechanics statistical 342.A 402
Mechanism, Higgs 132.D
Median 341.H 396.C 397.C
Median sample 396.C
Median unbiased estimator 399.C
Mediant (of two fractions in Farey sequence) 4.B
Medieval mathematics 372
Meeks, William Hamilton, III 235.E 275.C 275.D
Meet (in a Boolean algebra) 42.A
Meet (in an ordered set) 243.A
Meet (of sets) 381.B
Mehler formula App. A Table
Mehler integral representation, Laplace — App. A Table
Mehler, Ferdinand Gustav(1835-1895) App.A Tables 19.III
Mehra, Raman K. 86.r
Meinardus, Gunter(1926-) 328
Meinhardt, Hans 263.D
Meixner, Josef(1908-) 268.r 389.r
Melin, Anders(1943-) 345.A
Mellin transform 220.C
Mellin, Robert Hjalmar(1854-1933) 206.D 220.C
Melrose, Richard B.(1949-) 325.M
Member (of a set) 381.A
Membrane equation of a vibrating 325.A
Membrane permeable 419.A
Memory channel almost finite 213.F
Memory channel finite 213.F
Memory fading 163.I
Memory first-in first-out 96.E
Memory first-in last-out 96.E
Memory unit 75.B
Memoryless channel 213.C
Memoryless channel discrete 213.F
Menaechmus(375-325 B.C.) 187
Mendelson, Elliot(1931-) 33.D 33.r 319.r
Menelaus theorem (in affine geometry) 7.A
Menelaus(of Alexandria)(fl.98?) 7.A 187
Menger — Noebeling embedding theorem 117.D
Menger, Karl(1902-) 93.D 117.A 117.B 117.D 117.r 426
Menikoff, Arthurs(1947-) 325.H
Men’shov theorem, Looman — 198.A
Men’shov theorem, Looman — Rademacher — 317.B
Men’shov, Dmitrii Evgen’evich(1892-) 77.A 159.J 198.A 198.r 317.B
Meray, Hugues Charles Robert(1835-1911) 267
Mercer theorem 217.H
Mercer, J. 217.H
Mergclyan, Sergei Nikitovich(1928-) 164.J 336.F 367.G
Merging 96.C
Meridian (of a knot) 235.B
Meridian (of a surface of revolution) 111.H
Merluzzi, P. 303.r
Merman, G.A. 420.D
Meromorphic (in a domain) 272.A
Meromorphic curve 272. L
Meromorphic differential (on a Riemann surface) 367.H
Meromorphic function(s) 21.J 272.A
Meromorphic function(s) (on a complex manifold) 72.A
Meromorphic function(s) (on an analytic set) 23.D
Meromorphic function(s) transcendental 272.A
Meromorphic mapping, proper (between analytic spaces) 23.D
Meromorphy circle of (of a power series) 339.D
Meromorphy radius of (of a power series) 339.D
Mersenne number 297.E App. Table
Mersenne prime 297.E
Mersenne, Mann(1588-1647) 297.E App.B Table
Mertcns, Franz Carl Josef(1840-1927) 123.A 379.F
Mertens theorem (on the Cauchy product of two series) 379.F
Meschkowski, Herbert(1909-) 188.r
Mesh of a covering (in a metric space) 273.B
Meshalkin, Lev Dmitrievich(1934-) 136.E
Mesons 132.B
Messiah, Albert M.L.(1921-) 351.r
Messing, William(1945-) 450.Q
Meta-Abelian group 190.H
Metabolic model (in catastrophe theory) 51.F
Metamathematics 156.D
Metastable range (of embeddings) 114.D
Method(s) (p+1)-stage 303.D
Method(s) Abel, of summation 379.N
Method(s) Adams — Bashforth 303.E
Method(s) Adams — Moulton 303.E
Method(s) ADI 304.F
Method(s) alternating direction implicit (ADI) 304.F
Method(s) Arrow — Hurwicz — Uzawa gradient 292.E
Method(s) Bairstow 301.E
Method(s) Bernoulli 301.J
Method(s) Borel, of summation 379.O
Method(s) branch and bound 215.D
Method(s) Cesaro, of summation of order  379.M 379.M
Method(s) Cholesky 302.B
Method(s) circle 4.B
Method(s) collocation 303.I
Method(s) congruence 354.B
Method(s) conjugate gradient (C.G) 302.D
Method(s) constructive 156.D
Method(s) continuation 301.M
Method(s) Crout 302.B
Method(s) cyclic Jacobi 298.B
Method(s) Danilevskii 298.D
Method(s) Davidenko, of differentiation with respect to a parameter 301.M
Method(s) Dejon — Nickel 301.G
Method(s) difference 303.A
Method(s) direct (in the calculus of variations) 46.E
Method(s) discrete variable 303.A
Method(s) distribution-free 371.A
Method(s) Doolittle 302.B
Method(s) downhill 301.L
Method(s) Duhamel 322.D
Method(s) Durand — Kerner (DK) 301.F
Method(s) Durand — Kerner — Aberth (DKA) 301.F
Method(s) d’Alembert, of reduction of order 252.F
Method(s) Enskog 217.N
Method(s) Euclidean 150.F
Method(s) Euler (of describing the motion of a fluid) 205.A
Method(s) Euler (of numerical solution of ordinary differential equations) 303.E
Method(s) Euler (of summation) 379.P
Method(s) expansion 205.B
Method(s) extrapolation 303.F
Method(s) factorization 206.B
Method(s) finite element 223.G 304.C
Method(s) fixed point 138.B
Method(s) floatingpoint 138.B
Method(s) Frobenius App. A Table
Method(s) Galerkin 303.I 304.B
Method(s) Garside — Jarratt — Mack 301.N
Method(s) Gauss — Seidel 302.C
Method(s) Givens 298.D
Method(s) gradient 292.E
Method(s) Graeffe 301.N
Method(s) graphical, of statistical inference 19.B
Method(s) Green function 402.J
Method(s) Hessenberg 298.D
Method(s) Hill, of solution 268.B
Method(s) Hitchcock 301.E
Method(s) hodograph 205.B
Method(s) Horner 301.C
Method(s) Householder 298.D
Method(s) implicit 303.E
Method(s) implicit enumeration 215.D
Method(s) Ince — Goldstein 268.C
Method(s) indirect least squares 128.C
Method(s) interpolation 224.A
Method(s) isoparametric 304.C
| Method(s) Jacobi (in numerical solution of linear equations) 302.C
Method(s) Jacobi (of numerical computation of eigenvalues) 298.B
Method(s) Jacobi second, of integration 324.D
Method(s) Jeffreys 25.B
Method(s) killing (of obtaining a homotopy group) 202.N
Method(s) ladder 206.B
Method(s) Lagrange (of describing the motion of a fluid) 205.A
Method(s) Lagrange (of indeterminate coefficients) 106.L
Method(s) Lagrange — Charpit 322.B App. Table
Method(s) Lagrange, of variation of constants 252.D
Method(s) Lagrange, of variation of parameters 252.D
Method(s) Lanczos 298.D 301.N
Method(s) Laplace 30.B
Method(s) Lebesgue, of summation 379.S
Method(s) Lehmer 301.K
Method(s) Lighthill 25.B
Method(s) limited information maximum likelihood 128.C
Method(s) linear k-step 303.E
Method(s) linear multistep 303.E
Method(s) Mathieu 268.C
Method(s) maximum likelihood 399.M
Method(s) middle-square 354.B
Method(s) Milne 303.E
Method(s) modified minimum chi-square 400.K
Method(s) moment 399.L
Method(s) Monte Carlo 385.C
Method(s) multistep 303.E
Method(s) multivalue 303.E
Method(s) Newton — Raphson 301.D
Method(s) Noerlund, of summation 379.Q
Method(s) nonparametric 371.A
Method(s) of averaging 290.D
Method(s) of false position 301.C
Method(s) of feasible directions (in nonlinear programming) 292.E
Method(s) of harmonic balance 290.D 290.E
Method(s) of Lagrange multipliers 106.L
Method(s) of least squares (for estimation) 403.E
Method(s) of least squares (for numerical solution of linear equations) 397.J
Method(s) of least squares (for numerical solution of ordinary differential equations) 303.I
Method(s) of linearization 290.D
Method(s) of majorants 316.G
Method(s) of matched asymptotic expansions 25.B
Method(s) of moving frames 110.A
Method(s) of multiple scales 290.E
Method(s) of orthogonal projection 323.G
Method(s) of quadrature 313.D
Method(s) of steepest descent 25.C
Method(s) of successive approximation (for an elliptic partial differential equation) 323.D
Method(s) of successive approximation (for Fredholm integral equations of the second kind) 217.D
Method(s) of successive approximation (for ordinary differential equations) 316.D
Method(s) of successive iteration (for Fredholm integral equations of the second kind) 217.D
Method(s) of summation 379.L
Method(s) of variation of constants 55.B 252.I
Method(s) of variation of parameters App. A Table
Method(s) penalty 292.E
Method(s) Perron (in Dirichlet problem) 120.C
Method(s) perturbation 25.B
Method(s) Poincare 25.B
Method(s) Poincare — Lighthill — Kuo (P.L.K.) 25.B
Method(s) polynomial extrapolation 303.F
Method(s) power 298.C
Method(s) predictor-corrector (PC) 303.E
Method(s) projective approximation 304.B
Method(s) QR 298.F
Method(s) QZ 298.G
Method(s) rational extrapolation 303.F
Method(s) Rayleigh — Ritz 46.F 271.G
Method(s) renormalization 361.A
Method(s) Richardson 302.C
Method(s) Riemann, of summation 379.S
Method(s) Riesz, of summation of the kth order 379.R
Method(s) Ritz 46.F 303.I 304.B
Method(s) robust 371. A
Method(s) Rosen gradient projection 292.E
Method(s) Runge — Kutta 303.D
Method(s) Runge — Kutta — Gill 303.D
Method(s) saddle point 25.C
Method(s) scaling 346.E
Method(s) scoring 397.M
Method(s) simplex 255.C
Method(s) spectral 304.B
Method(s) stationary phase 30.B
Method(s) step by step 163.D
Method(s) Strum 301.C
Method(s) summable by Abel’s 379.N
Method(s) summable by Borel’s exponential 379.O
Method(s) summable by Borel’s integral 379.O
Method(s) summable by Cesaro’s, of order  379.M 379.M
Method(s) summable by Euler’s 379.P
Method(s) summable by Hoelder’s, of order p 379.M
Method(s) summable by M. Riesz’s, of order k 379.R
Method(s) summable by Noerlund’s 379.Q
Method(s) Sylvester elimination 369.E
Method(s) three-stage least squares 128.C
Method(s) threshold Jacobi 298.B
Method(s) two-phase simplex 255.C
Method(s) two-stage least squares 128.C
Method(s) variational 438.B
Method(s) WKB 25.B
Method(s) WKBJ 25.B
Metivier, Michel(1931-) 443.H
Metric 273.B
Metric 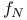 136.F 136.F
Metric  - 136.F - 136.F
Metric Bergman 188.G
Metric comparison theorem 178.A
Metric connection 80.K
Metric Einstein 364.I
Metric Einstein — Kaehler 232.C
Metric Finsler 152.A
Metric Hermitian 232.A
Metric Hodge 232.D
Metric invariant (on a measure space) 136.E
Metric Kaehler 232.A
Metric Kerr 359.E
Metric left invariant (in a topological group) 423.I
Metric multidimensional scaling 346.E
Metric Petersson 32.B
Metric Poincare 74.G
Metric pseudo- 273.B
Metric pseudo-Riemannian 105.P
Metric Riemannian 105.P
Metric Robertson-Walker 359.E
Metric space(s) 273
Metric space(s) compact 273.F
Metric space(s) complete 273.J
Metric space(s) discrete 273.B
Metric space(s) indiscrete pseudo- 273.B
Metric space(s) induced by a mapping 273.B
Metric space(s) precompact 273.B
Metric space(s) product 273.B
Metric space(s) pseudo- 273.B
Metric space(s) separable 273.E
Metric space(s) totally bounded 273.B
Metric standard Kaehler (of a complex projective space) 232.P
Metric structure almost contact 110.E
Metric structure contact 110.E
Metric subspace 273.B
Metric Teichmiiller 416
Metric topology 425.C
Metric vector space 256.H
Metrically isomorphic automorphisms (on a measure space) 136.E
Metrizable topological group 423.I
Metrizable topological space 273.K
Metrizable uniform space 436.F
Metrizable uniform space pseudo- 436.F
Meusnier theorem (on surfaces) 111.H
Meusnier, Jean Baptiste Marie Charles(1754-1793) 109 111.H 275.A 334.B
Meyer decomposition theorem, Doob — 262.D
Meyer, Franz(1856-1934) 267
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте




 -
-