|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Ito K. — Encyclopedic Dictionary of Mathematics |
|
|
 |
| Предметный указатель |
Vector field without source 442.D
Vector field without vortex 442.D
Vector field, integral of App. A Table
Vector flux (through a surface) 442.D
Vector function, measurable 308.G
Vector group 422.E
Vector integral 443.A
Vector invariant 226.C
Vector lattice 310.B
Vector lattice  -complete 310.C -complete 310.C
Vector lattice Archimedean 310.C
Vector lattice complete 310.C
Vector lattice normed 310.F
Vector line (of a vector field) 442.D
Vector measure 443.G
Vector measure absolutely continuous 443.G
Vector measure bounded 443.G
Vector measure completely additive 443.G
Vector measure finitely additive 443.G
Vector potential 130.A 442.D
Vector product 442.C App. Table
Vector representation (of a Clifford group) 61.D
Vector space 442.A
Vector space metric 256.H
Vector space over a field 256.H
Vector space prehomogeneous 450.V
Vector space standard (of an affine space) 7.A
Vector space tangent 105.H
Vector space topological 424.A
Vector triple product 442.C App. Table
Vector tube 442.D
Vector(s) (in a Euclidean space) 442
Vector(s) (in a linear space) 256.A
Vector(s) analytic 437.S
Vector(s) characteristic (of a linear mapping) 269.L
Vector(s) characteristic (of a linear operator) 390.A
Vector(s) characteristic (of a matrix) 269.F
Vector(s) coherent 377.D
Vector(s) collinear 442.A
Vector(s) column 269.A
Vector(s) contravariant 256.J
Vector(s) coplanar 442.A
Vector(s) covariant 256.J
Vector(s) cyclic (of a representation space of a unitary representation) 437.A
Vector(s) effect 102.A
Vector(s) eigen- (of a linear mapping) 269.L
Vector(s) eigen- (of a matrix) 269.F
Vector(s) eigen-, generalized 390.B
Vector(s) error 102.A
Vector(s) fixed 442.A
Vector(s) four- 359.C
Vector(s) four-, energy-momentum 258.C
Vector(s) free 442.A
Vector(s) free vacuum 150.C
Vector(s) fundamental (in a vector space) 442.A
Vector(s) horizontal 80.C
Vector(s) independent 66.F
Vector(s) integral 428.E
Vector(s) mean 341.B
Vector(s) mean curvature 365.D
Vector(s) normal 105.L 111.H 364.A
Vector(s) normalized 409.G
Vector(s) observation 102.A
Vector(s) orthogonal 139.G
Vector(s) p- 256.O
Vector(s) p-, bundle of 147.F
Vector(s) positive 7.A 442.A
Vector(s) Poynting 130.A
Vector(s) proper (of a linear mapping) 269.L
Vector(s) proper (of a linear operator) 390.A
Vector(s) proper (of a matrix) 269.F
Vector(s) root 390.B
Vector(s) row 269.A
Vector(s) tangent 105.H
Vector(s) tangent, holomorphic 72.A
Vector(s) tangent, of type (0,1) 72.C
Vector(s) tangent, of type (1,0) 72.C
Vector(s) unit 442.B
Vector(s) unit (of an affine frame) 7.C
Vector(s) vacuum 377. A
Vector(s) valuation 6.C
Vector(s) valuation, ring of 6.C
Vector(s) vector(s) eigen- (of a linear operator) 390.A
Vector(s) vertical 80.B
Vector(s) wave number (of a sine wave) 446
Vector(s) Witt 449
Vector(s) Witt, of length n 449.B
Vector(s) zero 442.A
Vector-valued integral 443
Vectorial form, canonical 417.C
Vectorial p-form 417.C
Vedesinov, N. 425.Q
Veech, William Austin(1938-) 136.H
Vekua, Il’ya Nestorovich(1907-1977) 217.J 323.r
Veldkamp, Ferdinando D. 13.R
Velo, Giorgio 150.r
Velocity group 446
Velocity phase (of a sine wave) 446
Velocity phase space 126.L
Velocity potential 205.B
Veneziano model 132.C 386.C
Veneziano, Gabriele(1942-) 132.C 386.C
Venkov, Boris Borisovich(1934-) 200.M
Venttsel’, Aleksandr Dmitrievich(1937-) 115.C 261.r 406.F
Ver Eecke, Paul(1867-1959) 187.r
Verbeure inequality, Roepstorff — Fannes — 402.G
Verbeure, Andre(1940-) 402.G
Verbiest, Ferdinand(1623-1688) 57.C
Verdier, Jean-Louis(1935-) 16.r 450.Q 450.r
Vergne, Michele 384.r
Verhulst, Pierre Francois(1804-1849) 263.A
Verner, James Hamilton(1940-) 303.r
Veronese surface 275.F
Veronese, Giuseppe(1854-1917) 275.F
Versal (unfolding) 51.D
Vershik, Anatolil Moiseevich(1933-) 136.D 136.r 183.r
Version (of a stochastic process) 407.A
Vertex (in a cell complex) 70.D
Vertex (in a Euclidean (simplicial) complex) 70.B
Vertex (in a simplicial complex) 70.C
Vertex (in the polyhedron of a simplicial complex) 70.C
Vertex (of a circular cone) 78.A
Vertex (of a complete quadrangle) 343.C
Vertex (of a convex cell in an affine space) 7.D
Vertex (of a convex polyhedron) 89.A
Vertex (of a geodesic triangle) 178.A
Vertex (of a graph) 186.B
Vertex (of a linear graph) 282.A
Vertex (of a parabola) 78.C
Vertex (of a polygon) 155.F
Vertex (of a simplex in an affine space) 7.D
Vertex (of a spherical triangle) 432.B
Vertex (of a star region) 339.D
Vertex (vertices) (of an angle) 139.D 155.B
Vertex adjacent 186.B
Vertex end 186.B
Vertex initial 186.B
Vertex isolated 186.B
Vertex terminal 186.B
Vertical angles 139.D
Vertical component (of a vector field) 80.C
Vertical slit mapping, extremal 367.G
Vertical vector 80.B
Very ample (divisor) 16.N
Very ample (linear system) 16.N
Very ample (sheaf) 16.E
Very weak Bernoulli process 136.E
Vesentini, Edoarc (1928-) 122.F
Vessiot extension field, Picard — 113
Vessiot theory, Picard — 113
| Vessiot, Ernest(1965-1952) 107.A 113 249.V
Vey classes, Godbillon — 154.G
Vey, Jacques 154.G 154.r 384.r
Vibrating membrane, equation of a 325.A
Vibrating string, equation of a 325.A
Vibration 318
Vibration normal 318.B
Vibration parametrically sustained 318.B
Vibration self-excited 318.B
Vick, James Whiyfield(1942-) 201.r
Viehweg, Eckart 72.I 72.r 232.D 232.r
Viete, F. 444
Viete, Francois 8 20 332 360 444
Vietoris axiom 425.Q
Vietoris exact sequence, Mayer- (for a proper triple) 201.C
Vietoris, Leopold(1891—) 201.A 201.C 201.E 201.L 425.Q
Vigneras, Marie-France(1946-) 391.C
Vigue, Jean-Pierre(1948-) 384.r
Vilenkin, Naum Yakovlevich(1920-) 112.r 125.r 162.r 218.r 341.r 389.r 395.r 407.C 437.AA
Villat integration formula App. A Table
Villat, Henri Rene Pierre(1879-1972) App.A Table
Ville, Jean A. 262.A
Vinberg, Ernest Borisovich(1937-) 122.G 351.I 384.C 384.r
Vinogradov mean value theorem 4.E
Vinogradov, Ivan M.(1891-1983) 4.C 4.E 123.B 123.E 242.M 295.E
Vinter, Richard B. 127.G
Virtanen, Kaarlo I. 62.C 352.A 352.C 367.E 367.I
Virtual arithmetic genus (of a divisor) 16.E
viscosity 205.B
Viscosity coefficient of bulk 205.C
Viscosity coefficient of shear 205.C
Viscosity magnetic 259
Viscosity, coefficient of 205.C
Vishik, Mark Iosifovich(1921-) 112.E 323.N
Visibility manifold 178.F
Vitali covering theorem 380.D
Vitali, Giuseppe(1875-1932) 270.G 380.D
Viterbi, Andrew J.(1935-) 213.E
Vitt, Aleksandr Adol’fovich 290.r
Vitushkin, Anatolii Georgievich(1931—) 164.J 169.E
Vivanti theorem 339.A
Vivanti, Giulio(1859-?) 217.r 339.A
Vladimirova, S.M. 365.J
Vogan, David Alexander, Jr.(1954-) 437.r
Vogel, Kurt(1888-1985) 24.r
Vogel, William R. 200.r
Vogt, Dietmar(1941-) 168.B
Voichick, Michael(1934-) 164.K
Voiculescu, Dan Virgil(1949-) 36.J 331.E
Voider, J.E. 142.C
Voigt, Juergen(1943-) 331.E
Volk, Isai Mikhallovich 289.E
Volkov, Yurii Aleksandrovich(1930-) 365.J
Volkovyskil, L. 198.r
Volterra integral equation 217.A
Volterra operator 68.J
Volterra theorem, Poincare — 198.J
Volterra type, integral equation of 217.A
Volterra type, integrodifferential equation of 22 2.A
Volterra, Vito(1860-1940) 20 68.J 162 163.B 198.J 217.A 222.A 263.B
Voltyanskii, V.G. 155.r
Volume (of a lattice in a Euclidean space) 92.D
Volume (of a polyhedron) 139.F
Volume (of a simplex in an affine space) 7.E
Volume (of an idele) 6.D
Volume element (of an oriented 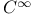 -manifold) 105.W -manifold) 105.W
Volume element associated with a Riemannian metric 105.W
Volume element integral of a function with respect to a (on a 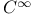 -manifold) 105.W -manifold) 105.W
Volume inner 270.G
Volume outer 270.G
von Eoetvoes, Roland(1848-1919) 359.D
von Karman transonic similarity 205.D
von Karman, Theodore(1881-1963) 205.E 433.C
von Koch, Helge(1870-1924) 246.K 450.I
von Mises theorem 399.K
von Mises, Richard(1883-1953) 298.r 342.A 354.E 399.K 399.r
von Neumann algebra 308.C
von Neumann algebra discrete 308.E
von Neumann algebra finite 308.E
von Neumann algebra induced 308.C
von Neumann algebra of type  308.E 308.E
von Neumann algebra of type  308.E 308.E
von Neumann algebra of type I 308.E
von Neumann algebra of type II 308.E
von Neumann algebra of type III 308.E
von Neumann algebra purely infinite 308.E
von Neumann algebra reduced 308.C
von Neumann algebra semifinite 308.E
von Neumann algebra structure theorem for, of type III 308.I
von Neumann condition 304.F
von Neumann density theorem 308.C
von Neumann inequality 251.M
von Neumann reduction theory 308.G
von Neumann selection theorem 22.F
von Neumann theorem, Weyl — 390.I
von Neumann uniqueness theorem 351.C
von Neumann — Halmos theorem 136.E
von Neumann — Morgenstern solution 173.D
von Neumann, J. 445
von Neumann, John(Johann)(1903-1957) 18.A 18.E 18.r 20 22.F 33.A—C 33.r 36.G 68.I 69.B 69.C 75.B 85.A 95 136.A 136.B 136.E 136.F 138.r 156.E 156.r 162 173.A 173.C 173.D 173.r 197.A 197.r 225.r 251.M 255.E 304.F 308.C 308.F 308.G 308.I 308.r 312.A 331.E 351.C 351.L 351.r 354.B 376 385.C 390.I 445
Vopenka, Petr(1935-) 33.r
Voronoi, Georgii Fedoseevich(1868-1908) 242.A
Vortex line 205.B
Vortex, vector field without 442.D
Vorticity 205.B
Vorticity theorem, Helmholtz 205.B
Voss, Heinz-Jurgen 186.r
Vossen theorem, Cohn — 111.I
Vranceanu, Gheorghe(1900-1979) 434.C
VSVO algorithm 303.E
Vulikh, Boris Zakharovich(1913-1978) 310.A
W-construction (of an Eilenberg — MacLane complex) 70.F
w-plane 74. D
w-point (of an entire function) 429.B
w-sphere 74.D
W-surface 111.I
W.B. process 136.E
Wada, Junzo(1927-) 164.C
Wada, Yasusi(Nei)(1787-1840) 230
Waelbroeck, Lucien(1929-) 36.M
Wage, M.L. 117.E
Wagner function 39.F
Wagner, Harvey Maurice(1931-) 307.r 408.r
Wagner, Herbert 39.F
Wagner, S.W. 95.r
Wagschal, Claude 321.G
Wagstaff, Samuel S. 14.L 145
Wahl, Jonathan Michael(1945-) 9.r
Wahlin, G.E. 145.r
Wait, R. 223.r 301.r 304.r
Waiting time 260.H
Waiting time distribution 307.C
Wakakuwa, Hidekiyo(1925-) 364.F
Wald theorem 399.H
Wald, Abraham(1902-50) 376 398.A 399.H 399.M 399.r 400.r 401.F 401.r 421.r
Waldhausen, Friedhelm(1938-) 65.E 235.B
Waldschmidt, Michel(1946-) 430.D 430.r
Wales, David B.(1939-) 151.I App.B Table
Walfisz(Val’fis), Arnold Z.(1892-1962) 4.D 123.D 220.B 242.r 295.D
Walker equation, Yule — 421.D
Walker metrics, Robertson — 359.E
Walker, Arthur G. 359.E
Walker, G. 421.D
Walker, M.R. 376.r
Walker, R.C. 425.r
Walker, Robert John(1909-) 9.r 15.B
Wall adiabatic 419.A
Wall diathermal 419.A
Wall group 114.J
Wall, Charles Terens Clegg(1936-) 114.B 114.F 114.H 114.J 114.K 114.r
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 -complete
-complete 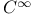 -manifold)
-manifold) 
