|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Ito K. — Encyclopedic Dictionary of Mathematics. Vol. 2 |
|
|
 |
| Предметный указатель |
Washington, A. 432.r
Washington, Lawrence Clinton 14.L 450.J
Washio, Yasutoshi 399.r
Washizu, Kyuichiro 271.r
Wasow, Wolfgang Richard 25.B r
Wassermann, Gordon 51.r
Watabe, Tsuyoshi 431.D
Watanabe, Kinji 323.J
Watanabe, Shinzo 44.E r r D F
Watanabe, Takeshi 260.J
Watari, Chinami 336.D
Water wave deep 205.F
Water wave long 205.F
Water wave shallow 205.F
Water wave(s) 205.F
Waternaux, Christine M. 280.r
Watson formula 39.E App. Table
Watson process Galton- 44.B
Watson process multi (k)-type Galton- 44.C
Watson transform 160.C 220.B
Watson — Nicholson formula App. A Table
Watson, George Leo 4.E 348.r
Watson, George Neville 39.E r Tables IVNTR
Watson, H.W. 44.B C r
Watt, J.M. 303.r
Wave Alfven 259
Wave capillary 205.F
Wave dispersive 446
Wave electromagnetic 446
Wave electromagnetic, theory of 130.B
Wave equation 325.A 446 App. Table
Wave expansion, partial 375.E 386.B
Wave fast 259
Wave front set 274.B 345.A
Wave front set analytic 274.D
Wave function 351.D
Wave function spheroidal 133.E
Wave gravity 205.F
Wave gravity, long 205.F
Wave guide 130.B
Wave longitudinal 446
Wave Mach 205.B
Wave number (of a sine wave) 446
Wave operator 375.B H
Wave operator generalized 375.B
Wave operator incoming 375.B
Wave operator modified 375.B
Wave operator outgoing 375.B
Wave partial 386.B
Wave partial, expansion 375.E 386.B
Wave plane 446
Wave polarized 446
Wave propagation 446
Wave scattering amplitude, partial 375.E
Wave shock 205.B 446
Wave sine 446
Wave sinusoidal 446
Wave slow 259
Wave spherical 446
Wave stationary 446
Wave steepness 205.F
Wave Stokes 205.F
Wave surface 446
Wave transverse 446
Wave water 205.F
Wave water, deep 205.F
Wave water, long 205.F
Wave water, shallow 205.F
Wave(s) 446
Wavelength (of a sine wave) 446
Wayland, Harold 298.r
WC group 118.D
Weak  topology 279.C topology 279.C
Weak (boundary component) 77.E
Weak Bernoulli process 136.E
Weak Bernoulli process very 136.E
Weak convergence (of a sequence of submodules) 200.J
Weak convergence (of operators) 251.C
Weak convergence (of probability measures) 341.F
Weak derivative 125.E
Weak dimension (of a module) 200.K
Weak extension (of a differential operator) 112.E F
Weak form of the boundary value problem (of partial differential equations) 304.B
Weak global dimension (of a ring) 200.K
Weak homotopy equivalence 202.F
Weak lacuna 325.J
Weak law of large numbers 395.B
Weak Lefschetz theorem 16.U
Weak minimum 46.C
Weak Mordell — Weil theorem 118.E
Weak Mordell- 118.E
Weak operator topology 251.C
Weak potential kernel 260.D
Weak solution 204.C 323.G 378.I
Weak solution, Hopf’s 204.C
Weak star topology 37.E 424.H
Weak topology (in a cell complex) 70.D
Weak topology (on a class of measures) 338.E
Weak topology (on a direct product space) 425.K
Weak topology (on a direct sum) 425.M
Weak topology (on a locally convex space) 424.H
Weak topology (on a normed linear space) 37.E
Weak topology (relative to the pairing <F, F>) 424.H
Weak topology hereditarily 425.M
Weak type (p, q), quasi-linear operator of 224.E
Weak* Dirichlet algebra 164.G
Weaker (equivalence relation) 135.C
Weaker (method of summation) 379.L
Weaker (topology) 425.H
Weaker (uniformity) 436.E
Weakly 136.C
Weakly 1-complete manifold 114.H
Weakly almost complex manifold 114.H
Weakly compact (linear operator) 68.M
Weakly compact cardinal number 33.E
Weakly continuous (function with values in a Banach space) 37.K
Weakly continuous representation (of a topological group) 69.B
Weakly dominated (statistical structure) 396.F
Weakly equivalent (transformations) 136.F
Weakly G-stationary (system of random variables) 395.I
Weakly hyperbolic linear (differential operator) 325.H
Weakly inaccessible (cardinal number) 33.E
Weakly inaccessible (ordinal number) 312.E
Weakly integrable 443.F
Weakly isomorphic (automorphisms) 136.E
Weakly measurable 443.B.I
Weakly mixing (automorphism) 136.E
Weakly modular 351.L
Weakly nonlinear differential equations 290.D
Weakly stationary process 395.A
Weakly stationary process of degree k 395.I
Weakly stationary random distribution 395.C
Weakly symmetric Riemannian space 412.J
Weakly wandering set 136.C
Weakly wandering under a group 136.F
Weakly, converge (in a normal linear space) 37.E
Weakly, converge (in a topological linear space) 424.H
Weakly, under a group 136.E
Weaver, W. 403.r
Web group 234.B
Weber differential equation 167.C App. Table
Weber formula App. A Table
Weber function 167.C App. Table
Weber — Hermite differential equation 167.C
Weber — Sonine formula App. A Table
Weber, Claude Alain 65.r
Weber, H.F. 39.G App. A Table
Weber, Heinrich 8.r 11.B r r Table IV 20
Weber, Wilhelm Eduard 363
Webster, Arthur Gordon 322.r
| Webster, Sidney M. 344.F
Wedderburn theorem (on commutativity of finite fields) 149.M
Wedderburn theorem (on simple algebras on a field) 29.E
Wedderburn theorem (on simple rings) 368.G
Wedderburn — Mal’tsev theorem (on algebras) 29.F
Wedderburn, Joseph Henry Maclagan 29.E F
wedge 125.V
Wedge infinitesimal 125.V
Wedge product (of derived functors) 200.K
Wedge theorem, edge of the 125.W
Wehrl, Alfred 212.r
Weierstrass 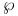 -function 134.F App. Table -function 134.F App. Table
Weierstrass approximation theorem 336.A
Weierstrass canonical form (of elliptic curves) 9.D
Weierstrass canonical form (of the gamma function) 174.A
Weierstrass canonical product 429.B
Weierstrass criterion for uniform convergence 435.A
Weierstrass elliptic functions 134.F App. Table
Weierstrass point 11.D
Weierstrass preparation theorem 21.E 370.B
Weierstrass sigma function 134.F
Weierstrass theorem (on compactness of subsets of 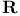 ) 355.D ) 355.D
Weierstrass theorem (on continuous functions on a compact set) 84.C
Weierstrass theorem (on essential singularities) 198.D
Weierstrass theorem (on expansion of meromorphic functions) 272.A
Weierstrass theorem (on transcendental entire functions) 124.B
Weierstrass theorem Bolzano- 140273.F
Weierstrass theorem Casorati- (on essential singularities) 198.D
Weierstrass theorem Lindemann- 430.D
Weierstrass theorem of double series 379.H
Weierstrass zeta function 134.F
Weierstrass — Enneper formula 275.A
Weierstrass — Stone theorem 168.B
Weierstrass, K. 447
Weierstrass, K. analytic function in the sense of 198.I
Weierstrass, Karl Theodor Wilhelm 9.D 11.B D E I N Q B C F D r Table
Weierstrass-type preparation theorem (for microdifferential operators) 274.F
Weight (in a barycenter) 7.C
Weight (of a covariant) 226.D
Weight (of a multiple covariant) 226.E
Weight (of a representation of a complex semisimple Lie algebra) 248.W
Weight (of a weighted homogeneous analytic function) 418.D
Weight (on a von Neumann algebra) 308.D
Weight equal, principle of 402.E
Weight extremal length with 143.B
Weight function (for the mean of a function) 211.C
Weight function (in numerical integration) 299.A
Weight function (in orthogonality) 317.A
Weight group (of a pair (T, K)) 92.C
Weight highest (of a representation of a complex simple Lie algebra) 248.W
Weight k, automorphic form of 32.B
Weight k, Fuchsian form of 32.B
weight k, Hilbert modular form of 32.B
Weight k, Siegel modular form of 32.F
Weight Kaellen — Lehmann 150.D
Weight lattice (of a pair (T, K)) 92.C
Weight m, automorphic form of 32.A
Weight w, invariant of 226.D
Weight(s) (of an automorphic form) 32.C
Weighted homogeneous (analytic function) 418.D
Weighted moving average 397.N
Weighting matrix 86.B
Weil cohomology 450.Q
Weil conjecture 450.Q
Weil conjecture Taniyama- 450.S
Weil domain 21.G
Weil group 6.E 450.H
Weil L-function 450.H
Weil measure 225.G
Weil number 3.C
Weil theorem Borel- 437.Q
Weil theorem Mordell- 118.E
Weil — Chatelet group 118.D
Weil, Andre 3.C E M r r H r r r C G D r r D E G r r r H M O-S r
Weinberg, B.L. 96.r
Weinberg, Louis 282.r
Weinberg, N. 425.U
Weinberg, Steven 132.C D r
Weinberg-Salam model, Glashow- 132.D
Weinberger, Hans Felix 323.r 327.r
Weingarten formula (for an isometric immersion) 365.C
Weingarten formula (in the theory of surface) 111.H App. Table
Weingarten surface 111.I
Weingarten, Leonhard Gottfried Johannes Julius 111.H I Table
Weinstein, Alan David 126.N 178.r
Weinstock, Robert 441.r
Weir, M.D. 425.r
Weirich formula App. A Table
Weisberger sum rule, Adler- 132.C
Weisberger, William I. 132.C
Weiss, Benjamin 136.E-G
Weiss, Edwin 14.r 200.r
Weiss, Guido Leopold 168.B 224.r
Weiss, Lionel 398.r
Weiss, Max L. 43.r
Weitsman, Allen W. 272.K r
Weitzenbock, Roland W. 226.C
Welch test 400.G
Welch, Bernard Lewis 400.G
Weldon, Edward J.,Jr. 63.r
Well-behaved 36.K
Well-chained (metric space) 79.D
Well-measurable 407.B
Well-measurable  -algebra 407.B -algebra 407.B
Well-ordered set 311.C
Well-ordering 311.C
Well-ordering theorem 34.B
Well-posed (initial value problem) 321.E
Well-posed (martingale problem) 115.C
Well-posed (problems for partial differential equations) 322.A
Wells, Raymond O’Neil.Jr. 164.K 232.r 344.D E
Welsh, James Anthony Dominic 66.r
Wendroff scheme, Lax- 304.F
Wendroff, Burton 304.F
Wentzel, Gregor 25.B
Wermer, John 164.F G I K r
West, James Edward 382.D
Westlake, Joan R. 302.r
Westwater, Michael John 146.A
Wets, M.J. 408.r
Weyl (of a semisimple Lie algebra) 248.R
Weyl canonical basis 248.P
Weyl chamber 13.J 248.R
Weyl chamber positive 248.R
Weyl character formula (on representation of compact Lie groups) 248.Z
Weyl conformal curvature tensor 80.P
Weyl criterion 182.H
Weyl form 351.C
Weyl form of the CCRs (canonical commutation relations) 337.A
Weyl formula 323.M
Weyl group (of a BN pair) 13.R
Weyl group (of a complex semisimple Lie algebra) 248.R
Weyl group (of a connected algebraic group) 13.H
Weyl group (of a Coxeter complex) 13.R
Weyl group (of a root system) 13.J
Weyl group (of a symmetric Riemannian space) 413.F
Weyl group affine (of a symmetric Riemannian space) 413.I
Weyl group k- 13.Q
Weyl integral formula 225.I
Weyl lemma 112.D
Weyl theorem (on Lie algebras) 248.F
Weyl theorem Cartan- 248.W
Weyl theory Peter- (on compact groups) 69.B
Weyl theory Peter- (on compact Lie groups) 249.U
Weyl — Stone — Titchmarsh — Kodaira theory 112.O
Weyl — von Neumann theorem 390.I
Weyl, Claus Hugo Hermann 7.r 9.r 11.B 13.H J Q R I N O r r H r P R W Z r V r G M r E r J Table
Weyl, Fritz Joachim 21.N r r
Weyl, H. 448
Weyrich, Rudolf 39.r App. A Table
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 topology
topology  -function
-function  )
)  -algebra
-algebra