|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Ito K. — Encyclopedic Dictionary of Mathematics. Vol. 2 |
|
|
 |
| Предметный указатель |
Value exceptional, Nevanlinna 272.E
Value exceptional, Picard 272.E
Value expectation (of an observable) 351.B
Value expected (of a random variable) 342.C
Value function 108.B 405.A
Value gap (of a point on a Riemann surface) 11.D
Value group (of a multiplicative valuation) 439.C
Value group (of an additive valuation) 439.B
Value initial (for ordinary differential equations) 316.A
Value initial (for partial differential equatons) 321.A
Value initial (for stochastic differential equations) 406.D
Value limit (of a mapping) 87.F
Value mean (of a function on a compact group) 69.A
Value mean (of a weakly stationary process) 395.C
Value most probable 401.E
Value principal (of inverse trigonometric functions) 131.E
Value principal (of log z) 131.G
Value principal, Cauchy (of an improper integral) 216.D
Value principal, Cauchy (of the integral of a function in 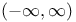 ) 216.E ) 216.E
Value proper (of a boundary value problem) 315.B
Value proper (of a linear mapping) 269.L
Value proper (of a linear operator) 390.A
Value proper (of a matrix) 269.F
Value range of (of a meromorphic function) 62.A
Value regular 105.J
Value sample 396.B
Value sample characteristic 396.C
Value Shapley 173.D
Value singular 302.A
Value singular, decomposition (SVD) 302.E
Value starting 303.E
Value stationary (of a function) 106.L
Value true, of parameter 398.A
Value truth (of a formula) 411.E
Value(s) (of an infinite continued fraction) 83.A
van Beijeren, Henk 402.G
van Ceulen, Ludolf 332
Van Daele, Alfons 308.H
van Dantzig, D. 109 434.C
van den Berg, Franciscus Johannes 19.B
van der Corput, Johannes Gualtherus 4.C 182.H 242.A
van der Pol differential equation 290.C
van der Pol, Balthasar 240.r 290.C
van der Waerden test 371.C
van der Waerden — Bortolotti covariant derivative 417.E
van der Waerden, Bartel Leendert 8.* r r
van Hove sense, limit in 402.G
van Hove, Leon Charles Prudent 351.K 402.G
van Kampen theorem (on fundamental groups) 170
van Kampen, Egbertos R. 170
Van Moerbeke, Pierre 287.C
van Roomen, Adriaan 444
van Schooten, Frans 444.r
Vandermonde determinant 103.G
Vandermonde, Alexandre Theophile 103.G 190.Q
Vandiver conjecture 14.L
Vandiver, Harry Shultz 14.L 145.* r
Vanishing cocycle 16.U
Vanishing cycle 418.F
Vanishing theorem (on compact complex manifolds) 194.G
Vanishing theorem Kodaira 232.D
Varadarajan, Veeravalli Seshadri 249.r
Varadhan, Sathamangalam Ranga Ayyangar Srinivasa 115.C D r D r
Varadier, M. 443.A
Varaiya, Pravin P. 86.D 108.B 292.F
Varchenko, A.N. 418.r
Varga, Otto 152.C
Varga, Richard Steven 302.r
Variability, measure of 397.C
Variable (of a polynomial) 369.A
Variable artificial 255.C
Variable auxiliary 373.C
Variable basic 255.A
Variable bound 411.C
Variable canonical (in analytical dynamics) 271.F
Variable change of (in integral calculus) 216.C
Variable complex 165.C
Variable complex, theory of functions of 198.Q
Variable component (of a linear system) 15.C 16.N
Variable dependent 165.C
Variable differential (of a differential polynomial) 113
Variable endogenous 128.C
Variable exogenous 128.C
Variable explanatory 403.D
Variable hidden, theories 351.L
Variable independent 165.C
Variable individual 411.H
Variable inner 25.B
Variable lagged 128.C
Variable method, discrete 303.A
Variable object 411.G
Variable outer 25.B
Variable predetermined 128.C
Variable predicate 411.G H
Variable proposition 411.E
Variable random 342.C
Variable random, 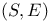 -valued 342.C -valued 342.C
Variable random,  -valued 342.C -valued 342.C
Variable random, independent 342.C
Variable random, joint 342.C
Variable random, n-dimensional 342.C
Variable real 165.C
Variable sampling inspection by 404.C
Variable separation of 322.C
Variable slack 255.A
Variable state 127.A
Variable(s) 165.C
Variable-step variable-order (VSVO) algorithms 303.E
Variance (of a probability distribution) 341.B
Variance (of a random variable) 342.C
Variance (of univariate quantitative data) 397.C
Variance analysis of 400.H 403.D
Variance between-group 397.L
Variance generalized 280.E 397.J
Variance matrix 341.B
Variance multivariate analysis of 280.B
Variance population 396.C
Variance sample 396.C
Variance sample generalized 280.E
Variance uniformly minimum unbiased estimator 399.C
Variance within-group 397.L
Variance-covariance matrix 341.B 397.J
Variate canonical 280.E
Variate fixed 403.D
Variation calculus of 46
Variation calculus of, classical theory of 46.C
Variation calculus of, conditional problems in 46.A
Variation calculus of, fundamental lemma in 46.B
Variation coefficient of 397.C
Variation curve 178.A
Variation first 46.B
Variation first, formula 178.A
Variation geodesic 178.A
Variation lower (of a set function) 380.B
Variation negative (of a mapping) 246.H
Variation negative (of a real bounded function) 166.B
Variation of constants, Lagrange’s method of 252.D
Variation of constants, method of 55.B 252.I
Variation of parameters, Lagrange method of 252.D
Variation of parameters, method of App. A Table
Variation one-parameter 178.A
Variation positive (of a mapping) 246.H
Variation positive (of a real bounded function) 166.B
Variation proper 279.F
Variation quadratic, process 406.B
Variation second formula 178.A
Variation total (of a finitely additive vector measure) 443.G
Variation total (of a mapping) 246.H
Variation total (of a real bounded function) 166.B
Variation total (of a set function) 380.B
| Variation upper 380.B
Variation vector field 178.A
Variation(s) (of an integral) 100.E
Variational derivative 46.B
Variational equation 316.F 394.C
Variational formula, constant 163.E
Variational inequality 440
Variational inequality of evolution 440.C
Variational inequality stationary 440.B
Variational method 438.B
Variational principles (in ergodic theory) 136.G
Variational principles (in statistical mechanics) 340.B 402.G
Variational principles (in the theory of elasticity) 271.G
Variational principles for the topological pressure 136.H
Variational principles with relaxed continuity requirements 271.G
Variational principles(s) 441
Variational problem, Gauss 338.J
Variety (algebraic variety) 16.A
Variety (of block design) 102.B
Variety Abelian 3
Variety Abelian, isogeneous 3.C
Variety Abelian, polarized 3.G
Variety Abelian, simple 3.B
Variety abstract 16.C
Variety abstract algebraic 16.C
Variety affine 16.A
Variety affine algebraic 16.A
Variety Albanese 16.P
Variety Albanese (of a compact Kahler manifold) 232.C
Variety algebraic 16
Variety algebraic group 13.B
Variety almost all points of a 16.A
Variety Brieskorn 418.D
Variety characteristic (of a microdifferential equation) 274.G
Variety Chow 16.W
Variety complex algebraic 16.T
Variety function on a 16.A
Variety generalized Jacobian 9.F 11.C
Variety group 13.B 16.H
Variety irreducible 16.A
Variety Jacobian 9.E 11.C 16.P
Variety Landau 146.C
Variety Landau — Nakanishi 146.C 386.C
Variety linear (in an  -module) 422.L -module) 422.L
Variety linear, linearly compact 422.L
Variety minimal 275.G
Variety nonsingular 16.F
Variety normal 16.F
Variety normal algebraic 16.F
Variety Picard 16.P
Variety Picard (of a compact Kahler manifold) 232.C
Variety prealgebraic 16.C
Variety product algebraic 16.A
Variety projective 16.A
Variety projective algebraic 16.A
Variety quasi-affine algebraic 16.C
Variety quasiprojective algebraic 16.C
Variety rational 16.J
Variety rational function on a 16.A
Variety reducible 16.A
Variety Schubert 56.E
Variety smooth 16.F
Variety strict Albanese 16.P
Variety toric 16.Z
Variety unirational 16.J
Variety Zariski topology of a 16.A
Varifold 275.G
Varopoulos, Nicholas Theodoros 192.U
Varopoulos, T. 17.C 267.r
Varouchas, J. 232.C
Varshamov — Gilbert — Sacks bound 63.B
Varshamov, Rom Rubenovich 63.B
Vasilesco, Florin 120.D
Vaughan, Robert Charles 123.E
Vaught, Robert L. 276.D F
Veblen, Oswald 90.r 109.* r r
Vector (in a linear space) 256.A
Vector algebra App. A Table
Vector analysis and coordinate systems App. A Table
Vector analytic 437.S
Vector bundle 147.F
Vector bundle (algebraic) 16.Y
Vector bundle ample 16.Y
Vector bundle complex 147.F
Vector bundle cotangent 147.F
Vector bundle dual 147.F
Vector bundle indecomposable 16.Y
Vector bundle normal 105.L
Vector bundle normal k- 114.J
Vector bundle quaternion 147.F
Vector bundle semistable 16.Y
Vector bundle stable 16.Y 237.B
Vector bundle stably equivalent 237.B
Vector bundle tangent 105.H 147.F
Vector characteristic (of a linear mapping) 269.L
Vector characteristic (of a linear operator) 390.A
Vector characteristic (of a matrix) 269.F
Vector coherent 377.D
Vector collinear 442.A
Vector column 269.A
Vector contravariant 256.J
Vector coplanar 442.A
Vector covariant 256.J
Vector cyclic (of a representation space of a unitary representation) 437.A
Vector effect 102.A
Vector eigen-(of a linear mapping) 269.L
Vector eigen-(of a linear operator) 390.A
Vector eigen-(of a matrix) 269.F
Vector eigen-, generalized 390.B
Vector error 102.A
Vector field (in a 3-dimensional Euclidean space) 442.D
Vector field (on a differentiable manifold) 105.M
Vector field Anosov 126.J
Vector field Axiom A 126.J
Vector field basic 80.H
Vector field contravariant 105.O
Vector field covariant 105.O
Vector field differentiation of App. A Table
Vector field formal 105.AA
Vector field fundamental 191.A
Vector field G- 237.H
Vector field Hamiltonian 126.L 219.C
Vector field holomorphic 72.A
Vector field integral of App. A Table
Vector field irrotational 442.D
Vector field Killing 364.F
Vector field Lagrangian 126.L
Vector field lamellar 442.D
Vector field Morse — Smale 126.J
Vector field of class  105.M 105.M
Vector field solenoidal 442.D
Vector field variation 178.A
Vector field without source 442.D
Vector field without vortex 442.D
Vector fixed 442.A
Vector flux (through a surface) 442.D
Vector four- 359.C
Vector four-, energy-momentum 258.C
Vector free 442.A
Vector free vacuum 150.C
Vector function, measurable 308.G
Vector fundamental (in a vector space) 442.A
Vector group 422.E
Vector horizontal 80.C
Vector independent 66.F
Vector integral 443.A
Vector invariant 226.C
Vector lattice 310.B
Vector lattice  -complete 310.C -complete 310.C
Vector lattice Archimedean 310.C
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 )
) 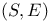 -valued
-valued  -valued
-valued  -module)
-module) 
 -complete
-complete