|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Ito K. — Encyclopedic Dictionary of Mathematics |
|
|
 |
| Предметный указатель |
Boundary value problem third (for elliptic differential equations) 323.F
Boundary value problem third (for harmonic functions) 193.F
Boundary value problem two-point (of ordinary differential equations) 315.A
Boundary value problem weak form of the (of partial differential equations) 304.B
Bounded  -operator 356.B -operator 356.B
Bounded (half-plane) 155.B
Bounded (in an affine space) 7.D
Bounded (metric space) 273.B
Bounded (ordered set) 311.B
Bounded (set in a topological linear space) 424.F
Bounded (set of real numbers) 87.B
Bounded (torsion group) 2.F
Bounded (vector lattice) 310.B
Bounded (vector measure) 443.G
Bounded approximation property (of a Banach space) 37.J
Bounded automaton, linear deterministic 31.D
Bounded automaton, linear nondeterministic 31.D
Bounded domain divisible 284.F
Bounded domain homogeneous 384.A 412.F
Bounded domain irreducible symmetric 412.F
Bounded domain sweepable 284.F
Bounded domain symmetric 412.F
Bounded from above (for real numbers) 87.B
Bounded from above (in an ordered set) 311.B
Bounded from below (for a filtration) 200.J
Bounded from below (for a spectral sequence) 200J
Bounded from below (for real numbers) 87.B
Bounded from below (in an ordered set) 311.B
Bounded functions 43.A
Bounded linear operator 37.C
Bounded matrix 269.K
Bounded mean oscillation (BMO) 168.B
Bounded metric space, totally 273.B
Bounded motion 420.D
Bounded order 310.B
Bounded quantifier 356.B
Bounded relatively 331.B
Bounded set in a locally convex space 424.F
Bounded set in a metric space 273.B
Bounded set in an affine space 7.D
Bounded set totally (in a metric space) 273.B
Bounded star body 182.C
Bounded T- 311.B
Bounded uniform space locally totally 436.H
Bounded uniform space totally 436.H
Bounded variation function of 166.B
Bounded variation in the sense of Tonelli 246.C
Bounded variation intcgrable process of 406.B
Bounded variation mapping of 246.H
Bounded variation set function of 380.B
Bounded variation vector measure of 443.G
Bounded, essentially (measurable function) 168.B
Bounded, totally 273.B
Boundedly complete  -ficld 396.E -ficld 396.E
Boundedness principle, upper (in potential theory) 388.C
Boundedness theorem, uniform 37.H
Boundedness, abscissa of (of a Dirichlet series) 121.B
Bouquet 202.F
Bouquet differential equation, Briot — 288.B 289.B
Bouquet formulas (on space curves) 111.F
Bouquet, Jean-Claude(1819-1885) 107.A 111.F 288.B 289.B
Bourbaki, Frechet space in the sense of 424.I
Bourbaki, Nicolas 8 13.r 20.r 22.r 34.r 35.r 60.r 61.r 67.r 74.r 84.r 87.r 88.r 103.r 105.r 106.r 122.r 131.r 135.r 149.r 162 168.C 172.r 187.r 216.r 221.r 225.r 248.r 249.r 256.r 265.r 266.r 267.r 270.r 277.r 284.r 310.I 311.r 312.r 337.r 348.r 355.r 360.r 362.r 368.r 379.r 381.r 409.r 423.r 424.I 424.r 425.S 425.W 425.Y 425.CC 425.r 435.r 436.r 443.A
Bourgin, David G. 201.r
Bourgne, Robert 17l.r
Bourguignon, Jean-Pierre(1947-) 80.r 364.r
Bourion, Georges 339.E
Boussinesq equation 387.F
Boussinesq, Joseph(1842-1929) 387.B 387.F
Boutroux, Pierre Leon(1880-1922) 265.r 288.B 288.C 288.r
Bowen, Rufus(1947-1978) 126.A 126.J 126.K 126.r 136.C 136.G 136.r 234.r
Bowman, Frank(1891-1983) 39.r
Box topology 425.K
Box, George E.P.(1919-) 102.r 128.r 301.L 371.A 421.G 421.r
Boxes 140
Boyle, James M. 298.r
Brachistochrone 93.H
Bracket 105.M
Bracket Lagrange 84.A 324.D
Bracket Poisson (of two functions) 105.M
Bracket Poisson (of two vector fields) 271.F 324.C 324.D
Bracket product (in a Lie algebra) 248.A
Bracket Toda 202.R
Bradley — Terry model 346.C
Bradley, G.J. 92.r
Bradley, Ralph Allan(1923-) 346.C
Brahmagupta(598-660) 118.A 209
Braid group 235.F
Braid(s) 235.F
Braid(s) closed 235.F
Bram, Joseph(1926-) 291.r
Brams, Steven John(1940-) 173.r
Branch (of a curve of class 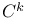 ) 93.G ) 93.G
Branch (of a graph) 282.A
Branch (of an analytic function) 198.J
Branch and bound methods 215.D
Branch divisor (in a covering of a nonsingular curve) 9.I
Branch point (of a covering surface) 367.B
Branch point (of an ordinary curve) 93.C
Branch point algebraic (of a Riemann surface) 367.B
Branch point fixed (of an algebraic differential equation) 288.A
Branch point logarithmic (of a Riemann surface) 367.B
Branch point movable (of an algebraic differential equation) 288.A
Branch source 282.C
Branched minimal immersion 275.B
Branched minimal surface 275.B
Branching Markov process 44.E
Branching processes 44 342.A
Branching processes age-dependent 44. E
Branching processes continuous state 44.E
Branching processes Galton-Watson 44.B
Branching processes Markov 44.D
Branching processes multitype Markov 44.E
Brandt law 241.C
Brandt, Heinrich(1886-1954) 190.P 241.C
Branges, Louis de(1932-) 176.K 438.C
Bratteli, Ola(1946-) 36.H 36.K 36.r 308.r 402.G 402.r
Brauer character (of a modular representation) 362.I
Brauer group (of a commutative ring) 29.K
Brauer group (of algebra classes) 29.E
Brauer theorem 450.G
Brauer, Richard Dagobert(1901-77) 14.E 27.D 27.E 29.E 29.F 29.K 118.C 151.J 151.r 362.G 362.I 362.r 427.B 450.D 450.G 450.L
Braun, Hel(1914-) 32.H 122.E 231.r
Brauner, Karl 418.r
Bravais class 92.B
Bravais group 92.B
Bravais lattice 92.B
Bravais type 92.B
Bravais type simple 92.E
Bravais, Auguste(1811-63) 92.B 92.F App.B Table
Breadth (of an oval) 89.C
Bredikhin, Boris Maksimovich(1920-) 123.E
Bredon, Glen E.(1932-) 383.r 431.r
Breiman, Leo(1928-) 260.r 342.r
Brelot solution, Perron — (of Dirichlet problem) 120.C
Brelot solution, Perron — Wiener — (of Dirichlet problem) 120.C
Brelot, Marcel(1903-) 120.C 120.E 120.r 193.J 193.L 193.N 193.U 207.C 338.G 338.H
Bremermann, Hans-Joachim(1926-) 21.D 21.I
Bremmer, Hendricus(1904-) 240.r
Brent, Richard Peirce(1946-) 123.B 142.A 450.I
Breuer, Manfred 390.J
Brewster, Sir David(1781-1868) 283.r
Brezis, David 88.E
Brezis, Haim(1944-) 88.E 162.* 162.r 286.C 286.X 440.r
Brianchon theorem in projective geometry 343.E
Brianchon theorem on conic sections 78.K
Brianchon, Charles Julien(1785-1864) 78.K 343.E
Bridge, Brownian 250.F 374.E
Brieskorn variety 418.D
Brieskorn, Egbert(1936-) 16.r 418.C 418.D 418.r App.A Table
| Brigham, E.Oran(1940-) 142.r
Brill — Noether number 9.E
Brill, Alexander Wilhelm von(1842-1935) 9.E 9.r 11.B 11.r 12.B
Brillinger, David Ross(1937-) 421.r
Brillouin, Leon Nicolas(1889-1969) 25.B 446.r
Brin, Matthew G. 136.G
Briot — Bouquet differential equation 288.B 289.B
Briot, Charles Auguste Albert(1817-1882) 107.A 288.B 289.B
Broadbent, S.R. 340.r
Brocker, Theodor 51.r
Broderick, Norma 92.r
Brodskii, Mikhail Samoilovich(1913-) 251.r 390.H
Brody, Robert 21.O
Broken line 155.F
Broken symmetry 132.C
Bromwich integral 240.D 322.D App. Table
Bromwich, Thomas John l’Anson(1875-1929) 240.D 322.D 379.r App.A Table
Bronshtein, M.D. 325.I
Bros, Jacques(1934-) 150.D 274.D 247.I 386.B 386.C
Brosilow, C.B. 303.r
Brouncker, Lord William(1620-1684) 332
Brouwer fixed-point theorem 153.B
Brouwer mapping theorem 99.A
Brouwer theorem on the invariance of domain 117.D
Brouwer, Dirk(1902-1966) 55.r
Brouwer, Luitzen Egbertus Jan(1881-1966) 65.G 79.D 99.A 117.A 117.D 117.r 153.B 156.A 156.C 202.B 305.A 426
Browder — Livesay invariant 114.L
Browder, Andrew(1931-) 164.r
Browder, Felix Earl(1927-) 112.F 112.Q 286.C 286.X 286.r 323.H
Browder, William(1934-) 114.J 114.L 114.r 427.B
Brown — Shield—Zeller theorem 43.C
Brown, Edgar H., Jr.(1926-) 202.T
Brown, Harold 92.F
Brown, Lawrence David(1940-) 396.r
Brown, Lawrence G.(1943-) 36.J 390.J 390.r
Brown, Leon 43.G
Brown, Morton(1931—) 65.G
Brown, Robert Freeman(1935-) 153.r
Brown, Robert(1773-1858) 5.D 45.A—C 45.F 45.I 176.C 176.I 250.F 406.B 406.G
Brown, Scott W.(1937-) 251.L
Brownian bridge 250.F 374.E
Brownian functional 176.I
Brownian motion 5.D 45 342.A
Brownian motion  - 45.B 406.B - 45.B 406.B
Brownian motion d-dimensional 45.C
Brownian motion on Lie groups 406.G
Brownian motion Ornstein — Uhlenbeck 45.I
Brownian motion right invariant 406.G
Brownian motion space-time 45. F
Brownian motion with an N-dimensional time parameter 45.I
Brownlee, John(1868-1927) NTR
BRS transformation 150.G
Bruck — Ryser — Chowla theorem 102.E
Bruck, Richard Hubert(1914-) 190.P 190.r 241.D
Bruhat decomposition (of an algebraic group) 13.K
Bruhat decomposition relative 13.Q
Bruhat, Francois(1929-) 13.K 13.Q 13.R 437.O 437.EE
Brumer, Armand 182.r 450.J
Brun theorems, Poincare — 420.A
Brun — Titchmarsh theorem 123.D
Brun, Viggo(1885-1978) 4.A 4.C 123.D 123.E
Brune, O. 282.r
Brunei, Antoine 136.C
Brunn 89.E
Brunovsky, Pavol 126.M
Bruns, Heinrich(1848-1919) 126.A 420.A
Brunschvicg, Leon(1869-1944) 329.r
Bruter, Claude Paul(1937-) 281.r
Buchholz, Herbert(1895-1971) 167.r
Buchner, Michael Anthony(1947-) 126.L
Buchsbaum, David Alvin(1929-) 284.G
Buck, R.Creighton(1920-) 43.F 43.r 106.r
Bucur, Ion(1930-1976) 52.r
Bucy filter, Kalman — 86.E 405.G
Bucy, Richard S.(1935-) 86.E 95.r 405.G 405.r
Bueckner, Hans 217.r
Buehlmann, Hans(1930-) 214.r
Buelow, Rolf 92.F
Buerger, Martin J. 92.r
Buergi,Joost(1552-1632) 265
Buffon, Georges Louis Leclerc, Comte de(1707-1788) 218.A 342.A 385.C
Buhler, Joe P. 450.G
Building 130.R 343.I
Bulirsch, Roland(1932-) 303.F
Bulk viscosity, coefficients of 205.C
Bundle group (of a fiber bundle) 147.B
Bundle mapping (map) 147.B
Bundle of homomorphisms 147.F
Bundle of p-vectors 147.F
Bundle space (of a fiber bundle) 147.B
Bundle(s)  237.F 237.F
Bundle(s) canonical 147.F
Bundle(s) complex conjugate 147.F
Bundle(s) complex line 72.F
Bundle(s) complex line, determined by a divisor 72.F
Bundle(s) conormal 274.E
Bundle(s) coordinate 147.B
Bundle(s) coordinate, equivalent 147.B
Bundle(s) cotangent 147.F
Bundle(s) cotangential sphere 274.E
Bundle(s) dual 147.F
Bundle(s) fiber 147.B
Bundle(s) fiber, associated 147.D
Bundle(s) fiber, complex analytic 147.O
Bundle(s) fiber, of class C 147.O
Bundle(s) fiber, orientable 147.L
Bundle(s) fiber, real analytic 147.O
Bundle(s) flat F- 154. B
Bundle(s) foliated 154.B 154.H
Bundle(s) frame, orthogonal 364.A
Bundle(s) frame, tangent orthogonal n- 364.A
Bundle(s) G- 147.B
Bundle(s) Hopf 147.E
Bundle(s) induced 147.G
Bundle(s) line 147.F
Bundle(s) Maslov 274.C
Bundle(s) n-sphere 147.K
Bundle(s) n-universal 147.G
Bundle(s) normal 105.C 114.B 154.B 154.E 364.C 274.E
Bundle(s) normal block 147.Q
Bundle(s) normal k-vector 114.J
Bundle(s) normal sphere 274.E
Bundle(s) principal 147.C
Bundle(s) principal fiber 147.C
Bundle(s) principal, associated 147.D
Bundle(s) product 147.E
Bundle(s) q-block 147.Q
Bundle(s) quotient 16.Y 147.B
Bundle(s) spin 237.F
Bundle(s) sub- 16.Y 147.F
Bundle(s) tangent 105.H 147.F 154.B 286.K
Bundle(s) tangent r-frame 108.H 147.F
Bundle(s) tangential sphere 274.E
Bundle(s) tautological line 16.E
Bundle(s) tensor 147.F
Bundle(s) unit tangent sphere 126.L
Bundle(s) universal 147.G 147.H
Bundle(s) vector 16.Y 147.F
Bundle(s) vector, ample 16.Y
Bundle(s) vector, complex 147.F
Bundle(s) vector, cotangent 147.F
Bundle(s) vector, dual 147.F
Bundle(s) vector, indecomposable 16.Y
Bundle(s) vector, normal 105.L
Bundle(s) vector, quaternion 147.F
Bundle(s) vector, quotient 16.Y
Bundle(s) vector, semistable 16.Y
Bundle(s) vector, stable (on algebraic varieties) 16.Y
Bundle(s) vector, stable (on topological spaces) 237.B
Bundle(s) vector, stably equivalent 237.B
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 -operator
-operator  -ficld
-ficld  )
)  -
- 