Complexes L.44 47 M.2 4
Complexes and congruences, spherical of 2nd degree, their circles and cyclides J.99
Complexes and spherical complexes, ap. to linear p.d.e M.5
Complexes in combinations and permutationa A.21
Complexes of 1st and 2nd degrees and linear congruences An.76 L.51 M.2 9 N.85 trZ.27
Complexes of 2nd degree G.8 17 18 cnJ.93 M.7 N.726
Complexes of 2nd degree of right lines which cut two quadrics harmonically M.23
Complexes of 2nd degree with a centre L.82
Complexes of axes of a quadric N.83
Complexes of nth degree, singularities M.12
Complexes, Linear N.85 Z.18
Complexes, linear, of an in-conic of a quadrilateral G.21
Complexes, quadratic ray-& web-complexes J.98
Complexes, tetrahedral in point space Z.22
Composite functions of a higher order G.2
Composite numbers for construction of factor tables A.45
Composite numbers, "Compteurs logarithmiques" C.40
Composite numbers, groups of J.78 LM.8 Me.79
Concavity and convexity 5174
Concentric circles LM.14
Concentric circles, 3 quadrics, intersection of E.39
Conchoid 5320 A.55 N.43
Concomitants of a ternary cubic AJ.4
Concurrent lines and collinear points 967—976 A.69
Concurrent lines and collinear points, th. on conic and triangle E.35
Concyclic conicoids 995 Q.11
Cone 1150—1159 6043 A.16 L.61 Me.62
Cone and cylinder, superfices, tr An.57
Cone and sphere 5652 thsMe.64
Cone, general d.e of E.18
Cone, intersection of two N.64
Cone, oblique eq 5598 J.2 Me.80
Cone, sections of 1150—1159
Cone, superfices of oblique frustrum J.2
Cone, through m points and touching 6-m lines LM.4
Cone, volume of frustrum Ac.41 N.13
Configuration of 16 points and 16 planes J.86
Configuration of 16 points and 16 planes,  and unicursal curves M.21 and unicursal curves M.21
Confocal conics 4550—4558 5007 tg.e J.54 LM.12 13 Me.66 68 73 N.80 Q.10 TE.24 Z.3
Confocal conics, Graves' theorem 4555
Confocal conics, Graves' theorem, Griffith's ext. LM.15
Confocal conics, tangents of 4555
Confocal quadrics 5656—5672 A.3 CD.4 5 9 G.16 M.18 thMe.72 Q.3
Confocal quadrics, relation to curves and cones CD.4 9
Confocal quadrics, volume bounded by three and the coord. planes A.36
Confocal surfaces Me.66
Conformable figures A.59 LM.10 M.19 Z.17
Congruences C.51 88 thJ.19 apJ.19 thMe.75 N.50 P.61
Congruences and irreducible modular functions C.61
Congruences and trigonometrical functions J.19
Congruences of 1st degree A.32
Congruences of 1st degree in several unknowns L.59
Congruences of 1st degree with composite modulus E.30
Congruences of 1st degree, sol. by binomial factorial Mem.44
Congruences of 1st degree, transformation of modulus Mel.2 N.59
Congruences of 2nd degree C.62 Mem.31
Congruences of 2nd degree, reduced forms C.74
Congruences of 3rd order and class LM.16
Congruences, 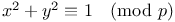 J.19 J.19
Congruences, 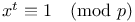 J.31 J.31
Congruences, binomial AJ.3 C.61
Congruences, binomial, classification of roots C.63
Congruences, binomial, expon. to base 3 Mel.4
Congruences, Cremonian LM.14
Congruences, higher, with real prime modulus An.83 J.31 54 99
Congruences, irreducible J.40
Congruences, linear LM.4
Congruences, linear, of circles in space C.93
Congruences, numerical An.60
Congruences, numerical, multiplication of 61
Congruences, resultant of systems of linear C.88
Congruent divisors of a number, no. of A.37
Conical functions M.18 19
Conical surfaces 5590 A.63 Ac.5 LM.3 M.3
Conical surfaces through 6 points, locus of vertex J.92
Conicoids 5599 A.48 Q.tg.c q.c t.c
Conicoids, 50-point Me.66
Conics 4032—5030 A.1 5 17 31 32 60 68 C.83 G.1 2 3 21 J.20 30 32 45 69 86 M.17 19 N.42 43 44 45 71 75 82 P.62 Q.8 tg.c Z.18 21 23
Conics and circle, intersection th 1263 A.59 N.64
Conics and companion quadric An.60 JP.7
Conics and polar, Desarques' th N.64
Conics and quadrics A.30 L.42 N.58 66 ths
Conics and quadrics, geo interpretation of variables N.66
Conics from oblique cone L.38
Conics of 14 points Me.66
Conics of 8 points J.65
Conics of 9 points A.43 G.1
Conics of 9 points and 9 lines G.7 8
Conics of Apollonius L.58
Conics under 5 conditions 4822—4843 L.10 59
Conics under 6 conditions, cn C.59
Conics under 7 conditions in space C.61
Conics with Jacobian=0 M.15
Conics, 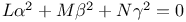 4755 4765 Me.62 4755 4765 Me.62
Conics, 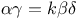 and derived equations 4697—4719 Q.4 and derived equations 4697—4719 Q.4
Conics, 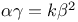 or or 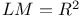 4699 4784 N.44 4699 4784 N.44
Conics, 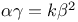 or or 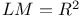 , , 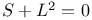 , &c. 4707 , &c. 4707
Conics, angles connected with 4375
Conics, anharmonic correspondents, problem of 5 conics and 5 lines N.56
Conics, arcs similar to N.44
Conics, areas of 4688 6097—6121 N.46 t.cQ.2 see
Conics, as curves in space tr A.37 M.64
Conics, auxiliary circle 1160
Conics, centre, coordinates of 402 4267 t.c t.c tg.eq ths prs
Conics, centre, locus of 4520 5028
Conics, chord of contact 4124 4281 4699—4721
Conics, chords of 4315 4322 p.c p.c Me.66 see
Conics, chords of, cutting an ellipse at a given angle E.28
Conics, chords of, intersecting 1214 4317
Conics, chords of, moving round an ellipse A.43 44 E.22
Conics, collinear relation to circle Z.1
Conics, conjoint lines of L.38
Conics, conjugate diameters 1193—1213 4346 ths CM.1 L.37 N.42 ths 69 Q.3
Conics, conjugate diameters, parallelogram on 1194 4367
Conics, conjugate diameters, relation to ellipse when equal A.18
Conics, conjugate points Q.8
Conics, construction of 1245 4822 A.28 43 E.29 Q.4 N.59 73
Conics, construction of, from conj. diameters 1253 A.52
Conics, construction of, with help of circle of curv. A.24
Conics, contact of 4527—4533 A.1 60 C.78 Pr.34
Conics, contact of, 4-pointic with a quartic M.12
Conics, contact of, 5-pointic 5190 5191 C.78 E.5 23 J.21 P.59
Conics, contact of, at 2 points Q.3 N.74
Conics, contact of, do. with each of 2 conics or circles 4803—4806 CD.5 6 E.31 34
Conics, contact of, with surfaces C.91 P.70 74
Conics, convexity from focal property N.56
Conics, criterion of Mobius J.89
Conics, criterion of species 4464—4477 t.c 5000
Conics, curvative centre and radius of 4534—4549 geo thsMe.73 N.79 85
Conics, curvative, chord of 1259 1264 Q.6
Conics, curvative, chord of, locus of mid-point A.70
Conics, curvative, geo cn 1265 A.17
Conics, definitions 1160
Conics, degenerate forms LM.2
Conics, diameters 1214 1235 eq cn N.65
Conics, director circle geo 1217 eq o.c LM.13 N.79
Conics, directrices 1160 trA.63 LM.11
Conics, eccentric values of coordinates 4275 CM.4
Conics, eccentricity 1151 4200
Conics, elementary formula G.9
Conics, ellipse and hyperbola 4250—4296
Conics, equation in  and p Q.13 and p Q.13
Conics, equations of 4251 4273 p.c tg.c 4870 J.2
Conics, equations of parabola 4201 t.c p.c
Conics, equations of parabola, general 4430 4713 t.c 4689 tg.c 5000
Conics, equations of, general 4400 4714 4719 t.c t.c tg.c tg.c p.c t.c CP.4 5 t.c 7 Mem.52 N.43 45 65 see general
Conics, equations of, intercept 4498
Conics, equi-conjugates, gen.eq 4491
Conics, formulae J.39 N.62
Conics, general equation, cond. for a circle 4467 t.c t.c Q.2
| Conics, general equation, cond. for a circle, from eq. of axes N.57
Conics, general equation, cond. for a hyperbola 4468 t.c A.39
Conics, general equation, cond. for a parabola 4430 t.c 4735 4746 4775 tg.c
Conics, general equation, cond. for a rectangular hyperbola 4737 t.c tg.c
Conics, general equation, cond. for an ellipse 4464 t.c
Conics, general equation, cond. for two right lines 4469 4475 t.c
Conics, generation of N.75 Z.23
Conics, generation of, by a moving chord of a circle A.34
Conics, generation of, Maclaurin's method 4830 LM.4
Conics, generation of, Newton's method 4829
Conics, graphic problems N.80
Conics, Hailey's pr N.76
Conics, harmonically circum-scribed Q.18
Conics, harmonically inscribed Q.18
Conics, intersecting a surface in 5 points C.63
Conics, intersecting in 4 points J.23
Conics, limiting cases 4465—4477 Me.68
Conics, normals 1171 sd5629—5632 A.16 24 32 cn 47 An.cn 78 C.72 84 J.cn 56 62 Me.66 Mel.2 N.70 81 Q.8 Z.11 18 26
Conics, normals, circle through feet of N.80
Conics, normals, cutting off the min. or max. arc or area N.44
Conics, normals, dividing ellipse most unequally E.29
Conics, normals, eccentric angles of the feet of four, th 4334
Conics, normals, equations of 4286 4483 4512
Conics, normals, intercepts 4294
Conics, normals, intercepts, segments 4309 4486
Conics, normals, least distance between two A.21 38
Conics, normals, number of real J.59 N.70 72
Conics, number cut by 8 lines in space J.68
Conics, number under double conditions C.59
Conics, octagram LM.2
Conics, parameter of N.43
Conics, passing through given points and touching given lines cn 4831—4840
Conics, passing through given points and touching given lines, 1 point and touching 3 lines Q.8
Conics, passing through given points and touching given lines, 2 points and touching 2 lines, locus of centre G.7
Conics, passing through given points and touching given lines, 2 points and touching 2 lines, locus of centre, four such conics, th Q.8
Conics, passing through given points and touching given lines, 2 points and touching a line Q.2
Conics, passing through given points and touching given lines, 3 points and touching a circle twice N.80
Conics, passing through given points and touching given lines, 3 points and touching a line Q.6
Conics, passing through given points and touching given lines, 3 points with given focus cn A.54
Conics, passing through given points and touching given lines, 4 foci of a conic Q.5
Conics, passing through given points and touching given lines, 4 points N.66 Q.2 8 Z.9
Conics, passing through given points and touching given lines, 4 points and touching a line cn 4833 A.65
Conics, passing through given points and touching given lines, 4 points, envelop of E.28
Conics, passing through given points and touching given lines, 4 points, envelop of, Do. of axes N.79
Conics, passing through given points and touching given lines, 4 points, locus of pole of a line 4770
Conics, passing through given points and touching given lines, 5 points cn 4831 eq A.27 A.9 24 64 An.50 N.57
Conics, pencil of M.19
Conics, perpendicular from centre on tangent 1195 4366—4373
Conics, perpendicular from centre on tangent, ditto from foci 1178 4300
Conics, pole of chord joining 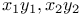 4326 4326
Conics, pole of chord joining 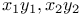 , parabola 4218 , parabola 4218
Conics, properties of 1274 A.4 25 70 p.cJ.38
Conics, quadrature of TE.6
Conics, rectification of 6071 6084 P.2 TI.16 Z.2
Conics, reduction of u, u' to the forms 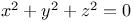 , , 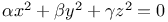 4995 4995
Conics, series of A.cn 67 cn
Conics, seven points of C.94
Conics, similar 4522
Conics, six points of A.62 J.92
Conics, systems of C.62 65 M.6 N.66
Conics, systems of 2 Q.7
Conics, systems of 4 L.54
Conics, systems of, and orthogonal lines N.71
Conics, systems of, and quadrics Z.6
Conics, tangent curves of Z.15
Conics, tangents or polar 1167—1169 4280—4285 4790 gn.eq A.61 cnCD.3 CM.1 p.eq N.79
Conics, tangents, at the points  , , 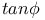 , ,  4799 4799
Conics, tangents, do. for parabola 4215 cn
Conics, tangents, do. for parabola, ratio of lengths 1243
Conics, tangents, intercepts on the axes 4292
Conics, tangents, locus of x'y' A.69
Conics, tangents, quadratic for abscissae of points of contact 4312
Conics, tangents, quadratic for abscissae of points of contact, parabola 4216
Conics, tangents, quadratic for m 4313
Conics, tangents, quadratic for m, parabola 4220
Conics, tangents, segments of 4307
Conics, tangents, subtend equal angles at focus 1181 1234 CM.2
Conics, tangents, two at the origin, eq 4489
Conics, tangents, two from x'y' 4311 gn.eq 4965 A.57 t.c cn A.53
Conics, theorems 1267 A.54 J.16 L.44 M.3 N.45 48 72 84 Q.4 6 7
Conics, theorems, 3 circles touch a conic in A, B, C and all cut it in D; A, B, C, D are concyclic J.36
Conics, theorems, conic and triangle Q.5
Conics, theorems, t.c by Pascal, Desarques, Carnot, and Chasles A.53
Conics, three 4707 4710
Conics, three, in contact 4803
Conics, three, Jacobian of 5023
Conics, touching a conic and line, cond. 5017
Conics, touching a curve twice J.45
Conics, touching a group of lines, and having a given characteristic and focus A.49
Conics, touching a quintic curve in 5 points, no of N.66
Conics, touching curves of any order C.59
Conics, touching five curves C.58
Conics, touching four lines 4804
Conics, touching four lines, locus of centre 4772 5028
Conics, touching four lines, locus of focus 5029 N.45 67
Conics, touching n lines N.61
Conics, touching two circles twice 4806
Conics, touching two conics twice 4803
Conics, touching two sides of the trigon 4784—4808
Conics, transformation of G.10
Conics, two 4936—5030 N.58
Conics, two, at infinity 4715—4716
Conics, two, common elements, cn A.68
Conics, two, common pole and polar, cn 4762
Conics, two, condition of touching 4942
Conics, two, condition of touching, t.c 5021
Conics, two, intersecting in 4 points 4700 A.32
Conics, two, intersecting in 4 points at  A.16 A.16
Conics, two, points of intersection tg.e 4973 cn
Conics, two, reciprocal properties E.29
Conics, two, reduction to 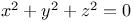 and and 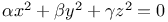 4995 4995
Conics, two, six chords of, eq 4941
Conics, two, tangents of, four eq 4981 J.75 Q.3
Conics, two, with common chords or tangents 4700—4705
Conics, two, with common points and tangents 4701—4707 LM.14 Z.18
Conjugate functions apLM.12 TI.17
Conjugate lines of surfaces CD.9
Conjugate point-pair of a conic Z.17
Conjugate points 1066 5184
Conjugate points in ellipse A.38
Conjugate tetrahedrons of a quadric, each vertex being the pole of the opposite side N.60 83
Conjugate triangle of a conic N.83
Connexes (1, n) corresponding to d.e A.69
Connexes (1, n) corresponding to d.e in space Mel.6
Connexes (1, n) corresponding to d.e of 1st order and class in simple involution G.20
Connexes (1, n) corresponding to d.e of 2nd order and class G.19
Connexes (1, n) corresponding to d.e, analogue in anal.geo. of space M.14
Connexes (1, n) corresponding to d.e, linear M.15
Cono-cunei A.2
Constant coefficients, theory I.23
Constant functions and their derivatives A.15
Contact 4-pointic on an algebraic surface M.9
Contact and circle of curvature 4527 5188 5134 A.30
Contact of 3rd order between 2 surfaces C.74
Contact of an implexe with an alg. surface C.84
Contact of curves 5188
Contact of curves with a parabola Z.2
Contact of curves with faisceaux of doubly infinite curves C.83
Contact of curves with surfaces L.78
Contact of curves with surfaces with triangles M.7
Contact of curves, cir. of curv. A.53 C.32 J.66 P.62 Pr.11 Q.7
Contact of lines with surfaces L.37 Q.1 17 Z.12
Contact of quadrics LM.5
Contact of spheres JP.2
Contact of surfaces J.4 JP.15 P.72 74 76 Q.12
Contact problem of Apollonius A.66
Contact problem of Apollonius, spherical At.19
Contact transformation C.85 M.23
Contingence angle of see "Torsion"
Contingence, Angle of 5725
|
 |
|
О проекте
|
|
О проекте



 and unicursal curves
and unicursal curves 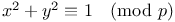
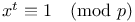
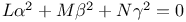
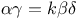 and derived equations
and derived equations 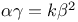 or
or 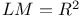
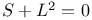 , &c.
, &c.  and p
and p 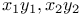
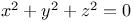 ,
, 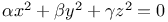
 ,
, 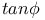 ,
, 
