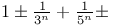Residues, ap. to integrals whose derivatives involve the roots of alg. eqs. C.23
Residues, ap. to reciprocity law of two primes and asymptotes C.76
Residues, biquadratic C.64 J.28 39 L.67
Residues, cubic A.43 63 C.79 J.28 32 L.76
Residues, quadratic Ac.1 J.28 71 Q.1
Residues, quadratic and partition of numbers J.61
Residues, quadratic of primes, also non-residues L.42 Mel.4
Residues, quadratic, ext. of Gauss's criticism Mo.76
Residues, quintic C.76 Z.27
Residues, septic C.80
Resultant alg. M.16 extC.58
Resultant alg. and discriminants and product of differences of roots of eqs., relation Me.80
Resultant alg. of 3 ternary quadrics J.57 N.69
Resultant alg. of covariants M.4
Resultant alg. of n equations An.56
Resultant alg. of two equations J.30 50 53 54 M.3 P.57 68
Resultant alg. of two integral functions Z.17
Reversible symbolic factors Q.9
Reversion of angles LM.6
Rhizic curves Q.11
Rhombus, circumscribing an equil. triangle A.45
Rhombus, quadrisection by two rectangular lines Mem.11
Riemann's function A.68 J.83 M.21
Riemann's function,  -formula, gz Ac.3 -formula, gz Ac.3
Riemann's function, ext. to hyper-geo.-functions of 2 variables C.95
Riemann's surface LM.8 M.6 18 thsZ.12
Riemann's surface of 3rd species M.17
Riemann's surface, irrationality of M.17
Riemann's surface, new kind of M.7 10
Right cone Me.72 73 75 76
Right line A.49 57 fQ.15 t.c
Right line and circle ths N.56
Right line and conic Q.7
Right line and conic, cn. for points of section A.59 66 N.85
Right line and conic, condition of touching 4315 4323 t.c
Right line and conic, quadratic for abscissae of the points 4319
Right line and conic, tg.e of the points 4903
Right line and curve 4131—4135
Right line and curve, a constant product C.82 83
Right line and curve, a constant ratio C.83
Right line and curve, ths. in which pairs of segments have a constant length C.83
Right line and curve, ths. in which systems of 3 segments have a constant product C.83
Right line and plane prs CD.1 CM.2 t.c q.c
Right line and quadric 5676
Right line and quadric of revolution N.82
Right line and quadric, harmonic division 5687
Right line at infinity 4612—4614 tg.c
Right line at infinity, cond. for touching a curve 4900
Right line through a point 4073 4088—4089 4099 t.c
Right line through a point and perp. or paral. to a given line sd5538—5539
Right line through a point, condition 4101
Right line through four lines in space A.1 CM.3
Right line through four lines in space, Gergonne's pr. J.2 N.17
Right line through two points 4083 sd5537 t.c 4789 p.c
Right line through two points on a conic, equation of, ellipse 4324
Right line through two points on a conic, equation of, parab. 4225
Right line under four given conditions C.68
Right line under given conditions C.73 74
Right line, constants, relations between sd. 5515
Right line, coordinates of G.10
Right line, coordinates of, relation between two 4897
Right line, crystallography A.34
Right line, drawn from x'y' across a conic, gen. eq. 4494
Right line, drawn from x'y' across a conic, method 4134
Right line, drawn from x'y' across a conic, quadratic for the segments in a parabola 4221
Right line, drawn from x'y' across a conic, quadratic for the segments in an ellipse 4314
Right line, equations of 4052—4066 p.c t.c sd q.c
Right line, geometry of A.64 thsJ.8
Right line, joining two points and crossing a conic, gn.eq. 4487 t.c
Right line, joining two points and crossing a conic, method 4131
Right line, joining two points and crossing a conic, quadratic for ratio of segments in a parabola 4214
Right line, joining two points and crossing a conic, quadratic for ratio of segments in an ellipse 4310
Right line, joining two points, coordinates of point dividing the distance in a given ratio 4032 t.c 5507
Right line, joining two points, coordinates of point dividing the distance in a given ratio, tg.eq. of the point 4879
Right line, pencils of C.70 L.72
Right line, pencils of, quadruple J.67
Right line, planes or points through or on given points, lines, or planes, number of such Z.6
Right line, pole of t.c 4671 tg.e
Right line, six coordinates of CP.11
Right line, system of At.68 G.9 10 16
Right line, system of, and planes, geo. of 2nd kind At.65
Right line, system of, in space G.11 12 L.46
Right line, system of, of 1st degree G.6
Right line, system of, of 2nd degree G.7
Right line, three points lying on, cond. 4036 t.c
Right line, three, condition of intersection 4097 t.c
Right line, touching a surface, condition 5786
Right line, touching a surface, quadric 5703
Right line, two, bisector of the angle 4113 sd5540 q.c
Right line, two, cond. of either touching the conic 4964
Right line, two, cond. of intersecting on a conic, gn.eq 4962
Right line, two, cond. of intersection sd5533
Right line, two, cond. of parallelism 4076 t.c sd5531
Right line, two, cond. of perp. 4078 t.c sd5532
Right line, two, coordinates 4090 t.c
Right line, two, drawn to the points of section of a right line and conic, eq. of A.69
Right line, two, right angle between them 4112 sd5520 5553 CP.2 N.66
Right line, two, shortest distance sd5534—5536
Right line, two, through origin, eq. of 4111
Right solid, M. I. of 6018 thA.34
Right-angled triangles 718 prs
Right-angled triangles with commensurable sides E.33
Rodrique's th. Me.80 84
Rolling and sliding solids, geo thsC.46
Rolling cones L.53
Roots of algebraic fractions N.46
Roots of an equation 50 402 A.14 CM.2 CP.8 E.36 J.20 31 N.42 56 P.1798 37 64 Q.1 5 6 see
Roots of an equation as continued fractions CM.3
Roots of an equation as definite integrals J.2
Roots of an equation as definite integrals, 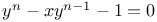 Me.81 P.64 Me.81 P.64
Roots of an equation as determinants of the coefficients A.59 61
Roots of an equation as functions of a variable parameter C.30
Roots of an equation in a converging series C.23 38
Roots of an equation in a given ratio J.10
Roots of an equation in g.p N.89
Roots of an equation in sums of rational functions of the coefficients Ac.6
Roots of an equation of a biquadratic, cn N.44
Roots of an equation of a biquadratic, cn by parab. and circle N.87
Roots of an equation of a quadratic 50—53
Roots of an equation of a quartic and of a Hessian, relation E.34
Roots of an equation of cubic L.44 N.42
Roots of an equation of cubic and biquadratic An.55 L.55
Roots of an equation of quintics C.59 60 LM.14 TI.18
Roots of an equation of the form 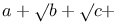 N.45 N.45
Roots of an equation which are the binary products of the roots of two eqs. An.79
Roots of an equation which satisfies a linear d.e of 2nd order C.94
Roots of an equation with a variable parameter C.12
Roots of an equation with equal differences G.15
Roots of an equation, commensurable 502 N.45 th57
Roots of an equation, commensurable, limits to the number N.59
Roots of an equation, common 462 C.80 88 N.55 69
Roots of an equation, continuity of N.76
Roots of an equation, discrimination of 409 A.46
Roots of an equation, do. of differences of roots C.98
Roots of an equation, do. of the common roots of two eqs. N.60 Z.15
Roots of an equation, equal 432—447 CD.5 E.33 Mel.1 P.1782 Q.9 18
Roots of an equation, existence of A.15 CD.2 CP.10 E.36 G.2 J.5 44 88 LM.1 Q.11 TI.26
Roots of an equation, expanded in power series J.48
Roots of an equation, forms for quadrics, cubics, and quartics Z.24
Roots of an equation, functions of the roots of another eq. 425 430
Roots of an equation, functions of the roots of another eq., ap N.50 E.40
Roots of an equation, functions of the roots of another eq., products in pairs Q.13
Roots of an equation, functions of the roots of another eq., squares of differences 541
Roots of an equation, functions of, relation to coefficients TE.28
Roots of an equation, functions of, similar L.54
Roots of an equation, geo.cn of JP.10
Roots of an equation, imaginary 408
Roots of an equation, imaginary, approx. N.45 53 Q.9
Roots of an equation, imaginary, between given limits A.21 L.44
Roots of an equation, imaginary, geo.cn A.15 45 C.24 86—88 JP.11 L.50 N.46 47 68
| Roots of an equation, imaginary, Newton — Fourier rule Q.16
Roots of an equation, imaginary, Newton — Sylvester rule 530 C.99 LM.1 Me.66 Pr.14 Q.9 TI.24
Roots of an equation, imaginary, Newton's rule Me.80 N.67 Pr.13
Roots of an equation, in infinite series A.69
Roots of an equation, incommensurable 506 see
Roots of an equation, infinite N.44 45
Roots of an equation, integral, by Newton's method of divisors 459
Roots of an equation, least M.9 TE.28
Roots of an equation, limits of 448 C.58 60 93
Roots of an equation, limits of, geo CP.12 N.43 45 59 72 80 81
Roots of an equation, limits of, number between given limits A.1 G.9 J.52 L.40
Roots of an equation, limits of, Rolle's th. 454 AJ.4 N.44
Roots of an equation, limits of, Rolle's th., ext L.64
Roots of an equation, limits of, Rolle, Fourier, and Descartes A.1
Roots of an equation, limits of, the eq. containing only odd powers of x N.63
Roots of an equation, number satisfying a given condition C.40
Roots of an equation, product of differences Me.80 P.61
Roots of an equation, rationalization of P.1798 14
Roots of an equation, real A.36 58 C.61 J.50 JP.10 N.50 P.57
Roots of an equation, real, developed in a series L.78 N.56
Roots of an equation, real, Fourier's th. N.44
Roots of an equation, real, limits of J.1 N.53 79
Roots of an equation, real, of a cubic N.72 Z.2
Roots of an equation, real, series which give the number of Z.2
Roots of an equation, real, to find four An.55
Roots of an equation, rule of signs 416—423 A.34 C.92 98 99 N.43 46 47 67 69 79
Roots of an equation, separation of A.28 70 J.20 N.68 72 74 75 80
Roots of an equation, separation of, by differences N.54
Roots of an equation, separation of, for biquadratics A.47
Roots of an equation, separation of, for numerical C.89 92 G.6
Roots of an equation, simultaneous eqs. C.5
Roots of an equation, squares of differences 541 CM.1 N.42 44 Q.4
Roots of an equation, sums of powers 534 E.38 thJ.9 N.53 75 gzMe.85 Q.19
Roots of an equation, surd forms of CM.3
Roots of an equation, symmetrical functions of 534 A.16 AJ.1 An.54 55 60 C.44 45 G.5 11 J.19 54 81 Me.81 N.48 50 55 66 84 P.57 Pr.8 Q.4 TI.25
Roots of numbers 108 A.17 26 35 C.58 E.36 Me.75 N.61 70
Roots of numbers as a continued fraction 195 A.6 12 49 CM.2 L.47 Me.85 Mem.10 TE.5 Z.17
Roots of numbers as a continued fraction to 25 decimal places Me.77
Roots of numbers, cube root and sq. root, limit of error N.48
Roots of numbers, cube root of 2 to 28 decimal places Me.76 78
Roots of numbers, cube root, Horner's method 37 A.67
Roots of numbers, fourth root A.30
Roots of numbers, nth root as a fraction A.46
Roots of numbers, square root 35 C.93 N.45 46 61 70
Roots of unity 475—481 C.38 J.40 L.38 54 59 Me.75 N.43 TE.21 Z.22
Roots of unity, 23 roots, composition of number 47 J.55 56
Roots of unity, cubic roots, alg. and geo. deductions C.84
Roots of unity, function theory Z.22
Rosettes N.48
Rotation CM.3 LM.3
Rotation of system of lines drawn through points on a directrix, modulus of C.21
Rotation, infinitesimal C.78
Roulettes 5229 Ac.63 C.70 CP.7 J.65 L.80 81 N.56 TE.16 Z.28
Roulettes, areas of, and Steiner's transf. E.35
Roulettes, generated by a circle rolling on a circle JP.21
Roulettes, generated by centre of curvature of rolling curve L.59
Roulettes, generated by focus of ellipse rolling on a right line A.48
Ruled surfaces An.68 CD.8 G.3 J.8 L.78 N.61
Ruled surfaces and guiding curve A.18
Ruled surfaces of minimum area L.42
Ruled surfaces of species, p = 0 M.5
Ruled surfaces, areas of parallel sections Z.20
Ruled surfaces, octic with 4 double conics C.60
Ruled surfaces, P. D. eq. of Me.77
Ruled surfaces, quadric Me.68
Ruled surfaces, quartic A.65
Ruled surfaces, quartic with 2 double lines A.65
Ruled surfaces, quintic J.67
Ruled surfaces, represented on a plane C.85
Ruled surfaces, symm. tetrahedral C.62
Ruled surfaces, torsal line M.17
Ruled surfaces, transformation of C.88
Scales of notation 342 J.1 L.48 5ry 10ry 20ry
Screws TI.25
Scrolls A.53 CD.7 CP.11 J.20 67 M.8
Scrolls, condensation of LM.13
Scrolls, cubic M.1 P.63 64 69 Pr.12 13 16 Z.cn28
Scrolls, cubic on a quadric surface Me.85
Scrolls, flexure and equilib. of LM.12
Scrolls, ruled A.68
Scrolls, ruled,  A.55 A.55
Scrolls, tangent curves of M.12
Sections of the cone 1150
Sectors and segments of conics and conicoids 6019—6162 G.1 thsZ.1
Secular eq. has real roots J.88
Self-conjugate triangle 4755 4967 G.8 N.67 Q.5 10
Self-conjugate triangle and tetrahedron in conics and quadrics Z.6
Self-conjugate triangle of 2 conics Me.77
Self-conjugate triangle of 3 conics 5025
Self-enveloping curves and surfaces Z.22
Self-reciprocal surface Mo.78
Self-reciprocal triangle 1020
Seminvariants AJ.7 E.th42 6 Q.19—21
Seminvariants, critical and Spencian functions Q.4 6
Seminvariants, critical and symm. functions AJ.6
Septic equations Mo.58
Series 125—129 149—159 248—295 756—817 1460 1471—1472 1500—1573 2708—2709 2743—2760 2852—2864 2880 2911—2968 3781 3820 A.4 5 9 14 18 23 60 No.39 47 C.29 pr92 CP.9 G.10 J.3 17 34 38 th53 L.th56 81 Me.64 N.59 th62 70 Q.3 Z.15 16 23 see "Expansions"
Series and definite integrals L.82 Man.46
Series and differentiations A.10 J.36
Series from 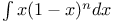 A.47 A.47
Series from  G.10 G.10
Series from infinite products Me.73
Series from log (1 + x),  and and 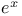 by intermitting terms in the expansions A.21 by intermitting terms in the expansions A.21
Series in a triangle problem A.64
Series in a.p see "Arithmetical progression"
Series in a.p, analogous series N.69
Series in cosines of multiple angles C.44 Mem.15
Series in Legendre's function 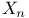 An.76 C.44 An.76 C.44
Series in theory of numbers C.89
Series of Abel C.93 N.85
Series of fractions L.40
Series of Gauss and Heine C.73 G.9
Series of Hermite, a th. E.29
Series of Lagrange C.23 34 52 L.57 N.76 gz85 Q.2
Series of Lagrange, an analogous series C.99
Series of Lagrange, remainder C.53
Series of Lambert, 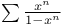 A.10 An.68 J.9 Z.6 A.10 An.68 J.9 Z.6
Series of Laplace's functions, 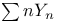 C.44 C.44
Series of Laplace's functions, 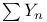 C.88 C.88
Series of Leibnitz J.89
Series of odd numbers A.64
Series of polynomials C.96
Series of posterns G.6
Series of powers 277 C.87 G.2 see
Series of powers in a convergent cycle, constants in M.25
Series of powers of a binomial Mem.13
Series of powers of terms in ar.p L.46
Series of powers or multiples of 3 A.27
Series of powers, approximate fractions J.90
Series of powers, cubes L.64 65 M.23 Mo.78 Q.8 Z.1
Series of powers, like numbers N.71 77
Series of reciprocals Q.8
Series of Schwab N.59
Series of spherical functions An.75
Series of Sterling, for transformation J.59
Series whose coefficients are the sums of divisors of the exponents, sq. of this series Me.85
Series whose terms are the coefficients of the same power of a single variable in a multiple integral C.20
Series with Bernoulli's nos. An.53
Series with Bernoulli's nos. and binomial coefficients A.23
Series with constant ultimate differences Pr.52
Series with terms alternately positive and negative C.64
Series, 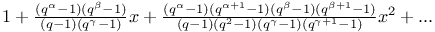 J.32 70 J.32 70
Series,  J.37 J.37
Series,  with x = 1 J.2 with x = 1 J.2
Series,  J.20 J.20
Series, 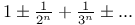 and and 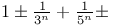 2940—2944 A.41 LM.8 Q.7 2940—2944 A.41 LM.8 Q.7
Series, 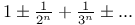 and and 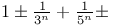 with n = 1, 2...8 2945 E.32 39 G.10 N.79 Z.3 with n = 1, 2...8 2945 E.32 39 G.10 N.79 Z.3
Series, 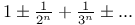 and and 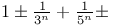 , Note that , Note that  2391 2391
Series,  2939 A.65 Me.78 2939 A.65 Me.78
Series,  , p = 1, 2, 3, or 4 276 A.64 E.34 , p = 1, 2, 3, or 4 276 A.64 E.34
|
 |
|
О проекте
|
|
О проекте



 -formula, gz
-formula, gz 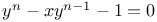
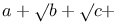

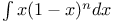

 and
and 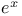 by intermitting terms in the expansions
by intermitting terms in the expansions 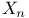
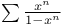
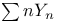
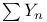
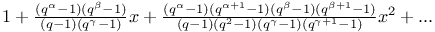


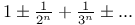 and
and