Series, 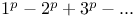 & & 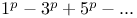 J.7 J.7
Series,  J.31 J.31
Series, 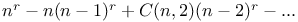 285 285
Series, 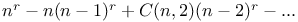 , r = n CM.1 , r = n CM.1
Series,  150 150
Series, 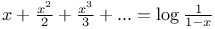 156 156
Series, 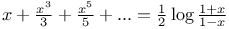 157 157
Series,  155 155
Series, 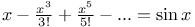 764 764
Series,  791 791
Series, 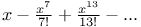 E.44 E.44
Series, 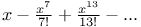 with x = 1 J.5 with x = 1 J.5
Series, 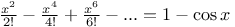 765 765
Series,  Q.6 Q.6
Series, 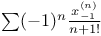 A.26 A.26
Series,  N.56 N.56
Series,  Z.15 Z.15
Series, 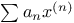 2709 2709
Series,  Z.1 Z.1
Series, 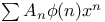 J.25 28 J.25 28
Series, 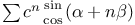 783 783
Series, 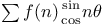 J.42 L.52 J.42 L.52
Series, 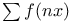 L.51 L.51
Series,  J.41 J.41
Series, 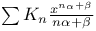 , ,  a pos. integer < a pos. integer <  , , 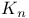 = the general term of some recurring series C.86 = the general term of some recurring series C.86
Series, 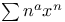 A.27 A.27
Series,  A.50 A.50
Series, 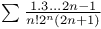 L.60 L.60
Series, 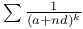 N.59 N.59
Series,  A.44 A.44
Series, 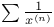 E.56 E.56
Series, 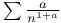 A.34 A.34
Series, 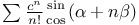 788 788
Series, 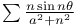 2962 M.5 2962 M.5
Series, 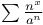 A.41 A.41
Series, 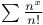 A.61 A.61
Series, 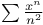 Mem.11 Mem.11
Series, 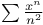 with x = 1 J.5 with x = 1 J.5
Series, 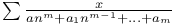 A.35 A.35
Series, 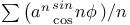 2922—2923 2922—2923
Series, 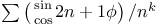 J.54 J.54
Series, 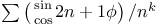 with k = 2 2960—2961 J.8 with k = 2 2960—2961 J.8
Series, 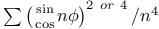 and and 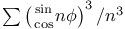 L.73 L.73
Series, 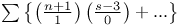 G.11 G.11
Series, 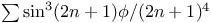 E.39 E.39
Series, 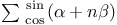 800 Q.3 800 Q.3
Series, 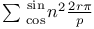 L.40 L.40
Series,  A.22 A.22
Series,  C.50 C.50
Series, a paradox Me.72
Series, a.p and g.p combined A.9
Series, application thA.48
Series, application to arith G.7
Series, binomial see "Binomial theorem"
Series, binomial, analogous series E.35 J.32 N.82
Series, binomial, analogous series with inverse binomial coefficients Me.80
Series, coefficients independently determined A.18
Series, combination A.26
Series, convergent and of d.i with a periodic factor L.53
Series, convergent and products, condition M.22
Series, convergent in Kepler's problems Ac.1799
Series, convergent whose terms are continuous functions of the same variable C.36
Series, convergent, multiplication of M.24
Series, convergent, power-series A.25
Series, convergent, representing functions M.5 22
Series, convergent, representing integrals of d.e C.40
Series, convergent: 239 A.2 6 8 14 26 41 67 69 No.44 C.10 11 28 40 43 J.2 3 11 13 16 22 42 45 76 L.39—42 M.10 20—22 Me.64 N.45 46 67 69 70 P.87 Z.10 11
Series, converted into continued fractions J.32 33 Mem.9
Series, converted into products of an infinite no. of factors J.12 L.57 N.47
Series, deduction from 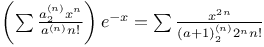 LM.9 LM.9
Series, derived A.22
Series, derived from 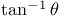 A.16 A.16
Series, developed in elliptic integrals of 1st and 2nd kind An.69
Series, difference 264 A.23 24
Series, differential transf. and reversal of Pr.7
Series, Dirichlet's f. for 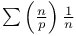 C.21 L.46 C.21 L.46
Series, discontinuous CP.6 L.54 Me.78 82 N.85
Series, divergent A.64 No.68 C.17 20 CP.8 10 J.11 13 41 M.10 Z.10
Series, division of AJ.5
Series, double C.63
Series, doubly infinite CD.6 M.24
Series, ext. of by any parameter A.48
Series, factorial 268 Mem.20 N.67 TE.20
Series, Fourier's A.39 C.91 92 96 CM.2 Z.27
Series, Gregory's 791
Series, harmonic periodic J.23 25
Series, integration of infinite A.3
Series, involving two angles L.74
Series, irrationality of some J.37
Series, Klein's higher An.71
Series, Laplace's (d.c) C.68
Series, limits of A.20 Me.76
Series, limits of, by the method of means J.13
Series, limits of, remainders C.34
Series, modular C.19
Series, multiple C.19
Series, multiple, "regulateur" of C.44
Series, neutral CP.11
Series, obtained by inversion from Taylor's series Mem.11
Series, periodic, critical values of CP.8
Series, products of contiguous terms of Mem.18
Series, recurring 251
Series, recurring, doubly An.57 J.33 38 Me.66 Mem.24 26 N.84
Series, recurring, of circles and spheres N.62
Series, recurring, of circles and spheres, f Z.14
Series, represented by rational fractional functions J.30
Series, reversion of 551 J.52 54 LM.2 TI.7
Series, self-repeating CP.9
Series, transformation of C.59 J.7 9
Series, transformation of 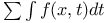 and others C.13 and others C.13
Series, transformation of, into a continued fraction Mem.20 Z.7
Series, trigonometrical A.63 Ac.2 C.95 97 M.4—6 16 17 22 24 J.71 72
Series, trigonometrical, conversion in multiples of arc L.51
Series, trigonometrical, representing an arbitrary function between given limits J.4
Series, trigonometrical, symbolic transf. of Q.3
Series, triple G.9
Series, two infinite, multiplication rule J.79
Seven planes problem N.56
Seven-point circle 4754c
Sextactic points of plane curves Pr.13 14
Sextic curves and ellipse, pr J.33
Sextic curves,  Q.15 Q.15
Sextic curves, bicursal LM.7
Sextic curves, mech.cn LM.2
Sextic developable Q.7 9
Sextic equation C.64 M.20
Sextic equation, irreducible J.37
Sextic equation, solution when the roots are connected by 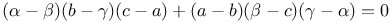 J.41 J.41
Sextic torse An.69
Sextinvariant to a quartic and quart-invariant to a sextic AJ.1
Shortest distance between two lines 5534 A.46 G.5 N.49 66
Shortest distance between two points on a sphere A.14 N.14 67 68
Shortest distance from the centre of a surface A.63
Shortest distance of a point from a line or plane N.44
Shortest line on a surface A.23 37 64
Shortest line on a surface in spheroidal trigonometry A.40
Signs CP.2 11 J.12 Me.73
Signs, 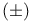 CD.6 7 Me.85 N.48 49 CD.6 7 Me.85 N.48 49
Signs, (=) Me.75
Similarity of curves and solids A.13
Similarly varying figures LM.16
Simpson's f. in areas 2992 C.78
Simson line of a triangle E.29
Sine and cosine in factors 807 A.27 J.27 L.54
Sine and cosine of 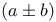 627 A.6 21 36 627 A.6 21 36
Sine and cosine of infinity CP.8 Me.71 Q.11
Sine and cosine of multiple arcs CM.4 TI.7 see
Sine and cosine of particular angles 690
Sine and cosine of particular angles, 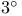 , , 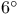 ,...to ,...to 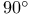 N.53 N.53
Sine and cosine, extension of meaning A.31 C.86
| Sine and cosine, sums of powers An.1
Sine and cosine, tables, formation of 688 A.66 N.42
Sine and cosine, values near 0 and 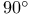 G.9 G.9
Sines of higher orders C.91 92
Sines of higher orders, ap. to d.e C.90 91
Sines, natural, limit of error N.43
Six points on a plane or sphere LM.2
Six-point circle of a triangle Me.82 83
Skew surfaces see "Scrolls"
Sliding rule LM.6
Small quantities of second order 1410
Smith's Prize questions, solutions Me.71 72—74 77
Solid angle A.42
Solid angle, section of No.19
Solid harmonics Me.80
Solid of revolution A.60 67
Solid of revolution between two ellipsoids A.2
Solid of revolution, cubature and quadrature of 5877—5880 A.68 N.42
Space homology G.20
Space theories An.70 LM.14 P.70 Z.17 18
Space theories, 21 coordinates of LM.10
Space theories, 3-dim. J.83
Space theories, 4-dim. J.83 M.24
Space theories, 6-dim. G.12
Space theories, absolutely real space G.6
Space theories, angles (4-dimen.) A.69
Space theories, areas and volumes A.69 CD.7
Space theories, bibliography of AJ.1 2
Space theories, circle G.12 16 18
Space theories, conics AJ.5
Space theories, continuous manifoldness of two dimensions LM.8
Space theories, curves C.79 M.18
Space theories, Feuerbach's points G.16
Space theories, Grassmann's "Ausdehnungslehre" AJ.1 CP.13 M.7 12 Z.24
Space theories, Grassmann's "Ausdehnungslehre", ap. to mechanics M.12
Space theories, hyperboloid Z.13
Space theories, imaginary quantities Z.23
Space theories, loci (anal.) C.24
Space theories, non Euclidean or n-dimensional A.6 29 58 ths64 AJ.4 5 An.71 C.75 G.6 10 12 23 M.4 5—7 Me.ths68 72 Pr.37
Space theories, plane triangle A.70
Space theories, planes (4-dimen.) A.68
Space theories, Plucker's "New geometry of" G.8 L.66 P.11 Z.11 12
Space theories, point groups thsAc.7
Space theories, polars and alg. forms J.84
Space theories, potential function An.82 83
Space theories, projection M.19
Space theories, projection, 4-dim. into 3-dim. AJ.2
Space theories, quadric, super lines of (5-dim.) Q.12
Space theories, quaternions CP.13
Space theories, regular figures AJ.3
Space theories, representation by correlative figures C.81
Space theories, reversion of a closed surface AJ.1
Space theories, screws, theory in elliptic space LM.15 16
Space theories, simplicissimum of nth order E.44
Space theories, space of constant curvature An.69 73 J.86 M.12
Sphere, 16 touching 4 spheres J.37 JP.10 Me.cn82 Mem.10 N.44 47 65 66 84 Z.14
Sphere, 4 spheres, pr. L.46
Sphere, 4 touching a 5th At.19
Sphere, 5 points of J.23 N.84
Sphere, 8 touching 4 planes E.19 N.50
Sphere, and circle, geo A.57
Sphere, cn. from 4 conditions JP.9
Sphere, cutting 4 spheres at given angles An.51 N.83
Sphere, cutting a sphere orthogonally and touching a quadric, locus of centre TI.26
Sphere, diameters, no. of all imaginable A.24
Sphere, equation of 5582
Sphere, geo C.92
Sphere, illumination of Z.27
Sphere, kinematics on a LM.12
Sphere, q.c Me.62
Sphere, sector of (eccentric) A.65
Sphere, small circle of Me.85
Sphere, ths and prs M.4
Sphere, touching an equal sphere E.31 32
Sphere, touching an equal sphere, as many as possible A.56
Sphere, volume, &c. of segment and zone 6050 A.3 32 39 An.57 P.1
Spherical areas 902
Spherical catenaries J.33
Spherical class cubics with double foci and cyclic arcs Q.15
Spherical conics thQ.3
Spherical conics and quadrangle Q.13
Spherical conics, homofocal L.60
Spherical coordinates CD.1 CM.1 ap2
Spherical coordinates, homogeneous G.6
Spherical curvature 5728 5740 5747 thE.34
Spherical curves A.35 36 Mem.10
Spherical curves and polars No.63
Spherical curves of 3rd class with 3 single foci Q.17
Spherical curves of 4th class with quadruple foci Q.18
Spherical curves of 4th order J.43
Spherical curves with elliptic function coordinates J.93
Spherical curves, equidistant An.71 J.25
Spherical curves, rectification of An.54
Spherical ellipse t.c Q.8
Spherical ellipse, quadrature, &c. L.45 N.48 54
Spherical epicycloid G.12
Spherical excess Mel.2
Spherical excess of a quadrilateral Me.75
Spherical excess, cn N.46
Spherical excess, f Z.6
Spherical figures, division of J.22
Spherical geometry G.4 J.6 8 13 thsl5 22 M.15 N.48 58 59 Q.4 TI.8
Spherical harmonics or "Laplace's functions" An.68 C.86 99 CD.1 CM.2 J.26 56 60 geo 70 80 82 90 L.45 48 LM.9 Me.77 78 85 P.57 Pr.8 18 Q.7 Z.24
Spherical harmonics or "Laplace's functions" and connected d.i Q.19
Spherical harmonics or "Laplace's functions" and homogeneous functions CM.2
Spherical harmonics or "Laplace's functions" and potential of ellipse and ellipsoid P.79
Spherical harmonics or "Laplace's functions" and ultra-spherical functions Z.12
Spherical harmonics or "Laplace's functions" as determinants Me.77
Spherical harmonics or "Laplace's functions",  by continued fractions JP.28 by continued fractions JP.28
Spherical harmonics or "Laplace's functions",  by continued fractions, by continued fractions, 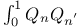 P.70 P.70
Spherical harmonics or "Laplace's functions", 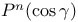 , ,  J.90 J.90
Spherical harmonics or "Laplace's functions", 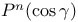 , ,  , , 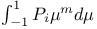 , &c. Q.17 , &c. Q.17
Spherical harmonics or "Laplace's functions", analogues of J.66 LM.11
Spherical loci in spherical coordinates TE.12
Spherical oblong An.52
Spherical oblong, area J.42
Spherical polygonometry J.2
Spherical polygons in- and circum-scribed to small circles of the sphere, by elliptic transcendents J.5
Spherical quadrilateral A.4 40
Spherical quadrilateral, surface of A.34 35
Spherical quadrilateral, th E.28 N.45
Spherical quartics, foci Q.21
Spherical quartics, foci, 4-cyclic and 3-focal LM.12
Spherical representation of surfaces C.68 75 94 96 M.13
Spherical surface represented on a plane Me.73
Spherical triangle A.9 ll 20 ths50 65 E.f30 J.10 pr28 JP.2
Spherical triangle and circle 898 A.29 33
Spherical triangle and differentials of sides and angles A.10
Spherical triangle and ex-circles 898 E.30
Spherical triangle and plane triangle A.1
Spherical triangle and plane triangle of the chords A.33 An.54 Z.1
Spherical triangle by small circles, area N.53
Spherical triangle of very small sides N.62
Spherical triangle, ambiguous case Me.77 85
Spherical triangle, angles of, calculated from sides A.51
Spherical triangle, cos (A + B + C), f. Me.72
Spherical triangle, graphic solution AJ.6
Spherical triangle, right angled 881 A.51
Spherical triangle, right angled, solution by a pentagon A.11
Spherical triangle, two, relations of sides and angles A.2
Spherical trigonometry 876 A.ths2 11 13 28 37 J.prs6 13 LM.11 N.42 Z.16
Spherical trigonometry, Cagnoli's th. 904
Spherical trigonometry, cot a sin b Me.64
Spherical trigonometry, cot a sin b, mnemonic 895 CM.3
Spherical trigonometry, d.e of circles Q.20
Spherical trigonometry, derived from plane A.26 27
Spherical trigonometry, formulae 882 A.5 16 24 26 N.45 46 53
Spherical trigonometry, formulae, ap. in elliptic functions A.40
Spherical trigonometry, formulae, graphically A.25
Spherical trigonometry, Gauss's eqs. 897 A.13 17 J.7 12 LM.3 13
|
 |
|
О проекте
|
|
О проекте



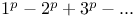 &
& 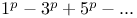

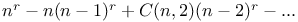

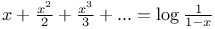
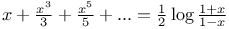

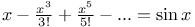

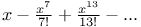
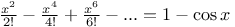

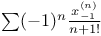

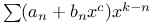
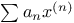

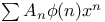
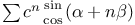
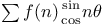
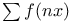

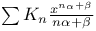 ,
,  a pos. integer <
a pos. integer <  ,
, 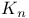 = the general term of some recurring series
= the general term of some recurring series 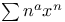

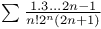
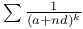

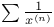
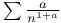
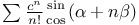
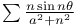
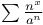
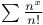
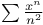
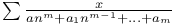
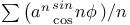
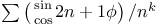
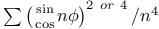 and
and 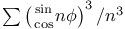
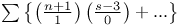
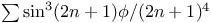
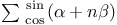
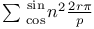
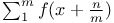
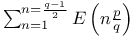
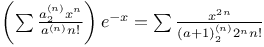
 and others
and others 
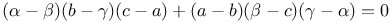
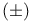
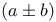
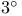 ,
, 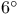 ,...to
,...to 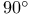
 by continued fractions
by continued fractions 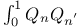
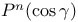 ,
, 
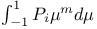 , &c.
, &c.