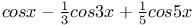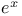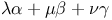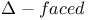Parabola, sector E.30 N.57
Parabola, sector, Lambert's th J.16
Parabola, segment of 6078 A.26 29
Parabola, segment of, solid generated by it N.42
Parabola, tangents see "Conics"
Parabola, through 4 points, cn 4837 J.26
Parabola, triangle of 3 tangents 1237 1268 A.47 Me.75
Parabola, trigonometry of CD.8
Paraboloid 5621 6126—6141 N.61 Q.13
Paraboloid of eight lines C.84
Paraboloid of revolution 6134
Paraboloid, elliptic 5622 A.11 L.58 P.96
Paraboloid, generating lines of 5624
Paraboloid, hyperbolic 5623 A.11
Paraboloid, quadrature of 6127 An.55.
Paraboloid, segment of 6127—6133 A.29
Paradoxes of De Morgan J.11 12 13 16
Parallel curves J.55 ths32 LM.3 Q.11 Z.5
Parallel curves of ellipse 4960 A.39 An.60 N.44 Q.12
Parallel curves, closed A.66
Parallel surface C.54 LM.12
Parallel surface of ellipsoid A.39 An.50 60 E.17 J.93
Parallel surface of surface of elasticity An.57
Parallelogram of Watt A.8 L.80
Parallelogram with sides through four given points A.39
Parallelopipeds on a spherical base N.45
Parallelopipeds on conjugate diameters 5648
Parallelopipeds, analogues of parallelograms LM.2 Me.68
Parallelopipeds, diagonals, &c. CM.1
Parallelopipeds, equality of A.4
Parallelopipeds, system of LM.8
Parallels A.8 47 At.51 J.11 73 Mel.1 3 Mem.50 Z.21 22
Parallels in analytic geometry A.44
Parallels, Thibaut's proof A.15
Partial differences: question in analysis J.16
Partial differential equations (P.D.E.) 3380—3445 C.3 11 16 78 95 96 thsCD.3 J.58 80 prs26 JP.7 10 11 L.36 80 83 M.11 Z.6 8 18
Partial differentials of  J.11 J.11
Partial fractions 235 1915 A.30 66 C.46 49 78 CM.1 G.2 J.1 5 9 10 11 22 32 50 JP.3 L.46 Mem.9 N.45 64 69 Q.5
Partition of numbers AJ.2 5 6 An.57 59 At.65 C.80 86 96 91 CP.8 J.13 61 85 M.14 Man.55 Me.78 79 Mem.13 geo.ap20 44 Mel.1 N.69 85 P.50 56 58 Pr.7 8 Q.1 2 7 15 Z.20 24 see "Indeterminate
Partition of numbers by Arbogast's derivatives L.82
Partition of numbers by elliptic and hyper-elliptic functions J.13
Partition of numbers into 10 triangular numbers C.62
Partition of numbers into 2 cubes L.70
Partition of numbers into 2 squares An.50 52 54 C.87 J.49 LM.8 9 N.54 78 alg65
Partition of numbers into 2 squares, odd squares Q.19
Partition of numbers into 3 squares J.40 L.59 60
Partition of numbers into 4 cubes N.77 N.79
Partition of numbers into 4 cubes, into 3 or 4 cubes A.60
Partition of numbers into 4 squares C.99 L.68 Pr.9 Q.1
Partition of numbers into 5 squares C.97 98 J.35
Partition of numbers into maximum nth powers C.95
Partition of numbers into p squares C.39 90 L.61 N.54
Partition of numbers into p squares and an integer L.57
Partition of numbers into parts, the sum of any two to be a sq. Mem.9
Partition of numbers into sum or difference of 2 cubes AJ.2
Partition of numbers into ten squares C.60 L.66
Partition of numbers into the product of two sums of sqs. L.57
Partition of numbers of n into 1, 2, 3, &c. different numbers E.34
Partition of numbers of pentagonal numbers C.96
Partition of numbers, 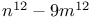 or its double into 2 cubes N.81 or its double into 2 cubes N.81
Partition of numbers, 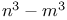 into into  N.49 N.49
Partition of numbers, 2 squares whose sum is a sq. E.20 N.50
Partition of numbers, 2 sums of 8 sqs. into 8 sqs. Me.78
Partition of numbers, 3 nos. whose sum is a cube, sum of sqs. a cube, and sum of cubes a sq. E.26
Partition of numbers, 3 nos., the sum or diff. of two to be a sq. Mem.18
Partition of numbers, 3 squares, the sum of every two being a sq. E.17
Partition of numbers, 4 odd, or 2 even and 2 odd Q.19 20
Partition of numbers, 4 squares, the sum of every two being a sq. E.16
Partition of numbers, 5 biquadrates whose sum is a square E.20
Partition of numbers, a cube into a sum of cubes E.22 23
Partition of numbers, a quadric into a sum of squares N.80 81
Partition of numbers, a series for the AJ.6
Partition of numbers, a square into a sum of cubes N.67
Partition of numbers, a sum of 4 sqs. into the product of 2 sums of 4 sqs. TI.21
Partition of numbers, formation of numbers out of cubes J.14
Partition of numbers, formula of verification Pr.24
Partition of numbers, n nos. whose sum is a sq. and sum of sqs. a biquadrate E.18 22 24
Partition of numbers, of complex numbers in Jacobi's th C.96
Partition of numbers, tables, non-unitary AJ.7
Partition of numbers, theorems AJ.6 C.40 96 Me.76 80 83
Partition of numbers, theorems, pr. symm. functions G.10
Partitions in planes and in space J.1
Partitions in theory of alg. forms G.19
Partitions, number of for n things E.10
Partitions, Sylvester's theorem Q.4
Partitions, trihedral of the X-ace and triangular of the X-gon Man.58
Pascal lines E.30
Pascal's theorem 4781 AJ.2 CD.3 4 CM.4 J.34 41 69 84 93 LM.8 Me.72 N.44 52 82 Q.1 4 5 9 Z.6 10
Pascal's theorem on a sphere A.60
Pascal's theorem, ap. to geo. analysis A.18
Pascal's theorem, extension of and analogues in space C.82 98 CD.4 5 6 G.11 J.37 75 M.22 Me.85
Pascal's theorem, Steiner's "Gegenpunkte" J.58
Pedal curve A.35 36 52 J.48 50 M.6 Me.80 81 Q.11 Z.5 21
Pedal curve of a cissoid, vertex for pole E.1
Pedal curve of a conic A.20 LM.3 Z.3
Pedal curve of a conic, central A.9 Me.83 N.71
Pedal curve of a conic, foci and vector eq. LM.13
Pedal curve of a conic, negative central E.20 29 TI.26
Pedal curve of a conic, negative focal E.16 17 20
Pedal curve of a conic, nth and n-1th E.18
Pedal curve of a parabola, focal and vector eqs. LM.13
Pedal curve of evolute of lemniscate E.30
Pedal curve which is its own pedal L.66
Pedal curve, circle and radius of curvature C.84 Z.14
Pedal curve, inversion and reciprocation of E.21
Pedal curve, rectification of difference of arcs of Z.3
Pedal surfaces A.22 35 36 M.6 J.50 Z.8
Pedal surfaces, counter AJ.5
Pedal surfaces, volumes of C.55 A.34 An.63 J.62 Pr.12
Pentagon, ths A.4 J.5 56 N.53
Pentagon, ths, diagonals of A.57
Pentagonal dedecahedron A.25
Pentahedron of given volume and minimum surface L.57
Periodic continued fractions A.62 68 C.96 J.20 33 53 N.68 Z.22
Periodic continued fractions with numerators not unity C.96
Periodic continued fractions, closed form of A.62
Periodic continued fractions, representing quadratic roots A.43
Periodic functions A.5 J.48 N.67 gzC.89 Mo.84
Periodic functions in theory of transcendents J.11
Periodic functions of 2 variables with 3 or 4 pairs of periods C.90
Periodic functions of 2nd species M.20
Periodic functions of several variables C.43 J.71
Periodic functions with non-periodic in def. integrals C.18
Periodic functions, 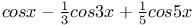 CD.3 CD.3
Periodic functions, 2n-ply, of n variables Mo.69
Periodic functions, 4-ply, of 2 variables J.13
Periodic functions, doubly C.32 40 70 90 J.88 L.54
Periodic functions, doubly, expansion in trig. series N.78
Periodic functions, doubly, monodromic and monogenous C.40
Periodic functions, doubly, of 2nd kind C.90 98 gzL.83
Periodic functions, doubly, of 3rd kind C.97
Periodic functions, doubly, with essential singular points C.89
Periodic functions, integrals between imaginary limits A.23
Periodic functions, multiply C.57 58
Periodic functions, real kind of Mo.66 84
Periodicity theory M.18
Periods cyclic, of the quadrature of an algebraic curve C.80 84
Periods in reciprocals of primes Me.73
Periods of integrals see "Integrals"
Periods of the exponential 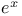 C.83 C.83
Periods, law of C.96
permutations 94 A1 C.22 CD.7 L.39 61 LM.15 Me.64 66 79 N.44 71 76 81 Q.1 Z.10
Permutations of 3q and 2q letters, 2 and 2 alike N.74 75
Permutations of n things C.95 N.83
Permutations of n things in groups L.65
Permutations with star arrangements Z.23
Permutations, alternate L.81
Permutations, ap. to differentiation and integration A.21
Permutations, number of values of a function through the permutations of its letters C.20 21 46 47 L.65
Permutations, successive ("battement de Monge") L.82
| Perpendicular from a point upon a line: length of 4094 t.c4624 eq4086 t.c4625 sd5530
Perpendicular from a point upon a plane 5554
Perpendicular from a point upon tangent of a conic 4366—4373
Perpendicular from a point upon tangent plane of a quadric 5627
Perpendicular from a point, ditto for any surface 5791—5793
Perpetuants AJ.7
Perspective 1083 A.69 G.3 thsL.37 Me.75 81
Perspective of coordinate planes CM.2
Perspective, analytical A.11
Perspective, drawing 1083—1086
Perspective, figures of circle and sphere A.57
Perspective, isometrical CP.1
Perspective, oblique parallel Z.16
Perspective, projection A.16 70
Perspective, relief A.36 70 N.57
Perspective, triangles 974 E.29 J.89 M.2 16
Perspective, triangles in a conic A.1
Petersburg problem A.67
Pfaff's problem A.60 C.94 J.61 82 M.17
Pfaff's problem, th of Jacobi J.57
Pfaffians, ths on Me.79 81
Piles of balls and shells N.72
Pinseux's theorem Mo.84
Plane J.20 45 Mem.22
Plane and line, problems CD.2 J.14
Plane coordinate geometry 4001—5473
Plane, cond. for intersection of two planes touching a quadric 5703
Plane, condition for touching a cone 5700
Plane, condition for touching a cone, ditto for a quadric 5635 5701
Plane, Equations of 5545 q.c p.c Z.1
Plane, equations of, under given conditions 5560—5573
Plane, figures, relation between A.55 J.52 M.3
Plane, kinematics of Q.16
Plane, motion of JP.2 LM.7
Plane, point-systems J.77
Plane, point-systems, perspective Z.17
Plane, representing a quadric N.71
Planimeter A.58 Mel.2 3
Planimeter, Amsler's 5452 C.77
Planimeter, polar A.51 N.80
Planimeter, Trunk's A.44
Planimetrical theorems A.37 60
Pluecker's complex surfaces M.7
Plueckerian characteristics of a curve discriminant Q.12
Plueckerian numbers of envelopes C.78
Point-pair, absolute on a conic Q.8
Point-pair, absolute on a conic, harmonic to two such Z.13
Point-plane system M.23
Points at infinity on a quadric N.65
Points in a plane, relation between four A.2 26
Points in a plane, relation between four, tg.eq of two 4669 4913
Points in space, represented by triplets of points on a line LM.2
Points of equal parallel transversals A.61
Points on a circle and on a sphere N.82
Points, four, or lines, ths CD.8
Points, roots in a closed curve N.68
Points, systems JP.9 M.6 25 N.58
Points, systems, of cubic curves Z.15
Points, three, coordinates of N.42
Points, three, pr A.8
polar 1016 4124 A.28 J.58 gzLM.2 Me.64 66 N.72 79
Polar curves, tangents of N.43
Polar line of two points with respect to a quadric 5685
Polar of 3 right lines A.1
Polar of a quartic L.57
Polar of conics 4762 thsN.58
Polar of cubic curves J.89 L.57 Mel.5 Q.2
Polar plane Q.2 Z.22
Polar plane of a quadric 5678 5687 An.71
Polar plane of a quadric, of four LM.13
Polar subtangent 5133
Polar surface of a cubic J.89
Polar surface of a cubic, twisted Z.23 24
Polar surface of a plane C.60 N.66
Polar surface of a point N.65
Polar surface of a triangle A.59
Polar surface of a triangle, perspective J.65
Polar surface, tetrahedron J.78 N.65 Z.13
Polar, developable 5728
Polar, inclined N.59
Pole and Polar 1016 4124
Pole of chords joining feet of normals of conic drawn from points on the evolute N.60
Pole of similitude 5587
Pole of the line 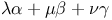 4671 4671
Political arithmetic trA.36—38
Pollock's geo. theorems Q.1
Poloids of Poinsot CD.3
Polyacrons, 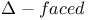 Man.62 Man.62
Polydrometry A.38 39
Polygonal numbers 287 Pr.10 11 12 13
Polygonometry thsAn.52 J.2 47 Mem.30
POLYGONS An.cn53 63 JP.4 9 N.74 Z.11 see
Polygons of circular arcs, cn A.3 J.76
Polygons of even number of sides LM.1
Polygons of n+2n sides, numbers related to A.62
Polygons of Poncelet, metrical properties L.79
Polygons, area of 748 4042 J.24 N.48 52
Polygons, articulated and pr. of configuration, tr An.84
Polygons, centroid N.77
Polygons, classification Q.2
Polygons, division into triangles A.1 8 L.38 39 LM.13 Pr.8
Polygons, family of N.83
Polygons, maximum with given sides J.26
Polygons, semi-regular JP.24
Polygons, semi-regular, star A.59 L.79 80
Polygons, sum of angles of A.52 N.50
Polygons, theorems A.1 2 C.26 prsCD.5 Mel.2 N.58
Polyhedral function (Prepotentials) CP.13
Polyhedrons 906 C.46 60—62 J.3 JP.4 9 15 24 L.66 Man.55 N.83 P.56 57 Pr.8 9 11 12 Q.7 Z.11 14
Polyhedrons, classification of C.51 52
Polyhedrons, convex, angles of C.74
Polyhedrons, convex, regular A.59
Polyhedrons, diagonals, number of N.63
Polyhedrons, Euler's theorem J.8 14 Mem.13 Z.9
Polyhedrons, F+S=E+2 906 A.24 E.20 27
Polyhedrons, maximum, for a given surface M.2 Mel.4
Polyhedrons, maximum, regular C.61
Polyhedrons, minimum surfaces of A.58
Polyhedrons, regular, ellipsoidal C.27
Polyhedrons, regular, self-conj. A.62
Polyhedrons, regular, star A.62 thsC.26
Polyhedrons, surface of A.53
Polyhedrons, surface of, volume J.24 N.52
Polyhedrons, symmetrical J.4 L.49
Polyhedrons, theorems E.39—42 J.18 N.43
Polynomials of two variables-analogous to Jacobi's A.16
Polynomials, determined from its partial differential A.4
Polynomials, geometry of JP.28 thQ.14
Polynomials, product of two N.44
Polynomials, system of L.56
Polynomials, value when the variable varies between given limits C.98
Polyzonal curves, 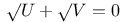 TE.25 TE.25
Porisms L.59 P.1798 Q.11 TE.3 4 9
Porisms of Euclid C.29 48 56—59 L.55
Porisms of in- and circum-polygon Me.83 P.61
Porisms of in- and circum-triangle LM.6 9 Q.1
Porisms of two circles Me.84
Porisms, Fermat's fourth A.46
Poristic equations LM.4 5
Poristic relations between two conics LM.8
Position, pr. relating to theory of numbers Mel.2
Potential thsAn.82 C.88 G.15 J.20 32 63 70 th81 85 M.2 3 N.70 Z.17
Potential of a circle J.76
Potential of a right solid J.58
Potential of a sphere Me.81
Potential of a sphere, surface of Me.83 Q.12 Z.7
Potential of cyclides C.83 J.61
Potential of cyclides, elliptic Me.78
Potential of ellipsoids J.98 Me.84 Q.14
Potential of ellipsoids, two homog. J.63 70
|
 |
|
О проекте
|
|
О проекте




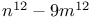 or its double into 2 cubes
or its double into 2 cubes 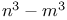 into
into