|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Dunford N., Schwartz J.T., Bade W.G. — Linear Operators, Part II: Spectral Theory. Self Adjoint Operators in Hilbert Space (Pure and Applied Mathematics: A Series of Texts and Monographs) |
|
|
 |
| Предметный указатель |
Morse, A.P. 87 235 393
Moser, J. 612
Moses, H.E. 1622
Moskovitz, D. 466
Muentz, C.H. 384
Multiplicative linear functional IV.6.23 277 see
Multiplicity of eigenvalues X.4 907
Munroe, M.E. 93 232 235
Murray, F.J. 554 884 886 1269
Myers, S.B. 382 397
Nachbin, L. 93 395 397 398 554
Nagata, J. 385
Nagumo, M. 79 84 394 608 609 726 883
Naimark, M.A. 876 883 884 886 932 1149 1160 1260 1261 1270 1274 1587 1588 1589 1590 1591 1593 1596 1597 1607 1608 1609 1610 1611
Nakamura, M. 233 391 395 539
Nakano, H. 80 395 471 927 928 929 1269 1273 1274
Nakayama, T. 927
Nathan, D.S. 726
Natural domain of existence of an analytic function 230
Natural embedding of a B-space II.3.18 66
Natural homomorphism onto factor space 38 39
NBV(I) 241
Neighborhood,  -, fundamental family of I.4.6 10 -, fundamental family of I.4.6 10
Neighborhood,  -, in a metric space I.6.1 18 -, in a metric space I.6.1 18
Neighborhood,  -, of a point or set I.4.1 10 -, of a point or set I.4.1 10
Nemyckii, V. 1587
Neumann, C. 608 1348 1349
Neumark, M. 396
Newburgh, J.D. 612
Newton, R.G. 1626
Nicolescu, M. 388
Niemytsky, V. 470
Nikodym see also "Radon — Nykodym theorem"
Nikodym, boundedness theorem IV.9.8 309
Nikodym, O.M. 93 160 176 181—182 234—235 309 387 390 392
Nikol'skii, V.N. 94 611
Nikovic, I.A. 1163
Nilpotent element 40
Nilpotent, topological nilpotent in B-algebra IX.2.5 869
Noerlund, N.E. 75
Non-singular linear transformation 45
Norm in a B-space II.3.1 59
Norm in a conjugate space II.3.5 60
Norm in an F-space II.1.10 51
Norm in Hilbert space IV.2.26 242
Norm in special spaces IV.2
Norm of an operator II.3.5 60
Norm, differentiability of 471—473 474
Norm, existence of 91
Norm, inequalities on  -norms VI.11.30—VI.11.37 535—536 -norms VI.11.30—VI.11.37 535—536
Norm, topology II.3.1 59
Normal operator in a finite dimensional space VII.2.14 563
Normal operator in Hilbert space X.1 887
Normal operator, real and imaginary parts of X.4 906
Normal structure, definition V.11.14 459
Normal structure, properties V.11.15—V.11.18 459
Normal subgroup 35
Normal topological space, compact Hausdorff space I.5.9 18
Normal topological space, definition I.5.1 15
Normal topological space, metric space I.6.3 19
Normal topological space, properties I.5.2—I.5.4 15—17
Normal topological space, regular space with countable base 24
Normed (normed linear space) see also "B-space"
Normed (normed linear space), definition II.3.1 59
Normed (normed linear space), study of II.3
Nowhere dense I.6.11 21
Null function see also "Null set"
Null function, criterion for III.6.8 147
Null function, definition III.2.3 103
Null set see also "Null function"
Null set, additional properties of III.9.2 169 III.9.8 III.9.16
Null set, criterion for III.6.7 147
Null set, definition III.1.11 100
O'Neill, B. 474
O, o 27
Ogasawara, T. 395 927
Ohira, K. 394
Ono, T. 88 400 884
Open set, criterion for I.4.2 10
Open set, definition I.4.1 10
Operational calculus X.1 890
Operational calculus for functions of an infinitesimal generator VIII.2.6 645
Operational calculus for unbounded closed operators VII.9.5 602
Operational calculus in finite dimensional space VII.1.5 558
Operational calculus in general complex B-space VII.3.10 568
Operational calculus, remarks on 607—609
Operator in a finite dimensional space 44
Operator topologies VI.1
Operator topologies, bounded strong VI.9.9 512
Operator topologies, bounded weak VI.9.7—VI.9.10 512
Operator topologies, continuous linear functionals in VI.1.4 477
Operator topologies, properties VI.9.1—VI.9.12 511—513
Operator topologies, remarks on 538
Operator topologies, strong VI.1.2 475
Operator topologies, strongest 538
Operator topologies, uniform VI.1.1 475
Operator topologies, weak VI.1.3 476
Operator, adjoint of VI.2
Operator, bound of II.3.5 60
Operator, bounded XII.1 1185
Operator, closed II.2.3 57 XII.1
Operator, compact, definition VI.5.1 485
Operator, compact, study of VI.5 VII.4
Operator, continuity of, discussion of 82—83
Operator, continuity of, in B-spaces II.3.4 59
Operator, continuity of, in F-spaces II.1.14—II.1.16 54
Operator, definition 36
Operator, equality of XII.1 1185
Operator, extensions of VI.2.5 478 554 XII.1
Operator, finite below  XIII.7.25 1455 XIII.7.25 1455
Operator, functions of see "Calculus"
Operator, graph of II.2.3 57 XII.1
Operator, hermitian IV.13.72 350 561
Operator, ideals of 552—553 611
Operator, identity 37
Operator, inverse of XII.1.2 1187
Operator, limits of, in B-spaces II.3.6 60
Operator, limits of, in F-spaces II.1.17—II.1.18 54—55
Operator, matrix of 44
Operator, non-singular 45
Operator, norm of II.3.5 60
Operator, normal VII.2.14 563 IX.3.14
Operator, perturbation of VII.6
Operator, polynomials in VII.1.1 556
Operator, product of 37 XII.1.1
Operator, projection 37 VI.3.1
Operator, projection, study of VI.3
Operator, quasi-nilpotent VII.5.12 581
Operator, range of VI.2.8 479
Operator, range of, with closed range VI.6
Operator, representation of, in 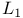 VI.8 VI.8
Operator, representation of, in C VI.7
Operator, representation of, in other spaces 542—552
Operator, resolvent VII.3.1 566
Operator, resolvent, study of VII.3
Operator, self adjoint IX.3.14 879 XII.1.7
Operator, spectral radius of VII.3.5 567
Operator, spectrum of VII.3.1 566
Operator, sum of 37 XII.1.1
Operator, symmetric X.4.1 906 XII.1.7
Operator, unbounded VII.9 Chap.
Operator, unbounded, adjoint of XII.1.4 1188
Operator, unbounded, spectrum and resolvent set of XII.1 1187
Operator, weakly compact, definition VI.4.1 482
Operator, weakly compact, study of VI.4
Operator, zero 37
Order of a pole 230
Order of a pole of an operator VII.3.15 57
Order of a zero 230
Ordered representation, definition X.5.9 916 XII.3.15
| Ordered representation, equivalence of X.5.9 916 XII.3.15
Ordered representation, measure of X.5.9 916 XII.3.15
Ordered representation, multiplicity of X.5.9 916 XII.3.15
Ordered representation, multiplicity sets of X.5.9 916 XII.3.15
Ordered set, definition I.2.2 4
Ordered set, directed set I.7.1 26
Ordered set, partially I.2.1 4
Ordered set, study of I.2
Ordered set, totally I.2.2 4
Ordered set, well I.2.8 7
Orientation of a closed curve 225
Origin of a linear space II.3.1 59
Orihara, M. 395
Orlicz, W. 80 81—82 83 93 94 235 387 388 391—392 400 543
Orthocomplement of a set in Hilbert space, definition IV.4.3 249
Orthocomplement of a set in Hilbert space, properties IV.4.4 249 IV.4.18
Orthogonal complement of a set in a normed space II.4.17 72
Orthogonal complement of a set in a normed space, remarks on 93
Orthogonal elements and manifolds in Hilbert space IV.4.3 249
Orthogonal projections in Hilbert spaces IV.4.8 250
Orthogonal series, exercises on VI.11.43—VI.11.47 537
Orthogonal series, study of IV.14
Orthonormal basis in Hilbert space IV.4.11 252
Orthonormal basis in Hilbert space, cardinality of IV.4.14 253
Orthonormal basis in Hilbert space, criteria for IV.4.13 253
Orthonormal basis in Hilbert space, existence of IV.4.12 252
Orthonormal set in Hilbert space, closed set IV.14.1 357
Orthonormal set in Hilbert space, complete set IV.4.8 250
Orthonormal set in Hilbert space, definition IV.4.8 250
Orthonormal set in Hilbert space, properties IV.4.9—IV.4.16 251—254
Outer measure III.5.3 133
Owchar, M. 406
Oxtoby, J.C. 722 728 729 1152
Pais, A. 1568
Paley, R.E.A.C. 405 406 541 1177 1181 1264
Parallelogram, identity 249
Parker, W.V. 1080
Partial isometry, definition XII.7.4 1248
Partially ordered set, bounds in I.2.3 4
Partially ordered set, completely ordered I.3.9 8
Partially ordered set, definition I.2.1 4
Partially ordered set, directed set I.7.1 26
Partially ordered set, fundamental theorem on I.2.5 5
Partially ordered set, study of I.2
Partially ordered set, totally ordered I.2.2 4
Partially ordered set, well ordered I.2.8 7
Peano, G. 1588
Peck, J.E.L. 471 474
Periodic function (almost periodic function), definition IV.2.25 242
Periodic function (almost periodic function), multiply IV.14.68 367
Periodic function (almost periodic function), study of IV.7
Perron, O. 1078
Perturbation of bounded linear operators, remarks on 611—612
Perturbation of bounded linear operators, study of VII.6 VII.8.1—VII.8.2 VII.8.4—VII.8.5
Perturbation of infinitesimal generator of a semi-group 630—639
Peter — Weyl theorem XI.1.4 940
Peter, F. 940 1145
Pettis, B.J. 81 83—84 88 232 235 318 387 391 473 540—541 543
Phillips' perturbation theorem VIII.1.19 631
Phillips' perturbation theorem, Hille — Yosida — Phillips' theorem VIII.1.13 624
Phillips, R.S. 233 234—235 373 388 390 393 395 462 463 466 541 543 553—554 612 624 726—728 729 883 1274
Phragmen, E. 1043 1115
Pick, G. 1080
Picone, M. 1583 1592
Pierce, R. 395
Pincherle, S. 80
Pinsker, A.G. 395
Pitt, H.R. 729
Plancherel theorem XI.3.9 963 XI.3.20
Plancherel, M. 963
Plessner, A.I. 929 1269 1274
Poincare, H. 607
Pointwise ergodic theorems, k-parameter continuous case in 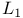 VIII.7.17 708 VIII.7.17 708
Pointwise ergodic theorems, k-parameter continuous case in  , ,  VIII.7.10 694 VIII.7.10 694
Pointwise ergodic theorems, k-parameter discrete case VIII.6.9 679
Pointwise ergodic theorems, one-parameter continuous case VIII.7.5 690
Pointwise ergodic theorems, one-parameter discrete case VIII.6.6 675
Pointwise ergodic theorems, remarks on 729—730
Pointwise Fubini — Jessen theorem III.11.27 209
Poisson summability IV.14.47 363
Poisson, S.D. 363
Polar decomposition of an operator X.9 935
Pole of an analytic function 229
Pole of an operator, criteria for VII.3.18 573 VII.3.20
Pole of an operator, definition VII.3.15 571
Pollard, H. 728 1161 1265
Polynomial in an operator in a finite dimensional space VII.1.1 556
Polynomial in an operator in a general space VII.3.10 568 VII.5.17
Polynomial in an operator, characteristic VII.2.1 561 VII.5.17 VII.10.8
Polynomial of an unbounded closed operator VII.9.6—VII.9.10 602—604
Pontrjagin, L. 47 79 1145 1157 1158 1160
Poole, E.G.C. 1433 1503
Positive definite operator, definition X.4.1 906
POVZNER, A. 1587 1626
Preparation theorem of Weierstrass 232
Price, G.B. 232—233
Principal value integral, definition XI.7.1 1050
Product of B-spaces 89—90
Product of operators 37
Product, Cartesian, of measure spaces III.11 235
Product, cartesian, of sets I.3.11 9
Product, Cartesian, of spaces I.8
Product, Cartesian, topology I.8.1 32
Product, Cartesian, Tychonoff theorem I.8.5 32
Product, intersection of sets 2
Product, scalar, in a Hilbert space IV.2.26 242
Projection and complements 553
Projection and extensions 554
Projection mapping in Cartesian products, continuity and openness I.8.3 32
Projection mapping in Cartesian products, definition I.3.14 9
Projection, definition 37 VI.3.1
Projection, exercises on VI.9.18—VI.9.25 513—514 VI.9.27—VI.9.29
Projection, natural order for VI.3.4 481
Projection, orthogonal or perpendicular IV.4.8 250 482
Projection, study of VI.3
Proper value, definition 606
Ptak, V. 84 466
Putnam, C.R. 934 935 1563 1587 1592 1599 1600 1610
Quasi-equicontinuity and weak compactness IV.6.14 269 IV.6.29
Quasi-equicontinuity for bounded functions IV.6.28 280
Quasi-equicontinuity for continuous functions IV.6.13 269
Quasi-nilpotent operator, definition VII.5.12 581
Quasi-uniform convergence as a criterion for continuous limit IV.6.11 268
Quasi-uniform convergence, definition IV.6.10 268
Quasi-uniform convergence, properties IV.6.12 269 IV.6.30—IV.6.31
Quigley, F.D. 385
Quotient of B-algebras IX.1 866
Quotient, group 35 see
Quotient, space 38
R(T) 567
Rabinovic, Yu.L. 612
Radicals in B-algebras IX.2.5 869
Radius, spectral VII.3.5 567
Radon measure, definition 142
Radon — Nikodym theorem for bounded additive set functions IV.9.14 315
Radon — Nikodym theorem, counterexample III.13.2 222
Radon — Nikodym theorem, general case III.10.7 181
Radon — Nikodym theorem, positive case III.10.2 176
Radon — Nikodym theorem, remarks on 234
Radon, J. 142 176 181—182 234 380 388 392 539 543
Raikov, D.A. 1152 1160 1274
Ramaswami, V. 884
Range of an operator VI.2.8 479
Range of an operator, closed, criterion for VII.4.1 577
Range of an operator, closed, study of VI.6 VI.9.15 VI.9.17
Range of an operator, remarks on 539
Rapoport, I.M. 1587
Rasevskii, P.K. 1149
Rayleigh equation X.4 907
Rayleigh, Lord 611 907 928
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 -, fundamental family of
-, fundamental family of  -norms
-norms