|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Faith C. — Rings and Things and a Fine Array of Twentieth Century Associative Algebra |
|
|
 |
| Предметный указатель |
Lie 87
Lie algebra (ring) 2.42s
Lie simple 2.42f
Lift/rad ring 3.30f
Ligh 30
Lilly, Eli 259
Linear compact (= l.c) 5.4Af
Linear transformation (= l.t.) 2.6 3.8A
Linearly compact module 5.4Af
Local FPF ring theorem 4.30
Local ring 3.14f (Cf. p.85)
Local, complete 5.4B
localization 12.3s
Locally split submodule 6.16s
Loewy 69
Loewy length 3.33as
Loewy module 3.33As
Los theorem 1.21A
Louden 175
Lowenstein-Skolem 134
Lower nil radical 2.38A
Lucas 167
Lucas theorem 9.28 9.35
Lueroth theorem 1.29
Lyons, Richard 300—302 302n
m-adic topology 16.Af
m.c. see "Closed"
Macdonald 181
MacEacharn 211
Mack, Mary Ellen 258
MacLane 52 77 206
MacLane theorem 5.15B
MacLane, Saunders 255
Maclean 166
Mal’cev 49 87 94 122 129 132
Mal’cev domain 6.28s
Mal’cev theorem 6.28
Manis 165
Manis valuation ring 9.10s
Mares 68
Marot 168
Marot ring 9.20s
Martindale 48 101 254
Martindale quotient ring §17 Notes
Maschke 175
Maschke theorem §10
Mastrian, Barbara 258
Matlis 63 85 93 159 231
Matlis problem 8.Hf 8.3
Matlis theorem 5.4B 5.4C 5.5 6.7A 6.9—6.10 6.19 13.4C
Matlis — Papp theorem 3.4C 8.1
Matlis, Eben 281
Matrix units 2.16D—2.16E 4.6B
Matrix, cancellable §10 p.174
Matrix, ring §2 2.16D—2.16J
Matsumura 213
Max module 7.27s
Max pair 9.10s
Max ring 3.32f
Maximal annihilator ideal 2.37Es
Maximal annulet (= maxulet) 2.37Es
Maximal completion 5.14As
Maximal completion of a valuation ring 5.14 As
Maximal condition 2.17As
Maximal essential extension 3.2D 3.2E
Maximal ideal 2.37s
Maximal order 4.28
Maximal principle 2.17B
Maximal quotient ring 9.27s 12.0Ass
Maximal regular ideal 4.4
Maximal ring 5.4Bs
Maximal valuation ring 5.4D
Maxulet 2.37Es 16.30s
Mazur, Barry C. 316n
Mazur, the "Famous" 316n
McAdam 233
McCarthy 164
McCarthy theorem 4.1C
McConnell 214
McConnell theorem 14.46
McCoy 40 94
McCoy rings 6.38f
McCoy Theorem 2.36 2.37B 6.40 16.1—16.2
McKenzie, Ralph 291
McKnight, J.D., Jr. ("Jim") 260
McLaughlin theorem 11.9
Megibben theorem 3.7
Meiss, Millard 283
Menal 30 65 101 104 121 123 157 159 169
Menal conjecture 13.35s
Menal theorem 4.16A 4.17B 6.3J 13.19 13.20 13.32—13.33
Menal — Moncasi theorem 6.3E
Menal — Vamos theorem 6.1 6.22—6.23
Menal, Pere xxix 287 308—310
Menini 127 197
Menini, Claudia xxix
Merzljakov 88
Meyberg 68
Michler 60 65 86 95 148 181 227 see
Michler — Villamayor theorem 7.38s
Mikhalev, A.B. 296—297
Mikhovski (Mihovski) 253—254
Mikhovski (Mihovski) theorem §17 Notes
Millay, Edna St. Vincent 323
Miller, Barbara xxix 258
Milnor 88
Milnor, John ("Jack") 269 312
Minimal prime ideal 2.22s 2.36A
Minimum condition on a module 2.17As
Mintz, Rose ("Rosie") 306
Miro, Joan 311n
Mitchell 52 77
Mitchell, Barry 305
Mittag-Leffler 269
Miyashita 60
mod-R 3.51
Module, aleph or 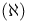 -generated 1.1 -generated 1.1
Module, algebraically compact 1.25s
Module, balanced 3.50Bf
Module, basic 3.52
Module, character 4.Bs
Module, compact faithful (CF) 3.9 3.62As
Module, completely decomposable 8.As
Module, completely injective 7.32s
Module, counter 16.9Cs
Module, cyclic presented 6.5A
Module, divisible 1.10
Module, dual 1.5
Module, essential over 3.2Ds
Module, essential sub 3.2Ds
Module, faithful 2.6s
Module, flat 4.As
Module, genus of a 5.23As
Module, indecomposable 1.2 8.As
Module, injective 3.2s
Module, irreducible 2.18as 16.9A
Module, irreducible sub 3.14B 16.9A
Module, linearly compact 5.4Af
Module, Loewy 3.33As
Module, nonsingular 4.1Es
Module, principal cyclic 3.30f
Module, projective 3.1As
Module, pseudo 6.36As
Module, pure-injective 1.25s 6.Af 6.46—6.57
Module, quasi-injective 3.9As
Module, quotient finite dimensional (= q.f.d.) see "Listing"
Module, radical of a 3.19As
Module, semiartinian 3.33As
Module, sigma 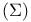 -cyclic 5.1As -cyclic 5.1As
Module, sigma 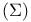 -injective 3.7As 3.14—3.16 7.33—7.34 -injective 3.7As 3.14—3.16 7.33—7.34
| Module, sigma or  -completely 7.33—7.34 -completely 7.33—7.34
Module, singular 4.1Es
Module, special 7.24s
Module, subdirect product of 2.6f
Module, subdirectly irreducible 2.17D
Module, torsionfree 1.6
Module, torsionless 1.5
Module, uniform 3.14A
Module, uniserial 5.1A'f
Mohammed 188—189
Mohammed — Sandomierski theorem 12.10
Moncasi 30 123
Moncasi, Jaume 309—310
Monk theorem 8.4E
Monroe, Marilyn 265
Montgomery see "Cohen"
Montgomery example 4.6D
Montgomery theorem §17 Notes
Montgomery, Deane 267—269
Montgomery, Kay 267
Montgomery, s. 48
Moore, E.H. 258
Morita 18 76—78 108 161 177 194 254
Morita duality 13.1s 13.4A
Morita equivalence 3.51f §17
Morita theorem 3.51 11.11s 13.4A 13.7 13.30F
Morita, Kiiti 320
Morse, Louise 266
Morse, Marston 264—266 275
Moskowitz, Morris ("Moe") 256n
Moss see "Ginn"
Mostert, Paul 256 260
Mostow 49
Mostow theorem 2.52f
MP (Matlis’ Problem) see "Matlis"
Mueller (Mueller, B.) theorem 13.1 13.5 13.27
Mueller, B. 68 108 181 188—189 197
Mueller, W. 103
Multiplicatively closed (= m.c.) subset 3.16Bf 3.17 12.3As
Murase theorem 5.2C
Myashita 60
Mycielski 121
Nagahara 24 251
Nagao 218
Nagata 34 74 85 108 167 210
Nagata Theorem 2.21Bf 9.24(1) 14.21
Nagata — Higman theorem 3.43Ass
Nakayama 24 30 55 79 218
Nakayama lemma 3.35
Nakayama theorem 2.13 5.2B 13.7 13.15A
Nakayama — Asano theorem 2.13 5.2A
Nakayama — Faith theorem 2.13 5.2A 13.7A—13.7B
Nastasescu 60 70 194 see
Nastasescu — Popescu theorem 3.33D
Neider, Charles 268 295
Neider, Joan 268 295
Neider, Susy 295
Nelson, Edward ("Ed") 282
Neroslavskii 141 see
Nesbitt 78
Nesbitt — Thrall theorem 13.30A
Netanyahu 288
Neumann, B.H. 176
Newton, H. 258
NFI module 7.38 7.43
Nicholson 68 122 157 158
Nicholson theorem 8.4B 8.4C
Nietzsche, Friedrich 297
Nil ideal 2.6s 2.34As
Nil radical 2.34s 2.35s
Nil ring 2.34As
Nilpotent element 2.6s
Nilpotent ideal 2.34As 3.37s
Nilpotent ring 12s 2.34As
Nilpotent, essentially 3.80
Nilpotent, locally 2.34B
Nilpotent, properly 2.29f
Nilpotent, strongly 2.33s
Nobusawa 24
Noether  -ring 3.15As -ring 3.15As
Noether depth 16.33s
Noether module 2.17As
Noether prime ideal, sup. 3.15B
Noether problem 2.21 2.21Bf
Noether ring 2.2s 2.17As
Noether spectrum (= spec) 14.35
Noether theorem 2.5A 2.18
Noether — Lasker theorem 2.27
Noether, E. 18—19 23 33 39 86 242
Noether, Emmy 285
Noether, locally 7.23
Noether, piecewise 9.36s
Nonsingular module 4.1Es
Nonsingular ring 4.1Es 5.3Ds §12
Northcott 206 213
Norton see "Hajarnavis"
Nouaze — Gabriel theorem 14.28B
Nullstellensatz, weak 3.36B
Nunke theorem 1.21A 1.21Bf
O'Keefe, Georgia xxix 306
O'Nan, Michael ("Mike") 300
O'Neill, John xxix
Oberst 68
Oberst, Ulrich 277
Odnoff, Erik 257
Oehmke, Bob 261
Oehmke, Theresa 261
Ohm 231
Onodera 122 133 251
Onodera theorem 13.14A 13.30F
Oppenheim, Joseph ("Joe") 283
Oppenheimer, J. Robert 270 281
Oppenheimer, Kitty 281
Opposite ring (algebra) 2.5Bf 2.43s 4.13
Order of a group 2.6ff
Order, linear see "Chain module (ring)"
Order, maximal 4.28
Order, reduced 2.7s
Ore 142
Ore condition 3.12Bf 6.26
Ore domain 6.26f
Ore ring 3.12Bf 7.35s
Ore theorem 6.26 7.14ff
Ornstein 139
Ornstein theorem 3.20
Ornstein, Ahuva 288
Ornstein, Avraham 288
Orsatti 194
Orthogonal idempotents 1.2
Orton, William ("Bill") 294
Oshiro 188 195
Osofsky 66 70 92 103 153 161 166 179 201 207 210
Osofsky example 4.24
Osofsky ring 3.4' f
Osofsky theorem 3.3 3.18A 4.2A 4.20 4.22 4.22ff 12.4C' 13.2 14.15.7
Osofsky — Smith Theorem 12.4C 12.4C' 12.4F—12.4G
Osofsky, Abe 277
Osofsky, Barbara 258 277 283—284 308—309
Osterburg see "Fisher" "Snider"
Ostrowski 111
O’Meara 95
O’Neill 9 67 123
O’Neill theorem 1.21Cff
p-Adic completion 5.4B 13.4C
p-adic integers 4.24 (Cf. p.85)
p-injective module 6.Es
Page, S. 104 171 287
Page, S. theorem 5.49—5.51
Pais, Abraham 278—279
Papp 63 159 see
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



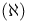 -generated
-generated 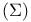 -cyclic
-cyclic  -completely
-completely  -ring
-ring