Авторизация
Поиск по указателям
Rektorys K. — Survey of Applicable Mathematics.Volume 2.
Обсудите книгу на научном форуме Нашли опечатку?
Название: Survey of Applicable Mathematics.Volume 2.Автор: Rektorys K. Аннотация: This major two-volume handbook is an extensively revised, updated second edition of the highly praised Survey of Applicable Mathematics, first published in English in 1969.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Издание: 2ndГод издания: 1994Количество страниц: 978Добавлена в каталог: 30.08.2014Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Bounded sequence I 339 Bounded set II 321 II Bounded variation, function of I 370 Bounds of real numbers I 5 Brachistochrone problem II 382 Branch of a multivalued function II 276 II Branch, point, algebraic (of finite order) II 274 Branch, point, transcendental (of infinite order) II 274 Branches of hyperbola I 119 Budan — Fourier theorem II 651 Bundle of planes I 204 C-region II 308 Calculus of observations II 778 II Calculus of observations, adjusted value II 779 Calculus of variations II 374 ff Calculus of variations, brachistochrone problem II 382 Calculus of variations, canonical form of Euler equations II 407 Calculus of variations, categories of problems with constraints II 405 Calculus of variations, categories of problems with generalized constraints II 406 Calculus of variations, categories of problems, elementary II 374 Calculus of variations, categories of problems, functional depending on functions of n variables II 392 Calculus of variations, categories of problems, Lagrange II 406 Calculus of variations, categories of problems, moving (free) ends of admissible curves II 395 Calculus of variations, categories of problems, parametric II 403 Calculus of variations, categories of problems, simplest case of isoparametric problem II 399 Calculus of variations, curves of r-th class (of class II 375 II Calculus of variations, distance of order r of curves II 376 II Calculus of variations, distance of order r of hypersurfaces II 392 II Calculus of variations, epsilon II 376 II Calculus of variations, Euler equation and special cases II 381 II Calculus of variations, Euler — Ostrgradski equation II 394 Calculus of variations, Euler — Poisson equation II 389 Calculus of variations, extremal of variational problem II 381 Calculus of variations, functions of class II 375 II Calculus of variations, Hamilton, differential equations II 407 Calculus of variations, Hamilton, function II 407 Calculus of variations, isoperimetric problem II 399 Calculus of variations, Lagrange variational problem II 406 Calculus of variations, Legendre tranformation II 407 Calculus of variations, necessary conditions for extremum II 381 II II II II II II II Calculus of variations, positive homogeneous functions II 403 Calculus of variations, problems with constraints II 405 Calculus of variations, problems with moving ends II 395 Calculus of variations, problems, parametric II 403 Calculus of variations, regular hypersurface II 392 Calculus of variations, system of, Euler equations II 387 II Calculus of variations, system of, Euler — Poisson equations II 391 Calculus of variations, transversality conditions II 396 II Calculus of variations, variation of functional in Du Bois-Reymond form II 380 Calculus of variations, variation of functional in Lagrange form II 380 Calculus, differential I 359 ff Calculus, integral I 448 ff Calculus, operational II 567 ff Calculus, tensor I 242 ff Calculus, vector I 225 ff Camp — Meidell inequality II 729 Canonical correlation II 785 Canonical form of Euler equations II 407 Canonical system of differential equations II 99 Cantelli inequality II 729 Caratheodory region II 308 Cardioid I 132 Cartesian coordinates in plane geometry I 167 Cartesian coordinates in plane geometry, congruent transformations I 186 Cartesian coordinates in plane geometry, relations with polar coordinates I 179 Cartesian coordinates in solid geometry I 195 Cartesian coordinates in solid geometry, relations with cylindrical and spherical coordinates I 197 Cartesian coordinates in solid geometry, singular points I 198 Cartesian coordinates in solid geometry, transformation by translation, rotation and reflection I 198 ff Cartesian product of sets I 45 Cask volume formulae I 111 Cassinian ovals I 151 Catenaries (chainettes) I 145 Catenaries (chainettes), constant strength I 147 Catenaries (chainettes), general I 145 Catenaries (chainettes), involute of (called tractrix) I 147 Cauchy — Dirichlet formulae II 37 Cauchy — Kovalewski theorem II 151 Cauchy — Riemann, equations, for functions of one complex variable II 246 Cauchy — Riemann, equations, for functions of several complex variables II 283 Cauchy — Riemann, integrals I 513 Cauchy — Schwarz inequality I 533 Cauchy, continuity definition I 366 Cauchy, form of Taylor theorem I 397 Cauchy, inequality I 8 I Cauchy, integral formula and theorem, for functions of one complex variable II 252 II Cauchy, integral formula and theorem, for functions of several complex variables II 285 Cauchy, integrals, type of II 255 Cauchy, method II 165 Cauchy, principal value of integral I 524 I II Cauchy, problem for partial differential equations II 150 II II Cauchy, product of series I 354 Cauchy, root test for convergence of series I 347 Cauchy, sequence II 327 Cauchy, theorem I 349 II II Cea lemma II 424 Censoring II 789 II Censoring, censored random sample II 789 Censoring, censored random sample, method of maximum likelihood for II 790 II Censoring, censored random sample, nonparametric estimation for II 792 Censoring, Kaplan — Meier (product-limit) estimator II 791 Censoring, random II 790 Censoring, type I (time) II 789 Censoring, type II (failure) II 789 Central difference II 680 Central element II 843 Central limit theorems II 730 II II Centre of curvature I 286 I Centre of curvature, construction for cyclic curves I 136 Centre of gravity, curves in space I 620 Centre of gravity, plane curves I 619 Centre of gravity, plane figures I 623 Centre of gravity, solids I 627 Centre of gravity, surfaces I 631 Centre, (singular point of differential equation) II 27 Centroids, plane figures I 95 ff Centroids, solids I 104 ff Cesaro summable series I 354 Chain of regions II 275 Chain, rule I 382 Chainettes see "Catenaries" Change of order of differentiation I 408 Chapman — Kolmogorov equations II 800 II Characteristic curve of family I 319 Characteristic direction II 152 Characteristic equation II 58 II Characteristic exponent II 50 Characteristic function II 81 II II II II Characteristic matrix of Jordan block I 60 Characteristic matrix of square matrix I 59 Characteristic polynomial II 50 Characteristic polynomial of k-step method II 498 Characteristic polynomial of matrix I 59 II Characteristic row II 842 Characteristic strip II 166 Characteristic value in eigenvalue problem II 59 II II Characteristic value in eigenvalue problem of integral equation II 225 Characteristic value in eigenvalue problem of matrix I 59 II Characteristics II 152 Characteristics of random variable II 697 Characteristics of random vector II 708 Characteristics, sample (empirical) II 736 ff Characteristics, theoretical II 736 Chasles theorem I 321 Chebyshev alterning, property II 670 Chebyshev alterning, set II 670 Chebyshev approximation II 669 Chebyshev equation I 712 Chebyshev expansion II 674 Chebyshev inequality II 729 Chebyshev polynomials I 711 II II II Chebyshev theorem II 669 Chi square test II 761 Choleski factorization II 600 circle I 113 I Circle (=disc) II 320 Circle, circumscribed on triangle I 80 Circle, closed II 321 Circle, conchoid of I 153 Circle, constructions of I 112 Circle, diameter, bounded and conjugate I 113 Circle, equation of I 181 Circle, equation of, in polar coordinates I 182 Circle, formulae for geometrical elements of I 99 Circle, inscribed in triangle I 80 Circle, involute of I 134 Circle, Involute of, curtate and prolate I 135 Circle, of curvature I 285 Circle, open II 320 Circle, parametric equations of I 181 Circle, rectification of, Kochanski and Sobotka I 113 I Circle, superosculating I 287 Circle, Thalet I 113 Circular cask, volume formula I 111 Circular frequency I 156 Circumferences, formulae for plane figures I 95 Cissoid of Diocles I 149 Clairaut differential equation II 32 Clairaut differential equation, generalized II 163 class II 742 Class, frequency II 742 Class, intervals (cells) II 742 Classical solution of partial differential equations II 149 II Classification, one-way II 782 II Classification, two-way II 782 Clausen transformation I 353 Closed (completed, extended) plane of complex numbers II 243 Closed circle I 402 II Closed curve I 261 Closed disc II 320 Closed interval I 359 Closed problem II 90 Closed region I 402 II Closed set II 321 II Closed subspace II 331 Closed system in Hilbert space II 337 Closure of a set in Euclidean space II 321 Closure of a set in metric space II 326 Clothoid I 141 Cluster point II 319 Codazzi fundamental equations for surfaces I 333 Coefficient(s) of determination II 771 Coefficient(s) of kurtosis (excess) II 702 Coefficient(s) of quadrature formula I 555 Coefficient(s) of skewness II 702 Coefficient(s) of variation II 702 Coercive functional II 370 Coercive operator II 372 Cofactor in determinant I 30 Collatz theory II 78 ff Combinations, definition and theorems I 18 Common logarithms I 15 Commutative groups and rings I 47 ff Commutative laws governing vectors I 226 I Compact operator II 351 Compact space II 329 Compact support II 339 Comparison, function of eigenvalue problem II 82 Comparison, test for convergence of series I 346 Comparison, theorem II 47 II Complement of a set II 322 Complementary subaspace II 335 Complete analytic function II 276 Complete hull II 410 Complete induction I 2 Complete integral II 161 Complete Reinhardt domain II 280 Complete sequence II 338 Complete space II 327 Complete system in Hilbert space II 337 Complete system of eigenvectors II 356 II II II Completely continuous operator (mapping) II 351 Completion of metric space II 327 II complex derivative II 282 Complex differential II 282 Complex differentiate function II 282 Complex function of real variable II 222 complex numbers I 9 ff Complex numbers, absolute value (modulus) of I 10 Complex numbers, conjugate of I 10 Complex numbers, principal value of argument I 11 Complex numbers, trigonometric form I 10 ff Complex potential of flow II 248 II Complex space I 668 II Complex variable, functions of I 243 ff Complex variable, functions of, application of the theory of functions II 248 II Complex variable, functions of, Cauchy integral theorem and formula II 252 II II II Complex variable, functions of, derivative II 246 II Complex variable, functions of, fundamental concepts II 243 ff Complex variable, functions of, integral of II 250 Complex variable, functions of, limit and continuity II 245 II Complex variable, functions of, logarithm and power II 272 ff Composite functions I 361 I Composite functions, continuity I 368 I Composite functions, differentiation I 382 I Composite functions, limit I 372 Composite quadrature formula I 556 Computation with small numbers I 398 ff Concavity and convexity I 391 Conchoid of circle I 153 Conchoid, Nicomedes I 152 Condition of minimal angle II 448 Condition, number of matrix II 605 Cone, frustum of, and its centroid I 108 I Cone, right circular I 108 Cone, virtual I 216 Cone, volume, surface areas, moment of inertia I 108 I Confidence, interval, one-sided and two-sided II 752 Confidence, level I 752 Confidence, limits, lower and upper II 752 Confidence, region II 753 Conformal mapping II 289 ff Conformal mapping of n-tuply connected regions II 295 Conformal mapping, "adjacent" regions II 310 Conformal mapping, boundary correspondence principle II 300 Conformal mapping, boundary properties II 304 Conformal mapping, Caratheodory region II 308 Conformal mapping, concept of II 289 Conformal mapping, dictionary of II 312 ff Conformal mapping, eccentric cylindrical condenser II 298 Conformal mapping, ellipse on circle II 316 II Conformal mapping, existence amd uniqueness II 293 Conformal mapping, extremal properties II 303 Conformal mapping, flow round an obstacle II 299 Conformal mapping, homographic II 291 Conformal mapping, hyperbola on upper half-plane II 315 II Conformal mapping, infinite strip with a cut on infinite strip II 313 Conformal mapping, Joukowski airofoils II 297 Conformal mapping, methods of performing II 296 ff Conformal mapping, methods of performing, by integral equations II 308 Conformal mapping, methods of performing, examples II 296 ff Conformal mapping, methods of performing, small parameter II 305 Conformal mapping, methods of performing, variational II 305 Conformal mapping, parabola on upper half-plane II 314 II Conformal mapping, plane with segments, on annulus II 317 Conformal mapping, plane with segments, on plane with segments II 318 Conformal mapping, Riemann theorem II 293 Conformal mapping, Riemann — Schwarz reflection principle II 301 Conformal mapping, Schwarz — Christoffel theorem II 302 Conformal mapping, sector of circle on upper half-plane II 314
Реклама
) 
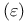-neighbourhood of order r of curve 
|
|
О проекте