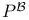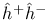|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Silverman J. — The arithmetic of dynamical systems |
|
|
 |
| Предметный указатель |
Algebraic set 90
Algebraic set, attached to an ideal 90
Algebraic set, ideal of 90
Algebraic variety 89 147 159
Algebraic variety, dynamically affine 376
Algebraic variety, quotient by finite group 161
Algebraic variety, quotient by infinite group 161 174
Algebraically closed field 26 242
Algebraically closed field,  is an 239 is an 239
Algebraically integrable map 430
Algebraically reversible affine automorphism 429
Algebraically stable morphism 396 433
Algebraically stable morphism, degree 396
Algebraically stable morphism, indeterminacy locus 396
Ample divisor 406
Ample divisor, bounded height is finite set 408
Analytic function see Holomorphic function
Annulus 302
Archimedean absolute value 83
Arithmetic complexity, height in  221 221
Arithmetic complexity, minimal discriminant 221
Arithmetic complexity, of orbit on K3 surface 435
Arithmetic function 6
Arzel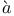 — Ascoli theorem 25 — Ascoli theorem 25
Attracting basin of  74 138 140 141 74 138 140 141
Attracting periodic point 19 47 326 362
Attracting periodic point, accumlates on a critical point 78
Attracting periodic point, basin of attraction 310
Attracting periodic point, every periodic point is an 78
Attracting periodic point, immediate basin of attraction 310
Attracting periodic point, in Berkovich space 310 324
Attracting periodic point, in Fatou set 22 40
Attracting periodic point, limit is repelling 274
Attracting periodic point, of Type I 310
Attracting periodic point, reduction is critical 78
Automorphism group 340 341
Automorphism group, 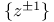 205 234 205 234
Automorphism group, containing 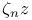 205 234 205 234
Automorphism group, contains 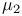 235 235
Automorphism group, cyclic 197 204
Automorphism group, dihedral 217 234 327
Automorphism group, extension of cocycle to  203 203
Automorphism group, involution 410
Automorphism group, is finite 196
Automorphism group, list of possible 197
Automorphism group, no twists if trivial 198
Automorphism group, noncommuting involutions 410 437
Automorphism group, of 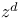 234 234
Automorphism group, of Chebyshev polynomial 332 336
Automorphism group, of Chebyshev polynomial, in characteristic  381 381
Automorphism group, of conjugate 196 234
Automorphism group, of elliptic curve 365
Automorphism group, of monomial map 327
Automorphism group, of monomial map, in characteristic  380 380
Automorphism group, of power map 327
Automorphism group, of power map, in characteristic  380 380
Automorphism group, of projective line 10
Automorphism group, order two 198 200
Automorphism group, points stabilized by 425 434
Automorphism group, polynomial map with nontrivial 234
Automorphism group, rational map 196
Automorphism group, symmetric group 197
Automorphism group, trivial for most maps 199 234
Automorphism, action on critical point 234
Automorphism, affine 390 427
Automorphism, affine regular 394
Automorphism, algebraically reversible 429
Automorphism, canonical height 431
Automorphism, finitely many periodic points 435
Automorphism, height inequality 399 431
Automorphism, height of periodic points 402
Automorphism, induced by degree two map 411 436 437
Automorphism, is subgroup of  234 234
Automorphism, nondegenerate 226
Automorphism, of order two 96 197 235
Automorphism, rational 430
B zivin’s theorem 274 275 zivin’s theorem 274 275
B zout’s theorem 428 zout’s theorem 428
Backward orbit, dense for shift map 314
Backward orbit, dense in Berkovich Julia set 311
Backward orbit, dense in Julia set 267
Backward orbit, equidistributed 315
Backward orbit, equidistributed for shift map 315
Backward orbit, of rational map 109 142
Bad reduction, becomes good reduction 218
Bad reduction, Berkovich Julia set of map with 307 311
Bad reduction, complex dynamics has 294
Bad reduction, composition of maps 77
Bad reduction, composition of maps can be good 77
Bad reduction, Green function of map with 294 318
Bad reduction, of 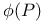 is not is not 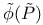 11 11
Bad reduction, product of primes of 221
Baire category theorem 268
Baker’s theorem 107 155
Banach algebra 298
Baragar’s theorem 443
Base locus 406 408
Basin of attraction 74 138 140 141 310
Basin of attraction, immediate 310 311
Benedetto’s theorem 284-286
Berkovich affine line 301
Berkovich affine line, as ringed space 304
Berkovich affine line, contained in Berkovich projective line 303
Berkovich affine line, contains  302 304 323 302 304 323
Berkovich affine line, Gel’fond topology restricts to  topology 304 323 topology 304 323
Berkovich affine line, has no Gauss point 302
Berkovich affine line, is Hausdorff 304
Berkovich affine line, is locally compact 304
Berkovich affine line, is tree 302
Berkovich affine line, is uniquely path connected 304
Berkovich affine line, no Type-lV fixed points 321
Berkovich affine line, polynomial map 304
Berkovich disk 295
Berkovich disk, annulus 302
Berkovich disk, base of open sets 300
Berkovich disk, big model 323
Berkovich disk, branch 300
Berkovich disk, closed branch 300
Berkovich disk, closed of radius 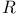 297 301 297 301
Berkovich disk, closed unit 297
Berkovich disk, contains unit disk in  295 301 323 295 301 323
Berkovich disk, dynamics of 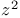 307 307
Berkovich disk, Gauss point 297 301
Berkovich disk, Gel’fond topology 300 322
Berkovich disk, Gel’fond topology restricts to  topology 301 323 topology 301 323
Berkovich disk, good reduction polynomial map 321 322
Berkovich disk, Hsia kernel 323
Berkovich disk, is compact 295 300 304
Berkovich disk, is connected 295
Berkovich disk, is Hausdorff 300 304
Berkovich disk, is metric space 295
Berkovich disk, is path-connected 300
Berkovich disk, is set of bounded seminorms 297
Berkovich disk, is set of seminorms 296
Berkovich disk, is uniquely path connected 304
Berkovich disk, Julia set of good reduction map 322
Berkovich disk, line segment from Gauss point to Type-lV point 322
Berkovich disk, line segment in 298
Berkovich disk, neutral fixed point 322
Berkovich disk, of radius 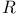 297 301 297 301
Berkovich disk, open branch 300
Berkovich disk, potential theory 323
Berkovich disk, radius of Type — IV point is positive 296
Berkovich disk, seminorm associated to each type of point 297
Berkovich disk, small model 323
Berkovich disk, topology on 299
Berkovich disk, union of annulus and open branch 302
Berkovich disk, visualizing 298
Berkovich Julia set, backward orbit dense in 311
Berkovich Julia set, equals closure of repelling periodic points 311
| Berkovich Julia set, has empty interior 311
Berkovich Julia set, is connected or infinitely many components 311
Berkovich Julia set, is perfect set 307 311
Berkovich Julia set, is uncountable 307 311
Berkovich projective line 301 302
Berkovich projective line, as homogeneous two-variable seminorms 303 305
Berkovich projective line, as ringed space 304
Berkovich projective line, attracting fixed point 324
Berkovich projective line, basin of attraction 310
Berkovich projective line, branch at infinity 302
Berkovich projective line, canonical measure 306
Berkovich projective line, canonical measure, support of 307
Berkovich projective line, contains  304 323 304 323
Berkovich projective line, contains Berkovich affine line 303
Berkovich projective line, domain of quasiperiodicity 310 311
Berkovich projective line, dynamics of 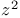 307 307
Berkovich projective line, Fatou set 306 311
Berkovich projective line, Fatou set, contains classical 306
Berkovich projective line, Gel’fond topology restricts to  topology 304 323 topology 304 323
Berkovich projective line, immediate basin of attraction 310 311
Berkovich projective line, is compact 304
Berkovich projective line, is Hausdorff 304
Berkovich projective line, is uniquely path connected 304
Berkovich projective line, Julia set 306
Berkovich projective line, Julia set, contains classical 306
Berkovich projective line, Julia set, equals closure of repelling periodic points 311
Berkovich projective line, Julia set, is nonempty 307
Berkovich projective line, Julia set, of bad reduction map 307 311
Berkovich projective line, Julia set, of good reduction map 307 311 322
Berkovich projective line, Montel theorem 310 311
Berkovich projective line, rational map 305
Berkovich projective line, recurrent point 310
Berkovich space 294
Berkovich space, attracting fixed point 324
Berkovich space, attracting periodic point 310
Berkovich space, basin of attraction 310
Berkovich space, canonical measure 6 306
Berkovich space, canonical measure, support of 307
Berkovich space, connectivity of 276
Berkovich space, different centers yield same point 295
Berkovich space, domain of quasiperiodicity 310 311
Berkovich space, dynamics of 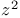 307 307
Berkovich space, dynamics on 304
Berkovich space, Fatou set 306 311
Berkovich space, fixed Gauss point 321
Berkovich space, Gauss point 297
Berkovich space, Gel’fond topology 322
Berkovich space, good reduction polynomial map 321 322
Berkovich space, Hsia kernel 323
Berkovich space, immediate basin of attraction 310 311
Berkovich space, invariant measure 104
Berkovich space, is set of seminorms 296
Berkovich space, Julia set 306
Berkovich space, Julia set, equals closure of repelling periodic points 311
Berkovich space, Julia set, is nonempty 307
Berkovich space, Julia set, of bad reduction map 307 311
Berkovich space, Julia set, of good reduction map 307 311 322
Berkovich space, Laplacian of local canonical height 104
Berkovich space, line segment from Gauss point to Type — IV point 322
Berkovich space, Montel theorem 310 311
Berkovich space, neutral fixed point 322
Berkovich space, path metric 306 323
Berkovich space, potential theory 323
Berkovich space, radius of a point 296
Berkovich space, radius of Type — IV point is positive 296
Berkovich space, recurrent point 310
Berkovich space, repelling periodic point 310
Berkovich space, rigid analytic space 298
Berkovich space, seminorm associated to each type of point 297
Berkovich space, topology on 299
Berkovich space, Type – I-IV points 295
Bicritical rational map 233
Bidegree 405 410
Bifurcation point 165
Bifurcation polynomial 165
Bifurcation polynomial, is a power 165 226 227
Big model of Berkovich disk 323
Big-Oh notation 93
Bihomogeneous polynomial 405 410
Binomial theorem 206 261
Birational map on Markoff variety 437
Bogomolov conjecture 129
Bogomolov conjecture, dynamical 129
Borel probability measure 127
Bounded height, finitely many points of 86 407
Bounded height, is finite set 408
Bounded height, Misiurewicz points have 167
Bounded seminorm on ![$\mathbb{C}_{p}[z]$](/math_tex/438e8f6f1b977bfdcd9bc501d9bcdbb582.gif) 296 296
Bounded seminorm, automatically nonarchimedean 321
Bounded seminorm, properties 321
Bounded seminorm, set of is Berkovich disk 297
Branch at infinity in 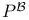 302 302
Branch of Berkovich disk 300
Brauer group 204
Brauer Siegel theorem 368
Brolin measure 307
Bulb 166
Canonical divisor 214
Canonical height 97 99 100 287
Canonical height, algorithm to compute 99 318
Canonical height, associated to an eigendivisor class 104
Canonical height, commuting maps have same 137
Canonical height, conjugation invariance 137
Canonical height, finitely many points of bounded 423
Canonical height, infinitely many points of bounded 435
Canonical height, is sum of Green functions 290 318
Canonical height, Lehmer’s conjecture 101 138
Canonical height, local 102 291 320
Canonical height, local for a polynomial map 140 141
Canonical height, lower bound for 101 137 138 221
Canonical height, normalization conditions 422
Canonical height, normalized local 141
Canonical height, of point in Fatou or Julia set 138
Canonical height, on abelian variety 409
Canonical height, on elliptic curve 409
Canonical height, on K3 surface 421 423 426 435 436
Canonical height, on K3 surface, with three involutions 438
Canonical height, product 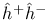 435 435
Canonical height, quadratic map 137 138
Canonical height, regular affine automorphism 431
Canonical height, sum of local canonical heights 103 293
Canonical height, Tate construction 97
Canonical height, transformation formulas 422 432
Canonical height, uniqueness 98
Canonical height, zero iff finite orbit 423 431
Canonical height, zero iff preperiodic point 99
Canonical height, zeta function 436
Canonical measure 127 306
Canonical measure, on Berkovich space 306
Canonical measure, support is Julia set 307
Canonical measure, supported at Gauss point 307
Cardiod 166
Cauchy estimate for norm of derivative 252 313
Cauchy residue theorem 20 248 314
Cauchy sequence 98 240 296
Chain rule 18 360
Chain rule, calculate multiplier with 19 47
Change of variables 11
Chaos 3 22
Chebyshev polynomial 29 30 95 329
Chebyshev polynomial, algebraic properties 41 329
Chebyshev polynomial, alternative normalization 30 331
Chebyshev polynomial, automorphism group 332 336
Chebyshev polynomial, automorphism group, in characteristic  381 381
Chebyshev polynomial, binomial coefficient identity 380
Chebyshev polynomial, commutativity 41 329 332 378
Chebyshev polynomial, defining equation 30
Chebyshev polynomial, derivative identity 381
Chebyshev polynomial, derivative in characteristic 2 381
Chebyshev polynomial, differential equation characterizing 334
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 is an
is an 
 — Ascoli theorem
— Ascoli theorem 

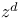

 zivin’s theorem
zivin’s theorem 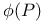 is not
is not 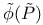
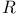
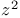

![$\mathbb{C}_{p}[z]$](/math_tex/438e8f6f1b977bfdcd9bc501d9bcdbb582.gif)