Авторизация
Поиск по указателям
Zeidler E. — Nonlinear Functional Analysis and Its Applications: Part 1: Fixed-Point Theorems
Обсудите книгу на научном форуме Нашли опечатку?
Название: Nonlinear Functional Analysis and Its Applications: Part 1: Fixed-Point TheoremsАвтор: Zeidler E. Аннотация: This is the first of a five-volume exposition of the main principles of nonlinear functional analysis and
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1986Количество страниц: 897Добавлена в каталог: 22.12.2011Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
171 147 604 160 324 160 160 324 A priori estimates, abstract forms of 265 A priori estimates, and existence 227 A priori estimates, and global solutions for ordinary differential equations 79 A priori estimates, basic ideas of 227ff A priori estimates, coerciveness and 173 (see also Part II) A priori estimates, for linear elliptic equations 234 257ff A priori estimates, for ordinary differential equations 79 794 A priori estimates, for parabolic equations 252 A priori estimates, for quasi-linear elliptic equations 246 A priori estimates, Fredholm operators, regularization and 263 A priori estimates, Galerkin method and see Part II A priori estimates, Gronwall's lemma and 83 A priori estimates, Leray - Schauder principle and 245 A priori estimates, linear operators and 230 A priori estimates, Ljapunov function and 84 Abstract Hammerstein equations 307ff Admissible homotopy 569 Admissible map 569 Algebraic geometry and bifurcation theory 352 442 639 690 Algebraic topology 726ff; see Part V Analytic major ant method 363 Analytic operator 362 Analytic set 646 Analytic space 646 ANE (absolute neighborhood extensor) 598 ANR (absolute neighborhood retract) 598 608 Antipodal theorem 700 Antipodal theorem with general symmetries 721 Antipodal theorem, basic ideas of the 694 Applications to celestial mechanics 65 126 222 736 Applications to differential inclusions 468 Applications to eigenvalue problems see Eigenvalue Applications to formal languages 510 Applications to foundations of set theory 489 511 Applications to functional differential equations 733 Applications to game theory 461 469 Applications to Haar measure 67 466 Applications to heat conduction 301 Applications to Hopf bifurcation 411 438 Applications to implicit functions 155 195 Applications to integral equations 10 21 36 57 58 60 156 289 316 387 633 634 670 788 790 Applications to integral inequalities 82 281 Applications to interval arithmetic 508 513 Applications to linear and nonlinear oscillations 91ff 101ff 201 401ff Applications to linear elliptic partial differential equations 233 Applications to mathematical biology 162 733 Applications to mathematical economics 255 469 Applications to mathematical logic (G 749 Applications to nonlinear boundary-value problems 326 Applications to nonlinear harmonic oscillator 59 Applications to ordinary differential equations see Ordinary Applications to periodic solutions see Periodic Applications to Prandtl's boundary-layer equation 341 Applications to quasi-linear elliptic partial differential equations 246 250 251 Applications to quasi-periodic solutions 104 221 Applications to semi-linear elliptic partial differential equations 60 239 317 344 347 390 634 671 Applications to semi-linear hyperbolic partial differential equations 60 64 Applications to semi-linear parabolic partial differential equations 60 329 Applications to systems of real equations 10 22 35 40 52 155 177 195 209 256 257 430ff 657 Applications to total differential equations 166ff Applications to variational inequalities 453 Applications to von Neumann algebras 486 Approximation as a proof strategy 55 56 187 188 497 500 525 537 542 Approximation methods (overview) 8 (see also Section 37.29 in Part III) Approximation of compact operators 55 Approximation of continuous functions (Weierstrass theorem) 770 Approximation of continuous operators by smooth generic operators 533 Approximation of nonsmooth functions 549 Asymptotic linear operators 296 603 706 Asymptotically stable equilibrium point 87 Asymptotically stable fixed point 158 Asymptotically stable solution of an elliptic equation 323 Asymptotically stable, weakly bifurcation solution 424 Autocombustion 314 346 Axiom of Choice 747 Axiom of choice, equivalent forms of the 512 Axiom of choice, independence of the 748ff Axiomatic systems, consistency 750 Axiomatic systems, independence 750 Axiomatic systems, undecidability 750 Axiomatic systems, unsolvability 750 Baire category 802 Baire category, and iterative methods 33 Baire space 801 Banach manifold 180; see Parts IV and V Banach manifold, one-dimensional 254 Banach space (B-space) 752 768 Banach space (B-space), 769 Banach space (B-space), basic concepts 768ff Banach space (B-space), bounded linear operator 773 Banach space (B-space), bounded set 769 Banach space (B-space), Cauchy sequence 769 Banach space (B-space), closed ball see List of symbols Banach space (B-space), closed set 769 Banach space (B-space), compact operator 53 Banach space (B-space), compact set 769 Banach space (B-space), completion 111 Banach space (B-space), complexification 770 Banach space (B-space), continuous linear functional 774 Banach space (B-space), continuous linear operator 764 773 Banach space (B-space), continuous operator 770 Banach space (B-space), convergence 769 775 Banach space (B-space), convergence principles 480 776 Banach space (B-space), dense set 769 Banach space (B-space), direct sum 765 Banach space (B-space), dual operator 775 Banach space (B-space), dual space 774 Banach space (B-space), duality 774 Banach space (B-space), equivalent norms 772 Banach space (B-space), examples 771ff Banach space (B-space), factor space 765 770 Banach space (B-space), geometry of A-spaces see Part III Banach space (B-space), graph closed operator ( 778 Banach space (B-space), important theorems 776ff 782 Banach space (B-space), infinite series 773 Banach space (B-space), norm 768 Banach space (B-space), norm convergence see Convergence Banach space (B-space), norm isomorphism 771 Banach space (B-space), open ball see List of Symbols Banach space (B-space), open set 769 Banach space (B-space), operator norm 773 Banach space (B-space), product space 755 770 Banach space (B-space), projection operator 766 Banach space (B-space), reflexive 11 Banach space (B-space), relatively compact set 769 Banach space (B-space), separable 770 Banach space (B-space), separation theorem see Part III Banach space (B-space), spectral theory 794 Banach space (B-space), splitting property 766 Banach space (B-space), strictly convex 486 Banach space (B-space), strong convergence see Convergence Banach space (B-space), strong 781 Banach space (B-space), topological direct sum 766 Banach space (B-space), triangle inequality 769 Banach space (B-space), uniformly convex 474 Banach space (B-space), weak (weak 775 Banach space (B-space), weak (weak 781 Basic ideas of approximation methods 8 19 Basic ideas of bifurcation theory 108 350ff 653 663 Basic ideas of differential calculus 131 Basic ideas of fixed-point index and mapping degree 519ff Basic ideas of fixed-point theory 9ff 61 Basic ideas of functional analysis, linear 768ff 787ff Basic ideas of functional analysis, nonlinear 2ff Basic ideas of implicit function theorem 150 Basic ideas of modern mathematical physics 100ff 656 726ff; Basic ideas of noncompact operators 489 Basic ideas of positivity 269ff Basic ideas of problems, quasi-linear 246 Basic ideas of problems, semi-linear 60 239 398 Basic ideas of stability 87 91 99 117 157 282 423 663 Basic ideas of the implicit function theorem and its applications 151 Basic ideas of the relation between classical analysis and nonlinear functional analysis 6 Basis of a linear space 765 Basis of a topology 751 Basis of neighborhoods 754 Bieberbach conjecture 257 Bifurcation at infinity 315 673 Bifurcation diagram 386 419 Bifurcation Feigenbaum 738ff Bifurcation generic 381 391 416 428 Bifurcation global 667ff Bifurcation Hopf 411 438 655 663 Bifurcation local 381 391 416 428 665 Bifurcation point 428 Bifurcation preventing secondary 681 Bifurcation stability and 423 655 Bifurcation subcritical 386 Bifurcation supercritical 386 Bifurcation theory, approach to, analytical 350ff Bifurcation theory, approach to, topological 653ff Bifurcation theory, approach to, variational see Chapter 45 in Part III Bifurcation theory, basic ideas of 350ff 654ff Bifurcation theory, basic technique of see Branching equations Bifurcation theory, basic topological principle (index jump) 657 Bifurcation theory, blowing-up of singularities 353 357 397 440 Bifurcation theory, implicit function theorem and 397 418 Bifurcation theory, rescaling technique 419 Bifurcation transcritical 386 Bifurcation transversality and 382 big bang 113 728; Biorthogonal system 786 Black holes in cosmos 113 730; Blowing-up of singularities 353 357 397 440 Blowing-up of solutions of differential equations 113 Boundary 751 Boundary point 751 Bounded operator 757 Bounded operator, linear 773 Bounded set in B-spaces 769 Bounded set in locally convex spaces 780 Bounded set in topological vector spaces 768 Branching equations) 657 Branching equations, basic ideas of 352 Branching equations, method of Ljapunov - Schmidt 375 Branching equations, solution methods for 352 378 418ff 430ff 440ff 689 Brouwer fixed-point theorem 51 Brouwer fixed-point theorem, constructive proof 254 Brouwer fixed-point theorem, elementary proof via the Knaster- Kuratowski - Mazurkiewicz lemma see Part IV Brouwer fixed-point theorem, numerous propositions which are equivalent to the see Chapter 77 in Part IV Brouwer fixed-point theorem, overview of other proofs 68 Brouwer fixed-point theorem, proof via the fixed-point index 530 Bundle see Parts IV and V Bundle fiber 729 Bundle tangent 183 Bundle vector 728 Cantor continuum hypothesis 749 Cantor discontinuum 583 594 Cantor discovery of set theory 591ff Cantor set 583 758 cardinal number 749 Cauchy sequence 762 Cauchy sequencein B-spaces 769 Cauchy sequencein locally convex spaces 780 Cauchy sequencein uniform spaces 801 Chain in ordered sets 503 Chain in ordered sets, 636 Chain rule 138 Chain rule for higher derivatives 192 Characteristic class 728; see Part V Characteristic number 269 Chemical reactions 119 Classification of differential equations 804 classification of integral equations 804 Classification of spaces in functional analysis 752 753 Closed set 751 754 Closed set characterization by M - S-sequences 759 760 Closed set characterization by sequences 759 760 Closed set in B-spaces 769 Cobordism theory 526 722; Codimension 765 Coercive 173 Coercive weakly 173 Cohomology 517 728; Coincidence degree 580 Collection (class) 746 Collection (class), nonset 746 Collection (class), set 746 Common fixed point of an operator family 66 486 Common zero of polynomials and resultants 434 Compact operator 53 Compact operator, 604 Compact operator, characterization by sequences 769 770 Compact perturbation of identity 173 Compact set 756 Compact set, characterization by finite 762 Compact set, characterization by M - S-sequences 759 760 Compact set, characterization by sequences 759 760 Compact set, in B-spaces 769 Compactness theorem of Arzela - Ascoli 772 Complementing condition 258 262 Complete locally convex space 780 Complete metric space 762 Complete orthonormal system 786 787 Complete uniform space 801 Component 757 Cone 276 Cone order 276 Continuation method 227 241 243 253 Continuation method, geometric heart of the 661 Continuation method, Leray - Schauder principle 245 556 628 631 658 Continuation method, of Poincar 203 Continuous operator 755 Continuous operator, basic properties 755 Continuous operator, characterization by M - S-sequences 759 760 Continuous operator, characterization by sequences 759 760 Continuous operator, in B-spaces 770 Continuous operator, in locally convex spaces 780 Continuum 564 Continuum Hypothesis 749 Continuum hypothesis, independence of the 749 Continuum of fixed points 564 Continuum of solutions 629 631 668 Convergence 801 Convergence in B-spaces 769 Convergence in B-spaces, norm convergence (identical with convergence) convergence in B-spaces, strong convergence (identical with convergence) convergence in B-spaces, weak 775 Convergence in B-spaces, weak 775 Convergence in metric spaces 761 Convergence in topological spaces 758 Convergence of iterative methods see Rate of convergence Convergence of M - S-sequences 760 Convergence of sequences 759 Convex functional 765 Convex hull 764 Convex operator 298 Convex set 764 Critical points of functionals 642 (see also Part III) Critical points of functionals, existence of three 644 Critical points of functionals, regular 642 Cusp catastrophe 437 Cycles 736 739 Cycles and fixed points of iterated maps 739 Dense set 751 Dense set characterization by sequences 769
Реклама
 |
|
О проекте
|
|
О проекте



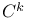 -diffeomorphism
-diffeomorphism  -compact (image compact)
-compact (image compact)  - weakly stable
- weakly stable  del's incompleteness theorem)
del's incompleteness theorem)  -neighborhood
-neighborhood 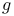 -closed)
-closed)  topology
topology  for periodic solutions
for periodic solutions