Авторизация
Поиск по указателям
Zeidler E. — Nonlinear Functional Analysis and Its Applications: Part 1: Fixed-Point Theorems
Обсудите книгу на научном форуме Нашли опечатку?
Название: Nonlinear Functional Analysis and Its Applications: Part 1: Fixed-Point TheoremsАвтор: Zeidler E. Аннотация: This is the first of a five-volume exposition of the main principles of nonlinear functional analysis and
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1986Количество страниц: 897Добавлена в каталог: 22.12.2011Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Neumann series 33 795 Newton diagram in bifurcation theory 432 Newton's method 206 Newton's method, accelerated (quadratic) convergence and 25 Newton's method, eigenvalue problems and 215 Newton's method, global 256 Newton's method, hard implicit function theorem and 218 Newton's method, in interval mathematics 513 Newton's method, quasi - Newton's method 207 Newton's method, quasilinearlization and 217 Newton's method, simplified 207 214 Newton's method, Smale's complexity theorem 257 Noncompactness measure, basic ideas 489ff Noncompactness measure, basic properties 493 Noncompactness measure, general 611 Noncompactness measure, Hausdorff 611 Noncompactness measure, Kuratowski 492 Noncompactness measure, semi-additive 611 Nonset 746 Normal form, basic ideas 105 Normal form, Birkhoff - Sternberg - Moser 736 Normal form, catastrophe theory and see Part IV Normal form, for diffeomorphisms 171 Normal form, for immersions and submersions see Part IV Normal form, for subimmersions (rank theorem) 195 Normal form, Jordan 373 Normal form, of branching equations 431 Normal form, typical difficulties 105 Normal order cone 277 Normal structure of a set 485 Nullhomotopic 715 Open mapping theorem, linear 111 Open mapping theorem, nonlinear 705 Open sets in B-spaces 769 Open sets in locally convex spaces 780 Open sets in metric spaces 761 Open sets in topological spaces 751 Operator, 171 Operator, 147 Operator, 172 Operator, 755 Operator, 278 Operator, 778 Operator, 141 Operator, 17 Operator, 496 Operator, 611 Operator, adjoint 786 Operator, affin 764 Operator, analytic 362 Operator, asymptotic linear 296 Operator, bijective 748 Operator, bilinear 141 Operator, bounded 757 Operator, closed 757 Operator, coercive 173 Operator, compact 53 Operator, compact perturbation of identity 173 Operator, concave 298 Operator, condensing 496 Operator, continuous 755 770 Operator, contractive 17 Operator, convex (functional) 765 Operator, convex (operator) 298 Operator, demiclosed 476 Operator, demicompact 479 Operator, diffeomorphism 171 Operator, differentiable 76 135 Operator, dual 775 Operator, embedding 233 Operator, equicontinuous (operator family) 772 783 Operator, essential 679 Operator, F-differentiable 135 Operator, fixed-point free 9 Operator, Fredholm 365 Operator, G-differentiable 135 Operator, H 231 Operator, homeomorphism 755 Operator, immersion 152 Operator, inessential 679 Operator, injective 748 Operator, linear 764 Operator, linear bounded 773 Operator, Lipschitz continuous 17 Operator, locally H 232 Operator, locally Lipschitz continuous 191 Operator, lower semi-continuous (functional) 456 760 Operator, lower semi-continuous (multivalued mapping) 450 Operator, monotone 646 (see also Part II) Operator, monotone increasing 278 Operator, multilinear 141 Operator, multivalued 447 Operator, nonexpansive 17 Operator, of analytic type 645 Operator, of monotone type 298 341 Operator, open 757 Operator, perturbation of identity 173 Operator, positive 278 Operator, potential 642 (see also Part III) Operator, power 360 Operator, projection 766 Operator, proper 173 Operator, pseudodifferential 264 Operator, quasi-bounded 706 Operator, quasi-concave 456 Operator, quasi-convex 456 Operator, quasi-linear 246 804 Operator, Schauder 55 Operator, self-adjoint 786 Operator, semi-linear 398 804 Operator, stable 706 Operator, strictly monotone 603 Operator, strictly monotone increasing 278 Operator, strongly continuous 498 Operator, strongly monotone 706 Operator, strongly monotone increasing 278 Operator, strongly positive 278 Operator, subimmersion 178 Operator, sublinear 299 Operator, submersion 183 Operator, superlinear 299 Operator, surjective 748 Operator, symplectic 734 Operator, upper semi-continuous (functional) 456 Operator, upper semi-continuous (multivalued mapping) 450 Operator, weakly coercive 173 Operator, with closed range 499 111 Optimal mixed strategy pair in game theory 462 Optimal strategy pair 461 Orbit 93 Orbital stable 162 Order cone 276 Order cone, generating 276 Order cone, normal 277 Order cone, total 276 Order interval 276 Ordered B-space 276 Ordered set 503 Ordinal number 591 Ordinary differential equations, basic ideas 73ff 100 Ordinary differential equations, bifurcation 401ff Ordinary differential equations, blowing-up 113 Ordinary differential equations, boundary-eigenvalue problems 791 Ordinary differential equations, center 95 101 Ordinary differential equations, center, stable, and unstable manifolds 112; see Part IV Ordinary differential equations, continuous dependence on the initial-values and on the parameters 165 Ordinary differential equations, differential inclusions (multivalued equations) 468 Ordinary differential equations, fixed-points and 10 27 57 78 81 502 Ordinary differential equations, global solutions via a priori estimates 79 Ordinary differential equations, Grobman - Hartman theorem on structural stability 99ff Ordinary differential equations, Gronwall's lemma 82 122 Ordinary differential equations, Hopf bifurcation 411 438 655 663 Ordinary differential equations, infinite systems 124 Ordinary differential equations, integral equations and 10 27 57 78 81 502 Ordinary differential equations, invariant sets 112 Ordinary differential equations, Kneser - Fukuhara theorem on generalized uniqueness 567 Ordinary differential equations, limit cycles 98 119 408 Ordinary differential equations, Ljapunov center theorem 655 Ordinary differential equations, Ljapunov stability theorem 87 99 Ordinary differential equations, main theorem 165 Ordinary differential equations, maximum principles 334 335 792 Ordinary differential equations, method of Green's function 59 791 Ordinary differential equations, monotonicity and uniqueness 122 Ordinary differential equations, Nagumo theorem on invariant sets 122 Ordinary differential equations, nonlinear boundary-value problems 215ff Ordinary differential equations, normal forms 105 Ordinary differential equations, on convex sets 123 Ordinary differential equations, on manifolds and flows see Part IV Ordinary differential equations, Peano theorem 57 81 Ordinary differential equations, periodic solutions see Periodic Ordinary differential equations, Picard - Lindel 27 33 Ordinary differential equations, plane vector fields 91ff Ordinary differential equations, Poincar 120 Ordinary differential equations, preventing secondary bifurcation 681 Ordinary differential equations, quasilinearization 217 Ordinary differential equations, quasiperiodic solutions 104 221 Ordinary differential equations, resonance 101 404 406 Ordinary differential equations, shooting method 215ff Ordinary differential equations, small divisors 103 220 Ordinary differential equations, stability 87 91ff Ordinary differential equations, uniqueness 27 33 122 Orthonormal system 786 oscillations see Applications to Parametrix method 264 Partition of unity 756 Periodic solutions, basic difficulties 101 Periodic solutions, bifurcation and 408 411 438 655 663 Periodic solutions, celestial mechanics and 126 736 Periodic solutions, eigenvalue problems and 410 411 438 Periodic solutions, existence of 65 88 408 411 438 482 726 736 Periodic solutions, Feigenbaum bifurcation (period doubling) 738 Periodic solutions, fixed points of the Poincar 86 Periodic solutions, functional differential equations and 733 Periodic solutions, Hopf bifurcation 411 438 655 663 Periodic solutions, limit cycles 98 119 408 Periodic solutions, mathematical biology and 733 Periodic solutions, nonrepelling fixed points and 200 733 Periodic solutions, symplectic transformations and 736 perspectives 99 600 726 Perturbation of identity 175 Perturbation of identity, 175 Perturbation of identity, compact 175 Phase transitions 115 743; Plane vector fields 91ff Poincar 86 Point, accumulation (limit) 754 Point, bifurcation 358 Point, boundary 751 Point, cluster 759 760 Point, equilibrium 86 Point, exterior 751 Point, fixed 9 Point, interior 751 Point, isolated 754 Point, regular (identical with noncritical, nondegenerate) 184 Point, saddle 457 Point, singular (identical with critical, degenerate) 184 Polyhedron 599 Polyhedron, topological 599 Population evolution 119 162 Prandtl's boundary-layer equation 341 Preimage 748 Preimage, theorem 185 Pricing system 469 Product, of B-spaces 770 Product, of topological spaces 755 Product, rule 138 Product, set 747 748 Projection operators 766 Projection operators, construction of 369 Proof ideas, approximation 56 478 497 501 537 542 Proof ideas, compactness 56 456 458 542 Proof ideas, convexity 456 458 Proof ideas, demiclosedness 479 Proof ideas, generalized solution operator and proof ideas, regularization 240 Proof ideas, iteration 17 Proof ideas, localization (partition of unity) 455 Proof ideas, noncompactness measure 500 Proof ideas, projection 379 Proof ideas, regularization and fast iteration 219 Proof ideas, retraction (extension) 51 563 Proof strategies, bifurcation theory 376 Proof strategies, fixed-point index or mapping degree 520 525 530 Proof strategies, genericity 108 188 253ff 525 Proof strategies, regular semi-linear equations 60 398 Pseudoresolvent 377 Quantum statistics 486 743; Quarks 67 109 729; Quasi - Newton methods 207 218 Quasi-linear 804 Quasi-linear, elliptic partial differential equations 246 251 Quasiperiodic motions 104 221 412 Rank of a linear operator 767 Rank theorem 178 195 Rate of convergence 20 31 Rate of convergence, acceleration of the 25 Rate of convergence, asymptotic 31 Rate of convergence, average 31 Rate of convergence, definition for general iterative methods 217 Rate of convergence, examples 26 42 43 Rate of convergence, linear 20 Rate of convergence, quadratic 26 Redundant symbols in calculus 204 Refinement of a covering 594 Reflexive B-space 774 Reflexive fixed point 184 Reflexive locally convex spaces 783 Reflexive point 184 Reflexive semi-linear operator equation 398 Reflexive topological space 596 Reflexive value 184 Reflexive zero 184 Region 758 Regula falsi in B-spaces 218 Regular critical point 440 Regularization, abstract methods of 263 Regularization,and fast iteration (hard implicit function theorem) 218 Regularization,of Fredholm operators 369 Regularization,of generalized solutions 240 249 259 Relatively closed 755 Relatively compact sets 756 Relatively compact sets in B-spaces 769 Relatively compact sets, characterization by finite 762 Relatively compact sets, characterization by finite M - S-sequences 759 760 Relatively compact sets, characterization by finite sequences 759 760 Relatively M - S-sequentially compact 759 Relatively open 755 Relatively sequentially compact 759 Resolvent 795 Resolvent set 795 Resonance, basic ideas of 101 Resonance, duality between resonance and nonresonance 354 402ff Restricted 126 204 222 Resultant 434ff Resultant system 434 retract 50 Retract, absolute neighborhood retract (ANR) 598 608 Retract, euclidean neighborhood retract (ENR) 609 Retract, neighborhood retract 598 Retraction 50
Реклама
-diffeomorphism 
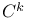-diffeomorphism (embedding diffeomorphism)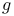 
-closed (graph closed) 
- linear 
-condensing 
lder continuous 
- Bendixson theorem on limit cycles 
-nets 
|
|
О проекте