Авторизация
Поиск по указателям
Grünbaum B. — Convex Polytopes
Обсудите книгу на научном форуме Нашли опечатку?
Название: Convex PolytopesАвтор: Grünbaum B. Аннотация: The appearance of Gruenbaum's book Convex Polytopes in 1967 was a moment of grace to geometers and combinatorialists. The special spirit of the book is very much alive even in those chapters where the book's immense influence made them quickly obsolete. Some other chapters promise beautiful unexplored land for future research. The appearance of the new edition is going to be another moment of grace. Kaibel, Klee and Ziegler were able to update the convex polytope saga in a clear, accurate, lively, and inspired way. —Gil Kalai, The Hebrew University of Jerusalem The original book of Gruenbaum has provided the central reference for work in this active area of mathematics for the past 35 years...I first consulted this book as a graduate student in 1967; yet, even today, I am surprised again and again by what I find there. It is an amazingly complete reference for work on this subject up to that time and continues to be a major influence on research to this day. —Louis J. Billera, Cornell University The original edition of Convex Polytopes inspired a whole generation of grateful workers in polytope theory. Without it, it is doubtful whether many of the subsequent advances in the subject would have been made. The many seeds it sowed have since grown into healthy trees, with vigorous branches and luxuriant foliage. It is good to see it in print once again. —Peter McMullen, University College LondonThe combinatorial study of convex polytopes is today an extremely active and healthy area of mathematical research, and the number and depth of its relationships to other parts of mathematics have grown astonishingly since Convex Polytopes was first published in 1966. The new edition contains the full text of the original and the addition of notes at the end of each chapter. The notes are intended to bridge the thirty five years of intensive research on polytopes that were to a large extent initiated, guided, motivated and fuelled by the first edition of Convex Polytopes. The new material provides a direct guide to more than 400 papers and books that have appeared since 1967. Branko Grünbaum is Professor of Mathematics at the University of Washington.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Издание: Second EditionГод издания: 2003Количество страниц: 466Добавлена в каталог: 30.06.2008Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Hirsch conjecture for special polytopes 355a Hirsch conjecture, 354 Hirsch conjecture, monotone 355b Hirsch conjecture, strong monotone 355b Homeomorphism, C-homeomorphism 210 Homogeneous coordinates 7a Homogeneous function 13 Homogeneous metric space 415 Homogenization see “Projective transformation” Homothetic 1 Homothetic, positively 1 318 Hyperbolic space 7a Hyperplane 2 Hyperplane arrangement see “Arrangement” Hyperplane arrangement, complex 410b Hyperplane arrangement, number of regions 410b Hyperplane arrangement, number of simplicial regions 410b Hyperplane arrangement, simplicial 410b Hyperplane arrangement, zonotopes 410a Hyperplane arrangements, real, and oriented matroids 410a Hyperplane at infinity 4 Hyperplane, Euler see “Euler hyperplane” Hyperplane, separating 10 Hyperplane, supporting 10 Hypersimplex 69a icosahedron 215 Illuminating set 422 Illumination 423c Improper face 17 incidence 143 Incidence equation 144 169 Incidence graph 216 Incidence system 171 Incident objects 143 Indecomposable lens 240 245 Indecomposable polytope 318 329 Indecomposable polytope, examples 323 Index of a 2 arrangement 394 Inner diagonal 423c Inradius 423c Inscribable type 285 Integer programming 69a Interior of a set 6 Intersection property 142b Intersectional basis 13 Intersectional family 3 13 Irredundant set of halfspaces 26 Isometry 252 Isomorphism of complexes 199 Isomorphism of Gale-diagrams 89 Isomorphism of Gale-transforms 89 Isomorphism of partially ordered sets 231 Isomorphism of polytopes see “Combinatorial equivalence” Isomorphism problem 52b Isoperimetric problem 292 417 Javaview xii Jordan’s Theorem 142 231 k-content 423b Keller’s problem 411 423a Klee — Minty cube 389b Kleetope 217 Kleetope, dimensional unambiguous graphs 227 Kleetope, f-vectors of centrally symmetric polytopes 169 Kleetope, Hamiltonian circuits 357 Kleetope, path-lengths 372 Kleetope, spanning trees 296 Knapsack polytope 121b Kneser problem 129b Kruemmungsschwerpunkt see “Steiner point” Kruskal’s theorem 179 LADDER 369 Lattice 21 Lattice polytope 52a Lattice tiling 423a Lattice, deciding polytopality 296d Lattice, Eulerian see “Eulerian lattice” Lattice, face 50 Lattices, semilattices and abstract cell complexes 206 Lawrence construction 96a Lens 239 245 Lens, indecomposable 240 LIMIT 5 Line 3 Line arrangement 410a Line arrangement, number of triangles 410b Line arrangement, projective plane 428c Line shelling 142a Line-free set 24 linear combination 2 Linear dependence 2 Linear hull 3 Linear hyperplane arrangement 69b Linear program see “Simplex algorithm” Linear program, random 389b Linear program, standard forms 96a Linear programming 356 Linear programming, computer experience 381 Linear programming, duality 96a Linear programming, paths on polytopes 342 347 Linear programming, simplex algorithm 356 Linear programming, standard form 381 Linear systems 337 Linked complex 40 Locally similar polytopes 52c Lower bound conjecture 183 (see also “Lower bound theorem”) Lower bound theorem for polytopes 198a Lower bound theorem for simplicial manifolds 198a Lower bound theorem, g-theorem 198b Lower semicontinuity of 83 Lower semicontinuity of 393 Lower semicontinuity of 416 lrs 52b m-sequence 198a Main theorem about polytopes 52a Manifold see “Eulerian manifold” Markov chain 340b Matching polytope 69a McMullen’s conditions 198a Mean Width 315 Mean width of a compact convex set 427 Measure polytope 56 Menger’s theorem 224b (see also “Whitney’s theorem”) Merging of polytopes 373 Metric function 5 Metric space 5 Metric space, complete 6 Metric space, homogeneous 415 Minkowski sum 340a Minkowski’s theorem 332 339 Misanthrope problem 296d Mixed volume 324 Mixed volume, hardness of computing 340b Mixed volume, randomized algorithm for computing 340c Moebius function 142b Moment curve 61 Moment curve, trigonometric 69b Monotone Hirsch conjecture 355b Morse function 142a Multi-k-gon 272 Multi-k-valent 282 Multiplicity 86 109 Nash equilibrium 423c Neighborly family of centrally symmetric polytopes 129b Neighborly family of polytopes 128 Neighborly family of simplices 129b Neighborly polytope 123 129a—129b Neighborly polytope, centrally symmetric 116 Neighborly polytope, diameter 345 Neighborly polytope, f-vectors 162 174 Neighborly polytope, k-almost 126 Neighborly polytope, k-neighborly polytope 122 Neighborly polytope, simplicial 124 Neighborly polytopes, number of see “Number” Neighborly set 126 Non-rational 96a Non-revisiting path conjecture 355a (see also “ Nonbasic gradient 378 Nonbasic variable 378 Normal fan of a polytope 52c Normally equivalent polytopes 52c Number of 2-arrangements 394 Number of 3-polytopes (asymptotically) 296c Number of 3-polytopes with n edges 290 Number of d-polytopes with d+3 vertices 114 121a Number of neighborly 4-poly topes 129a Number of neighborly 6-polytopes 129a Number of neighborly d-polytopes 129a 428c Number of neighborly d-polytopes with d+3 vertices 121a Number of neighborly simplicial d-polytopes with d+3 vertices 121a 428c Number of simplicial 3-polytopes with p vertices 290 Number of simplicial d-polytopes with d+3 vertices 111 121a Number of simplicial polytopes and spheres 121a Objective function 347 Octahedron, 1-skeleton 216 Octahedron, d-octahedron 55 Octahedron, projection 72 Octahedron, regular polytopes 412 Open set 5 Optimization, combinatorial see “Combinatorial optimization” Optimization, convex 30b Optimization, non-linear 30b Oracle 52b Oracle, computation of volume 340b Order dimension 296d Organic compounds 356 362 Orientation of a polytope graph 355b Orientation of a polytope graph, induced by a linear function 296b Oriented matroid 30b Oriented matroid, arrangements 410a Oriented matroid, compatible 96b Oriented matroid, cyclic see “Cyclic oriented matroid” Oriented matroid, duality and Gale-diagrams 96a Oriented matroid, equilibrated systems of vectors 340c Oriented matroid, realizable 410a Orthant 305 Orthogonal vectors 2 Outward normal 13 Packing 411 Parallelotope 56 335 Path code 382 Path in a graph 212 Path on a polyhedron 341 Path, 354 Path, 375 Path, Hamiltonian 356 Path, length 366 Path, simple 356 Path, simplex 376 Path, steep 376 Path, strict 375 Path-length 366 Perles, M.A., angle-sums relations of simplicial polytopes 307 Perles, M.A., automorphisms and symmetries of d-polytopes with d+3 vertices 120 Perles, M.A., conjecture on facet subgraphs of simple polytopes 234a Perles, M.A., d+3 points in general position 120 Perles, M.A., d-polytopes with d+3 vertices 121a Perles, M.A., dimension of f-vectors of quasi-simplicial polytopes 153 Perles, M.A., Gale-diagrams 96a Perles, M.A., Gale-transformation 85 Perles, M.A., neighborly families of simplices 129b Perles, M.A., non-rational 8-polytope 94 Perles, M.A., number of d-polytopes with d+3 vertices 114 Perles, M.A., number of simplicial d-polytopes with d+3 vertices 111 Perles, M.A., polytopes with few vertices 108 Perles, M.A., projectively unique d-poly topes with d+3 vertices 120 Perles, M.A., rational realizations of d-polytopes with d+3 vertices 119 Perles, M.A., reconstruction of polytopes from (d-2)-skeleta 234a Perles, M.A., reconstruction of simplicial polytopes from [d/2]-skeleta 234a Perles, M.A., results on intersections of simplices and flats 74 Perles, M.A., solution of a problem of Klee’s 426 Permissible projective transformation 4 Perturbation 96b Petrie arc 258 294 Piecewise affine mapping 41 Piecewise affine refinement map 205 Piecewise linear function 316 326 Piecewise projective mapping 41 Planar graph, 2-diagrams 244 Planar graph, 3-polytopes 235 Plane of symmetry 246 Platonic solids 296d 412 Point, exposed see “Exposed point” Point, extreme see “Extreme point” Pointed cone 24 Polar cone 49 Polar set 47 Polyhedral at a point 36 Polyhedral cell-complex 52c Polyhedral combinatorics 69a Polyhedral complex 51 Polyhedral computation 52b Polyhedral set 26 Polyhedral set, bounded 32 Polyhedral set, face numbers 139 Polyhedral set, quasi-polyhedral set 36 Polyhedral sets, complex of 41 Polyhedron, class (d,n) 347 Polyhedron, diameter 347 Polyhedron, number of vertices 188 197 Polyhedron, paths 341 Polyhedron, simplex-algorithm 378 Polymake xii 52b Polynomial inequalities 52b Polytope 31 Polytope algebra 340a Polytope with d+3 vertices 121a Polytope with d+4 vertices 96a Polytope without triangle faces 198d Polytope, 0/1-polytope 69a Polytope, antipodal 420 Polytope, approximation 326 Polytope, centrally symmetric see “Centrally symmetric polytope” Polytope, coding size of coordinates 296d Polytope, congruent-faced 414 Polytope, cubical see “Cubical polytope” Polytope, cyclic see “Cyclic polytope” Polytope, decomposable see “Decomposable polytope” Polytope, dual 46 Polytope, equifacetted 423a Polytope, generalized regular 423a Polytope, height 389b Polytope, hyperbolic 7a Polytope, indecomposable see “Indecomposable polytope” Polytope, infinite-dimensional 52 Polytope, inscribed in a sphere 296c Polytope, neighborly see “Neighborly polytope” Polytope, prescribing shapes of faces 296d Polytope, projectively unique 68 Polytope, pyramidal see “Pyramidal polytope” Polytope, quasi-simplicial see “Quasi-simplicial polytope” Polytope, quotient 129b Polytope, random 129b Polytope, rational see “Rational polytope” Polytope, reconstruction of the combinatorial structure see “Reconstruction” Polytope, regular see “Regular polytope” Polytope, regular-faced 414 Polytope, rigidity 129b Polytope, rooted, oriented 3-polytope 289 Polytope, self-dual 52d Polytope, semiregular 413 423a
Реклама
 |
|
О проекте
|
|
О проекте



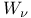 paths
paths 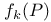

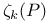
 -path
-path