Heath T.L. (ed.) — Thirteen Books of Euclid's Elements, Vol. 1 :: Электронная библиотека попечительского совета мехмата МГУ

Главная Ex Libris Книги Журналы Статьи Серии Каталог Wanted Загрузка ХудЛит Справка Поиск по индексам Поиск Форум

Авторизация

Поиск по указателям

Heath T.L. (ed.) — Thirteen Books of Euclid's Elements, Vol. 1

Обсудите книгу на научном форуме

Нашли опечатку?
Выделите ее мышкой и нажмите Ctrl+Enter

Название: Thirteen Books of Euclid's Elements, Vol. 1

Автор: Heath T.L. (ed.)

Аннотация:

Volume 1 of three-volume set containing complete English text of all 13 books of the Elements plus critical apparatus analyzing each definition, postulate and proposition in great detail. Covers textual and linguistic matters; mathematical analyses of Euclid's ideas; classical, medieval, Renaissance and modern commentators; refutations, supports, extrapolations, reinterpretations and historical notes. Total in set: 995 figures.

Язык:

Рубрика: Математика/

Статус предметного указателя: Готов указатель с номерами страниц

ed2k: ed2k stats

Год издания: 1908

Количество страниц: 424

Добавлена в каталог: 02.04.2008

Операции: Положить на полку | Скопировать ссылку для форума | Скопировать ID

Предметный указатель

Plane (or plane surface), Plane Loci of Apollonius      14 259 330
Plane (or plane surface), Plato's definition of      171
Plane (or plane surface), possible origin of Euclid's def.      171
Plane (or plane surface), Proclus' and Simplicius' interpretation of Euclid's def.      171
Planudes, Maximus      72
Plato      1 2 3 137 155—156 159 184 187 203 221
Plato, "rational diameter of      5" 399
Plato, def. of plane surface      171
Plato, def. of straight line      165—166
Plato, generation of cosmic figures by putting together triangles      226
Plato, rule for rational right-angled triangles      356 357 359 360 385
Plato, supposed invention of Analysis by      134
Playfair, "Playfair's" Axiom      220
Playfair, and Eucl. Post. 5      313
Playfair, comparison of Axiom with Post. 5      313—314
Playfair, John      103 111
Playfair, used to prove I.      29 312
Pliny      20 333
Plutarch      21 29 37 177 343 351
Point, an-Nairizi on      157
Point, attributes of, according to Aristotle      156
Point, interpretation of Euclid's definition      155
Point, is it sufficient?      156
Point, modern explanations by abstraction      157
Point, motion of, produces line      157
Point, negative character of Euclid's def.      156
Point, other definitions by "Herundes," Posidonius      156
Point, Plato's view of, and Aristotle's criticism      155—156
Point, Pythagorean definition of      155
Point, Simplicius      157
Point, terms for $(\sigma\tau i\gamma\mu\nu ,\;\sigma\nu\mu\epsilon io\nu)$       156
Polybius      331
Polygon, sum of exterior angles      322
Polygon, sum of interior angles (Proclus' proof)      322
Porism, (1) = corollary      134 278—279
Porism, (2) as used in Porisms of Euclid, distinguished from theorems and problems      10 11
Porism, account of the Porisms given by Pappus      10—13
Porism, and Zeuthen      15
Porism, interpolated Porisms (corollaries)      60—61 381
Porism, modern restorations by Simson and Chasles      14
Porism, two senses      13
Porism, views of Heiberg      11 14
Porphyry      17
Porphyry, commentary on Euclid      24
Porphyry, Symmikta      24 34 44 136 277 283 287
Posidonius, book directed against the Epicurean Zeno      34 43
Posidonius, definition offigure      41 183
Posidonius, on parallels      40 190
Posidonius, the Stoic      20 21 27 28n. 189 197
Postulate 4, converse true only when angles rectilineal (Pappus)      201
Postulate 4, proofs, of, resting, on, other      195—196 231
Postulate 4, significance of      200
Postulate 5      202—203
Postulate 5, attempts, to, prove      202—203
Postulate 5, Carnot, Laplace, Lorenz, W. Bolyai, Gauss, Worpitzky, Clairaut, Veronese, Ingrami      220
Postulate 5, due to Euclid himself      202
Postulate 5, I.      30
Postulate 5, is logical equivalent of      220
Postulate 5, Legendre      213 214 220
Postulate 5, Nasiraddin      202—203
Postulate 5, others by Proclus      207 220
Postulate 5, Posidonius and Geminus      220
Postulate 5, Post., 5, proved, from, and, compared, with, ", Playfair's"      202—203
Postulate 5, Proclus, on      202—203
Postulate 5, substitutes for, "Playfair's" axiom (in Proclus)      220
Postulate 5, Wallis      220
Postulate, distinguished from axiom, by Aristotle      118—119
Postulate, distinguished from axiom, by Proclus (Geminus and "others")      121—123
Postulate, distinguished from hypothesis, by Aristotle      120—121
Postulate, distinguished from hypothesis, by Proclus      121—122
Postulate, Euclid's view of, reconcileable with Aristotle's      119—120 124
Postulate, famous "Postulate of Archimedes"      234
Postulate, Postulates      1 2
Postulate, postulates do not confine us to rule and compass      124
Postulate, postulates in Archimedes      120 123
Postulate, significance of      195—196
Potts, Robert      112 246
Prime (of numbers), two senses of      146
Principles, First      117—124
Problem, (2) deficient problem $(\epsilon\lambda\lambda i\pi\epsilon s\;\pi\rho o\beta\lambda\eta\mu\alpha),$ giving too little      129
Problem, another classification (1) problem in excess $(\pi\lambda\epsilon o\nu\alpha\zeta o\nu)$ , asking too much      129
Problem, in widest sense anything propounded (possible or not) but generally a construction which is possible      128—129
Problem, Problem, distinguished from theorem      124—128
Problem, problems classified according to number of solutions (a), one solution, ordered $(\tau\epsilon\tau\alpha\gamma\mu\epsilon\nu\alpha)$ (b), a definite number, intermediate $(\mu\epsilon\nu\alpha)$ (c), an infinite number of solutions, unordered $(\alpha\tau\alProblem, pha\kappa\tau\alpha)$       128
Proclus, attempt to prove Postulate      5 206—208
Proclus, books quoted by name in      34
Proclus, character of MS. used by      62 63
Proclus, commentary on Eucl. I, sources of      29—45
Proclus, commentary on Plato's Republic, allusion in to " side-" and "diagonal-" numbers in connexion with Eucl. II.      9 10 399—400
Proclus, commentary probably not continued, though continuation intended      32—33
Proclus, details of career      29—30
Proclus, famous "summary"      37—38
Proclus, his own contributions      44—45
Proclus, list of writers quoted      44
Proclus, object and character of      31—32
Proclus, on advantages of Euclid's Elements, and their object      115—116
Proclus, on difficulties in three distinctions between postulates and axioms      123
Proclus, on first principles, hypotheses, postulates, axioms      121—124
Proclus, on the nature of elements and things elementary      114—116
Proclus, on theorems and problems      124—129
Proclus, remarks on earlier commentators      19 33 45
Proof $(\alpha\pi o\delta\epsilon i\xi is)$ , necessary part of proposition      129—130
Proposition, formal divisions of      129—131
Protarchus      5
Psellus, Michael, scholia by      70 71
Pseudaria of Euclid      7
Pseudaria of Euclid, Pseudographemata      7n.
Pseudoboethius      92
Ptolemy I.      1 2
Ptolemy I. story of Euclid and Ptolemy      1
Ptolemy, attempt to prove it      204—206
Ptolemy, Claudius      30n.
Ptolemy, Harmonica of, and commentary on      17
Ptolemy, on Parallel-Postulate      28n. 34 43 45
Pythagoras      4n. 36
Pythagoras, and proof of      352—355
Pythagoras, by Zeuthen      355—356
Pythagoras, probable method of discovery of I.      47
Pythagoras, rule for forming right-angled triangles in rational numbers      351 356—359 385
Pythagoras, story of sacrifice      37 343 350
Pythagoras, suggestions by Bretschneider and Hankel      354
Pythagoras, supposed discoverer of application of areas      343—344
Pythagoras, supposed discoverer of the irrational      351
Pythagoras, supposed discoverer of theorem of I.      47 343—344 350—354
Pythagoreans      19 36 155 188 279
Pythagoreans, "rational" and "irrational diameter of      5" 399—400
Pythagoreans, angles of triangle equal to two right angles, theorem and proof      317—320
Pythagoreans, gnomon Pythagorean      351
Pythagoreans, method of application of areas (including exceeding and falling-short)      343 384 403
Pythagoreans, term for surface $(\chi\rho oi\alpha)$       169
Pythagoreans, three polygons which in contact fill space round point      318
Q.E.D. (or F.)      57
Qadizade ar-Rumi      5n. 90
Quadratic equation, geometrical solution of      383—385 386—388
Quadratic equation, solution assumed by Hippocrates      386—387
Quadratrix      265—266 330
Quadrature $(\tau\epsilon\tau\rho\alpha\gamma\omega\nu i\sigma\mu os),$ definitions of      149
Quadrilaterals, varieties of      188—190
Quintilian      333
Qusta b. Liiqa al-Ba'labakki, translator of "Books XIV, XV"      76 87 88
Radius, no Greek word for      199
Ramus, Petrus (Pierre de la Ramee)      104
Ratdoit, Erhard      78 97
Rational $(\rho\eta\tau os),$ "rational diameter of      5" 399—400
Rational $(\rho\eta\tau os),$ (of ratios)      137
Rational $(\rho\eta\tau os),$ rational rightangled triangles      see “Right-angled triangles”
Rauchfuss      see “Dasypodius”
Rausenberger, O.      157 175 313
Rectangle = rectangular parallelogram      370
Rectangle, "rectangle contained by"      370

Rectilineal angle "rectilineal segment"      196
Rectilineal angle definitions classified      179—181
Rectilineal angle rectilineal figure      187
Reductio ad absurdum      134
Reductio ad absurdum, a variety of Analysis      140
Reductio ad absurdum, by exhaustion      285 293
Reductio ad absurdum, described by Aristotle and Proclus      136
Reductio ad absurdum, nominal avoidance of      369
Reductio ad absurdum, synonyms for, in Aristotle      136
Reduction $(\alpha\pi\alpha\gamma\omega\gamma\eta),$ first " reduction " of a difficult construction due to Hippocrates      135
Reduction $(\alpha\pi\alpha\gamma\omega\gamma\eta),$ technical term, explained by Aristotle and Proclus      135
Regiomontanus (Johannes Mueller of Koenigsberg)      93 96 100
Reyher, Samuel      107
Rhaeticus      101
Rhomboid      189
Rhombus, meaning and derivation      189
Riccardi, P.      96 112 202
Riemann, B.      219 273 274 280
Right angle, construction when drawn at extremity of second line (Heron)      270
Right angle, definition      181
Right angle, drawing straight line at right angles to another, Apollonius' construction for      270
Right-angled triangles, connexion of rules with Eucl. II.      4 8 360
Right-angled triangles, discovery of rules by means of gnomons      358—360
Right-angled triangles, rational right-angled triangles in Apastamba      361 363
Right-angled triangles, rational rule for finding, by Plato      356 357 359 360 385
Right-angled triangles, rational rule for finding, by Pythagoras      356—359
Roeth      357—358
Rouche and de Comberousse      313
Rudd, Capt. Thos.      110
Ruellius, Joan. (Jean Ruel)      100
Russell, Bertrand      227 249
Sa'id b. Masud b. al-Qass      90
Saccheri, Gerolamo      106 144—151 167—168 185—186 194 197—198 200—201
Sathapatha — Brahmana      362
Savile, Henry      105 166 245 250 262
Scalene $(\sigma\kappa\alpha\lambda\eta\nu os\;or\;\sigma\kappa\alpha\lambda\eta\nu\eta s)$       187—188
Scalene $(\sigma\kappa\alpha\lambda\eta\nu os\;or\;\sigma\kappa\alpha\lambda\eta\nu\eta s)$ of cone (Apollonius)      188
Scalene $(\sigma\kappa\alpha\lambda\eta\nu os\;or\;\sigma\kappa\alpha\lambda\eta\nu\eta s)$ of numbers ( = odd)      188
Schessler, Chr.      107
Scheubel, Joan.      101 107
Schiaparelli, G.V.      163
Schliissel, Christoph      see “Clavius”
Schmidt, Max C.P.      304 319
Schmidt, W., editor of Heron, on Heron's date      20—21
Scholia to Elements and MSS. of      64—74
Scholia to Elements and MSS. of "Schol. Vat. " partly derived from Pappus' commentary      66
Scholia to Elements and MSS. of "Schol. Vind. "      69—70
Scholia to Elements and MSS. of classes of, " Schol. Vat. "      65—69
Scholia to Elements and MSS. of evidence in, as to text      64—65 66—67
Scholia to Elements and MSS. of historical information in      64
Scholia to Elements and MSS. of Joannes Pediasimus      72—73
Scholia to Elements and MSS. of many scholia partly extracted from Proclus on Bk. I.      66 69 72
Scholia to Elements and MSS. of miscellaneous      71—74
Scholia to Elements and MSS. of numerical illustrations in, in Greek and Arabic numerals      71
Scholia to Elements and MSS. of scholia by Maximus Planudes      72
Scholia to Elements and MSS. of scholia by Psellus      70—71
Scholia to Elements and MSS. of scholia in Latin published by G. Valla, Commandinus, Conrad Dasypodius      73
Scholia to Elements and MSS. of scholia on Eucl. II.      13 407
Scholia to Elements and MSS. of sometimes interpolated in text      67
Schooten, Franz van      108
Schopenhauer      227 354
Schotten, H.      167 174 179 192—193 202
Schumacher      321
Schur, F.      328
Schweikart, F.K.      219
Scipio Vegius      99
Sectio Canonis by Euclid      17
Section $(\tau o\mu\nu),$ = point of section      170 171 383
Segment of circle, angle of      253
Segment of circle, segment less than semicircle called 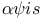$\alpha\psi is$       187
Semicircle      186
Semicircle, angle of      182 253
Semicircle, centre of      186
Seqt      304
Serenus of Antinoeia      203
Serle, George      110
Setting-out $(\epsilon\kappa\theta\epsilon\sigma is),$ may be omitted      130
Setting-out $(\epsilon\kappa\theta\epsilon\sigma is),$ one of formal divisions of a proposition      129
Sextus Empiricus      62 63 184
Shamsaddin as — Samarqandi      5n. 89
Sigboto      94
Simon, Max      108 155 157—158 167 202 328
Simplicius      22 167 171 184 185 197 203 223 224
Simplicius commentary on Euclid      27—28
Simplicius on Eudemus' style      35 38
Simplicius on lunes of Hippocrates      29 35 386—387
Simplicius on parallels      190—191
Simson, definition of plane      172—173 185 186 255 259 287 293 296 322 328 384 387 403
Simson, on "vitiations" in Elements due to Theon      46 103 104 106 111 148
Simson, Robert on Euclid's Porisms      14
Sind b. 'AH Abu 't-Taiyib      86
Smith and Bryant      404
Speusippus      125
Sphaerica, early treatise on      17
Spiral of Archimedes      26 267
Spiral, "single-turn"      122—123 164—165
Spiral, in Pappus = cylindrical helix      165
Spire (tore) or Spiric surface      163 170;
Spire (tore) or Spiric surface, varieties of      163
Spiric curves or sections, discovered by Perseus      161 162—164
St Vincent, Gregory of      401 404
Steenstra, Pybo      109
Steiner, Jakob      193
Steinmetz, Moritz      101
Steinschneider, M.      8n. 76
Stephanus Gracilis      101—102
Stephen Clericus      47
Stobaeus      3
Stoic "axioms"      41 221
Stoic "axioms", illustrations $(\delta\epsilon i\gamma\mu\alpha\tau\alpha)$       329
Stolz, O.      328
Stone, E.      105
Straight line, Archimedes' assumption respecting      166
Straight line, by Legendre      169
Straight line, by Leibniz      169
Straight line, cannot have a common segment      196—199
Straight line, division -of straight line into any number of equal parts (an-Nairizi)      326
Straight line, Euclid's definition, interpreted by Proclus and Simplicius      166—167
Straight line, in Heron      168
Straight line, language and conjecture as to origin      168
Straight line, language and construction of      167
Straight line, one or two cannot make a figure      169 183
Straight line, other definitions      168—169
Straight line, pre-Euclidean (Platonic) definition      165—166
Straight line, two straight lines cannot enclose a space      195—196
Stroemer, Marten      113
Studemund, W.      92n.
Subtend, meaning and construction      249 283 350
Suidas      370
Sulatman b. 'Usma (or Oqba)      85 90
Superposition, apparently assumed by Aristotle as legitimate      226
Superposition, Bertrand Russell on      227 249
Superposition, Euclid's dislike of method of      225 249
Superposition, no use theoretically, but merely furnishes practical test of equality      227
Superposition, objected to by Peletarius      249
Superposition, used by Archimedes      225
Surface, $\epsilon\pi i\phi\alpha\nu\epsilon i\alpha$ in Euclid $(not\;\epsilon\pi i\pi\epsilon\delta o\nu)$       169
Surface, alternative definition of, in Aristotle      170
Surface, classifications of surfaces by Heron and Geminus      170
Surface, composite, incomposite, simple, mixed      170
Surface, divisions or sections of solids are surfaces      170 171
Surface, homoeomeric (uniform)      170
Surface, loci on surfaces      329 330
Surface, plane surface      see “Plane”
Surface, produced by motion of line      170
Surface, Pythagorean term for, $\chi\rho oi\alpha$ ( = colour, or skin)      169
Surface, spheroids      170
Surface, spiric surfaces      163 170
Surface, terms for, in Plato and Aristotle      169
Surface-loci of Euclid      15 16 330

1 2 3 4 5

О проекте