јвторизаци€
ѕоиск по указател€м
Tenenbaum M., Pollard H. Ч Ordinary differential equations: an elementary textbook for students of mathematics, engineering, and the sciences
ќбсудите книгу на научном форуме Ќашли опечатку?
Ќазвание: Ordinary differential equations: an elementary textbook for students of mathematics, engineering, and the sciencesјвторы: Tenenbaum M., Pollard H. јннотаци€: This book has been written primarily for you, the student. We have tried to make it easy to read and easy to follow.
язык: –убрика: ћатематика /—татус предметного указател€: √отов указатель с номерами страниц ed2k: ed2k stats √од издани€: 1985 оличество страниц: 819ƒобавлена в каталог: 05.11.2010ќперации: ѕоложить на полку |
—копировать ссылку дл€ форума | —копировать ID
ѕредметный указатель
Principal value of 199 Principle of superposition 211 254 Problem involving a centrifugal force 380 Problems, first order equations 107Ч195 377-7 377-10 Problems, first order equations, accretion 122Ч124 Problems, first order equations, atmospheric pressure 186Ч188 Problems, first order equations, body falling in water 144 Problems, first order equations, chain around a cylinder 188Ч189 Problems, first order equations, decomposition 131Ч132 Problems, first order equations, dilution 122Ч124 Problems, first order equations, electric circuit 184Ч185 377-7 377-10 Problems, first order equations, first order processes 137 Problems, first order equations, flow of heat, steady state 185Ч186 Problems, first order equations, flow through an orifice 183Ч184 Problems, first order equations, geometric 107Ч111 Problems, first order equations, growth 131Ч132 Problems, first order equations, horizontal motion 160Ч162 Problems, first order equations, inclined motion 164Ч166 Problems, first order equations, interest 126Ч127 Problems, first order equations, isogonal trajectory 115Ч117 Problems, first order equations, moon 151-6 151-7 151-8 156-38 156-39 Problems, first order equations, motion of a complex system 189Ч191 Problems, first order equations, oceanic pressure 186 187 Problems, first order equations, orthogonal trajectories 117Ч120 Problems, first order equations, pursuit curves 168Ч175 Problems, first order equations, raindrop 143 Problems, first order equations, relative pursuit curves 177Ч182 Problems, first order equations, rocket motion 191Ч193 Problems, first order equations, rope around a cylinder 188Ч189 Problems, first order equations, rotation of a liquid in a cylinder 193Ч194 Problems, first order equations, second order processes 134Ч137 Problems, first order equations, steady state flow of heat 185Ч186 Problems, first order equations, straight line motion 138Ч166 Problems, first order equations, temperature 129Ч130 Problems, first order equations, variable mass 191Ч193 Problems, first order equations, vertical motion 139Ч151 Problems, linear second order equations 313Ч389 Problems, linear second order equations, bending of beams 383Ч389 Problems, linear second order equations, circle, particle moving on circumference of 316Ч317 Problems, linear second order equations, damped harmonic motion 347Ч353 Problems, linear second order equations, damped harmonic motion, definition of 347 Problems, linear second order equations, damped motion, forced 359Ч364 Problems, linear second order equations, damped motion, free 347Ч353 Problems, linear second order equations, elastic helical spring 324Ч326 Problems, linear second order equations, elastic helical spring in parallel 330-13 Problems, linear second order equations, elastic helical spring in series 330-14 Problems, linear second order equations, electric circuit, simple 369Ч375 Problems, linear second order equations, forced motion with damping 359Ч364 Problems, linear second order equations, forced undamped motion 338Ч342 Problems, linear second order equations, free damped motion 347Ч353 Problems, linear second order equations, free undamped motion 313Ч329 see Problems, linear second order equations, particle moving on a straight line 314Ч316 Problems, linear second order equations, particle moving on circumference of a circle 316Ч317 Problems, linear second order equations, pendulum, simple see "Pendulum" Problems, linear second order equations, problem involving a centrifugal force 380 Problems, linear second order equations, rolling bodies 381Ч383 Problems, linear second order equations, simple electric circuit 369Ч375 Problems, linear second order equations, simple harmonic motion 313Ч329 see Problems, linear second order equations, straight line, particle moving on 314Ч316 Problems, linear second order equations, twisting bodies 383 Problems, linear second order equations, undamped motion 313Ч342 Problems, linear second order equations, undamped motion, examples of 323Ч329 Problems, linear second order equations, undamped motion, forced 338Ч342 Problems, linear second order equations, undamped motion, free 313Ч321 Problems, special types of second order nonlinear equations 506Ч530 Problems, special types of second order nonlinear equations, central force 521Ч522 Problems, special types of second order nonlinear equations, geometric 528Ч530 Problems, special types of second order nonlinear equations, pursuit 523Ч525 Problems, special types of second order nonlinear equations, special central force 521Ч522 Problems, special types of second order nonlinear equations, suspension cable 507Ч514 Problems, system of equations 440Ч492 Problems, system of equations, biological 447Ч451 Problems, system of equations, central force see "Particle in motion in space subject to a central force" Problems, system of equations, electrical 451Ч455 Problems, system of equations, mechanical 440Ч443 Problems, system of equations, particle in motion in space see "Particle in motion in space subject to a central force" Problems, system of equations, plane motion of a particle see "Particle in motion in space subject to a central force" Problems, system of equations, plane motion of a projectile 463Ч465 Problems, system of equations, planetary motion 491Ч492 Problems, system of equations, projectile in plane 463Ч465 Product of two operators 257 Projectile, motion of a 463Ч465 Proof by mathematical induction 250 Properties of Bessel function of first kind 619Ч622 Properties of Laguerre polynomials 627Ч630 Properties of Laplace transforms 295Ч296 Properties of Legendre polynomials 598Ч605 Properties of polynomial operators see "Polynomial operators" pure imaginary number 197 Pursuit curves 168Ч175 523Ч525 Pursuit curves, relative 177Ч182 Radioactive material 2 Radius of curvature 528 Raindrop problem 143 Range of a function 9 11 Ratio test for convergence 532 Real number system 197 Real part of a complex number 197 Rectangular form of a complex number 199 Recursion formula 577 Recursion formula for Bessel equation 610 Recursion formula for Hermite polynomial 607-18 Recursion formula for Laguerre equation 625 Recursion formula for Legendre polynomial 601 Reduction of order method 241Ч246 Region, bounded 14 Region, F-2 of atmosphere 155 Region, simply connected 71 Regular singularity 571 Regular singularity at 586 Relative pursuit curve 177Ч182 Remainder function 670 Resistance, coefficient of 348 Resistance, electric 370 resistors 369 Resistors in series 454-2 Resonance 373 443 Resonance, undamped 341 Resonant frequency, undamped 341 Response of a system, impulsive 344 Riccati equation 97 247 Riemann integral 70 Riemann-integrable 70 731 Rocket motion 191Ч193 Rodrigue's formula 602 Rolling bodies 381Ч383 Roots in conjugate pairs 204-9 Roots of indicial equation 574Ч584 Roots, imaginary 217Ч220 Roots, real and distinct 213Ч214 Roots, real but some multiple 214Ч217 Rope around a cylinder 188Ч189 Rotating tube, particle in a 380 Rotation of a liquid 193Ч194 Rotational inertia 381 Rounding off errors 636 690 Runge Ч Kutta formulas for second order equation 709 Runge Ч Kutta formulas for system of two first order equations 702Ч703 Runge Ч Kutta formulas for y'=f(x,y) 653Ч658 Runge Ч Kutta formulas, comment on error in 657Ч658 Satellites 488Ч491 496 Second order equations 500Ч504 707Ч711 712-1 712-3 Second order equations, numerical solution of 707Ч711 Second order equations, numerical solution of, by Adams' method 712-3 Second order equations, numerical solution of, by Milne's method 710Ч711 Second order equations, numerical solution of, by Runge Ч Kutta method 709 Second order equations, numerical solution of, error in 712-1 Second order equations, special types of nonlinear 500Ч504 Second order processes, problems 134Ч137 Separable variables 52 Separated variables 52 Sequence of functions, convergence of a 728 732 Sequence of functions, uniform convergence of a 729 732 Series for 201 Series for cos x and cos z 201 Series for sin x and sin z 201 Series for tan x 742 Series of functions 732Ч733 Series of functions, converges uniformly 732 733 Series, binomial 599 Series, convergence of 531 532 Series, defines a function 533 Series, determination of coefficients of a 534 Series, differentiation of a 534 Series, equality of 534 Series, expansion of inverse operators by 272Ч277 Series, Frobenius 572 Series, Frobenius, solution of homogeneous linear equation by 572Ч584 Series, Frobenius, solution of nonhomogeneous linear equation 585-18 Series, hypergeometers 587 Series, interval of convergence of 532 Series, Maclaurin 535 Series, partial sums of 732 Series, power 531 Series, ratio test for convergence of 532 Series, solutions by see "Solution by series methods" Series, Taylor 535 Series, Taylor, with remainder 537 Set, elements of a 6 Set, meaning of a 5Ч6 Simple beam 384 Simple electric circuit 369Ч375 simple harmonic motion 313Ч329 Simple harmonic motion, amplitude 317 Simple harmonic motion, definition of 314 Simple harmonic motion, description of the motion 315 317 Simple harmonic motion, elastic helical spring 324Ч326 Simple harmonic motion, equilibrium position 317 Simple harmonic motion, examples of bodies moving in 323Ч329 Simple harmonic motion, frequency, natural (undamped) 318 319 Simple harmonic motion, harmonic oscillators 323Ч329 Simple harmonic motion, natural (undamped) frequency 318 319 Simple harmonic motion, particle moving on a straight line 314Ч316 Simple harmonic motion, particle moving on circumference of a circle 316Ч317 Simple harmonic motion, pendulum 327Ч329 Simple harmonic motion, period 318 Simple harmonic motion, phase and phase angle 318 Simple harmonic motion, simple pendulum 327Ч329 Simple harmonic motion, spring, helical 324Ч326 Simply, connected region 71 Simply, supported beam 384 Simpson's rule 675 678 679 684 Simultaneous equations see "Entries under System" Sine x, sin z, expansions of 201 Sine, hyperbolic 203 Singular point of y'=f(x,y) 43 744Ч747 Singular solution 34 Singularity of a linear equation 570 Singularity of a linear equation, irregular 572 Singularity of a linear equation, regular 571 Singularity of a linear equation, regular at 586 Sixth degree interpolating polynomial 677 Sliding friction 160 Slope field 39 Slowly varying amplitude 345 Solution about a regular singularity 572Ч584 585 586 Solution about a regular singularity at 586 Solution about a regular singularity of a homogeneous linear equation, where roots of indicial equation, differ by an integer 578Ч582 Solution about a regular singularity of a homogeneous linear equation, where roots of indicial equation, do not differ by an integer 574Ч578 Solution about a regular singularity of a homogeneous linear equation, where roots of indicial equation, equal 583Ч584 Solution about a regular singularity of a nonhomogeneous linear equation 585-18 Solution by series methods 537Ч584 Solution by series methods by Frobenius series see "Solution about a regular singularity" Solution by series methods by Taylor series of a linear equation 537Ч546 Solution by series methods by Taylor series of a nonlinear equation of order n 562Ч567 Solution by series methods by Taylor series of a system of first order equations 555Ч559 Solution by series methods by Taylor series of a system of linear first order equations 559Ч562 Solution by series methods by Taylor series of y'=f(x,y) 548Ч553 Solution of a system of equations 394Ч420 Solution of a system of equations by numerical methods 702Ч706 Solution of a system of equations by Picard's method 723Ч726 726-9 Solution of a system of equations by series method 555Ч562 see Solution of a system of equations of a first order linear system 396Ч397 Solution of a system of equations of a first order system 394Ч396 Solution of a system of equations of a linear system with constant coefficients 398Ч420 Solution of a system of equations of a linear system with constant coefficients by Laplace transforms 418Ч420 Solution of a system of equations of a linear system with constant coefficients by polynomial operators 398Ч417 Solutions of differential equations by integrating factors 82Ч90 94 Solutions of differential equations by inverse operators 272Ч282 288Ч291 Solutions of differential equations by Laplace transforms 296Ч302 Solutions of differential equations by lineal element diagram 39 Solutions of differential equations by method of Frobenius 572Ч584 Solutions of differential equations by numerical methods 631Ч718 Solutions of differential equations by operators 262Ч265 272Ч282 288Ч291 Solutions of differential equations by partial fraction expansion of inverse operators 288Ч291 Solutions of differential equations by Picard's method of successive approximations 720Ч726 Solutions of differential equations by polynomial operators 262Ч265 Solutions of differential equations by power series 537Ч553 562Ч567 Solutions of differential equations by reduction of order 242Ч246 Solutions of differential equations by separation of variables 51Ч55 Solutions of differential equations by series 537Ч553 562Ч567 Solutions of differential equations by substitution and other means 101 Solutions of differential equations by successive approximations 720Ч726 Solutions of differential equations by undetermined coefficients 221Ч230 Solutions of differential equations by variation of parameters 233Ч240 Solutions of differential equations, by choice of method 99 Solutions of differential equations, by complex variables 230Ч231 Solutions of differential equations, definition of 22 Solutions of differential equations, existence of see "Existence and uniqueness theorems" Solutions of differential equations, explicit 21Ч23 Solutions of differential equations, general 28Ч37 Solutions of differential equations, general, definition of 35 Solutions of differential equations, implicit 24Ч27 Solutions of differential equations, linear combination of 208 Solutions of differential equations, multiplicity of 28Ч31 Solutions of differential equations, n-parameter family of 30 31Ч33 Solutions of differential equations, number of independent 208 786 Solutions of differential equations, particular 35 Solutions of differential equations, singular 34 Solutions of differential equations, steady state 361 372 Solutions of differential equations, transient 361 372 Solutions of differential equations, uniqueness of see "Existence and uniqueness theorems" Solutions of differential equations, Wronskian of 781-Comment 64.15 Solve a differential equation, meaning of 22 81 Special central force problem 521Ч522 Special types of second order equations 500Ч504 Special types of second order equations, x absent 503Ч504 Special types of second order equations, x and y' absent 500Ч501 Special types of second order equations, y absent 502Ч503 Specific, gravity 153 Specific, heat 130 Specific, weight 512 Speed of escape 148 Speed, terminal 142 Spring constant 324 Spring, helical see "Elastic helical springs" Sputnik 491-7 Stable motion 339 Starting formula 680 Starting methods 632 Starting methods, polygonal method see "Polygonal method" Starting methods, polygonal method, an improvement of 641Ч643 Starting methods, Runge Ч Kutta see "Runge Ч Kutta formulas" Starting methods, Taylor series 645Ч652 Static friction 160 Steady state, current 372 Steady state, flow of heat 185Ч186 Steady state, motion 361 Steady State, Solution 361 372 Stiffness coefficient of a spring 324
–еклама
 |
|
ќ проекте
|
|
ќ проекте





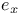 and
and