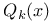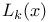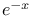Àâòîðèçàöèÿ
Ïîèñê ïî óêàçàòåëÿì
Tenenbaum M., Pollard H. — Ordinary differential equations: an elementary textbook for students of mathematics, engineering, and the sciences
Îáñóäèòå êíèãó íà íàó÷íîì ôîðóìå Íàøëè îïå÷àòêó?
Íàçâàíèå: Ordinary differential equations: an elementary textbook for students of mathematics, engineering, and the sciencesÀâòîðû: Tenenbaum M., Pollard H. Àííîòàöèÿ: This book has been written primarily for you, the student. We have tried to make it easy to read and easy to follow.
ßçûê: Ðóáðèêà: Ìàòåìàòèêà /Ñòàòóñ ïðåäìåòíîãî óêàçàòåëÿ: Ãîòîâ óêàçàòåëü ñ íîìåðàìè ñòðàíèö ed2k: ed2k stats Ãîä èçäàíèÿ: 1985Êîëè÷åñòâî ñòðàíèö: 819Äîáàâëåíà â êàòàëîã: 05.11.2010Îïåðàöèè: Ïîëîæèòü íà ïîëêó |
Ñêîïèðîâàòü ññûëêó äëÿ ôîðóìà | Ñêîïèðîâàòü ID
Ïðåäìåòíûé óêàçàòåëü
Energy, potential 329 473 Envelopes of a family of curves 747—754 Envelopes of a family of solutions 754—757 Envelopes of a family, definition of 748 Envelopes of a family, eliminant of 750 Equation, indicial 574 Equilibrium position 317 Equivalent triangular system 405 406 416 Error in an improvement of polygonal method 644-3 Error in fifth degree polynomial 679 Error in Milne method 688—689 Error in Newton's interpolation formulas 670—671 Error in polygonal method 636—638 Error in polynomial interpolation 670—671 679 Error in Runge — Kutta 657—658 Error in Simpson's rule 679—684 Error in Taylor series 537 649—652 653-4 Error in third degree polynomial 679 Error in trapezoidal rule 679 683 Error in Weddle's rule 679 Error, function 670 Errors, arithmetical 636 Errors, cumulative 636 690 Errors, formula 636 690 Errors, general comment on 636 690—691 703 Errors, rounding off 636 690 Escape velocity 148 Euler equation 247 Exact differential 72 Exact differential equation 70—78 248-25 Exact differential equation, definition of 73 248-25 Exact differential equation, necessary and sufficient condition for 73 248-25 Exact differential equation, recognizable 80—81 Exact differential equation, solution of 73 76 Existence and uniqueness theorems 720 Existence and uniqueness theorems, first order equation y'=f(x,y) 734—743 Existence and uniqueness theorems, linear equation of order n 783—786 Existence and uniqueness theorems, nonlinear equation of order n 765—767 Existence and uniqueness theorems, system of n first order equations 763—765 Existence and uniqueness theorems, system of n linear first order equations 768—770 Explicit solution 21—23 Explicit solution, definition of 22 Exponential complex function 201 Exponential shift theorem for inverse operators 277 Exponential shift theorem for polynomial operators 260 f(a), definition of 11 f(b,y), meaning of 18-10 f(x,a), definition of 13 F-2 region of atmosphere 155 Factorial function (n!) 306—308 596 Factors of polynomial operators 258 Falling bodies see "Vertical motion" Faltung theorem 303 Family, n-parameter of solutions 30 31—33 Family, n-parameter, envelopes of 754—756 Family, of curves, envelopes of 748—754 Field of force 473 Field, conservative 473 Field, direction 39 Field, slope 39 Fifth degree interpolating polynomial 676 678 679 Finite differences 659—661 First order equations with homogeneous coefficients 57—60 First order equations with linear coefficients 63—68 First order equations with separable variables 51—55 First order equations, Bernoulli 95—96 First order equations, Clairaut 757—760 First order equations, exact 70—78 First order equations, existence and uniqueness theorem for 734—743 First order equations, integrating factors of 84—90 94—95 First order equations, linear 91—95 First order equations, miscellaneous 101—103 First order equations, perturbation theory 713—715 First order equations, problems giving rise to see "Problems first First order equations, recognizable exact 80—82 First order equations, Riccati 97 247-22 247-23 247-24 First order equations, solution of y'=f(x,y) by numerical methods see "Numerical methods" First order equations, solution of y'=f(x,y) by Picard's method 720—723 First order equations, solution of y'=f(x,y) by series method 548—553 First order, processes 137 Flow through an orifice 183 Force of gravity 140 Force, central see "Particle in motion in space subject to a central force" Force, centrifugal 380 Force, centripetal 380 Force, damping 349 350 351 Force, electromotive 369 Force, field 473 Force, field of 473 Force, frictional 160 Force, function 473 Force, impressed (forcing function) 338 360 Force, induced electromotive 185 Force, intermittent 344 Force, inversely proportional to cube of distance 521—522 Force, inversely proportional to square of distance 481—488 494 Force, moment of 381 Force, proportional to distance 476—479 Forced motion with damping 359—364 Forced motion, undamped 338—342 Forcing function 338 Formula errors 636 679 Forward differences, ( 659—668 Fourth degree interpolating polynomial 681-2 Fractions of inverse operators 287 289 Fractions, partial 283—284 Free motion, damped 347—353 Free motion, undamped 313—329 see Frequency, damped 351 Frequency, impressed 339 Frequency, natural (undamped) 318 319 Frequency, normal 443 Frequency, resonance 373 443 Frequency, undamped resonant 341 Friction, coefficient of 160 Friction, sliding 160 Friction, static 160 Frictional force 160 Frobenius, method 572 see Frobenius, series 572 Function of one independent variable 6—11 14—17 48 Function of one independent variable, definition of 6 9 Function of one independent variable, definition of f(a) 11 Function of one independent variable, differential of a 48 Function of one independent variable, elementary 17 Function of one independent variable, implicit 14—17 Function of one independent variable, range of 9 Function of two independent variables 11—14 18 50 57—58 Function of two independent variables, definition of 11 Function of two independent variables, definition of f(b,y) 18-10 Function of two independent variables, definition of f(x,a) 13 Function of two independent variables, differential of a 50 Function of two independent variables, domain of definition of a 11 Function of two independent variables, homogeneous, of order n 57 58 Function of two independent variables, range of 11 Functions, analytic 537 Functions, analytic on an interval 537 Functions, beta 306 Functions, complementary 210 Functions, continuous 730 731 Functions, continuous at a point 730 Functions, continuous in a region 731 Functions, continuous on an interval 730 Functions, Dirac 344 Functions, elementary 17 Functions, elliptic 333 334 Functions, error 670 Functions, factorial (n!) 306—308 596 Functions, force 473 Functions, forcing 338 360 Functions, gamma 306—309 Functions, homogeneous, of order n 57 58 Functions, hypergeometric 587 Functions, Legendre 594 Functions, Legendre of second kind, 597 Functions, orthogonal 602 Functions, polynomial interpolating 662 Functions, remainder 670 Functions, sequence of 728 729 732 Functions, series of 732—733 Functions, unit impulse 344 Functions, vector point 473 Fundamental theorem of algebra 197 198 Fundamental theorem of calculus 70 Gamma function 306—309 Gamma function, definition of 307 Gauss's equation 587 General solution of a differential equation 28—37 General solution of a first order linear equation 93 General solution of a homogeneous linear equation 210 788 General solution of a nonhomogeneous linear equation 210 789 General solution, definition of a 35 Geometric problems leading to Clairaut equation 761 Geometric problems leading to first order equation 107—111 Geometric problems leading to special types of second order equations 528—530 Grammian 777 Graphical solutions 38—44 424—438 Gravitation, Newton's universal law of 491 Gravitational constant 139 491 Gravity, force of 140 Gravity, specific 153 Growth problems 131—132 Hailey's comet 492 Hanging cable 507—514 Harmonic motion, damped 348—353 Harmonic motion, undamped see "Simple harmonic motion" Harmonic oscillators 323—329 377 Harmonic oscillators, elastic helical spring 324—326 Harmonic oscillators, simple pendulum 327—329 Heat, specific 130 Heat, steady state flow of 185—186 Helical spring see "Elastic helical springs" Hermite, equation 607 Hermite, polynomials 607 Homogeneous function, order of a 57 58 Homogeneous in x, equation 505 Homogeneous linear equations see "Linear differential equations" Hooke's law 324 Horizontal motion 160—162 Hyperbolic, cosine 203 Hyperbolic, sine 203 Hyperbolic, tangent 203 Hypergeometric, equation 587 Hypergeometric, function 587 Hypergeometric, series 587 I (current) 369 370 i (imaginary unit) 197 Identically zero, meaning of 775 Imaginary number, part of a complex number 197 Imaginary number, pure 197 Impedance of a circuit 372 Implicit function 14—17 Implicit function, definition of 16 Implicit solution of an equation 24—27 Implicit solution of an equation, definition of 24 Impressed, force (forcing function) 338 Impressed, frequency 339 Improper integrals, convergence of 292 294 Improper integrals, divergence of 292 Improvement of polygonal method 641—643 Impulse function, unit 344 Impulsive response of a system 344 Inclined motion 164—166 Incomplete elliptic integral of the first kind 334 Independence of functions see "Linear independence of functions" Independent solutions of linear equation, number of 208 786 Indicial equation 574 Induced, current 185 Induced, electromotive force 185 Inductance, coefficient of 370 Inductance, mutual 454 Induction, mathematical 250 inductor 369 Inertia 381 Inertia, moment of 381 Inertia, rotational 381 Infinite series see "Series" Initial conditions 33—37 Initial conditions, definition of 36 Initial conditions, number of 36 Input of a system 341 360 372 Integrable combinations 81 Integral curve, definition of 39 Integral, convergence of 292 Integral, divergence of 292 Integral, elliptic of the first kind, complete 333 Integral, elliptic of the first kind, incomplete 334 Integral, improper 292 294 Integral, Riemann 70 Integrals, approximations to see "Numerical methods" Integrating factors 82—90 Integrating factors of first order equation 84—90 94—95 Integrating factors of second order linear equation 248 Integrating factors, definition of 82 Interest problems 126—127 Intermittent force 344 Interpolation see "Polynomial interpolation" interval 6 Interval of convergence 532 Inverse Laplace transform, definition of 296 Inverse Laplace transform, linear property of 296 Inverse operators 268—282 Inverse operators, definition of 269 270 Inverse operators, exponential shift theorem for 277 Inverse operators, meaning of 269—271 Inverse operators, partial fraction expansion of 287 Inverse operators, series expansion of 272—277 Inverse operators, solution of linear equation by 272—282 288—291 Inverse square law 481—488 491 494 Irregular singularity 572 Isoclines of a direction field 40—41 Isogonal trajectory 115—117 Isogonal trajectory, definition of 115 Isothermal surface 185 Kepler's laws 492 kinetic energy 329 475 Kirchhoff's, first law 451 Kirchhoff's, second law 370 Kutta see "Runge — Kutta formulas" Laguerre equation 624—630 Laguerre polynomials, 625—630 Laguerre polynomials, properties of 627—630 Laguerre polynomials, properties of, analogue of Rodrigue's formula 627—629 Laguerre polynomials, properties of, integral property 629—630 Laguerre polynomials, properties of, orthogonal with respect to weight function 630 Laplace transforms, construction of table of 302—306 309—311 Laplace transforms, definition of 294 Laplace transforms, Faltung theorem 303 Laplace transforms, inverse 296 Laplace transforms, properties of 295—296 Laplace transforms, solution of a linear equation by 296—302 Laplace transforms, solution of a system by 418—420 Laplace transforms, tables of 306 310 Law of conservation of angular momentum 472 Law of conservation of energy 329 475 Law of the mean 731 Law of the mean of universal gravitation 491 Legendre equation 586 591—605 606-13 Legendre equation, comment on solution of 593—594 Legendre equation, functions 594
Ðåêëàìà
, delta) 
|
|
Î ïðîåêòå