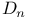|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| McConnell J.C., Robson J.C. — Noncommutative Noetherian Rings |
|
|
 |
| Предметный указатель |
Ring(s), context equivalent 3.6.4
Ring(s), Dedekind 5.2.10
Ring(s), derivation 15.1.4
Ring(s), extension 1.6.2
Ring(s), FBN 6.4.7
Ring(s), filtered 1.6.1
Ring(s), fixed 7.8.1
Ring(s), fully bounded 6.4.7
Ring(s), G----- 13.9.12 14.5.6
Ring(s), generic matrix 13.1.19
Ring(s), Goldie 2.3.1
Ring(s), graded 1.6.3
Ring(s), graded-division 10.3.19
Ring(s), graded-simple 10.3.19
Ring(s), group-graded 10.3.18
Ring(s), hereditary 5.2.2
Ring(s), ideal invariant 6.8.13
Ring(s), irreducible 6.8.17
Ring(s), Jacobson 9.1.2
Ring(s), localization of 2.1.4
Ring(s), locally nilpotent 13.8.5
Ring(s), Morita equivalent 3.5.5
Ring(s), Morita invariants of 3.5.10
Ring(s), Noetherian 0.1.6
Ring(s), of differential operators 15.0.0 ff 15.5.1
Ring(s), of Morita context 1.1.6
Ring(s), PI 13.0.0 ff 13.1.6
Ring(s), pri----- 0.1.8
Ring(s), prime 0.2.3
Ring(s), primitive 0.3.2
Ring(s), principal ideal 0.1.8
Ring(s), quotient 2.1.3 3.1.1
Ring(s), regular 7.7.1
Ring(s), semiprime 0.2.7
Ring(s), semiprimitive 0.3.9
Ring(s), semisimple 0.1.11
Ring(s), simple 0.1.10
Ring(s), skew Laurent polynomial 1.4.3
Ring(s), skew Laurent power series 1.4.4
Ring(s), skew polynomial 1.2.4
Ring(s), skew power series 1.4.1
Ring(s), trace 13.9.2 13.9.4
Ring(s), weakly ideal invariant 6.8.13
Saturated chain 13.10.13
Schanuel’s Lemma 7.1.2
Schanuel’s lemma, long 7.1.2
Schelter’s theorem 13.10.12
Schur’s lemma 0.1.9
Second layer, condition 4.3.12
Second layer, link 4.3.7
Semi-invariants 14.1.15
Semicentre 14.4.6
Semidirect product of groups 1.5.7
Semiprime ideal 0.2.7
Semiprime ring 0.2.7
Semiprimitive ideal 0.3.9
Semiprimitive polynomial ring 13.2.7
Semiprimitive ring 0.3.9
Semirnaximal 5.8.5
Semisimple action 14.1.12
Semisimple Lie algebra 14.1.12
Semisimple module 0.1.2
Semisimple ring 0.1.11
Semistable 6.9.8
Serre, conjecture 11.8.0
Serre, theorem 11.7.4 11.7.13
Simple module 0.1.2
Simple module, endomorphism ring of 9.0.0 ff
Simple ring 0.1.10
Simple ring,  being 1.8.6 being 1.8.6
Simple ring, 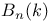 being 1.3.9 being 1.3.9
Simple ring,  of 12.7.1 ff of 12.7.1 ff
Simple ring, 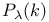 being 1.8.7 being 1.8.7
Simple ring, 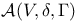 being 14.8.9 being 14.8.9
Simple ring, and enveloping algebras 14.3.6 14.3.7
Simple ring, being Dedekind domain 7.11.1 ff
Simple ring, being pri-ring 11.2.13
Simple ring, bounds on generators in 6.7.8
Simple ring, centre of 2.1.16
Simple ring, cyclic module over 5.7.3
Simple ring, derivation ring being 15.3.8
Simple ring, examples of 1.8.1 ff 1.9.8 7.8.14
Simple ring, GK dimension of 8.3.19
Simple ring, global dimension of 7.5.5
Simple ring, ideals in tensor product of 9.6.9
Simple ring, Krull dimension of 6.6.7 6.6.11 6.9.24 8.3.19
Simple ring, not being  domain 12.7.16 domain 12.7.16
Simple ring, not being matrix ring 12.7.7 ff
Simple ring, polynomials over 6.7.9
Simple ring, projective modules over 11.7.14
Simple ring, skew group ring being 7.8.12
Simple ring, Weyl algebra being 1.3.5
Singular ideal 2.2.4
Singular ideal,  - 10.3.5 - 10.3.5
Singular ideal, being nilpotent 2.3.4
Skew enveloping algebra see “Enveloping algebra”
Skew group ring see “Group ring”
Skew Laurent polynomial ring see “Skew polynomial ring”
Skew Laurent power series ring 1.4.4
Skew polynomial ring (Laurent) 1.2.1 ff 1.4.1
Skew polynomial ring (Laurent),  of 12.5.1 ff 12.6.1 of 12.5.1 ff 12.6.1
Skew polynomial ring (Laurent), and derivation ring 15.3.2
Skew polynomial ring (Laurent), being integral domain 1.2.9 1.4.5
Skew polynomial ring (Laurent), being Noetherian 1.2.9 1.4.5
Skew polynomial ring (Laurent), being non-Noetherian 1.2.11
Skew polynomial ring (Laurent), being pri-ring 11.2.13
Skew polynomial ring (Laurent), being prime 1.2.9 1.4.5
Skew polynomial ring (Laurent), being regular 7.7.5
Skew polynomial ring (Laurent), being simple 1.8.4 1.8.5
Skew polynomial ring (Laurent), GK dimension of 8.2.11 8.2.15 8.2.16
Skew polynomial ring (Laurent), global dimension of 7.5.3 7.9.1 7.10.1 9.1.14
Skew polynomial ring (Laurent), having projectives stably free 12.3.3
Skew polynomial ring (Laurent), in several variables 1.6.1 ff
Skew polynomial ring (Laurent), incomparability in 14.2.9
Skew polynomial ring (Laurent), Krull dimension of 6.5.4 6.6.6 6.6.10 6.9.1 9.1.14
Skew polynomial ring (Laurent), leading ideals of 1.2.9 1.8.5
Skew polynomial ring (Laurent), over commutative rings 6.9.1 ff
| Skew polynomial ring (Laurent), primes of 10.6.1 ff
Skew polynomial ring (Laurent), stably free ideals of 11.2.7 11.2.8
Skew polynomial ring (Laurent), universal property of 1.2.5
Skew power series ring 1.4.1
Skew power series ring, global dimension of 7.5.3
sl(2,k), dimensions of enveloping algebra of 8.5.12 ff
Small’s theorem 4.1.4
Smash product 1.9.7
Socle 6.2.14
Socle, Krull 6.2.14
Socle, Krull-sequence 6.2.14
Solvable Lie algebra 6.6.2 14.1.7
Somewhat commutative 8.6.9
Somewhat commutative, derivation ring being 15.1.21
sparse 4.6.11
Spectrum,  10.6.3 10.6.3
Spectrum, J 11.6.6
Spectrum, prime 0.2.3
Splitting field 13.3.6
Stability 11.0.0 ff
Stable g- 14.2.4
Stable G----- 10.5.4
Stable generation 6.7.1 ff 11.6.11
Stable ideal 1.8.2
Stable identity 13.1.16
Stable isomorphism 12.1.4
Stable matrix 11.5.16
Stable range of module 6.7.2
Stable rank 11.3.4
Stable unimodular row 11.3.2
Stably free 11.1.1 11.2.1
Stably free-graded 12.2.10
Stably isomorphic 12.1.4
Standard filtration 1.6.2 7.6.7 8.1.9
Standard matrix units 2.2.11
Standard monomials 1.7.5
State space 12.8.1
Strict 7.6.12
Strongly nilpotent element 0.2.5
Structure of semiprime Goldie rings 3.0.0 ff
Subisomorphic 3.3.4
Symmetric  -algebra 14.8.3 -algebra 14.8.3
Symmetric algebra 15.1.18
Syzygy theorem 12.3.1 ff
Topology, patch 4.6.14
Topology, Zariski 4.6.14
Tor 7.1.10
Torsion,  6.2.18 6.2.18
Torsion, free 3.4.2
Torsion, less 3.4.2
Torsion, module 2.1.17
Torsion, theory 2.4.1
Torsion, with respect to 4.3.2
Torsion, |G|-free 10.5.9
Trace, function 12.7.13
Trace, ideal 10.5.16
Trace, of group ring 7.8.6
Trace, of ring 12.7.13
Trace, proper 12.7.13
Trace, ring 13.9.2 13.9.4
Trace, universal function 12.7.13
Translate of filtration 8.6.14
Translate of grading 7.6.2
Triangular matrix ring 0.1.4
Triangular matrix ring, chain conditions in 1.1.4 1.1.9 1.1.10
Triangular matrix ring, global dimension of 7.5.1
Uniform, dimension 2.2.10
Uniform, module 2.2.5
Uniform, right ideals 3.3.2 ff
Unimodular, row/column 11.1.8
Unimodular, stable row/column 11.3.2
Universal enveloping algebra see “Enveloping algebra”
Unstable 6.9.8
Weak algorithm 11.3.9
Weak dimension 7.1.4
Weak global dimension 7.1.9
Weight(s), Jordan — Holder 14.4.10
Weight(s), space 14.1.19
Weyl algebra ( , ,  , ,  ) 1.3.1 ff ) 1.3.1 ff
Weyl algebra ( , ,  , ,  ), ),  1.8.6 1.8.6
Weyl algebra ( , ,  , ,  ), ),  1.3.9 1.3.9
Weyl algebra ( , ,  , ,  ), ), 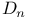 6.6.18 6.6.18
Weyl algebra ( , ,  , ,  ), ),  of 12.1.6 12.3.3 of 12.1.6 12.3.3
Weyl algebra ( , ,  , ,  ), ),  of overringof 12.3.3 12.6.14 12.7.7 of overringof 12.3.3 12.6.14 12.7.7
Weyl algebra ( , ,  , ,  ), and ), and 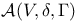 14.8.1 ff 14.8.1 ff
Weyl algebra ( , ,  , ,  ), and algebraic g 14.7.1 ff ), and algebraic g 14.7.1 ff
Weyl algebra ( , ,  , ,  ), and Nullstellensatz 9.1.8 9.4.22 ), and Nullstellensatz 9.1.8 9.4.22
Weyl algebra ( , ,  , ,  ), and solvable g 14.9.1 ff ), and solvable g 14.9.1 ff
Weyl algebra ( , ,  , ,  ), as derivation ring 15.1.5 ), as derivation ring 15.1.5
Weyl algebra ( , ,  , ,  ), as differential operators 1.3.6 ), as differential operators 1.3.6
Weyl algebra ( , ,  , ,  ), as factor of enveloping algebra 14.6.1 ff ), as factor of enveloping algebra 14.6.1 ff
Weyl algebra ( , ,  , ,  ), being almost commutative 8.4.3 ), being almost commutative 8.4.3
Weyl algebra ( , ,  , ,  ), being Dedekind 5.2.11 7.11.3 ), being Dedekind 5.2.11 7.11.3
Weyl algebra ( , ,  , ,  ), being maximal order 5.1.6 ), being maximal order 5.1.6
Weyl algebra ( , ,  , ,  ), being not a principal ideal ring 7.11.8 ), being not a principal ideal ring 7.11.8
Weyl algebra ( , ,  , ,  ), being simple Noetherian domain 1.3.5 1.3.8 1.8.6 ), being simple Noetherian domain 1.3.5 1.3.8 1.8.6
Weyl algebra ( , ,  , ,  ), embeddings of 6.6.19 ), embeddings of 6.6.19
Weyl algebra ( , ,  , ,  ), general linear rank of first 11.1.16 ), general linear rank of first 11.1.16
Weyl algebra ( , ,  , ,  ), generators of right ideals of 6.7.8 ), generators of right ideals of 6.7.8
Weyl algebra ( , ,  , ,  ), GK dimension of 8.1.15 8.2.7 ), GK dimension of 8.1.15 8.2.7
Weyl algebra ( , ,  , ,  ), GK dimension of modules over 8.5.3 ff ), GK dimension of modules over 8.5.3 ff
Weyl algebra ( , ,  , ,  ), global dimension of 7.5.8 ), global dimension of 7.5.8
Weyl algebra ( , ,  , ,  ), global dimension of overrings of 9.1.12 9.4.23 ), global dimension of overrings of 9.1.12 9.4.23
Weyl algebra ( , ,  , ,  ), has finite endomorphism rings 9.5.5 9.7.5 ), has finite endomorphism rings 9.5.5 9.7.5
Weyl algebra ( , ,  , ,  ), has projectives stably free 12.3.3 ), has projectives stably free 12.3.3
Weyl algebra ( , ,  , ,  ), has stably free ideals 11.1.4 11.2.11 ), has stably free ideals 11.1.4 11.2.11
Weyl algebra ( , ,  , ,  ), in derivation ring 15.2.6 15.3.2 ), in derivation ring 15.2.6 15.3.2
Weyl algebra ( , ,  , ,  ), Krull dimension of 6.5.8 6.5.10 6.6.15 7.5.9 ), Krull dimension of 6.5.8 6.5.10 6.6.15 7.5.9
Weyl algebra ( , ,  , ,  ), Krull dimension of overrings of 9.1.12 9.4.23 ), Krull dimension of overrings of 9.1.12 9.4.23
Weyl algebra ( , ,  , ,  ), matrices over 7.11.7 ), matrices over 7.11.7
Weyl algebra ( , ,  , ,  ), modules over 5.7.18 ), modules over 5.7.18
Weyl algebra ( , ,  , ,  ), over ), over  4.6.13 11.7.8 4.6.13 11.7.8
Weyl algebra ( , ,  , ,  ), quotient division ring of 6.6.18 9.6.12 ), quotient division ring of 6.6.18 9.6.12
Weyl algebra ( , ,  , ,  ), skew group ring over 7.8.14 12.7.7 ), skew group ring over 7.8.14 12.7.7
Weyl algebra ( , ,  , ,  ), stable range of 11.2.15 ), stable range of 11.2.15
Word 1.6.2
Zariski topology 4.6.14
Zariski — Lipman conjecture 15.6.3
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 being
being 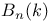 being
being  of
of 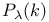 being
being 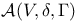 being
being  domain
domain  -
- 
 -algebra
-algebra 
 ,
,  ,
,  )
)