|
|
 |
| Àâòîðèçàöèÿ |
|
|
 |
| Ïîèñê ïî óêàçàòåëÿì |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Gierz G., Hofmann K.H., Keimel K. — Continuous Lattices and Domains |
|
|
 |
| Ïðåäìåòíûé óêàçàòåëü |
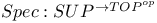 is left adjoint to is left adjoint to 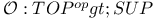 412 V—4.7 412 V—4.7
 space, all irreducible subspaces are Baire 427 V—5.30 space, all irreducible subspaces are Baire 427 V—5.30
 space, all irreducible subspaces are Baire is sober 427 V—5.30 space, all irreducible subspaces are Baire is sober 427 V—5.30
 space, all irreducible subspaces are Baire, all subspaces are Baire 427 V—5.30 space, all irreducible subspaces are Baire, all subspaces are Baire 427 V—5.30
 space, all irreducible subspaces are Baire, order generates a continuous lattice 418 V—5.10 space, all irreducible subspaces are Baire, order generates a continuous lattice 418 V—5.10
 space, all irreducible subspaces are Baire, sobrification is locally compact 418 V—5.10 space, all irreducible subspaces are Baire, sobrification is locally compact 418 V—5.10
 space, all irreducible subspaces are Baire, strict embedding in a locally compact space 418 V—5.10 space, all irreducible subspaces are Baire, strict embedding in a locally compact space 418 V—5.10
 space, all irreducible subspaces are Baire, when space, all irreducible subspaces are Baire, when 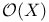 is a continuous lattice 418 V—5.10 is a continuous lattice 418 V—5.10
 space, all irreducible subspaces are Baire, with space, all irreducible subspaces are Baire, with 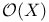 continuous but not locally compact 425 V—5.25(i) continuous but not locally compact 425 V—5.25(i)
 continuous function 328 IV—5.16 continuous function 328 IV—5.16
 continuous function is Scott-continuous on countably based domains 250 III—4.20 continuous function is Scott-continuous on countably based domains 250 III—4.20
 -complete posets 328 IV—5.16 -complete posets 328 IV—5.16
Adjoint, lower see “Lower adjoint”
Adjoint, upper see “Upper adjoint”
Alexander’s Lemma, generalization of 105 I—3.22
Algebraic domain 115 I—4.2
Algebraic domain is Lawson-compact 259 III—5.15
Algebraic domain, as a domain of ideals of a poset 118 I—4.10
Algebraic domain, characterization in Scott topology 143 II—1.15
Algebraic domain, domain of open filters 128 I—4.31
Algebraic domain, products are algebraic 119 I—4.12
Algebraic lattice 115 I—4 115
Algebraic lattice and injective spaces 186 II—3.18
Algebraic lattice and Scott-continuous functions 175 II—2.36
Algebraic lattice is a projective limit of finite lattices 317 IV—4.14
Algebraic lattice is a subalgebra of a power set lattice 121 I—4.16
Algebraic lattice, closed order generating subsets 402 V—2.5(i)
Algebraic lattice, closed topologically generating subsets 402 V—2.5(i)
Algebraic lattice, completely irreducible elements 126 I—4.25 402
Algebraic lattice, distributive see “Distributive algebraic lattice”
Algebraic lattice, irreducibles order-generate 126 I—4.26
Algebraic lattice, smallest closed order generating subset 402 V—2.5(i)
Algebraic lattice, subalgebra of 120 I—4.14
Algebraic lattice, topologically generating subsets 402 V—2.5(ii)
Algebraic lattice, when arithmetic 117 I—4.8
Algebraic lattice, with multiplicative way-below relation 117 I—4.8
Algebraic poset 115 I—4.2
Algebraic semilattice 115 I—4.2
Algebraic semilattice, domain of open filters 128 I—4.31
Antichain 4 O—1.6
Antitone net 2 O—1.2
Approximate identity 165 II—2.13
Arc-chain, in a compact pospace 470 VI—5.9
Arc-chain, in a pospace 469 VI—5.5
Arc-chain, limit of in a compact pospace 469 VI—5.7
Arithmetic lattice 117 I—4.7
Arithmetic lattice, distributive see “Distributive arithmetic lattice”
Arithmetic lattice, pseudo-prime elements 118 I—4.9
Ascending chain condition 52 I—1.3(4)
Ascending chain condition and domains 55 I—1.7
Asymmetric space 485 VI—6.31
atom 13 O—2.7(1)
Atomic lattice 13 O—2.7(1)
Atomless Boolean algebra 14 O—2.7(3)
Atomless Boolean algebra, Auxiliary order see “Auxiliary relation”
Auxiliary relation 57 I—1.11
Auxiliary relation, approximating 59 I—1.13 293
Auxiliary relation, auxiliary relation with strong interpolation property derived from 72 I—1.28 301
Auxiliary relation, interpolation property for 61 I—1.17
Auxiliary relation, multiplicative 107 I—3.27
Auxiliary relation, on a complete lattice 293 IV—3.4
Auxiliary relation, strong interpolation property for 60 I—1.17 301 301
Auxiliary relation, sup closure of 72 I—1.29
Axiom of approximation 54 I—1.6
Baire category theorem 112 I—3.40
Baire Category Theorem for continuous lattices 113 I—3.40.7
Baire Category Theorem for locally compact spaces 113 I—3.40.8
Baire space 45 O—5.13
Basis of a domain 240 III—4.1
Basis of a topology 44 O—5.8
Basis, abstract basis 249 III—4.15
Bi-Scott topology 501 Remark following VII—2.3
Bi-Scott topology, when Hausdorff 505 VII—2.12
Bicontinuous function 218 III—1.21
Bicontinuous lattice 501 VII—2.5 (see also “Linked bicontinuous lattice”)
Bifinite domain 169 II—2.21
Bifinite domain is a projective limit of finite domains 316 IV—4.12
Bifinite domain is Lawson-compact 258 III—5.14
Bitopological space 218 III—1.21
Boolean algebra 12 O—2.6
Boolean algebra is a continuous lattice 124 I—4.20
Boolean algebra is algebraic 124 I—4.20
Boolean algebra is arithmetic 124 I—4.20
Boolean algebra is atomic 124 I—4.20
Boolean algebra is completely distributive 124 I—4.20
Boolean algebra, complete 12 O—2.6
Boolean algebra, prime element in 99 I—3.12
Boolean algebra, way-below relation in 52 I—1.3(3)
Boolean lattice see “Boolean algebra”
Bottom, of a poset 5 O—1.8
Bound, lower 1 O—1.1
Bound, upper 1 O—1.1
Bounded complete domain 54 I—1.6
Bounded complete domain and densely injective spaces 182 II—3.11
Bounded complete domain is an FS-domain 202 II—4.21
Bounded complete domain, closure properties 86 I—2.11
Bounded complete poset 9 O—2.1
C*-algebra 62 I—1.21
C*-algebra, closed prime ideals 109 I—3.34
C*-algebra, primitive ideal of 109 remarks following I—3.34
Cantor tree 434 V—6.4
Cartesian closed category 163 remarks preceding II—2.10
Cartesian closed category, algebraic bounded complete domains and Scott-continuous maps 173 II—2.31
Cartesian closed category, algebraic L-domains and Scott-continuous maps 173 II—2.32
Cartesian closed category, algebraic lattices and Scott-continuous maps 165 II—2.12 173
Cartesian closed category, bifinite domains and Scott-continuous maps 170 II—2.23
Cartesian closed category, bounded complete domains and Scott-continuous maps 173 II—2.31
Cartesian closed category, complete lattices and Scott-continuous maps 164 II—2.10
Cartesian closed category, continuous lattices and Scott-continuous maps 165 II—2.12 173
Cartesian closed category, countably based bifinite domains 251 III—4.22
Cartesian closed category, countably based continuous lattices 247 remarks following III—4.12
Cartesian closed category, countably based FS-domains 251 III—4.21
Cartesian closed category, counterexample: countably based L-domains 251 III—4.23
Cartesian closed category, dcpos and Scott-continuous maps 164 II—2.10
Cartesian closed category, FS-domains and Scott-continuous maps 168 II—2.19
Cartesian closed category, L-domains and Scott-continuous maps 173 II—2.32 202
Category of algebraic lattices and sup and way-below preserving maps 272 IV—1.13
Category of algebraic lattices and sup, and way-below preserving maps 272 IV—1.13
Category of algebraic lattices is cartesian closed 165 II—2.12
Category of algebraic lattices, and inf and directed sup preserving maps 272 IV—1.13
Category of algebraic lattices, and Scott-continuous functions 158 II—2.2
Category of arithmetic lattices, and inf and directed sup preserving maps 272 IV—1.13
Category of bifinte domains, and Scott-continuous functions is cartesian closed 170 II—2.23
Category of complete lattices, and inf and directed sup preserving maps 270 IV—1.9
Category of complete lattices, and inf and directed sup preserving maps, and inf preserving maps 266 IV—1.1
Category of complete lattices, and inf and directed sup preserving maps, and Scott-continuous functions 158 II—2.2
Category of complete lattices, and inf and directed sup preserving maps, and Scott-continuous functions is cartesian closed 164 II—2.10
Category of complete lattices, and inf and directed sup preserving maps, and sup and Scott open set preserving maps 270 IV—1.9
Category of complete lattices, and inf and directed sup preserving maps, and sup preserving maps 266 IV—1.1
Category of continuous lattices and inf and directed sup preserving maps 270 IV—1.9
Category of continuous lattices and Scott-continuous functions 158 II—2.2
Category of continuous lattices and Scott-continuous functions is cartesian closed 165 II—2.12
Category of continuous lattices and sup and way-below preserving maps 270 IV—1.9
Category of continuous lattices, having weight less than a fixed cardinal 330 IV—5.18
Category of continuous semilattices 281 IV—2.2
Category of domains with open filter morphisms 281 IV—2.2
Category of FS-domains, and Scott-continuous functions is cartesian closed 168 II—2.19
Category of posets and lower adjoints 266 IV—1.1
Category of posets and upper adjoints 266 IV—1.1
Category of sup semilattices with 0, and maps preserving sup and 0 272 IV—1.13
Category of sup semilattices, and monotone maps 175 II—2.36
Category, duality of see “Duality of categories”
Chain 4 O—1.6
Chain Modification Lemma, for strict chains 295 IV—3.11
Chain, Complete see “Complete chain”
Chain, gap in 128 I—4.30
Chain, gap in is embeddable in a cube 300 IV—3.21
Chain, way-below relation 51 I—1.3(1)
Character of a dcpo 283 IV—2.7
Character poset of a dcpo 283 IV—2.7
| Clopen set 17 O—2.9
Closed sets in a compact Hausdorff space, form a continuous lattice 454 VI—3.8
Closed sets in a compact Hausdorff space, form a continuous lattice, Vietoris topology on 454 VI—3.8
Closure operator 26 O—3.8
Closure operator is Scott open 270 IV—1.8
Closure operator, image is closed under directed sups 270 IV—1.8
Closure operator, lattice of, on a complete lattice 301 IV—3.25
Closure operator, lattice of, on a complete lattice, on a continuous lattice 301 IV—3.25
Closure operator, on a continuous lattice 87 I—2.12
Closure operator, on an algebraic domain 119 I—4.13
Closure operator, on an algebraic lattice 120 I—4.14
Closure operator, preserves sups 29 O—3.12
Closure system 29 remarks preceding O—3.13 29
Closure system, closed under directed sups 29 O—3.14 82
Co-compact topology 44 O—5.10
Co-compact topology, on a domain 482 VI—6.24
Co-cone, in a category 308 remarks following IV—4.3
Co-prime element 98 I—3.11
Co-prime element, form a dcpo 111 I—3.39
Co-retraction 179 remarks preceding II—3.5
Coalesced sum 73 I—1.31 327
Cofinal map 24 remarks preceding O—3.4
Coherent space 474 VI—6.2
Colimit, in a category 308 remarks following IV—4.3
Compact convex set 110 I—3.36
Compact convex set, closed convex subsets form a continuous lattice 66 I—1.23
Compact convex set, converse of Krein-Milman Theorem 399 V—1.11
Compact convex set, primes in  topologically generate 407 V—3.10 topologically generate 407 V—3.10
Compact convex set, where closed convex subsets do not form a continuous lattice 467 VI—4.6
Compact element 49 I—1.1 115 126
Compact element in the lattice of open sets of a space 127 I—4.28(i)
Compact lattice, characterization of connectivity 472 VI—5.15
Compact lattice, characterization of connectivity has bi-Scott topology 501 VII—2.3
Compact lattice, characterization of connectivity has Scott and dual Scott topology 501 VII—2.3
Compact metric semilattice, with small semilattices 458 VI—3.17
Compact metrizable pospace admits a radially convex metric 445 VI—1.18
Compact open topology 187 remarks preceding II—4.1
Compact pospace 479 VI—6.18
Compact pospace is stably compact 477 VI—6.11
Compact pospace, embedding in a continuous lattice 459 VI—3.21
Compact pospace, metrizable, admits a radially convex metric 445 VI—1.18
Compact pospace, totally order-disconnected 490 VI—7.8
Compact saturated sets 66 I—1.24
Compact saturated sets and open filters in O(X) 146 II—1.20
Compact semilattice 443 VI—1.11
Compact semilattice has enough subinvariant pseudometrics 446 VI—2.3
Compact semilattice has small semilattices 458 VI—3.19
Compact semilattice is complete 443 VI—1.13
Compact semilattice is embeddable in a compact lattice 500 VII—2.1
Compact semilattice is Hausdorff by convention 443 VI—1.11
Compact semilattice is meet continuous 443 VI—1.13
Compact semilattice is stably compact 483 VI—6.25
Compact semilattice with small semilattices 450 VI—3.1
Compact semilattice with small semilattices is a complete continuous semilattice 451 VI—3.4(ii)
Compact semilattice with small semilattices is embeddable in a cube 453 VI—3.7
Compact semilattice with small semilattices, at a point 450 VI—3.1 456
Compact semilattice with small semilattices, characterization 453 VI—3.7
Compact semilattice with small semilattices, continuous morphisms between 452 VI—3.4(iii)
Compact semilattice with small semilattices, quotient has small semilattices 453 VI—3.5
Compact semilattice, characterization of connectivity 470 VI—5.11
Compact semilattice, characterization of connectivity, of continuous homomorphisms 448 VI—2.7
Compact semilattice, characterization of connectivity, of convergence in 447 VI—2.6
Compact semilattice, characterization of connectivity, of order connectivity 471 VI—5.14
Compact semilattice, closed lower sets 449 VI—2.10 456
Compact semilattice, closed lower sets, form a compact lattice 500 remarks preceding VII—2.1
Compact semilattice, closed subsemilattices 448 VI—2.8(i) 449
Compact semilattice, compact elements 449 VI—2.12
Compact semilattice, Fundamental Theorem 451 VI—3.4
Compact semilattice, local minimum is compact 470 VI—5.10(ii)
Compact semilattice, metric, with small semilattices 458 VI—3.17
Compact semilattice, points joined by arc-chains 471 VI—5.12(ii)
Compact semilattice, topology is compatible 443 VI—1.13
Compact semilattice, universal continuous lattice quotient 461 VI—3.24
Compact semilattice, when a continuous lattice 451 VI—3.4 455
Compact semilattice, when a topological lattice 500 VII—2.2
Compact semilattice, which is not a continuous lattice 466 VI—4.5
Compact semitopological semilattice has closed graph 520 VII—4.7
Compact semitopological semilattice has closed graph is topological 521 VII—4.8 521
Compact space 43 O—5.7
Compact totally disconnected semilattice, Fundamental Theorem 457 VI—3.13
Compatible topology, for a poset 440 VI—1.2
Complemented lattice 12 O—2.6
Complete Boolean Algebra see “Boolean algebra”
Complete category 307 remarks preceding IV—4.2
Complete chain 9 O—2.1
Complete chain is a continuous lattice 55 I—1.7
Complete chain, when algebraic 128 I—4.30
Complete continuous semilattice 54 I—1.6
Complete distributive lattice see “Distributive complete lattice”
Complete distributive law 85 I—2.8
Complete Heyting algebra see “Heyting algebra and
Complete lattice 9 O—2.1
Complete lattice is sober 198 II—4.16
Complete lattice with continuous and join continuous Scott topology 518 VII—4.4
Complete lattice with continuous Scott topology 516 VII—4.1 517
Complete lattice, 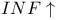 -maps preserve irreducibles 402 V—2.8 -maps preserve irreducibles 402 V—2.8
Complete lattice, characterization when linked bicontinuous 502 VII—2.9
Complete lattice, distributive see “Distributive complete lattice”
Complete lattice, function space is a frame 200 II—4.19
Complete lattice, function space is meet continuous 200 II—4.19
Complete lattice, irreducible elements order generate 402 V—2.7
Complete lattice, lattice of congruences 302 IV—3.27
Complete lattice, lattice of continuous kernel operators 302 IV—3.27
Complete lattice, Lawson topology on see “Lawson topology”
Complete lattice, lower topology on see “Lower topology”
Complete lattice, patch topology on primes is functorial 489 VI—7.6
Complete lattice, Scott topology is a continuous lattice 198 II—4.16 200
Complete lattice, smallest closed order generating subset 402 V—2.7
Complete lattice, sober subspaces in the lower topology 414 V—4.8
Complete lattice, spectrum 408 V—4.1
Complete lattice, when bi-Scott topology is Hausdorff 505 VII—2.12
Complete lattice, when interval topology is Hausdorff 502 VII—2.9
Complete lattice, when Scott topology is locally compact sober 516 VII—4.1(iii)
Complete lattice, when Scott topology is productive 498 VII—1.13
Complete lattice, when topology contains Scott topology 496 VII—1.9
Complete lattice, when topology is Scott topology 496 VII—1.9
Complete semilattice 9 O—2.1
Complete semilattice, closed lower sets 448 VI—1.8(ii)
Complete semilattice, Lawson closed subsemilattices 237 III—3.26
Completely distributive algebraic lattice 521 VII—4.10
Completely distributive lattice 85 I—2.8 85 521
Completely distributive lattice and injective spaces 185 II—3.17
Completely distributive lattice is continuous and dually continuous 102 I—3.16
Completely distributive lattice is embeddable in a cube 303 IV—3.32
Completely distributive lattice is hypercontinuous 515 VII—3.12
Completely distributive lattice is linked bicontinuous 503 VII—2.10
Completely distributive lattice, co-primes form a domain 398 V—1.7
Completely distributive lattice, co-primes form a domain, in 397 V—1.6
Completely distributive lattice, way-way-below relation 303 IV—3.31
Completely irreducible element 125 I—4.21 125
Completely prime filter 414 V—4.10
Composition is Scott-continuous 163 II—2.9 206
Condition, ( ) 433 V—6.1 ) 433 V—6.1
Condition, ( ) 433 V—6.1 ) 433 V—6.1
Conditional sup semilattice 117 I—4.5
Cone, over a diagram 305 IV—4.1
Congruence relation 14 O—2.7(4)
Congruence relation, on a continuous lattice 88 I—2.14
Construction of function space algebras, on DCPO 339 IV—6.11
Construction of Scott topology algebras, on 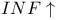 339 IV—6.12 339 IV—6.12
Continuous (semi)lattice is meet-continuous 56 I—1.8
Continuous frame 101 I—3.15 (see also “Distributive continuous lattice”)
Continuous Heyting algebra see “Distributive continuous lattice”
Continuous lattice 54 I—1.6 (see also “Complete lattice”)
Continuous lattice has small semilattices 451 VI—3.4
Continuous lattice is a compact semilattice 224 III—2.15 303
Continuous lattice is a retract of a power set lattice 123 I—4.18
Continuous lattice is an FS-domain 202 II—4.21
Continuous lattice is an injective space in the Scott topology 179 II—3.5
Continuous lattice is embeddable in a cube 292 IV—3.3
|
|
 |
| Ðåêëàìà |
 |
|
|
 |
|
Î ïðîåêòå
|
|
Î ïðîåêòå



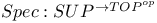 is left adjoint to
is left adjoint to 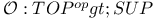
 space, all irreducible subspaces are Baire
space, all irreducible subspaces are Baire 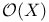 is a continuous lattice
is a continuous lattice  continuous function
continuous function  topologically generate
topologically generate 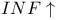 -maps preserve irreducibles
-maps preserve irreducibles 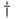 )
)  )
)