|
|
 |
| јвторизаци€ |
|
|
 |
| ѕоиск по указател€м |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Rencher A.C., Barnett V., Bradley R.A. (Ed) Ч Multivariate Statistical Inference and Applications |
|
|
 |
| ѕредметный указатель |
Expected value of sample variance ![$[E(s^2)]$](/math_tex/e760a5391dcacf7ebf4e092c87bca40582.gif) 3 3
Expected value of sum or product of random variables 5
Expected value of univariate random variable [E(y)] 2
Experimentwise error rates 2
F-test(s) and 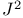 67 67
F-test(s), partial F-test for discriminant functions 211
F-test(s), partial F-test in MANOVA 110
F-test(s), tables of power of the F-test 423Ч426
Factor analysis 377Ч398
Factor analysis and grouped data 393Ч394
Factor analysis and regression 394Ч395
Factor analysis of correlation matrix 379Ч380. 383 385Ч386. 392
Factor analysis of covariance matrix 377Ч388. 391Ч395
Factor analysis, and principal components 361 377
Factor analysis, applicability of the model 392Ч393
Factor analysis, assumptions 378Ч379
Factor analysis, communality 379 381
Factor analysis, eigenvalues 381Ч383
Factor analysis, eigenvectors 381
Factor analysis, factor scores 391Ч392
Factor analysis, factors 377Ч379
Factor analysis, factors, interpretation of 378 381 390Ч391
Factor analysis, fit of the model 392Ч393
Factor analysis, loadings 378 381Ч385
Factor analysis, loadings, estimation of 381Ч385
Factor analysis, loadings, estimation of, comparison of methods 385Ч386
Factor analysis, loadings, estimation of, iterated principal factor method 384
Factor analysis, loadings, estimation of, maximum likelihood method 384Ч385
Factor analysis, loadings, estimation of, principal axis method 383Ч384
Factor analysis, loadings, estimation of, principal component method 381Ч383
Factor analysis, loadings, estimation of, principal factor method 383Ч384
Factor analysis, model 378Ч379
Factor analysis, model, scale invariance of 379Ч380
Factor analysis, nonlinear models 394
Factor analysis, number of factors to retain 381 386Ч387
Factor analysis, robustness to nonnormality 394
Factor analysis, rotation of loadings in the model 380
Factor analysis, rotation of sample loadings and factors 387Ч390
Factor analysis, rotation of sample loadings and factors, interpretation of factors 390Ч391
Factor analysis, rotation of sample loadings and factors, oblique rotation 389Ч390
Factor analysis, rotation of sample loadings and factors, oblique rotation, pattern matrix 390
Factor analysis, rotation of sample loadings and factors, oblique rotation, structure matrix 390
Factor analysis, rotation of sample loadings and factors, orthogonal rotation 388Ч389
Factor analysis, rotation of sample loadings and factors, orthogonal rotation, equamax 389
Factor analysis, rotation of sample loadings and factors, orthogonal rotation, quartimax 389
Factor analysis, rotation of sample loadings and factors, orthogonal rotation, varimax 388
Factor analysis, specific variance 379
Factor analysis, tests of significance 386Ч387
Factor analysis, three-mode factor analysis 394
Factor analysis, total variance 382
FisherТs LSD test 134
Fracture data 171
Function of a random variable, distribution of 38Ч40
Gauss Ч Markov theorem, multivariate 284Ч285
Gauss Ч Markov theorem, univariate 268
Generalized linear models 266
Generalized quadratic form 54Ч55
Generalized variance 8Ч9 40
Glucose data 35
H matrix 122 203
Height-weight data 4
Hierarchical linear models 306
HotellingТs  see У see У -testsФ -testsФ
Hypothesis tests see УTests of hypothesesФ
Idempotent matrix 414
Identity matrix 400
Imputation see УMissing dataФ
Independence and zero covariance 5 46Ч47
Independence, two random variables 5
Independence, two random vectors 46
Influence and canonical correlation 333
Influence and canonical variates 333
Influence and classification analysis 262
Influence and discriminant analysis (descriptive) 206
Influence and multivariate regression 301Ч303
Influence and principal components 372Ч373
Intercorrelation, index of 20Ч22
Intercorrelation, index of, condition number 20
Intercorrelation, index of, variance inflation factor 21
Inverse matrix 407
j vector and J matrix 400
Jackknife 327
Jacobian 39
Largest root test see УRoyТs testФ
Latent root regression 363 370
Latent roots see УEigenvaluesФ
Lawley Ч Hotelling test 130Ч131 292 295Ч296
Lawley Ч Hotelling test, F-approximations 130Ч131
Lawley Ч Hotelling test, table of critical values 441Ч445
Least squares estimators 267Ч268 281Ч282
Length of a vector 402
Library data 397
Likelihood function 49
Likelihood ratio 62Ч65
Likelihood ratio test for discriminant functions 209
Likelihood ratio test in canonical correlation 321Ч326
Likelihood ratio test in multivariate regression 289Ч299
Likelihood ratio test in principal components 349Ч351 357Ч359
Likelihood ratio test in random-x regression 279
Likelihood ratio test, comparing covariance matrices 138
Likelihood ratio test, generalized likelihood ratio 262
Likelihood ratio test, one-sample  -test 71Ч72 -test 71Ч72
Likelihood ratio test, one-sample t-test 62Ч65
Likelihood ratio test, one-way MANOVA 122Ч126
Likelihood ratio test, random effects MANOVA 111
Likelihood ratio test, two-sample  -test 91Ч92 -test 91Ч92
Likelihood ratio test, two-sample t-test 85Ч86
Linear classification functions 231Ч232 236
Linear classification rule 231Ч232 236
Linear function(s) of random variables 14Ч20
Linear function(s) of random variables, confidence intervals for 74 92Ч94
Linear function(s) of random variables, correlation of two linear combinations 15 20
Linear function(s) of random variables, covariance matrix for several linear combinations 15Ч16 20
Linear function(s) of random variables, covariance matrix for two sets of linear combinations 16 20
Linear function(s) of random variables, covariance of two linear combinations 15 19
Linear function(s) of random variables, linear combinations of subvectors 16Ч17 20
Linear function(s) of random variables, mean of a single linear combination 14 19
Linear function(s) of random variables, variance of a single linear combination 15 19
Linear hypotheses in ANOVA and MANOVA 163Ч171
Linear hypotheses in multivariate regression 295Ч297
Linear hypotheses in univariate regression 272Ч273
Linear hypotheses, one sample 72Ч74 79Ч80 83Ч85
Linear hypotheses, one sample, for 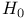 : : 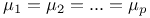 83Ч85 83Ч85
Linear hypotheses, two samples 94Ч95
Log linear models 2
Logistic classification 254Ч259
Logistic classification, comparison with linear classification 256Ч257
Logistic classification, quadratic logistic classification 258
Logistic classification, several groups 258
Logistic regression 254Ч255
Longley data 368
LSD test 134
Mahalanobis distance 22Ч23
Manova 121 (see also УMultivariate analysis of varianceФ)
Matrix (matrices), addition of 401
Matrix (matrices), algebra of 399Ч416
Matrix (matrices), bilinear form 404
Matrix (matrices), centering matrix 9Ч10
Matrix (matrices), characteristic equation 411
Matrix (matrices), Cholesky decomposition 42 408
Matrix (matrices), correlation matrix 11Ч12 (see also УCorrelation matrixФ)
Matrix (matrices), covariance matrix 8Ч9 (see also УCo variance matrixФ)
Matrix (matrices), covariance matrix, positive definite 9Ч10
Matrix (matrices), data matrix 7
Matrix (matrices), definition 399
Matrix (matrices), determinant 409
Matrix (matrices), diagonal matrix 11 400
Matrix (matrices), diagonal of a matrix 400
Matrix (matrices), differentiation 50Ч51 414Ч416
Matrix (matrices), eigenvalues 411Ч414 (see also УEigenvaluesФ)
| Matrix (matrices), eigenvalues and determinant 411
Matrix (matrices), eigenvalues and trace 411
Matrix (matrices), eigenvalues of inverse 411
Matrix (matrices), eigenvalues of positive definite matrix 412
Matrix (matrices), eigenvalues of product 411
Matrix (matrices), eigenvalues of symmetric matrix 412
Matrix (matrices), eigenvalues, characteristic equation 411
Matrix (matrices), eigenvectors 411Ч414 (see also УEigenvectorsФ)
Matrix (matrices), factoring of 402
Matrix (matrices), idempotent matrix 414
Matrix (matrices), identity 400
Matrix (matrices), inverse 407
Matrix (matrices), inverse of partitioned matrix 407
Matrix (matrices), inverse of product 407
Matrix (matrices), J matrix 400
Matrix (matrices), j vector 400
Matrix (matrices), multiplication of 401
Matrix (matrices), nonsingular matrix 407
Matrix (matrices), O (zero matrix) 401
Matrix (matrices), operations with 401Ч406
Matrix (matrices), operations with, distributive law 401
Matrix (matrices), operations with, factoring 402
Matrix (matrices), operations with, product 401Ч406
Matrix (matrices), operations with, product of matrix and vector 405
Matrix (matrices), operations with, product of matrix and vector as linear combination 405
Matrix (matrices), operations with, product of vectors 401Ч402
Matrix (matrices), operations with, product, noncommutativity of 401
Matrix (matrices), operations with, product, with diagonal matrix 404
Matrix (matrices), operations with, sum 401Ч402
Matrix (matrices), operations with, sum, commutativity of 401
Matrix (matrices), orthogonal matrices 410
Matrix (matrices), partitioned matrices 404Ч406
Matrix, Perron Ч Frobenius theorem 414
Matrix, positive definite matrix 408
Matrix, positive semidefinite matrix 408
Matrix, products 401 404
Matrix, quadratic form 48Ч49 404
Matrix, quadratic form, generalized quadratic form 54Ч55
Matrix, random matrix, expected value of 10
Matrix, rank 406
Matrix, rank, full rank 406
Matrix, singular matrix 407 408
Matrix, size of a matrix 399
Matrix, spectral decomposition 412
Matrix, square root matrix 413
Matrix, trace 410
Matrix, triangular matrix 400
Matrix, vectors see УVector(s)Ф
Matrix, zero matrix (O) and zero vector (0) 401
Maximum likelihood estimators 49Ч52
Maximum likelihood estimators in regression 286Ч289
Maximum likelihood estimators of covariance matrix 49Ч51
Maximum likelihood estimators of mean vector 49Ч51
Maximum likelihood estimators, invariance property of 51Ч52
Maximum likelihood estimators, likelihood function 49
Maximum likelihood estimators, multivariate normal 49
Mean see also УExpected valueФ
Mean deviation 28
Mean population 2
Mean sample 2
Mean vector, partitioned 12Ч13
Mean vector, population 8
Mean vector, sample 7
Misclassification rates see УError rate(s)Ф
Missing data 23Ч27 299Ч300
Missing data and classification analysis 262Ч263
Missing data and hypothesis tests 299Ч300
Missing data, estimation of missing values (imputation) 24Ч27
Missing data, listwise deletion 23
Missing data, missing at random 23
Missing data, missing completely at random 23
Missing data, pairwise deletion 24
Models, generalized linear models 2
Models, log linear models 2
Moment generating function 43Ч45
Moments 42Ч43
Multicolinearity 40 327 364 368
Multidimensional scaling 373
Multiple correlation 275 (see also УR^2Ф)
Multiple regression see УRegression multipleФ
Multivariate analysis 1
Multivariate analysis of covariance see УAnalysis of covariance multivariateФ
Multivariate analysis of variance (Manova) 121Ч200
Multivariate analysis of variance (MANOVA) and canonical correlation 312 333
Multivariate analysis of variance (MANOVA) and eigenvalues 131
Multivariate analysis of variance (MANOVA), additional information, test for 174Ч178
Multivariate analysis of variance (MANOVA), approximate tests, based on M-estimators 194
Multivariate analysis of variance (MANOVA), approximate tests, based on ranks 194
Multivariate analysis of variance (MANOVA), contrasts 145Ч148 150Ч151
Multivariate analysis of variance (MANOVA), contrasts, orthogonal 146
Multivariate analysis of variance (MANOVA), contrasts, simultaneous 146Ч148
Multivariate analysis of variance (MANOVA), discriminant functions 157
Multivariate analysis of variance (MANOVA), discriminant, functions 128 151 УDiscriminant
Multivariate analysis of variance (MANOVA), FisherТs LSD test 134
Multivariate analysis of variance (MANOVA), H and E matrices 122 124
Multivariate analysis of variance (MANOVA), higher order models 151
Multivariate analysis of variance (MANOVA), individual variables, tests on 134
Multivariate analysis of variance (MANOVA), Lawley Ч Hotelling test 130Ч131
Multivariate analysis of variance (MANOVA), Lawley Ч Hotelling test, F-approximations 130Ч131
Multivariate analysis of variance (MANOVA), Lawley Ч Hotelling test, table of critical values 441Ч445
Multivariate analysis of variance (MANOVA), likelihood ratio test see УWilksТ  Ф Ф
Multivariate analysis of variance (MANOVA), mixed models 151
Multivariate analysis of variance (MANOVA), one-way classification 121Ч134
Multivariate analysis of variance (MANOVA), one-way classification, contrasts 145Ч148
Multivariate analysis of variance (MANOVA), one-way classification, unbalanced data 155Ч160
Multivariate analysis of variance (MANOVA), one-way model 155Ч160
Multivariate analysis of variance (MANOVA), PillaiТs test 130Ч131
Multivariate analysis of variance (MANOVA), PillaiТs test, F-approximations 130
Multivariate analysis of variance (MANOVA), PillaiТs test, table of critical values 435Ч437
Multivariate analysis of variance (MANOVA), power of the tests 137Ч138 140Ч142
Multivariate analysis of variance (MANOVA), power of the tests, tables of power of the F-test 423Ч426
Multivariate analysis of variance (MANOVA), robustness of test statistics 137Ч138
Multivariate analysis of variance (MANOVA), RoyТs test (union-intersection) 127Ч129
Multivariate analysis of variance (MANOVA), RoyТs test (union-intersection), table of critical values 435Ч437
Multivariate analysis of variance (MANOVA), selection of variables, stepwise 177
Multivariate analysis of variance (MANOVA), step-down test 177
Multivariate analysis of variance (MANOVA), stepwise discriminant analysis 177Ч178
Multivariate analysis of variance (MANOVA), test on individual variables 134
Multivariate analysis of variance (MANOVA), test statistics and eigenvalues 131
Multivariate analysis of variance (MANOVA), test statistics, comparison of 132 135Ч138
Multivariate analysis of variance (MANOVA), test statistics, power of 137Ч138 140Ч142
Multivariate analysis of variance (MANOVA), test statistics, robustness of 137Ч138
Multivariate analysis of variance (MANOVA), tests on a subvector 174Ч178
Multivariate analysis of variance (MANOVA), tests on individual variables 157
Multivariate analysis of variance (MANOVA), tests on individual variables, experimentwise error rate 134
Multivariate analysis of variance (MANOVA), tests on individual variables, protected tests 134
Multivariate analysis of variance (MANOVA), two-way classification 148Ч151
Multivariate analysis of variance (MANOVA), two-way classification, contrasts 150Ч151
Multivariate analysis of variance (MANOVA), two-way classification, discriminant functions 151
Multivariate analysis of variance (MANOVA), two-way classification, interaction 148Ч149
Multivariate analysis of variance (MANOVA), two-way classification, main effects 148Ч149
Multivariate analysis of variance (MANOVA), two-way classification, model 148
Multivariate analysis of variance (MANOVA), two-way classification, test statistics 149
Multivariate analysis of variance (MANOVA), two-way model 168Ч174
Multivariate analysis of variance (MANOVA), two-way model, constrained model 169Ч171
Multivariate analysis of variance (MANOVA), unbalanced data 152 155Ч160 168Ч174
Multivariate analysis of variance (MANOVA), unbalanced data, Bonferroni confidence intervals 157
Multivariate analysis of variance (MANOVA), unbalanced data, contrasts 156Ч160
Multivariate analysis of variance (MANOVA), unbalanced data, simultaneous confidence intervals 156Ч157
Multivariate analysis of variance (MANOVA), union-intersection test see УRoyТs testФ
Multivariate analysis of variance (MANOVA), WilksТ A (likelihood ratio) 122Ч126
Multivariate analysis of variance (MANOVA), WilksТ A (likelihood ratio), Chi-square approximation 126
Multivariate analysis of variance (MANOVA), WilksТ A (likelihood ratio), effect of each variable 132Ч134
Multivariate analysis of variance (MANOVA), WilksТ A (likelihood ratio), F-approximation 125Ч126
Multivariate analysis of variance (MANOVA), WilksТ A (likelihood ratio), properties of 124Ч126
Multivariate analysis of variance (MANOVA), WilksТ A (likelihood ratio), table of critical values 427Ч434
Multivariate analysis of variance (MANOVA), WilksТ A (likelihood ratio), transformations to exact F 125Ч126
Multivariate central limit theorem 53
Multivariate data 1Ч2 7
Multivariate descriptive procedures 1
|
|
 |
| –еклама |
 |
|
|
 |
|
ќ проекте
|
|
ќ проекте



![$[E(s^2)]$](/math_tex/e760a5391dcacf7ebf4e092c87bca40582.gif)
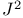

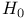 :
: 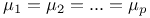
 Ф
Ф