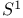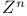Авторизация
Поиск по указателям
Lee J.M. — Introduction to Topological Manifolds
Обсудите книгу на научном форуме Нашли опечатку?
Название: Introduction to Topological ManifoldsАвтор: Lee J.M. Аннотация: This book is an introduction to manifolds at the beginning graduate level. It contains the essential topological ideas that are needed for the further study of manifolds, particularly in the context of differential geometry, algebraic topology, and related fields. Its guiding philosophy is to develop these ideas rigorously but economically, with minimal prerequisites and plenty of geometric intuition. A course on manifolds differs from most other introductory mathematics graduate courses in that the subject matter is often completely unfamiliar. Unlike algebra and analysis, which all math majors see as undergraduates, manifolds enter the curriculum much later. It is even possible to get through an entire undergraduate mathematics education without ever hearing the word "manifold." Yet manifolds are part of the basic vocabulary of modern mathematics, and students need to know them as intimately as they know the integers, the real numbers, Euclidean spaces, groups, rings, and fields. In his beautifully conceived introduction, the author motivates the technical developments to follow by explaining some of the roles manifolds play in diverse branches of mathematics and physics. Then he goes on to introduce the basics of general topology and continues with the fundamental group, covering spaces, and elementary homology theory. Manifolds are introduced early and used as the main examples throughout. John M. Lee is currently Professor of Mathematics at the University of Washington.
Язык: Рубрика: Математика /Геометрия и топология /Общая топология /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 2000Количество страниц: 385Добавлена в каталог: 19.04.2005Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
10 59 60 170 11 11 171 345 345 345 see “Boundary” 342 22 344 62 34 344 119 55 344 343 97 347 44 51 51 344 356 204 339 315 155 152 61 179 235 AB (category of abelian groups) 171 Abelian group 203 353 Abelian group, free 204 Abelian groups, category of 171 Abelian Lie group 11 Abelianization 227 Abelianization of fundamental groups of surfaces 228 Abelianization, characteristic property 227 Abelianization, functor 231 Abelianization, uniqueness 231 Absolute value 20 Abstract simplex 96 Abstract simplicial complex 96 Accumulation point 26 348 Action of a group 59 266 Action of a group, quotient by 61 Action of fundamental group on fiber 245 Action, continuous 60 Action, free 60 Action, left 59 266 Action, proper 266 Action, right 59 Action, transitive 60 Affine chain 293 Affine hyperplane 92 Affine map 92 Affine singular simplex 293 Affine subspace 92 Algebra, fundamental theorem of 191 Algebraic geometry 11 Algebraic topology 6 Algebraic variety 12 Algebraically closed 11 Algorithm, reduction 196 Ambient Euclidean space 17 Analysis situs 4 angle 7 347 Angle, function, for a path in 179 182 Angle-sum theorem 7 Antipodal map 248 321 Antipodal map, degree of 321 Antipodal map, homotopic to identity 322 Antisymmetric relation 341 Area 7 Associative 352 Associativity of path class product 153 Automorphism group of covering 248 Automorphism of covering 248 Automorphism of group 354 Axiom of Choice 346 Axiom, Cartesian product 340 Axiom, existence 343 Axiom, power set 339 Axiom, specification 338 Axiom, union 339 Axioms for set theory 338—347 Baire category theorem 85 Ball, closed 348 Ball, closed is a closed set 349 Ball, closed is a manifold with boundary 62 Ball, Euclidean 31 Ball, Euclidean, regular 83 Ball, open 348 Ball, open is an open set 349 Ball, unit, in 22 Barycenter 110 Barycentric subdivision 110 315 Base of covering 234 Base point 151 Base point, change of 154 Base point, nondegenerate 212 Based at a point 151 Basis and continuity 29 Basis for a topology 29 Basis for discrete topology 29 Basis for Euclidean topology 29 Basis for free abelian group 204 Basis for metric topology 29 Basis for product topology 48 50 Basis for subspace topology 42 Basis for trivial topology 29 Basis in a set 27 Basis of Euclidean balls 38 Basis, countable 32 Basis, criterion 27 Basis, neighborhood 32 Basis, neighborhood, countable 32 Basis, standard, for 204 Basis, topology generated by 27 Belongs to a set 338 Betti number 328 Big crunch 14 Bijective 342 Body, rigid 13 Bound, lower 341 Bound, upper 341 Boundary 25 26 Boundary of a boundary 294 Boundary of a boundary, simplicial 323 Boundary, face 93 Boundary, manifold with 34 Boundary, of a manifold with boundary 34 38 Boundary, of a simplex 93 Boundary, of a singular simplex 293 Boundary, operator 297 Boundary, operator, simplicial 323 Boundary, operator, singular 293 Boundary, simplicial 324 Boundary, singular 294 Boundary, topological 35 Bounded, above 341 Bounded, below 341 Bounded, not a topological property 22 Bounded, set 349 Bouquet of circles 55 Branch 9 Brouwer fixed point theorem 192 334 Calabi — Yau manifold 15 Cardinality 344 Cardinality of fibers of covering 236 247 Cartesian coordinates 3 Cartesian product 340 346 Cartesian product, axiom 340 Cartesian product, finite 346 Cartesian product, infinite 346 Category 170 Category of Abelian groups 171 Category of commutative rings 171 Category of complex vector spaces 171 Category of groups 171 Category of real vector spaces 171 Category of rings 171 Category of sets 171 Category of simplicial complexes 171 Category of topological spaces 171 Category, first 86 Category, homotopy 173 Category, pointed homotopy 173 Category, second 86 Category, theorem, Baire 85 Cauchy sequence 350 Cauchy sequence vs. convergent sequence 350 Center of a group 208 Center of gravity 110 Chain, affine 293 Chain, complex 297 Chain, complex, homology groups of 297 Chain, group, simplicial 323 Chain, group, singular 293 Chain, homotopic 303 Chain, homotopy 303 317 Chain, map 297 Chain, simplicial 323 Chain, singular 293 Change of base point 154 Characteristic property, abelianization 227 Characteristic property, disjoint union topology 62 Characteristic property, free abelian group 204 Characteristic property, free group 200 Characteristic property, free product 198 Characteristic property, product topology 49 Characteristic property, quotient topology 56 Characteristic property, subspace topology 41 Characteristic zero 330 Characterization of quotient, maps 57 Chart 31 Chart on a manifold with boundary 34 Chart, coordinate 31 Choice function 346 Choice, axiom of 346 circle 2 45 Circle as coset space of 61 Circle, fundamental group 180 Circle, generating 45 Circle, homotopy lifting property 181 Circle, path lifting property 181 Circle, representative 157 Circle, unique lifting property 181 Circle, unit 45 class 339 Class, equivalence 340 Classical mechanics 12 Classification of 1-manifolds 118 Classification of 2-manifolds 6 137 229 Classification of 3-manifolds 7 Classification of coverings 283 Classification of manifolds 6 Classification of n-manifolds 7 Classification of surface presentations 137 Classification of surfaces 6 137 229 Classification of torus coverings 286 Closed ball 348 Closed ball is a closed set 349 Closed ball is a manifold with boundary 62 Closed cube 351 Closed map 27 Closed map, lemma 79 Closed map, product of 62 Closed map, vs. homeomorphism 27 Closed set 24 26 Closed set and continuity 25 Closed set and limit points 26 Closed set in a compact space 74 Closed set in a discrete space 25 Closed set in a metric space 349 Closed set in a subspace 41 Closed set, intersection, in a metric space 349 Closed set, intersection, in a topological space 24 Closed set, union, in a metric space 349 Closed set, union, in a topological space 24 Closure 25 26 Closure and sequences 38 Closure, normal 201 Cluster point 26 348 Coboundary 329 Coboundary, operator 329 Cochain, complex 329 Cochain, map 330 Cochain, singular 329 Cocycle 329 Codomain 342 Coffee cup 5 Coherent topology 99 Coherent topology of finite union 114 Cohomology with field coefficients 331 332 Cohomology, functor 330 Cohomology, Mayer — Vietoris sequence 335 Cohomology, singular 329 Cohomology, topological invariance 330 Collection 338 Combinatorial equivalence 112 Combinatorial group theory 203 Combinatorial invariant 113 142 Combinatorial invariant, Euler characteristic 113 142 Combinatorial invariant, orientability 115 Commutative diagram 355 Commutative rings, category of 171 Commutator subgroup 227 Compact vs. limit point compact 77 78 Compact vs. sequentially compact 78 Compact, implies closed and bounded 350 Compact, limit point 76 Compact, locally 81 Compact, relatively 82 Compact, sequentially 77 Compact, set, continuous image 73 352 Compact, set, continuous image in a Hausdorff space 74 Compact, set, continuous image in a metric space 350 Compact, topological space 73 Compact, topological space, product of 74 Compact, topological space, quotient of 74 Compactification, one-point 89 Complement 339 Complementary edge pair 139 Complete, metric space 350 Complete, ordered field 343 Complex, analysis 8 Complex, analytic 8 Complex, chain 297 Complex, general linear group 10 Complex, manifold 33 Complex, numbers 344 Complex, projective space 12 62 Complex, simplicial, abstract 96 Complex, simplicial, category of 171 Complex, simplicial, Euclidean 93 Complex, special linear group 11 Complex, vector spaces, category of 171
Реклама
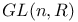(general linear group) 
(set of morphisms) 
(complex special linear group)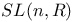 
(special linear group) 
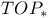(pointed topological category) 
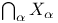, (intersection) 
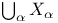(union) 
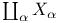(disjoint union) 
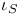(inclusion map) 
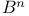(unit ball in 
) 
(set of complex numbers)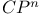 
(complex projective space) 
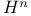(upper half space) 
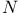(set of natural numbers) 
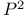(projective plane) 
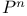(real projective space) 
(set of rational numbers) 
(set of real numbers)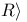 
(free vector space) 
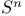(unit n-sphere) 
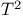(torus) 
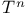(n-torus) 
(set of integers) 
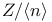(integers modulo n) 
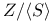(free abelian group) 
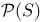(power set) 
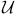-small chain 
(fundamental group) 
(fundamental group) 
(exponential quotient map) 
|
|
О проекте