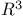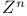Авторизация
Поиск по указателям
Lee J.M. — Introduction to Topological Manifolds
Обсудите книгу на научном форуме Нашли опечатку?
Название: Introduction to Topological ManifoldsАвтор: Lee J.M. Аннотация: This book is an introduction to manifolds at the beginning graduate level. It contains the essential topological ideas that are needed for the further study of manifolds, particularly in the context of differential geometry, algebraic topology, and related fields. Its guiding philosophy is to develop these ideas rigorously but economically, with minimal prerequisites and plenty of geometric intuition. A course on manifolds differs from most other introductory mathematics graduate courses in that the subject matter is often completely unfamiliar. Unlike algebra and analysis, which all math majors see as undergraduates, manifolds enter the curriculum much later. It is even possible to get through an entire undergraduate mathematics education without ever hearing the word "manifold." Yet manifolds are part of the basic vocabulary of modern mathematics, and students need to know them as intimately as they know the integers, the real numbers, Euclidean spaces, groups, rings, and fields. In his beautifully conceived introduction, the author motivates the technical developments to follow by explaining some of the roles manifolds play in diverse branches of mathematics and physics. Then he goes on to introduce the basics of general topology and continues with the fundamental group, covering spaces, and elementary homology theory. Manifolds are introduced early and used as the main examples throughout. John M. Lee is currently Professor of Mathematics at the University of Washington.
Язык: Рубрика: Математика /Геометрия и топология /Общая топология /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 2000Количество страниц: 385Добавлена в каталог: 19.04.2005Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Solid geometry 7 South pole 45 Space 18 Space, curve 2 Space, discrete 19 Space, Euclidean 347 Space, Hausdorff 31 Space, identification 52 Space, metric 348 Space, product 48 Space, quotient 52 Space, topological 18 Space, variable 152 Space-filling curve 188 Spacetime 14 Spacetime, homogeneous and isotropic 14 Special linear group 11 Special loop 190 Special orthogonal group 11 Special unitary group 11 Specification axiom 338 Sphere 3 44 Sphere is simply connected 217 Sphere with n handles 129 Sphere, Euler characteristic 143 Sphere, fundamental group 188 Sphere, not a retract of the ball 334 Sphere, presentation 133 Sphere, quotient of disk 120 Sphere, quotient of square 120 Sphere, singular homology 309 Sphere, turning inside out 5 Sphere, unit 23 44 Sphere, unit in 3 Square root, complex 8 Stable trajectory 14 Stack of pancakes 234 Standard basis for 204 Standard presentation 133 137 Standard simplex 292 Star, open 114 Star-shaped 162 Steinitz, Ernst 112 Stereographic projection 45 62 187 Stereographic projection and one-point compactification 89 Straight-line homotopy 150 Strictly increasing 345 String 15 String, theory 14 Strong deformation retract 161 Strong deformation retraction 161 Structure theorem, covering group 250 SU(n) (special unitary group) 11 Subbasis 36 Subcategory 171 Subcategory, full 171 Subcomplex of a Euclidean simplicial complex 95 Subcomplex of an abstract simplicial complex 96 Subcover 32 73 350 Subcover, countable 32 Subdividing 133 Subdivision 109 Subdivision, barycentric 110 315 Subdivision, elementary 112 Subdivision, operator, singular 316 Subgraph 101 Subgroup 353 Subgroup of a cyclic group 357 Subgroup of a free abelian group 205 Subgroup of a topological group 59 61 63 Subgroup, normal 354 Subsequence 345 Subset 338 Subset of a countable set 344 Subset, proper 338 subspace 39 40 Subspace of a metric space 40 Subspace of a subspace 41 Subspace, affine 92 Subspace, closed sets 41 Subspace, Hausdorff 42 Subspace, second countable 42 Subspace, topology 40 Subspace, topology, basis for 42 Subspace, topology, characteristic property 41 Subspace, topology, uniqueness 47 Sum in a category 175 Sum in a category, uniqueness 175 Sum in the category of groups 199 Sum in the topological category 177 Sum, connected 126 Sum, connectedis a manifold 126 Sum, connectedwith sphere 129 Sum, direct 177 Supremum 343 surface 3 117 119 Surface of genus n 144 Surface of revolution 45 Surface, classification 6 Surface, fundamental group of 220 Surface, fundamental group of, abelianized 228 Surface, nonorientable 144 Surface, orientable 144 Surface, presentation 132 Surface, presentation and fundamental group 217 Surface, presentation, classification 137 Surface, Riemann 9 Surface, universal covering of 282 Surjective 342 symmetric 340 Symmetric group 353 Symmetry of a metric 348 Terminal point of a path 150 Terminal vertex 131 tetrahedron 92 Theta space 162 Thurston geometrization conjecture 7 Thurston, William 7 Tietze, Heinrich 112 203 time variable 152 Tofu sandwich theorem 254 TOP (topological category) 171 Topological boundary 35 Topological category 171 Topological category, pointed 171 Topological embedding 40 Topological group 58 Topological group, discrete 58 Topological group, discrete subgroup of 270 Topological group, fundamental group of 191 Topological group, product of 59 Topological group, quotient of 63 Topological group, subgroup of 59 63 Topological group, universal covering space of 290 Topological invariance of Euler characteristic 327 Topological invariance of homology groups 296 Topological invariance of the fundamental group 159 Topological invariant 6 Topological manifold 33 Topological property 4 22 Topological space 18 Topologically equivalent 4 22 Topologically equivalent, presentations 133 Topologist’s sine curve 69 72 88 topology 4 18 Topology, algebraic 6 Topology, discrete 19 Topology, disjoint union 37 Topology, euclidean 19 Topology, generated by a basis 27 Topology, generated by a subbasis 36 Topology, metric 19 Topology, product 48 Topology, quotient 52 Topology, relative 40 Topology, subspace 40 Topology, trivial 19 Torsion, element 205 Torsion, free 205 Torsion, subgroup 205 Torus 3 51 Torus as a quotient of the square 55 79 Torus, coverings of 270 286 Torus, Euler characteristic 143 Torus, fundamental group 189 220 Torus, homeomorphic to doughnut surface 51 80 Torus, n-dimensional 51 Torus, n-dimensional, coset space of 61 Torus, n-holed 129 Torus, presentation 133 Total ordering 341 Totally ordered set 37 341 Trajectory, periodic 14 Trajectory, stable 14 Transformation, elementary 133 Transformation, linear 20 Transitive 340 Transitive, group action 60 Transitivity of covering group 249 Translation, left 59 63 Translation, right 59 Transpose of linear map 173 Transposition 353 TREE 163 Tree, contractible 163 Tree, maximal 214 Triangle inequality 89 348 Triangle inequality for hyperbolic metric 289 Triangle, Euclidean 7 Triangulable 100 Triangulation 100 Triangulation of 1-manifolds 102 Triangulation of 2-manifolds 104 Triangulation of 3-manifolds 105 Trigonometric function 20 Trivial group 353 Trivial topology 19 Turning the sphere inside out 5 Twisted edge pair 139 Two origins, line with 62 Tychonoff’s theorem 75 Type, homotopy 161 U(n) (unitary group) 11 Uncountable set 344 Unfolding 135 Union of an indexed collection 345 Union of closed sets in a metric space 349 Union of closed sets in a topological space 24 Union of open sets in a metric space 349 Union of open sets in a topological space 18 Union of sets 339 Union, axiom 339 Union, connectedness of 67 Union, countable 344 Union, disjoint 340 345 Union, disjoint, topology 37 Unique lifting property 237 Unique lifting property of the circle 181 Uniqueness of abelianization 231 Uniqueness of covering spaces 260 Uniqueness of free abelian group 208 Uniqueness of free group 201 Uniqueness of free product 199 Uniqueness of product topology 49 Uniqueness of quotient spaces 57 Uniqueness of subspace topology 47 Unit ball in 22 Unit circle 45 Unit interval 54 Unit sphere 23 44 Unitary group 11 Unitary group, special 11 Universal coefficient theorem 330 Universal covering 261 Universal covering of a topological group 290 Universal covering of compact surfaces 282 Universal covering of n-holed torus 275 Universal covering, space 261 Universal covering, space, existence 262 Universal mapping properties 174 Upper bound 341 Upper half space 34 Variety, algebraic 12 VECTc (category of complex vector spaces) 171 Vector field 192 322 Vector field, index 192 Vector space 347 Vector space, free 97 Vector spaces, complex, category of 171 Vector spaces, real, category of 171 VECTr (category of real vector spaces) 171 Vertex of a presentation 131 Vertex of a simplex 92 Vertex of an abstract simplicial complex 96 Vertex, initial 131 Vertex, map 95 96 Vertex, point 124 Vertex, scheme 96 Vertex, terminal 131 Vertices see “Vertex” Volume 7 254 Wedge of spaces 55 Wedge of spaces, fundamental group, singular homology 334 Well-ordered 88 341 Well-ordering theorem 88 346 Winding number 179 182 Word 130 194 Word, empty 194 Word, problem 203 Word, reduced 195 World sheet 15 Zero-dimensional, Euclidean space 31 347 Zero-dimensional, homology 298 Zero-dimensional, manifold 37 Zigzag lemma 310 Zorn’s Lemma 347
Реклама
 |
|
О проекте
|
|
О проекте