Авторизация
Поиск по указателям
Liu Q., Erne R. — Algebraic Geometry and Arithmetic Curves
Обсудите книгу на научном форуме Нашли опечатку?
Название: Algebraic Geometry and Arithmetic CurvesАвторы: Liu Q., Erne R. Аннотация: This book is a general introduction to the theory of schemes, followed by applications to arithmetic surfaces and to the theory of reduction of algebraic curves. The first part introduces basic objects such as schemes, morphisms, base change, local properties (normality, regularity, Zariski's Main Theorem). This is followed by the more global aspect: coherent sheaves and a finiteness theorem for their cohomology groups. Then follows a chapter on sheaves of differentials, dualizing sheaves, and grothendieck's duality theory. The first part ends with the theorem of Riemann-Roch and its application to the study of smooth projective curves over a field. Singular curves are treated through a detailed study of the Picard group. The second part starts with blowing-ups and desingularization (embedded or not) of fibered surfaces over a Dedekind ring that leads on to intersection theory on arithmetic surfaces. Castelnuovo's criterion is proved and also the existence of the minimal regular model. This leads to the study of reduction of algebraic curves. The case of elliptic curves is studied in detail. The book concludes with the fundamental theorem of stable reduction of Deligne-Mumford. The book is essentially self-contained, including the necessary material on commutative algebra. The prerequisites are therefore few, and the book should suit a graduate student. It contains many examples and nearly 600 exercises.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 2002Количество страниц: 463Добавлена в каталог: 21.05.2007Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
338—345 338—345 173 89 383 73 275 282 210 68 381 385 256 51 377 383 80 163 94 271 81 94 135 496 182 377 355 389 258 2 81 260 267 198 10 10 306 299 306 430 279 431 279 189 35 34 126 164 50 80 94 44 166 60 81 30 205 446 100 18 385 53 50 82 229 34 158 173 166 201 172 169 229 165 158 37 106 402 354 354 216 238 239 497 496 395 27 63 a(X), Abelian rank of a curve 314 A*, set of invertible elements of a ring A 44 Abelian rank of a curve 314 316 521 531 555 Abelian variety 298 Abelian variety, good reduction 502 Abelian variety, models 456 Abelian variety, potential good reduction 502 Abelian variety, serni-Abelian reduction see “Reduction” Abelian variety, torsion points 299 Abhyankar's theorem on the desingularization of surfaces 362 Abhyankar's theorem on the exceptional locus of birational morphisms 411 Absolute value 467 Adjunction formula 239 390 Affine line 27 43 Affine morphism 172 191 Affine morphism, and base change 193 Affine open subset 44 Affine open subset, complement has codimension 1 124 Affine open subset, of a projective space 76 Affine open subset, of an affine space 76 Affine scheme 43 Affine scheme, and quasi-coherent sheaves 160 Affine scheme, cohomology of sheaves 186 Affine scheme, is separated 100 Affine scheme, Serre's criterion 187 Affine space 47 Affine space, open subset 192 Affine variety 55 Algebra 5 Algebra, finite 29 Algebra, finitely generated 20 29 Algebra, flat 6 Algebra, graded 20 Algebra, homogeneous 53 Algebra, simple 227 Algebraic group 307 314 Algebraic set 30 Algebraic set, and rational points 49 Algebraic variety 55 Algebraic variety, complete 107 Algebraic variety, Jacobian criterion of smoothness 130 Algebraic variety, proper 105 109 112 Algebraic variety, smooth 141—142 219 220 Algebraization of coherent sheaves 553 Alteration 361 374 407 Ample divisor 266 (see also “Ample sheaf”) Ample divisor, on a curve 315 Ample divisor, the support is connected 266 Ample sheaf 169—178 194 197 198 Ample sheaf, on a curve 305 Ample sheaf, on a projective scheme over a Dedekind scheme 205 Ample sheaf, on a proper scheme 196 Ann(M), annihilator of a module M 13 Annihilator 13 173 Approximation theorem 379 531 Arcwise connectedness 331 Arithmetic surface 353 Arithmetic surface, minimal 418 423—424 Arithmetic surface, relatively minimal 418 Arithmetic surface, smooth 368—370 370 388 418 526 Arithmetic surface, with normal crossings 404 406—408 425 426 530 Artin — Rees lemma 21 Ass(M), set of associated prime ideals of M 253 Associated point 254 Associated prime ideal 253 Automorphisms, of a curve 300 Automorphisms, of a minimal arithmetic surface 418 Automorphisms, of a projective space 176 Automorphisms, of a stable curve 520 529 Automorphisms, of models 460 A[[T]], ring of formal power series with coefficients in A 18 Base change 81 Base point 176 Betti number 472 Betti number, of the dual graph associated to a curve 511 Bezout identity 384 Birational map 112 Birational map, and base change 146 194 Birational map, of normal fibered surfaces 354—355 Birational map, of smooth fibered surfaces 370 Birational morphism 96 304 Birational morphism, and blowing-up 328 332 Birational morphism, and dimension 334 Birational morphism, and multiplicities 410 Birational morphism, and Picard groups 408 Birational morphism, decomposition into a sequence of blowing-ups 396 Birational morphism, elimination of indeterminacy 396 Birational morphism, exceptional locus see “Exceptional locus” Birational morphism, of normal fibered surfaces 369—371 417 Birational morphism, of regular fibered surfaces 395 Birational morphism, quasi-finite 152 Birational morphism, to a Dedekind scheme 124 Birational morphism, to a normal scheme 201 Birational morphism, to a regular scheme 272 Blowing-up 318—332 Blowing-up, along a closed point 402 Blowing-up, along a regular closed subscheme 325 Blowing-up, and finite morphisms 344 Blowing-up, universal property 324 Branch locus 290 347 CaCl(X), group of Cartier divisors modulo linear equivalence 257 Canonical divisor 282 389 Canonical divisor, on a curve 282 288 Canonical divisor, on a fibered surface 389 429 Canonical map 288 296 Canonical model 440 457 Canonical sheaf 239 (see also “Dualizing sheaf”) Cartier divisor 256 Cartier divisor, effective 256 Castelnuovo's criterion 416 Catenary ring 332—333 Catenary scheme 333 Cayley — Hamilton identity 122 Chevalley's Theorem 154 Chow's Lemma 109 196 Class group 58 268 Closed fiber 84 347 Closed immersion 38 155 164 Closed point 27 Closed point, a scheme without closed point 114 Closed point, in an algebraic variety 60 76 Closed subscheme 46 Closed subscheme, of a projective scheme 53 Closed subscheme, of an affine scheme 47 Cochain 180 Cochain, alternating 180 Codifferent 250 289 codim(Z,X), codimension of Z in X 69 Codimension 69 75 333 Cohen — Macaulay see “Module” “Ring” “Scheme” Coherent sheaf 161 Coherent sheaf, direct image 163 Coherent sheaf, finiteness of cohomology 195 Coherent sheaf, higher direct image 195 Coherent sheaf, on a projective scheme 167 Cohomological flatness 209 385 393 Cohomology (Cech) 182 Cohomology and flat base change 190 Complete intersection 206—207 287 Complete intersection, and duality 250 Complete intersection, is cohomologically flat 209 Complete intersection, is geometrically connected 207 Completion see “Formal completion” Completion of a curve 125 546 COMPLEX 4 35 Complex, exact 4 Component group see “Group of components” Conductor 250 conic 147—148 285 418 Conic, good reduction 479 Conic, reduction 529 Conic, regular model 426 Connected component 66 97 Constructive subset 98 Contraction 357—360 371—372 430—437 450 Contraction, of (–2)-curves 440 447 516 Contraction, of exceptional divisors 416—417 Coordinates, system of 129 Covering 544 Cup product 194 Curve 75 275 Curve, (-2)-curve 434 Curve, (-l)-curve 412 Curve, a proper curve over a field is projective 315 Curve, a regular proper flat curve over a Dedekind domain is projective 353 Curve, affine 315 Curve, affine plane 302 Curve, completion 125 546 Curve, flat curve over a scheme 347 Curve, normal but not smooth 278 Curve, of arithmetic genus see “Conic” Curve, projective plane 110 280 300 CYCLE 252 267 Cycle, direct image 271 397 Cycle, of codimension 1 267 Cycle, positive 267 Cycle, prime 267 Cycle, restriction to an open subset 269 Cycle, support 267 D(f), open subset associated to a function f 27 d-uple embedding 176 209 de Jong's theorem on alteration 407 Decomposition group 146 532 Dedekind domain 11 115 344 Dedekind scheme 115—116 Dedekind scheme, is regular 128 Dedekind scheme, open subscheme 123 Degeneration 84 Degree, of a Cartier divisor 275 Degree, of a divisor in a projective space 287 Degree, of a finite morphism 176 Degree, of an invertible sheaf 282 Degree, transcendence 73 Deligne — Mumford's theorem on stable reduction 533 Depth 335—345 depth M, depth of a module 335 Derivation 210 218 Derived functor 185 Desingularization 361 Desingularization, a scheme without desingularization 373 Desingularization, embedded resolution 404 Desingularization, in the strong sense 361 Desingularization, minimal 424 Determinant 236 241 Differential 178 Differential forms 127 216 Differential forms, of higher order 238 Dilatation 395 dim A, dimension of a ring A 69 dim X, dimension of a topological space X 68 DIMENSION 68—77 96 144 333—335 Dimension, and surjective morphism 76 Dimension, and surjective morphisms 110 Dimension, formula 333
Реклама
 |
|
О проекте
|
|
О проекте



 , property
, property 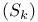 , property
, property 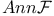 , annihilator of an
, annihilator of an 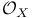 -module
-module 
 , algebra obtained by extension of scalars
, algebra obtained by extension of scalars 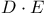 , intersection of a divisor D with a vertical divisor E
, intersection of a divisor D with a vertical divisor E  , transcendence degree of K over k
, transcendence degree of K over k 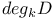 , degree of a Cartier divisor D
, degree of a Cartier divisor D 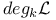 , degree of an invertible sheaf
, degree of an invertible sheaf 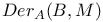 , derivations of B into M
, derivations of B into M 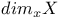 , dimension of X at
, dimension of X at 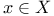
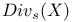 , group of divisors with support in
, group of divisors with support in 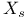
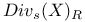 , real vector space
, real vector space 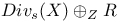
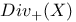 , effective Cartier divisors
, effective Cartier divisors  , open subset associated to a homogeneous element f
, open subset associated to a homogeneous element f 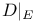 , restriction of a Cartier divisor to a closed subscheme E
, restriction of a Cartier divisor to a closed subscheme E 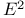 , self-intersection of a vertical divisor E
, self-intersection of a vertical divisor E  , product of two morphisms
, product of two morphisms 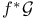 , pull-back of a sheaf of modules
, pull-back of a sheaf of modules 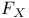 , absolute Frobenius
, absolute Frobenius  , direct image of a cycle
, direct image of a cycle 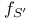 , morphism obtained by base change
, morphism obtained by base change 
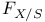 , relative Frobenius
, relative Frobenius 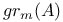 , graded ring associated to an ideal m
, graded ring associated to an ideal m 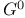 , identity component of an algebraic group G
, identity component of an algebraic group G  , Cech cohomology group of
, Cech cohomology group of 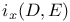 , intersection number of D and E at x
, intersection number of D and E at x 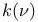 , residue field of a valuation
, residue field of a valuation 
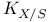 , canonical divisor on a fibered surface
, canonical divisor on a fibered surface 
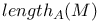 , length of an A-module M
, length of an A-module M 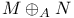 , tensor product over A
, tensor product over A 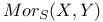 , set of morphisms of S-schemes from X to Y
, set of morphisms of S-schemes from X to Y 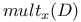 , multiplicity of a Cartier divisor at a point x
, multiplicity of a Cartier divisor at a point x 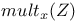 , multiplicity of a cycle at a point x
, multiplicity of a cycle at a point x ![$M[\alpha]$](/math_tex/71b4a202da0ec40b8e02059e864ab83d82.gif) , the
, the  -torsion elements of M
-torsion elements of M 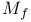 , localization of M at f
, localization of M at f  , localization of M at a prime ideal
, localization of M at a prime ideal 
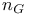 , multiplication by n in a commutative group G
, multiplication by n in a commutative group G  , group of divisors of degree 0
, group of divisors of degree 0  , arithmetic genus of a curve X
, arithmetic genus of a curve X 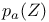 , arithmetic genus of a vertical divisor Z
, arithmetic genus of a vertical divisor Z 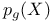 , geometric genus
, geometric genus 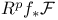 , higher direct image of a sheafs
, higher direct image of a sheafs 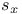 , germ of a section s
, germ of a section s  , restriction of a section s to an open subset V
, restriction of a section s to an open subset V 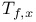 , tangent map
, tangent map  , closed subscheme associated to a quasi-coherent sheaf of ideals
, closed subscheme associated to a quasi-coherent sheaf of ideals  , closed subset defined by a homogeneous ideal I
, closed subset defined by a homogeneous ideal I 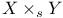 , fibered product of the S-schemes X, Y
, fibered product of the S-schemes X, Y  twist by the Frobenius
twist by the Frobenius 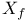 , open subset of X associated to a function
, open subset of X associated to a function 
 , reduced scheme associated to X
, reduced scheme associated to X  , S'-scheme obtained by base change
, S'-scheme obtained by base change 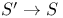
 , set of common zeros of the polynomials
, set of common zeros of the polynomials 
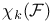 , Euler — Poincare characteristic of a coherent sheaf
, Euler — Poincare characteristic of a coherent sheaf  , discriminant of a Weierstrass model
, discriminant of a Weierstrass model 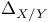 , diagonal morphism
, diagonal morphism  , formal completion of A for the I-adic topology
, formal completion of A for the I-adic topology 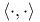 , symmetric bilinear form on
, symmetric bilinear form on 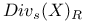
 , projective space associated to a vector space V
, projective space associated to a vector space V  , projective space over a ring A
, projective space over a ring A  , projective space over a scheme S
, projective space over a scheme S  , conormal sheaf of X in Y
, conormal sheaf of X in Y  , restriction of a sheaf to an open subset U
, restriction of a sheaf to an open subset U 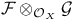 , tensor product of two
, tensor product of two 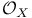 -modules
-modules 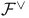 , dual of an
, dual of an 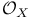 -module
-module 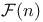 , twist of
, twist of 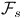 , pull-back of
, pull-back of 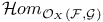 , sheaf of homomorphisms from
, sheaf of homomorphisms from 
 , nth tensor power of an invertible sheaf
, nth tensor power of an invertible sheaf  normal sheaf of X in Y
normal sheaf of X in Y 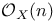 , twist of
, twist of  , direct sum indexed by I
, direct sum indexed by I 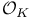 , valuation ring of K
, valuation ring of K  , multiplicity of a hypersurface at a point x
, multiplicity of a hypersurface at a point x 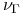 , valuation associated to a closed irreducible subset Г of codimension 1
, valuation associated to a closed irreducible subset Г of codimension 1 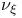 , valuation associated to a point of codimension 1
, valuation associated to a point of codimension 1 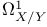 or
or  sheaf of relative differential forms
sheaf of relative differential forms 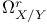 differential forms of order r
differential forms of order r 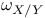 dualizing (or canonical) sheaf
dualizing (or canonical) sheaf  , group of components of the Neron model of E
, group of components of the Neron model of E 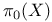 , scheme of connected components of X
, scheme of connected components of X  -Process
-Process  , radical of an ideal I
, radical of an ideal I  , Zariski closure of {x}
, Zariski closure of {x} 