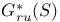Авторизация
Поиск по указателям
Hewitt E., Ross K.A. — Abstract Harmonic Analysis (Vol. 1)
Обсудите книгу на научном форуме Нашли опечатку?
Название: Abstract Harmonic Analysis (Vol. 1)Авторы: Hewitt E., Ross K.A. Аннотация: Abstract theory remains an indispensable foundation for the study of concrete cases. It shows what the general picture should look like and provides results that are useful again and again. Despite this, however, there are few, if any introductory texts that present a unified picture of the general abstract theory.A Course in Abstract Harmonic Analysis offers a concise, readable introduction to Fourier analysis on groups and unitary representation theory. After a brief review of the relevant parts of Banach algebra theory and spectral theory, the book proceeds to the basic facts about locally compact groups, Haar measure, and unitary representations, including the Gelfand-Raikov existence theorem. The author devotes two chapters to analysis on Abelian groups and compact groups, then explores induced representations, featuring the imprimitivity theorem and its applications. The book concludes with an informal discussion of some further aspects of the representation theory of non-compact, non-Abelian groups.
Язык: Рубрика: Математика /Анализ /Продвинутый анализ /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Издание: Second EditionГод издания: 1979Количество страниц: 519Добавлена в каталог: 02.04.2005Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
7 29 29 —31 269 303 303 302 303 272 13 360 109 416—417 402 202 109 434 419 124 125 125 124 124 7 28—29 29—31 201 209 78 3 404 414 3 50 433 367 415 370 198 256 240 27 3 434 104 92 3 366 256 27 265 299 269 119 120 167 272 (see also “M_a(G)$”) 7 29 29—31 7 28—29 350 29—31 212—213 7 29 29—31 247 250 252 7 64 29 29—31 259 108 400 116 202—203 109 433 400 118 11 13—14 118 13 118 314 13 0-dimensional group, small subgroups of 62 0-dimensional space 11 a-adic integers 109 (see also “ a-adic numbers 109 (see also “ a-adic solenoid 114 Abe, M. 425 439 Abelian group, nondiscrete topologies 27 (see also “Group”) Absolutely continuous measure 180 269 Additive function 452 Adjoint homomorphism 392 Adjoint in 310 Adjoint in 310 Adjoint in an algebra 313 Adjoint in M(G) 300 Adjoint n 299 Adjoint operator 466 Alaoglu’s theorem 458 Aleksandrov, A.D. 215 Aleksandrov, P. S. 47 386 Alexander, J.W. 78 354 398 Algebra 469 Algebra 313 Algebra , convolution 263 Algebra homomorphism 470 Algebra imbedded in algebra with unit 470 Algebra of sets 118 Algebra with adjoint operation 313 Almost everywhere 124 Almost periodic function 247 (see also “ Annihilator 365 Anzai, H. 399 425 439 Approximate unit 303 Approximation theorems 431—432 435 Arbitrarily small sets 62 Arcwise connected space 11 Arens, R.F. 439 Arhangel’skii, A. 398 Aronszajn, N. 465 Aubert, K. E. 214 Automorphism group 426—429 Automorphism group, examples 433 Automorphism group, inner automorphism subgroup 439 Automorphism group, modular function of 438 Automorphism group, non locally compact 435 Automorphism, inner 4 Automorphism, topological 426 208 Baer, R. 31 51 Baire category theorem 42 456 Baire measurable function 118 Baire sets 118 Baire sets and Haar measure 280 Balanced neighborhood 453 Balcerzyk, S. 425 Banach 313 Banach algebra 469 Banach fields, characterized 473 Banach space 455 Banach space, reflexive 457 Banach space, weak topology 458 Banach space, weak- 458 Banach — Steinhaus theorem 456 Basis of a group 442 Basis of a measure space 215 Basis, orthonormal 465 Beaumont, R.A. 425 Beurling, A. 261 282 Bilinear functional 453 Bilinear functional, bounded 468 Birkhoff, G. 83 243 461 Bochner, S. 1 282 Bohr compactification 430 Borel measurable function 118 Borel sets 118 Bounded bilinear functional 468 Bounded linear function 454 Bounded linear function, relatively 46l Bounded order 439 Bounded order, characterized 449 Bourbaki, N. 32 46 52 134 135 150 166 184 461 Braconnier, J. 56 60 208 278 373 395 396 398 399 417 425 426 438 Buck, R.C. 275 283 Bunyakovskii’s inequality 137 Bunyakovskii’s inequality, characterized as Banach algebra 481 Bunyakovskii’s inequality, closed ideals in 482 Bunyakovskii’s inequality, conjugate space of 170 175 Bunyakovskii’s inequality, multiplicative linear functionals of 483 Bunyakovskii’s inequality, structure space is X 483 Calderon, A. P. 261 Cancellation laws 98—99 Cancellation semigroup 258 Caratheodory outer measure 123 Cardinal number of character groups 382 396 Cardinal number of nondiscrete locally compact groups 31 Cardinal number, notation for 2 Cartan, E. 32 Cartan, H. 57 135 214 283 398 Cartesian product of sets 2 Category, Baire 456 Category, theorem of Baire 42 456 Cauchy — Bunyakovskii — Schwarz inequality 464 Cauchy’s inequality 137 Center of a topological group 46 64 429 Character group 355 Character group and structure space of 358 360 361 Character group of 404 414 Character group of 405 Character group of 405 Character group of 402—403 Character group of 402 Character group of 400 Character group of 403 Character group of closed subgroup 380 Character group of finite group 367 Character group of local direct product 373 Character group of products 362—365 Character group of quotient group 365 Character group of R 367 Character group of T 366 Character group of weak direct product 364 Character group of Z 366 Character group with only one element 350 370 Character group, 360 Character group, P-topology of 361 Character group, topologically isomorphic with the group 422 Character of a measure space 215 Character of a measure space and dimension of 225 Character(s) 345 Character(s), 346 Character(s), extensibility of 380 Character(s), product of 355 Character(s), real 390 393 Character(s), sufficiently many 345 Characteristic function 2 Chevalley, C. 67 106 214 Cluster point of a net 14 Cofinal set 2 Cohen, L.W. 78 Cohen, P.J. 184 Commutative algebra 469 Commutator subgroup 358 Compact elements 92 103 Compact groups have discrete character groups 362 Compact groups, algebraic structure of Abelian 410—414 Compact groups, small subgroups 61—62 Compact groups, torsion characterized 406 Compact groups, torsion-free characterized 406 Compact space 11 Compactification, Bohr 430 Compactly generated group 35 Complete lattice 462 Complete measure space 216 Complete regularity of groups 70 Completely regular space 9 Completely simple semigroup 101 Complex algebra 469 Complex linear space 452 Complex measure(s) 118 Complex measure(s), Fubini’s theorem for 183 Complex measure(s), product of 182 Complex n-dimensional space 3 Component 11 Component of e as direct factor 395 Component of e in topological groups 60—63 Composition of functions 2 Concentrated a measure 180 Conjugacy classes of a group 5 Conjugate elements of a group 5 Conjugate of a matrix 7 Conjugate space 457 Conjugate space of 170 175 Conjugate space of 148 Conjugate-linear function 452 Connected groups 62 383 385 390 410 Connected groups, center of 64 429 Connected space 11 Continuous measure 269 132 Continuous representation 341 Continuous representation, strongly 335 Continuous representation, weakly 335 Continuous unitary representations, equivalences 346 Convergent net 14 Convex combination 452 Convex set 452 Convex set in ordered group 25 Convolution 262 Convolution algebra 263 Convolution algebra, 263 Convolution algebra, 275 Convolution algebra, 265 Convolution algebra, 275 Convolution algebra, noncommutative 275—276 Convolution of functions and measures 286 290—298 Convolution of measures 266—269 Convolution, associativity of 262 Convolution, involving unbounded functionals 278 Convolution, using right Haar measure 306 Correspondence 2 Corson, H.H. 81 Cotlar, M. 375 Countable set 2 Countably additive measure 118 Countably compact space 11 Covering (cover) 2 Cyclic groups, finite 5 Cyclic representation 315 Cyclic vector 315 Daniell, P. J. 134 Dantzig, D. van 32 51 67 78 83 117 425 Daublebsky von Sterneck, R. 282 Davis, H.F. 215 Day, M.M. 238 241 245 372 Devinatz, A. 399
Реклама
 |
|
О проекте
|
|
О проекте



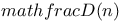
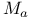
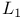
 -mesh
-mesh  -topology
-topology 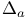
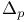
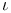 -almost everywhere
-almost everywhere 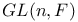
 (rational numbers)
(rational numbers)  (real line)
(real line) 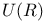
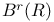

 (integers)
(integers) 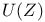
 as convolution algebra
as convolution algebra 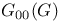
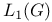 , isomorphic with
, isomorphic with 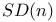
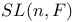
![$[0,\infty]$](/math_tex/73a3477a7ce601a3104ece4f55fbb1c082.gif)
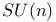

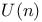
 -functions
-functions 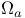

 -algebra of sets
-algebra of sets  -representation
-representation  -mesh
-mesh 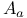 ”)
”)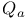 ”)
”)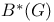
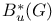
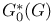
 topology
topology 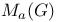
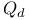
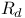
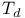

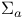
 -topology of
-topology of 
 -measurable implies continuous
-measurable implies continuous 
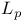
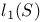
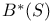

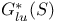 and
and