Авторизация
Поиск по указателям
Hsiung C.-C. — A first course in differential geometry
Обсудите книгу на научном форуме Нашли опечатку?
Название: A first course in differential geometryАвтор: Hsiung C.-C. Аннотация: The origins of differential geometry go back to the early days of the differential calculus, when one of the fundamental problems was the determination of the tangent to a curve. With the development of the calculus, additional geometric applications were obtained. The principal contributors in this early period were Leonhard Euler (1707- 1783), GaspardMonge(1746-1818), Joseph Louis Lagrange (1736-1813), and AugustinCauchy (1789-1857). A decisive step forward was taken by Karl FriedrichGauss (1777-1855) with his development of the intrinsic geometryon a surface. This idea of Gauss was generalized to n( > 3)-dimensional spaceby Bernhard Riemann (1826- 1866), thus giving rise to the geometry that bears his name. This book is designed to introduce differential geometry to beginning graduate students as well as advanced undergraduate students (this introduction in the latter case is important for remedying the weakness of geometry in the usual undergraduate curriculum). In the last couple of decades differential geometry, along with other branches of mathematics, has been highly developed. In this book we will study only the traditional topics, namely, curves and surfaces in a three-dimensional Euclidean space E3. Unlike most classical books on the subject, however, more attention is paid here to the relationships between local and global properties, as opposed to local properties only. Although we restrict our attention to curves and surfaces in E3, most global theorems for curves and surfaces in this book can be extended to either higher dimensional spaces or more general curves and surfaces or both. Moreover, geometric interpretations are given along with analytic expressions. This will enable students to make use of geometric intuition, which is a precious tool for studying geometry and related problems; such a tool is seldom encountered in other branches of mathematics.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1981Количество страниц: 361Добавлена в каталог: 17.02.2014Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Abelian group 24 Accumulation point 5 Admissible arc 301 Affine group 53 Affine transformation 52 Affine transformation, isometric 56 Angle, between curves on surface 199 Angle, between vectors 25 Angle, extenor 252 Angle, interior 252 Angle, interior, of geodesic triangle 264 Antipodal, mapping 246 Antipodal, point 303 Arc length 83 84 88 Arc length, of curve on surface 176 Arc length, reparametrization of curve by 84 Area, element of 271 Associated Bertrand curves 93 Asymptotic curve 192—193 Asymptotic curve, of developable surface 208 Asymptotic direction 184 Axiom of Completeness 9 Axis of helix 79 Axis of revolution 158 Basis, natural 28 Basis, of a space 28 Basis, orthonormal 28 Beltrami — Enneper Theorem 197 Bertrand curve 92—96 97 98 Binormal 91 Binormal, vector 92 Bolzano, intermediate value theorem of 14 Bonnet’s theorems 212 272 305 309 Boundary of set 3 Boundary point of set 3 Bounded set 9 Buffon’s needle problem 128 130 Cartesian product 3 Catenary 168 220 Catenoid 168 220 Catenoid, as minimal surface of revolution 220 Cauchy — Crofton formula 128 Cauchy — Riemann equations 202 Cauchy, convergence condition 10 Cauchy, sequence 10 Cauchy’s formula 115 Center of curvature 95 103 104 185 Chain rule 20 Christoffel symbols 210 Christoffel’s problem 286 Christoffel’s problem, uniqueness theorem for 289 Circular helix 95 104 Closed form 71 Closed plane curve 85 86 Closed plane curve, diameter of 113 123 Closed plane curve, exterior of 110 Closed plane curve, interior of 110 Closed set 4 Closure of set 5 Cluster point 5 Cohn — Vossen's theorem 275 Compact space 11 Complement of set 5 38 Complete surface 249 294 298 299 305 Completely integrable system 212 Component, of set 8 Component, of vector 23 Cone 161 Cone, as ruled surface 215 Cone, local isometry of, to plane 198 Confocal quadrics 204 206 Conformal mapping 198 Conformal mapping, Liouville’s theorem for 206 Conformal mapping, local 199 Conformal mapping, local, of planes 202 Conformal mapping, local, of Poincare half-plane to Euclidean plane 251 Conformal mapping, local, of spheres to planes 200 201 Conformal mapping, local, of surfaces 200 Conjugate directions 194 Conjugate directions, net 194 197 Conjugate point 302—305 Connected space 7 Conoid 202 Contact of order 101 Continuous mapping 6 Convergence of sequence 10 Convex hull 137 289 Convex set 137 Convex surface 275 Convex surface, in direction 284 Convex surface, of constant Gaussian curvature 280 Convex surface, of constant mean curvature 285 Coordinate functions, of curve on surface 172 Coordinate functions, of vector field on 32 Coordinate system 151 Coordinate system, isothermal 200 222 Coordinate system, spherical 204 Coordinate, neighborhood 151 Covariant differential 225 Covering 11 Covering, finite 11 Covering, open 11 Covering, sub 11 Critical point 19 180 Crofton's theorem 143 Cubic parabola 104 Curvature, center of see Center of curvature Curvature, Gaussian 189 196 211 270 Curvature, Gaussian, intrinsic property of 211 272 Curvature, Gaussian, of torus 194 Curvature, geodesic 299 Curvature, lines of 192 204 206 Curvature, lines of, differential equation of 189 Curvature, mean 189 196 270 Curvature, normal 177 183 184 Curvature, of curve 90 91 96 Curvature, principal 184 189 Curvature, radius of 91 116 Curve, asymptotic see Asymptotic curve Curve, Bertrand see Bertrand curve Curve, closed 85 86 Curve, continuous 85 Curve, convex 1 13 134 135 137 Curve, curvature of 90 91 96 Curve, Mannheim 98 Curve, of class 85 Curve, of constant width 113 114 138 Curve, on surface 171—172 Curve, oriented 89 Curve, periodic 86 Curve, piecewise (sectionally) regular (smooth) 82 86 133 Curve, plane 92 93 136 Curve, rectifiable 83 Curve, regular 82 Curve, reparametrzation of 80—81 83 Curve, reparametrzation of, by arc length 84 Curve, simple 85 Curve, smooth 82 86 Curve, spherical 104 Curve, torsion of 91 92 96 Curve, vertex of 123 138 139 Cusp 79 Cycloid 87 Cylinder 4 157 244 Cylinder, as ruled surface 215 Cylinder, first fundamental form of 179 Cylinder, geodesics of 239 Cylinder, local isometry of, to plane 198 Cylinder, parametrization of 158 Cylindrical helix 97 107 Darboux frame 176 Decomposition of space 38 Deformation of curve 147 Derivative mapping 41 Determinant of three vectors 25 Developable surface 208 Diameter, of closed plane curve 113 123 Diameter, of set 9 Diffeomorphism 43 Diffeomorphism, area-preserving 202 Diffeomorphism, orientation-preserving or exteriorreversing 245 Differential, form see Form differential Differential, mapping 41 Differential, of function 64 Dimension of space 28 Direct sum of spaces 38 Direction,asymptotic 184 Direction,principal 184 189 Directional derivative 33 173 Disconnected space 7 Distance, in 2 24 Distance, on surface 293—294 Divergence of sequence 10 Dual basis 40 65 Dual space 39 Dupin, indicatrix 184 Dupin, theorem 204 Element, of arc 176 Element, of area 218 271 Ellipsoid 157 186 244 Empty set 4 Enneper's minimal surface 223 Envelope, of family of curves 112 Envelope, of family of tangent planes 227 Equator 154 Equivalent knots 86 Erdmann’s theorem 76 Euclidean coordinate functions, of form 65 Euclidean coordinate functions, of mapping 35 Euclidean coordinate functions, of vector field 30 85 Euclidean group of rigid motions 56 Euclidean space 1 Euler angles 50 Euler characteristic 255 256 Euler equation 76 Euler’s formula 184 Evolute 98 ex 4 Exact form 71 Existence and uniqueness theorem, for system of partial differential equations of first order 309 Existence and uniqueness theorem,for system of ordinary differential equations of first order 307 Existence theorem for curves 105 307 Extenor multiplication 67 Exterior angle 252 Exterior angle, derivative 69 Exterior differential form 64 267 Exterior point 3 Exterior product 69 Exterior, of closed plane curve 110 Extremal curve of integral 76 Extremum 18 Extremum, absolute 20 Extremum, relative (local) 18 20 Extrinsic property 227 Fary — Milnor theorem, H 5 Fenchel's Theorem 140 Field, frame 31 Field, Frenet frame 90 Field, vector see Vector field Finite covering 11 First fundamental form 175 176 First fundamental form, coefficients of 177 First variation, of integral 75 First variation, of length 300 Form, closed 71 Form, exact 71 Form, exterior differential 64 267 Form, fundamental 175—178 269 Form, on 64 Form, on surface 173 174 Form, structural equations for 75 269 Four-vertex theorem 123 Frame 31 Frame, Darboux 176 Frame, moving 88 Frame, positively or negatively oriented 60 85 Frame, right- or left-handed oriented 60 85 Frenet formulas 90 109 Frenet frame Held 90 Function, continuous 6 Function, harmonic 222 Function, height 145 Function, Laplacian of 222 Function, of class 170 Function, periodic 19 Function, regular value of 155 244 Fundamenial forms 175—178 269 Fundamenial forms, relation among 208 Fundamental theorem, for curves 105 Fundamental theorem, for surfaces 212 272 309 Gauss — Bonnet formula, global 257 Gauss — Bonnet formula, local 256 Gauss — Bonnet theorem 256 Gauss — Bonnet theorem, applications of 261—264 Gauss, equations 210 211 Gauss, mapping 176 Gauss, trihedron 176 Gaussian curvature see Curvature Gaussian Generalized uniqueness theorem for curves 105 General linear group 47 Genus of surface 256 Geodesic circles 236 Geodesic curvature 229—231 239 Geodesic disk 293 Geodesic parallels 232 Geodesic polar coordinates 234 Geodesic polar coordinates, area of geodesic circle in 237 Geodesic polar coordinates, first fundamental form in 233 Geodesic polar coordinates, Gaussian curvature in 236 Geodesic polar coordinates, perimeter of geodesic circle in 236 Geodesic torsion 237—238 Geodesic torsion, of asymptotic curves 240 Geodesic triangle 264 Geodesic triangle, excess of 264 Geodesics 229 Geodesics, as shortest arcs, in large 298 299 Geodesics, as shortest arcs, in small 233 304 Geodesics, as shortest arcs, of sphere 239 Geodesics, characterizations of 231 Geodesics, closed 261 262 Geodesics, differential equations of 231 Geodesics, existence and uniqueness of 231 Geodesics, field of 232 Geodesics, of circular cylinder 239 Geodesics, of plane 239 Geodesics, of Poincare half-plane 251 Gradient, on 72 Gradient, on surface 181 Graph 13 Graph, of 155 160 Graph, of 218 Graph, of 196 Graph, of 196 Graph, of 196 Graph, of 180 Greatest lower bound 9 Green’s formula 258 268 Group 47 Group, abelian 24 Group, affine 5 3
Реклама
with curve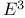 
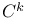
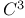function 
|
|
О проекте