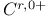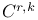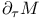Авторизация
Поиск по указателям
Candel A., Conlon L. — Foliations I
Обсудите книгу на научном форуме Нашли опечатку?
Название: Foliations IАвторы: Candel A., Conlon L. Аннотация: This is the first of two volumes on the qualitative theory of foliations. This volume is divided into three parts. It is extensively illustrated throughout and provides a large number of examples. Part 1 is intended as a "primer" in foliation theory. A working knowledge of manifold theory and topology is a prerequisite. Fundamental definitions and theorems are explained to prepare the reader for further exploration of the topic. This section places considerable emphasis on the construction of examples, which are accompanied by many illustrations.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1999Количество страниц: 398Добавлена в каталог: 21.11.2013Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
19 276 19 276 232 279 192 287 237 189 189 189 189 158 127 19 15 240 231 239—245 233 247 232 231 126 240 46 157 20 20 159 149 311 240 (n, 351 (n, 352 (n, 356 (n, 348 (n, 349 (R, 360 2-holed torus 17 2nd countable 24 A(L) 145 Accessible set 145 Action of a Lie group 284 Adjoint 237 Ahlfors, L. 343 Alexander horned sphere 111 Almost periodic function 285 Annihilator of a distribution 36 Annihilator, ideal 34 36 Architecture (of foliations) 166 ARM 131 Arnol'd, V.I. 218 Asymptote of a leaf 115 Asymptote of an end 115 Asymptotic 103 115 116 Atlas(es), foliated 23—33 275—281 Atlas(es), foliated, associated to a foliation 21 Atlas(es), foliated, class 23 278 Atlas(es), foliated, coherence of 25 278 Atlas(es), foliated, coherent regular refinement of 29 278 Atlas(es), foliated, regular 27 278 Atlas(es), laminated 235 Attie, O. 194 340 Averaging sequence 251—253 339 343 Averaging sequence, definition of 252 Averaging sequence, topological 331 Bebutov, M. 273 286 Biregular cover 123 125 Biregular foliated atlas 121 125 Blank, S. 225 Bohr, H. 275 Bonding map 281 Border 127 Border plaque 127 Border plaque, special 127 Boundary operator on currents 237 Boundary, tangential 20 22 Boundary, transverse 20 22 Bounded linear map on 233 Bounded subset of 237 Bounded subset of 233 Bundle, flat 54 Bundle, foliated 46—55 Bundle, structure cocycle for 54 Bundle, structure group of 54 Bundle, structure group of, reduction of 54 Bundle-like metric 362 C(M) 279 Candel, A. 342 Cantor set 18 104 108 275 276 283 291 Cantor set of ends 111 Cantwell, J. 142 165 171 Category 62 Category, morphism of 62 Category, object of 62 Chart(s), coherently foliated 23 25 276 Chart(s), foliated 19 276 Chart(s), foliated, codimension of 19 Chart(s), foliated, transversal of 20 276 Circle at infinity 12 104 Cobordism (of foliations) 100 Cocycle conditions 28 280 Cocycle holonomy 28 280 Codimension of foliated chart 19 Codimension of foliation 6 21 Codimension, arbitrary 229—386 Codimension, one 121—225 Coherence of foliated atlases 25 278 Coherence of invariant measures 302 Collar 82 Collar, foliated 82 Completely integrable system 34 Completeness 50 Compressible 307 Concordance (of foliations) 99 Conforma, metrics, class of 342 Conformal metrics 294 341 Conformal Riemann surfaces 294 Conlon, L. 165 171 Connes, A. 345 Contraction, linear 183 Contraction, Sacksteder 183 Convolution 288 Corner 19 Critical point 152 Critical point, nondegenerate 152 Cross section 46 49 Cross section, local 286 Current 231 Current of degree 0 236 Current of integration 234 Current, closed 237 Current, de Rham 229 Current, diffuse 233 Current, Dirac 233 Current, exact 237 Current, foliation 231 239—245 Current, foliation cycle 235 240 Current, foliation cycle as a holonomy-invariant measure 245—251 Current, foliation, Dirac 239 Current, holonomy-invariant measure for a foliation 234 Current, holonomy-invariant measure for a lamination 235 Current, p- 233 Current, p-boundary 237 Current, p-cycle 237 Current, singular 233 Current, singular submanifold 234 Cut and paste 3 Cycle foliation 229 231 235 240 Cycle foliation as a holonomy-invariant measure 245—251 Cycle foliation in foliated spaces 303 Cycle limit 158 Cycle, asymptotic 253 Cylindrical coordinates 90 de Rham cohomology in foliated spaces 303 De Rham current 229 de Rham homology 237 de Rham homology of foliated spaces 304 de Rham theorem 238 Deadend component 267 Deadend component, generalized 268 Deformation of a foliation 17 95—101 Denjoy's example 107—108 205 Denjoy's foliation 166 184 Denjoy's theorem 166 217 Denjoy, A. 108 166 184 Depth 192 Derived set 114 Diagonal action 76 Diffeomorphism in foliated spaces 277 Differentiability class of a foliated atlas 21 278 Differentiability class of a foliated manifold 21 Differentiability class of a foliated space 279 Differentiability class of a foliation 21 Differential graded ideal 37 Dippolito semistability theorem 134 Dippolito, P. 134 Dirac current 233 Dirac foliation current 239 Distance in a 320 Distance, leafwise 299 Distance, leafwise, Riemannian 299 distribution 34 Distribution, completely integrable 36 Distribution, involutive 36 Distribution, k-plane 34 36 Dominate 311 Dominate, strictly 312 Double along 126 Duminy's theorem on ends 118 184 199 Duminy's vanishing theorem 66 184 193 208 218 325 372 379 Duminy, G. 66 118 165 184 193 199 372 379 End(s) 90 110—118 End(s) of leaves 115—118 End(s) of manifolds 110—115 End(s), Cantor set of 111 372 End(s), definition of 111 End(s), fundamental neighborhood system of 112 End(s), periodic 198 End(s), proper 116 End(s), totally proper 116 entropy 229 347—386 Entropy of a flow 352 Entropy of a foliation 356—358 Entropy of a foliation, relative to a foliated atlas 356—357 Entropy of a map 348—351 Entropy of a pseudogroup 352—355 Entropy of a transformation group 351—352 Entropy, geometric of a foliated manifold 358—368 Entropy, geometric of a foliated space 368—370 Entropy, geometric, definition for foliated manifolds 360 Epstein, D.B.A. 65 Ergodic flow-invariant measure 253 Ergodic theorem 254 Escher, M.C. 86 Euclidean half space 15 Euler characteristic of a foliated space 342 Euler characteristic, average 331 Euler characteristic, average, nonzero 331 Euler characteristic, average, zero 331 Euler class 147 340 Exotic characteristic class 38 Expanding map 284 296 373 Expansion of a regular foliated atlas 280 Exploding ball 258 Exploding disk 258 Exploding plateau 258 Fiber bundle 5 Fiber bundle, base of 5 Fiber bundle, fiber of 5 Fiber bundle, foliated 46—55 Fiber bundle, total space of 5 Finite depth 197—204 First return map 45 First return time 47 Flow on a metric space 273 285 352 Flow, entropy of 352 Flow, local 8 Flow, Sacksteder 214—217 Flowbox 337 Flowline 8 Foelner condition 252 306 326 Foliated atlas 23 275—281 Foliated atlas, class 23 278 Foliated atlas, coherent regular refinement of 29 278 Foliated atlas, regular 27 278 Foliated bundle 46—55 283 Foliated bundle, incomplete 49 Foliated chart 19 276 Foliated chart, transversal of 20 276 Foliated manifold 5—23 Foliated manifold, definition of 21 Foliated manifold, differentiability class of 21 Foliated manifold, geometrically taut 261 Foliated manifold, harmonic 261 Foliated normal neighborhood 63 Foliated product 126 Foliated space 32 273—307 Foliated space, orientable 279 Foliated space, transverse model of 275 Foliation(s) as a coherence class of foliated atlases 31 279 Foliation(s) as a maximal foliated atlas 31 279 Foliation(s) as an equivalence relation 22 279 Foliation(s) of class 31 Foliation(s) of class 32 Foliation(s) of class 32 Foliation(s) of finite depth 197 Foliation(s) of manifolds with boundary 15 Foliation(s) without holonomy 205 Foliation(s), analytic 162 Foliation(s), boundary 240 Foliation(s), cobordant 100 Foliation(s), codimension of 6 21 Foliation(s), concordant 99 Foliation(s), current 231 Foliation(s), cycle 229 231 235 240 Foliation(s), cycle in foliated spaces 303 Foliation(s), defined by a closed, nonsingular 1-form 40 Foliation(s), definition of 20—21 279 Foliation(s), differentiability class of 21 279 Foliation(s), dimension of 6 21 279 Foliation(s), entropy 229 Foliation(s), geometrically taut 261 Foliation(s), harmonic 261 Foliation(s), homologically taut 266 Foliation(s), homotopic 98 Foliation(s), induced on 31 Foliation(s), induced on 31 Foliation(s), infinitesimally trivial at the boundary 91 Foliation(s), integrably homotopic 96 Foliation(s), integral to a 32 123—126 Foliation(s), integral to a 32 Foliation(s), leafwise orientable 89 Foliation(s), lifted by covering map 81 Foliation(s), minimal 103 Foliation(s), normal bundle of 28 Foliation(s), smooth leaved 31 Foliation(s), tangent to submanifold 15 Foliation(s), taut, arbitrary codimension 261—272 Foliation(s), taut, codimension one 146 Foliation(s), taut, codimension-one 257—261 Foliation(s), transverse G-structure of 33 Foliation(s), transverse to submanifold 15
Реклама
 |
|
О проекте
|
|
О проекте



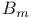
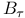
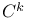 norm
norm 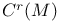 (for foliated spaces)
(for foliated spaces) 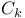
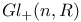
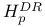
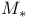
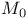
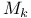
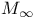
 -limit set
-limit set 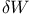
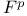
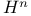
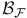
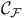
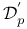
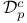
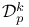
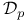
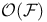

 -limit set
-limit set 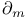

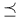
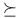
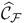
 )-separation by
)-separation by 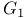

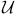
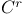
 -orbit
-orbit