Авторизация
Поиск по указателям
Candel A., Conlon L. — Foliations I
Обсудите книгу на научном форуме Нашли опечатку?
Название: Foliations IАвторы: Candel A., Conlon L. Аннотация: This is the first of two volumes on the qualitative theory of foliations. This volume is divided into three parts. It is extensively illustrated throughout and provides a large number of examples. Part 1 is intended as a "primer" in foliation theory. A working knowledge of manifold theory and topology is a prerequisite. Fundamental definitions and theorems are explained to prepare the reader for further exploration of the topic. This section places considerable emphasis on the construction of examples, which are accompanied by many illustrations.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1999Количество страниц: 398Добавлена в каталог: 21.11.2013Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Foliation(s), transversely affine 42 373 Foliation(s), transversely homogeneous 34 Foliation(s), transversely Lie 34 Foliation(s), transversely orientable 28 Foliation(s), transversely Riemannian 33 Form on a foliated space 301 Form, closed, defining foliation 24 Form, closed, group of periods of 42 Form, closed, periods of 24 41 Form, defining foliation 24 Form, distributional 231 Form, transverse to a foliation 240 265 Frobenius theorem 34—43 Frobenius Theorem, statement of 37 Frobenius theorem, vector field version 36 Fundamental domain 72 G-orbit 18 282 285 G-orbit, global 286 G-orbit, local 286 Gabai, D. 197 Gauss — Bonnet theorem 148 331 General position 151—164 General position, definition of 152 Geodesic spray 88 Geodesic spray, leafwise 89 Germ(s) of a foliation 63 Germ(s) of a local homeomorphism 60 Germ(s), isomorphic (of foliations) 63 Germinal holonomy 59—67 Ghys, E. 194 229 345 358 360 374 Gluck, H. 266 Glue 18 Gluing, tangential 90 Gluing, transverse 81 Godbillon — Vey class 38 99 192 Godbillon — Vey class of Hirsch foliation 373 Godbillon — Vey class, generalized 40 Godbillon — Vey class, invariance, under concordance 101 Godbillon — Vey class, invariance, under integrable homotopy 101 Godbillon — Vey class, invariance, under isotopy 101 Godbillon — Vey class, invariance, under turbulization 100 Godbillon — Vey class, naturality of 81 Godbillon — Vey class, nonvanishing of 347—348 Godbillon — Vey class, not a homotopy invariant 99 Godbillon — Vey number 101 Godbillon — Vey number, continuous variation of 101 Godbillon — Vey number, invariance under cobordism 101 Goodman, S. 147 252 gr(G) 312 Graph 157 Graph, closed 157 Group of periods 219 Group, Archimedean ordered 213 Group, Archimedean ordered, Hoelder's theorem 213 Group, general linear 288—290 Group, Lie, action of 284 Group, Lie, foliation by cosets 10 37 Group, Lie, locally free action of 285 Group, ordered 212 Group, special linear 11 Groupoid 59 62 Growth function 311 Growth type 311 Growth type of a covering space 318 319 Growth type of a function 312 Growth type of a group 317 Growth type of a group orbit 319 Growth type of a leaf 313 Growth type of a manifold 312 Growth type of a pseudogroup orbit 320 Growth type, exactly polynomial 313 Growth type, exponential 314 Growth type, fractional 316 Growth type, linear 313 Growth type, nonexponential 315 Growth type, quadratic 313 Growth type, quasi-polynomial 315 Growth type, subexponential 315 Growth type, weakly polynomial 316 gv( 38 81 Haar measure 287 Haefliger structure 99 Haefliger, A. 137 151 162 189 Hahn — Banach theorem 241 Halmos, P. 306 Hausdorff metric (or distance) 362 Hausdorff — Banach — Tarski paradox 306 Hector, G. 65 165 171 Herman, M. 218 hessian 152 Hilbert space, lamination of 299 Hirsch foliation, construction 371 Hirsch foliation, entropy of 373 Hirsch foliation, Godbillon — Vey class of 373 Hirsch, M.W. 370 Holonomy 45—67 Holonomy cocycle 28 280 Holonomy cocycle for a foliated bundle 50 Holonomy germ 59 Holonomy germ, an invariant of homotopy 60 Holonomy group 281 Holonomy group of a leaf 62 Holonomy group, germinal (of a leaf) 46 Holonomy group, infinitesimal (of a leaf) 46 66 Holonomy group, total 213 Holonomy group, total (of a foliated bundle) 45—55 Holonomy groupoid 46 62 Holonomy homomorphism(s) of a leaf 63 280 Holonomy homomorphism(s), conjugate 64 Holonomy homomorphism(s), infinitesimal (of a leaf) 66 Holonomy homomorphism(s), total (of a foliated bundle) 48 Holonomy pseudogroup 55—59 280 Holonomy pseudogroup of a foliated manifold 46 Holonomy pseudogroup of a foliated space 280 Holonomy pseudogroup of a foliation 59 Holonomy pseudogroup of a regular foliated atlas 59 280 Holonomy transformation 58 Holonomy transformation, total (of a foliated bundle) 51 Holonomy, fixed point of 133—134 Holonomy, germinal 59—67 Holonomy, trivial of a leaf 65 Holonomy, trivial of an open, saturated set 205—208 Holonomy, unbounded 173 Homology 3-sphere 148 Homology class defined by a compact leaf 138 245 Homology direction 254 Homology intersection 138 Homotopy of foliations 98 Homotopy, integrable (of foliations) 96 Homotopy, piecewise 98 Hopf fibration 268 Hopf, E. 306 Hurder, S. 194 340 348 Hyperbolic plane 11 Hyperbolic plane, a leaf at infinite level 194 Hyperbolic plane, Poincare disk model 104 Hyperbolic surface 294 Imanishi, H. 209 Inaba, T. 194 Incomplete foliated bundle 49 Infinite jail cell window 311 Infinite jungle gym 311 Infinite Loch Ness monster 111 311 332 Infinite repetition 181 198 Infinitesimally 91 Infinitesimally trivial (at boundary) 91 Integrability condition 36 Integral manifold 36 Inverse limit 276 281 Inverse system 281 Isotopy of foliations 95—96 Isotopy of imbeddings 152 Isotopy of immersions 152 Isotopy, pull-back 96 Isotopy, push-forward 96 Isotropy group 284 Jacob's Ladder 311 332 Januszkiewicz, T. 340 Jessen, B. 275 Jouanolou, J.P. 142 Juncture 179 Juncture of spiral 197 Juncture, compact 180 Juncture, noncompact 181 Kakutani, S. 286 Kellum, M. 354 Key lemma 170 Kopell Lemma 166 Kopell Lemma, generalized 177—178 Kopell Lemma, proof 178 Kopell, N. 166 Kronecker's theorem 9 Lamination 235 273 Lamination in Hilbert space 273 Lamination, abstract 32 273 Langevin, R. 229 358 360 374 Laudenbach, F. 225 Leaf 5 279 Leaf space 6 Leaf, closed 103 Leaf, closed at infinity 330 Leaf, compact 137 Leaf, finite depth 197—204 Leaf, generic 65 Leaf, proper 115 133—135 Leaf, resilient 184 323 379 Leaf, semiproper 118 133—135 Leaf, semistable 134 Leaf, totally proper 192 Leafwise distance 299 Leaves, 2-dimensional 331—345 Leaves, border 107 Length of a plaque chain 29 Level set 6 Level(s), 189 Level(s), 0 189 Level(s), filtration 189 Level(s), finite 189 Level(s), infinite 189 Level(s), theory of 166 187—196 380 Lie foliation 34 Lie group 10 Lie group, actions of 284—290 Lie group, foliation by cosets 10 37 Limit cycle 158 Limit set 115—118 Limit set of a leaf 115 Limit set of an end (e-limit set) 115 Limit set, 158 Limit set, 46 157 Linear foliation of the 2-torus 9 24 Linear fractional transformation 11 Lipschitz foliated chart 369 Lipschitz foliated space 369 Lipschitz triple 369 Local flow 8 Local minimal set 166 171—177 Local minimal set, definition of 176 Local minimal set, exceptional 176 Local minimal set, single leaf 176 Long line 8 Map, first return 46 Map, first return of a flow 46 Map, transverse to a foliation 80 Mapping cylinder 48 Markov chain (topological) 107 Markov minimal set 107 Mean curvature 262 Measure, absolutely continuous 218 Measure, continuous 214 Measure, holonomy-invariant 213—214 301—307 325—329 373—378 Measure, holonomy-invariant as a foliation current 234—235 Measure, holonomy-invariant as a foliation cycle 236 245—251 Measure, holonomy-invariant for laminations 235—236 Measure, holonomy-invariant, coherent 302 Measure, holonomy-invariant, definition of 234 302 Measure, support of 214 302 Measure, transverse (holonomy) invariant 215 302 Millet, K. 65 Milnor, J. 138 Minimal set 103 Minimal set with locally dense leaves 103 Minimal set, exceptional 104 Minimal set, local 166 171—177 Minimal set, local, definition of 176 Minimal set, Markov 107 199 Montel space 237 Morita, S. 218 Morse function 152 Morse index of a critical point 152 Morse lemma 151 152 Morse singularity 151 152 Morse, M. 151 Moser, J. 98 Moussu, R. 165 Nadkarmi, M. 307 Nishimori, T. 165 194 Nonstandard analysis 142 Norm on a finitely generated group 316 Norm, 232 Norm, uniform 375 Normal bundle 61 Normal fence 209 Normal fence, closed 211 Normal neighborhood (foliated) 63 Novikov order 149 Novikov, S.P. 87 209 258 Nucleus 131 Octopus decomposition 130 131 Open book decomposition 268 Orbit 18 284 Orbit, long, almost closed 254 Orientable foliated space 279 Orientable leafwise 89 Orientable, transversely 28 Orientation cover 94 Overdetermined system 34 P( 219 Pair of pants 161 202 371 Paradoxical decomposition 306 Paradoxical pseudogroup 307 Pelletier, F. 165 Penrose tilings 291 Periodic end 198 Perturbation 68 Perturbation, k-parameter 69 Phillips, A. 331 Pixton, D. 167 Plante, J.F. 229 252 306 325 329 Plaque 20 276 Plaque chain 29 57 278 Plaque chain, covering a path 60 Plaque chain, length of 278 Plaque loop 51 Plateau, exploding 259 Plateau, moving 258 Poincare disk 104 Poincare duality 138 193 201 Poincare recurrence set 329 Poincare rotation number 217
Реклама
) 
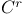(of foliations) 
- 
- 
) 
|
|
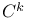
О проекте