|
|
 |
| јвторизаци€ |
|
|
 |
| ѕоиск по указател€м |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Luke Y.L. Ч Special Functions and Their Approximations. Volume II |
|
|
 |
| ѕредметный указатель |
 -function see "Hypergeometric function (confluent)" -function see "Hypergeometric function (confluent)"
 -Method II 66Ч91 -Method II 66Ч91
Airy functions I 205 217 see "Approximation" "Expansion"
Anger Ч Weber functions I 218 II 39 54 55
Approximation II 287Ч291
Approximation, Airy functions I 217 see
Approximation, ber, bei I 216 see
Approximation, Bessel functions, 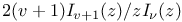 , RFP II 82 83 , RFP II 82 83
Approximation, Bessel functions, 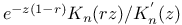 , RF II 259Ч265 , RF II 259Ч265
Approximation, Bessel functions, $H_{\nu}^{(1)}(x), BCRF II 290 291
Approximation, Bessel functions, 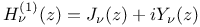 II 229Ч232 436Ч448 II 229Ч232 436Ч448
Approximation, Bessel functions, 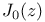 , by approximate inversion of its Laplace transform II 265 , by approximate inversion of its Laplace transform II 265
Approximation, Bessel functions, 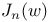 , , 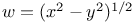 , TR II 220 , TR II 220
Approximation, Bessel functions, 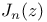 , TR II 218 219 265 , TR II 218 219 265
Approximation, Bessel functions, 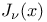 , , 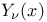 , , 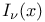 , , 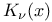 , BCP II 290 291 , BCP II 290 291
Approximation, Bessel functions, 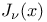 , , 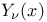 , , 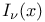 , , 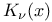 , R II 162 165 , R II 162 165
Approximation, Bessel functions, 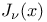 , , 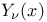 , zeros of, RF II 228Ч233 , zeros of, RF II 228Ч233
Approximation, Bessel functions,  , zeros of, RF II 228Ч233 259 264 , zeros of, RF II 228Ч233 259 264
Approximation, Bessel functions, 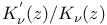 , RFP II 83 84 , RFP II 83 84
Approximation, Bessel functions, 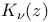 , P II 111Ч116 , P II 111Ч116
Approximation, Bessel functions, 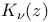 , RF II 111Ч116 229 230 436Ч448 , RF II 111Ч116 229 230 436Ч448
Approximation, Bessel functions, 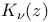 , TR II 221Ч225 , TR II 221Ч225
Approximation, Bessel functions, Airy functions I 217
Approximation, Bessel functions, ber, bei, ker, kei, and derivatives, BCP II 291 I 216
Approximation, best in sense of, Chebyshev I 303Ч305 II 68
Approximation, best in sense of, least square I 270
Approximation, best in sense of, mean square I 268 305
Approximation, best in sense of, other II 72 73
Approximation, beta function (incomplete), P, RF, RFP II 178
Approximation, binomial function, 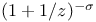 }$, RFP II 179 }$, RFP II 179
Approximation, binomial function, 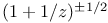 }$, Newton Ч Raphson process II 183 184 }$, Newton Ч Raphson process II 183 184
Approximation, binomial function, 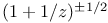 }$, Newton Ч Raphson process, P II 387 388 }$, Newton Ч Raphson process, P II 387 388
Approximation, binomial function, 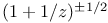 }$, Newton Ч Raphson process, RFP II 182Ч185 269 395 }$, Newton Ч Raphson process, RFP II 182Ч185 269 395
Approximation, binomial function, 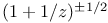 }$, Newton Ч Raphson process, sum of partial fractions II 183 269 }$, Newton Ч Raphson process, sum of partial fractions II 183 269
Approximation, binomial function, 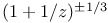 , RFP II 397 , RFP II 397
Approximation, binomial function, 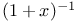 , BCP II 288 , BCP II 288
Approximation, binomial function, 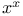 , , 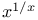 , RF II 180 181 , RF II 180 181
Approximation, binomial function, 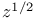 , RFP II 185 396 , RFP II 185 396
Approximation, binomial function, |x|, BCP II 288
Approximation, binomial function, |x|, RFP II 185 396
Approximation, cosine see "Approximation sine
Approximation, cosine integral see "Approximation sine
Approximation, Debye functions, RFP II 273Ч281
Approximation, derivatives, repeated, R II 165
Approximation, differential equation, solution of, first order Riccati, RFP II 77Ч86
Approximation, differential equation, solution of, generalized second order Riccati, RFP II 86Ч91
Approximation, differential equations, integral equations, and other functional equations, solution of, collocation, or selected points II 69
Approximation, differential equations, integral equations, and other functional equations, solution of, P II 66Ч69
Approximation, differential equations, integral equations, and other functional equations, solution of, RF II 66Ч69 256Ч269
Approximation, Duffing's equation, RFP II 89 90
Approximation, economized II 66 105 106 111
Approximation, elliptic functions (Jacobian), sn(x,k), cn(x,k), RFP II 90 91
Approximation, elliptic integrals, first two kinds, complete, BCP II 291
Approximation, elliptic integrals, three kinds, complete and incomplete, RFP II 85 86 269Ч273
Approximation, elliptic integrals, three kinds, complete, TR II 220 221
Approximation, error functions, 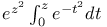 , zeros of, RFP II 227 228 , zeros of, RFP II 227 228
Approximation, error functions, 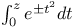 , RFP II 422Ч429 , RFP II 422Ч429
Approximation, error functions,  , P and RF II 106 107 , P and RF II 106 107
Approximation, error functions,  , RFP II 206 207 213 422 423 430Ч435 , RFP II 206 207 213 422 423 430Ч435
Approximation, error functions,  , TR II 225 226 , TR II 225 226
Approximation, error functions, repeated integrals, R II 165
Approximation, exponential function, 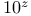 , BCP II 288 , BCP II 288
Approximation, exponential function,  , BCP II 288 , BCP II 288
Approximation, exponential function,  , P and RF II 69Ч73 , P and RF II 69Ч73
Approximation, exponential function,  , RFP II 73Ч75 83 191Ч193 256 399 , RFP II 73Ч75 83 191Ч193 256 399
Approximation, exponential function,  , RFP II 273 274 , RFP II 273 274
Approximation, exponential integral, 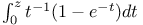 , P and RF II 196Ч198 403 411Ч414 , P and RF II 196Ч198 403 411Ч414
Approximation, exponential integral, 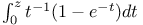 , RFP II 197 402 405Ч408 , RFP II 197 402 405Ч408
Approximation, exponential integral,  , P and RF II 106 107 , P and RF II 106 107
Approximation, exponential integral,  , RFP II 206 207 212 213 403 404 415Ч421 , RFP II 206 207 212 213 403 404 415Ч421
Approximation, exponential integral, ratio involving, RFP II 265Ч267
Approximation, exponential integral, repeated integrals, R II 165
Approximation, Fermi Ч Dirac integrals, BCP and BCRF II 291
Approximation, Fresnel integrals, P II 290
Approximation, Fresnel integrals, RFP II 207 422Ч435
Approximation, Fresnel integrals, TR II 226
Approximation, G-function, P and RF II 118Ч132
Approximation, gamma function see also "Approximation incomplete
Approximation, gamma function,  , BCRF II 288 , BCRF II 288
Approximation, gamma function, 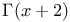 , BCP II 287 , BCP II 287
Approximation, gamma function, logarithmic derivative, 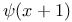 , BCP II 287 , BCP II 287
Approximation, hypergeometric function, confluent, the  -function, -function, 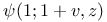 see "Approximation incomplete z)$"/>" see "Approximation incomplete z)$"/>"
Approximation, hypergeometric function, confluent, the  -function, -function, 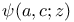 , P and RF II 111Ч116 , P and RF II 111Ч116
Approximation, hypergeometric function, confluent, the  -function, -function, 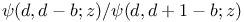 , RFP II 202 , RFP II 202
Approximation, hypergeometric function, confluent, the  , , 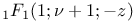 see "Approximation incomplete z)$"/>" see "Approximation incomplete z)$"/>"
Approximation, hypergeometric function, confluent, the  , u'(z)/u(z), , u'(z)/u(z), 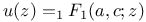 , RFP II 80Ч83 196 , RFP II 80Ч83 196
Approximation, hypergeometric function, Gaussian, the  , , 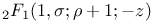 II 167Ч185 II 167Ч185
Approximation, hypergeometric function, Gaussian, the  , , 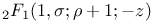 , P and RF II 167Ч169 175Ч176 , P and RF II 167Ч169 175Ч176
Approximation, hypergeometric function, Gaussian, the  , , 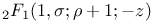 , RFP II 84 169Ч173 176Ч178 , RFP II 84 169Ч173 176Ч178
Approximation, hypergeometric function, Gaussian, the  , u'(z)/u(z), , u'(z)/u(z), 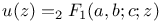 , RFP II 173Ч175 , RFP II 173Ч175
Approximation, hypergeometric function, generalized, the  , P and RF II 92Ч132 150 , P and RF II 92Ч132 150
Approximation, incomplete gamma function II 186Ч213 see sine "Approximation error "Approximation exponential "Approximation Fresnel "Approximation hypergeometric "Approximation confluent"
Approximation, incomplete gamma function, 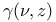 , P and RF II 186Ч188 , P and RF II 186Ч188
Approximation, incomplete gamma function, 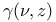 , RFP II 188Ч194 , RFP II 188Ч194
Approximation, integrals by use of orthogonal polynomials II 243Ч245
Approximation, integrals, Simpson's rule and other Newton Ч Cotes formulas II 218
Approximation, integrals, TR II 214Ч226 see Laplace "Approximation sine
Approximation, ker, kei I 216 see
Approximation, Laplace integral or transform, numerical integration II 245
Approximation, Laplace integral or transform, P and RF II 106Ч118
Approximation, Laplace transform, inversion of, numerical integration II 251Ч255
Approximation, Laplace transform, inversion of, orthogonal polynomials II 249Ч251
Approximation, Laplace transform, inversion of, RF II 255Ч269
Approximation, Laplace transform, inversion of, TR II 225
Approximation, Legendre functions, R II 165
Approximation, logarithm, 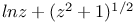 , RFP II 393 394 , RFP II 393 394
Approximation, logarithm, ln(l+z), BCP II 288
Approximation, logarithm, ln(l+z), P II 385 386
Approximation, logarithm, ln(l+z), RFP II 389 390
Approximation, logarithm, ln[(1+z)/(1-z)], RFP II 391 392
Approximation, Lommel functions, P and RF II 116Ч118
Approximation, Mellin Ч Barnes integral, TR II 225
Approximation, Painleve's first transcendent, RFP II 89
Approximation, Power series or ratio of two power series, RFP II 76
Approximation, psi( )-function or logarithmic derivative of the gamma function see "Approximation gamma )-function or logarithmic derivative of the gamma function see "Approximation gamma
Approximation, Riccati differential equations, solutions of, RFP II 77Ч91
Approximation, sine and cosine, integrals, 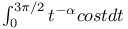 , zeros of, RFP II 228 , zeros of, RFP II 228
Approximation, sine and cosine, integrals,  , ,  , RF II 196Ч198 402Ч408 415Ч421 , RF II 196Ч198 402Ч408 415Ч421
Approximation, sine and cosine, integrals,  , ,  , RFP II 197 403 404 409 410 , RFP II 197 403 404 409 410
Approximation, sine and cosine, integrals, 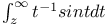 , , 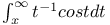 , RF II 207 403 404 415Ч421 , RF II 207 403 404 415Ч421
Approximation, sine and cosine, inverse, arc sin z, RFP II 393 394
Approximation, sine and cosine, sin z, cos z, BCP II 288
Approximation, sine and cosine, sin z, cos z, RF II 74 193 399Ч401
Approximation, Struve functions, P and RF II 116Ч118
Approximation, Struve functions, RF II 449Ч452
Approximation, tangent, inverse, arc tan z, BCP II 288
Approximation, tangent, inverse, arc tan z, P II 385 386
Approximation, tangent, inverse, arc tan z, RFP II 391 392
Approximation, tangent, tan z, BCP II 288
Approximation, tangent, tan z, RF II 399Ч401
Approximation, tangent, tan z, RFP II 83
Approximation, Whittaker function see "Hypergeometric function confluent"
Associated Bessel function I 219
Asymptotic expansion I 1Ч7 see asymptotic"
Asymptotic expansion, elementary properties I 3 4
Asymptotic expansion, expansion definition I 2
Asymptotic expansion, Watson's lemma I 4Ч7
Basic series I 291 II 283
bcp see "Best polynomial in the Chebyshev sense"
BCRF see "Best rational function in the Chebyshev sense"
Ber, bei functions I 216 II 291 347Ч351 see "Approximation" "Expansion"
Bernoulli and generalized Bernoulli polynomials, definition and elementary properties I 18Ч22
Bernoulli and generalized Bernoulli polynomials, Fourier series for 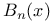 I 23 I 23
Bernoulli and generalized Bernoulli polynomials, integrals of I 22 23
Bernoulli and generalized Bernoulli polynomials, recurrence formulas I 20 22 35
Bernoulli and generalized Bernoulli polynomials, table of 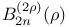 I 34 I 34
Bernoulli and generalized Bernoulli polynomials, table of 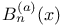 I 19 I 19
Bernoulli and generalized Bernoulli polynomials, table of 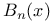 I 20 I 20
Bernoulli numbers, definition I 19 20
Bernoulli numbers, expansion of cot z, tan z, csc z, ln cos z in series involving I 23
| Bernoulli numbers, table of I 20
Bessel functions, Airy functions, connection with I 217
Bessel functions, approximation see "Approximation"
Bessel functions, associated I 219
Bessel functions, asymptotic expansions I 203Ч205 215
Bessel functions, ber, bei functions, connection with I 216
Bessel functions, computation by use of recurrence formulas II 162
Bessel functions, confluent hypergeometric function, connection with I 120 135 213
Bessel functions, definitions, connecting relations, power series I 39 40 212 213
Bessel functions, derivatives with respect to order II 52 53
Bessel functions, derivatives with respect to order at half an odd integer I 216
Bessel functions, difference-differential properties I 48 214 II 232
Bessel functions, expansions, expansions in series of see "Expansion"
Bessel functions, G-functions, connection with I 226Ч234
Bessel functions, integrals involving I 115 165 177 219 220 287 289 II 33 34 39 53 54 see
Bessel functions, Jacobi polynomial, connection with I 52
Bessel functions, ker, kei functions, connection with I 216
Bessel functions, products I 216 228Ч230 232Ч234 II 50Ч53
Bessel functions, Wronskians I 214 215
Bessel functions, zeros of I 205 II 228Ч234
Bessel polynomials I 274 II 194 253
Best approximation see "Approximation"
Beta function, complete I 15 16 18
Beta function, incomplete I 210 II 178
Beta transform I 58 59 170Ч176 286
BF see "Bessel function or
Binomial coefficient I 9 35
Binomial function I 38 40 49 209 210 II 179Ч185 see binomial "Expansion powers
Catalan's constant II 293
Chebyshev polynomials of the first kind I 308Ч312 see "Approximation"
Chebyshev polynomials of the first kind, connection with Pade approximation for square root II 183
Chebyshev polynomials of the first kind, definition and basic properties I 273 296 297 300 301
Chebyshev polynomials of the first kind, difference-differential properties I 297Ч299 301 302 316 321 324
Chebyshev polynomials of the first kind, evaluation of series of, by use of backward recurrence formula I 325Ч329
Chebyshev polynomials of the first kind, expansions in series of (based on orthogonality property with respect to integration) see also "Expansion"
Chebyshev polynomials of the first kind, expansions in series of (based on orthogonality property with respect to integration), asymptotic estimate of coefficients I 293Ч296
Chebyshev polynomials of the first kind, expansions in series of (based on orthogonality property with respect to integration), evaluation of coefficients I 286Ч293
Chebyshev polynomials of the first kind, expansions in series of, differential and integral properties I 314Ч325
Chebyshev polynomials of the first kind, expressed in powers of x II 294 296 297
Chebyshev polynomials of the first kind, integrals involving I 293Ч295 299 300Ч302 316Ч325
Chebyshev polynomials of the first kind, Jacobi polynomial, connection with I 273 296
Chebyshev polynomials of the first kind, minimax and mean square properties I 303Ч307
Chebyshev polynomials of the first kind, orthogonality property with respect to integration I 273 299 301
Chebyshev polynomials of the first kind, orthogonality property with respect to summation I 307 310 311
Chebyshev polynomials of the first kind, powers of x expressed in terms of II 295 298
Chebyshev polynomials of the first kind, solution of differential and integral equations by expansions in series of II 66Ч69 234Ч241
Chebyshev polynomials of the second kind I 312Ч314 see
Chebyshev polynomials of the second kind, connection with Pade approximation for square root II 183
Chebyshev polynomials of the second kind, definition and basic properties I 273 296Ч300
Chebyshev polynomials of the second kind, integrals involving I 299 300
Chebyshev polynomials of the second kind, Jacobi polynomial, connection with I 273 296
Chebyshev polynomials of the second kind, orthogonality property with respect to integration I 273 299
Chebyshev polynomials of the second kind, orthogonality property with respect to summation I 312 313
Chebyshev, best approximation in sense of I 303
Christoffel Ч Darboux formulas I 272
Collocation II 69
Computation, by use of recurrence formulas I 317Ч319 325Ч329 II 26Ч28 159Ч166 282Ч285
Confluence principle and theorems I 48Ч57
Constants, mathematical, table of II 292 293
Continued fractions see "Approximation RFP"
Continued fractions, convergence II 80
Cosecant I 23 210
Cosine see "Sine and cosine"
Cosine integral see "Sine and cosine integrals"
Cotangent see "Tangent"
Coulomb wave functions I 135 212
cp see "Chebyshev polynomials of the first kind"
Cylinder function I 204 213
D operator I 24Ч26
Darboux, method of generating functions I 254Ч259
Debye functions II 273Ч281
Delta ( ) operator I 24Ч26 ) operator I 24Ч26
Difference equations (or recurrence formulas), use of in computation I 317Ч319 325Ч329 II 159Ч166 282Ч285
Differential, integral and functional equations see "Approximation" "Expansion"
Dilogarithm II 310
Dixon's theorem I 104
Duffing's equation II 89 90
E-function I 148
Economized approximation II 66 105 106 111
Elliptic functions II 90 91
Elliptic integrals I 211 II 91 see "Expansion"
Error functions I 135 223 224 II 195 201 see "Expansion"
Euler Ч Mascheroni constant,  I 9 II 293 I 9 II 293
Evaluation of expansions in series of functions where functions satisfy a linear finite difference equation I 325Ч329
expansion II 287Ч291
Expansion,  -function, CP II 25 28 238Ч240 -function, CP II 25 28 238Ч240
Expansion,  -function, G-functions II 25 -function, G-functions II 25
Expansion, Airy functions I 217 see
Expansion, Anger Ч Weber functions, BF II 39 54 55
Expansion, Anger Ч Weber functions, CP II 39
Expansion, ber, bei I 216 see
Expansion, Bessel functions, 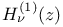 , ,  CP II 27 CP II 27
Expansion, Bessel functions, 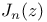 , , 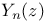 , , 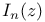 , , 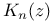 , BF II 37 49 50 52 53 , BF II 37 49 50 52 53
Expansion, Bessel functions, 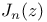 , , 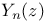 , , 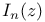 , , 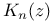 , CP II 37 38 290 331Ч333 338Ч341 347Ч351 , CP II 37 38 290 331Ч333 338Ч341 347Ч351
Expansion, Bessel functions, 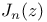 , , 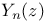 , , 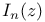 , , 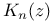 , GP II 38 , GP II 38
Expansion, Bessel functions, 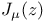 , , 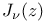 , BF II 50Ч53 , BF II 50Ч53
Expansion, Bessel functions, 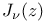 , , 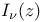 , BF II 49 50 , BF II 49 50
Expansion, Bessel functions, 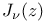 , , 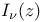 , CP II 35 37 234Ч238 290 352Ч356 359Ч367 , CP II 35 37 234Ч238 290 352Ч356 359Ч367
Expansion, Bessel functions, 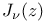 , , 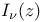 , GP II 37 , GP II 37
Expansion, Bessel functions, 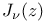 , , 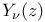 , zeros of, CP II 233 234 , zeros of, CP II 233 234
Expansion, Bessel functions, 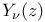 , , 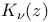 , BF II 49 , BF II 49
Expansion, Bessel functions, 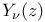 , , 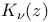 , CP II 240 290 352Ч356 359Ч367 , CP II 240 290 352Ч356 359Ч367
Expansion, Bessel functions,  , , 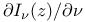 , BF II 52 53 , BF II 52 53
Expansion, Bessel functions, Airy functions I 217 see
Expansion, Bessel functions, ber, bei, ker, kei, and derivatives, CP II 347Ч351 I 216
Expansion, Bessel functions, integrals involving, 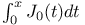 , $\int^{x}_{0} t^{-1}[1-J_{0}(t)] dt, BF II 39 54 , $\int^{x}_{0} t^{-1}[1-J_{0}(t)] dt, BF II 39 54
Expansion, Bessel functions, integrals involving, 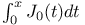 , $\int^{x}_{0} t^{-1}[1-J_{0}(t)] dt, CP II 39 334 , $\int^{x}_{0} t^{-1}[1-J_{0}(t)] dt, CP II 39 334
Expansion, Bessel functions, integrals involving, 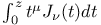 , ,  , BF II 33 34 53 54 , BF II 33 34 53 54
Expansion, Bessel functions, integrals involving, 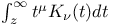 , CP II 240Ч242 , CP II 240Ч242
Expansion, Bessel functions, integrals involving, 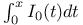 , , ![$\int_{0}^{x} t^{-1}[I_{0}(t)-1] dt$](/math_tex/489baad08d379dd32f5e03f1c8bf9c9082.gif) , , 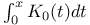 , , 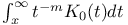 , m=0,1, CP II 242 342Ч345 , m=0,1, CP II 242 342Ч345
Expansion, Bessel functions, integrals involving, 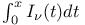 , , 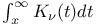 , ,  , CP II 368 369 , CP II 368 369
Expansion, Bessel functions, integrals involving, 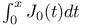 , ,  , , 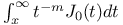 , , 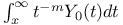 , m=0,1, CP II 334Ч336 , m=0,1, CP II 334Ч336
Expansion, Bessel functions, integrals involving, 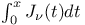 , , 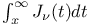 , ,  , CP II 357 358 , CP II 357 358
Expansion, Bessel functions, integrals involving, 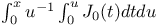 , , ![$\int_{0}^{x} u^{-1} \int_{0}^{u} t^{-1}[1-J_{0}(t)] dtdu$](/math_tex/7810fc2fc64b3f3cdd6bb90289c99ad582.gif) , CP II 337 , CP II 337
Expansion, Bessel functions, integrals involving, 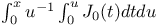 , , ![$\int_{0}^{x} u^{-1} \int_{0}^{u} t^{-1}[1-J_{0}(t)] dtdu$](/math_tex/7810fc2fc64b3f3cdd6bb90289c99ad582.gif) , CP, the same integrals with J replaced by I, CP II 346 , CP, the same integrals with J replaced by I, CP II 346
Expansion, binomial function see "Expansion powers
Expansion, Chebyshev polynomial of first kind, powers of x II 294 296 297
Expansion, Chebyshev polynomial of first kind, powers of x, derivatives and integrals of, CP I 297Ч299
Expansion, cosine see "Expansion sine
Expansion, cosine integral see "Expansion sine
Expansion, cotangent see "Expansion tangent"
Expansion, differential equations, integral equations, other functional equations, solutions of, CP II 234Ч242
Expansion, dilogarithm, 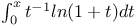 , CP II 310 , CP II 310
Expansion, elliptic integrals,  's II 63 64 's II 63 64
Expansion, elliptic integrals, first two kinds (complete and incomplete), sin 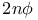 II 35 376Ч382 II 35 376Ч382
Expansion, elliptic integrals, first two kinds (complete), CP II 291 383 384
Expansion, elliptic integrals, Legendre polynomials II 36
Expansion, error functions, 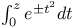 , BF II 42 43 57 58 , BF II 42 43 57 58
Expansion, error functions, 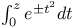 , CP II 42 43 57 289 290 323 324 , CP II 42 43 57 289 290 323 324
Expansion, error functions, 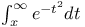 , CP II 289 290 324 , CP II 289 290 324
Expansion, error functions, repeated integrals, CP II 290
Expansion, exponential function, 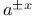 , , 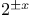 , , 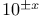 , BF II 32 , BF II 32
Expansion, exponential function, 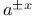 , , 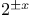 , , 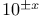 , CP II 32 315 , CP II 32 315
Expansion, exponential function, 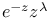 , BF II 45 , BF II 45
Expansion, exponential function, 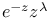 , JP I 285 , JP I 285
Expansion, exponential function,  , BF II 32 45 46 , BF II 32 45 46
Expansion, exponential function,  , CP II 32 288 313 314 363 , CP II 32 288 313 314 363
Expansion, exponential function,  , GP I 287 II 32 , GP I 287 II 32
Expansion, exponential function,  , JP I 285 , JP I 285
Expansion, exponential function, 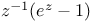 , BF II 33 34 , BF II 33 34
Expansion, exponential function, 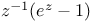 , CP II 34 , CP II 34
Expansion, exponential integral, 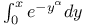 , , 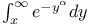 II 289 II 289
Expansion, exponential integral, 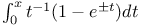 , BF II 41 56 , BF II 41 56
Expansion, exponential integral, 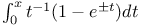 , CP II 41 289 321 , CP II 41 289 321
Expansion, exponential integral,  , P.V. , P.V. 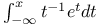 , CP II 289 322 , CP II 289 322
Expansion, Fresnel integrals, $\int_{0}^{x} t^{-1/2} e^{it} dt, BF II 43 58
Expansion, Fresnel integrals, $\int_{0}^{x} t^{-1/2} e^{it} dt, CP II 43 290 328
Expansion, Fresnel integrals,  , CP II 27 290 329 330 , CP II 27 290 329 330
Expansion, G-function,  's I 139 142 145Ч147 's I 139 142 145Ч147
Expansion, G-function, CP II 27 28
|
|
 |
| –еклама |
 |
|
|
 |
|
ќ проекте
|
|
ќ проекте



 -function
-function  -Method
-Method 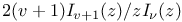 , RFP
, RFP 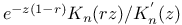 , RF
, RF 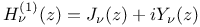
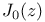 , by approximate inversion of its Laplace transform
, by approximate inversion of its Laplace transform 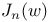 ,
, 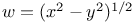 , TR
, TR 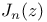 , TR
, TR 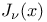 ,
, 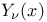 ,
, 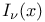 ,
, 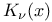 , BCP
, BCP  , zeros of, RF
, zeros of, RF 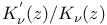 , RFP
, RFP 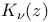 , P
, P 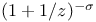 }$, RFP
}$, RFP 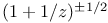 }$, Newton Ч Raphson process
}$, Newton Ч Raphson process 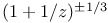 , RFP
, RFP 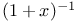 , BCP
, BCP 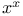 ,
, 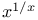 , RF
, RF 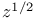 , RFP
, RFP 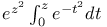 , zeros of, RFP
, zeros of, RFP 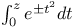 , RFP
, RFP  , P and RF
, P and RF 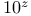 , BCP
, BCP  , BCP
, BCP  , RFP
, RFP 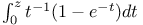 , P and RF
, P and RF  , P and RF
, P and RF  , BCRF
, BCRF 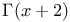 , BCP
, BCP 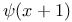 , BCP
, BCP 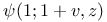
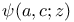 , P and RF
, P and RF 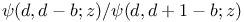 , RFP
, RFP  ,
, 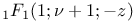
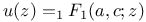 , RFP
, RFP  ,
, 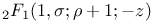
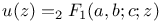 , RFP
, RFP  , P and RF
, P and RF 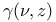 , P and RF
, P and RF 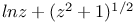 , RFP
, RFP 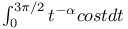 , zeros of, RFP
, zeros of, RFP  ,
,  , RF
, RF 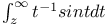 ,
, 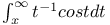 , RF
, RF 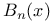
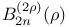
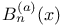
 ) operator
) operator 
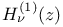 ,
,  CP
CP 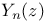 ,
, 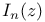 ,
, 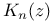 , BF
, BF 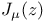 ,
, 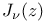 , BF
, BF 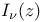 , BF
, BF 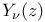 , zeros of, CP
, zeros of, CP  ,
, 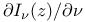 , BF
, BF 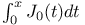 , $\int^{x}_{0} t^{-1}[1-J_{0}(t)] dt, BF
, $\int^{x}_{0} t^{-1}[1-J_{0}(t)] dt, BF 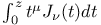 ,
,  , BF
, BF 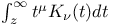 , CP
, CP 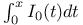 ,
, ![$\int_{0}^{x} t^{-1}[I_{0}(t)-1] dt$](/math_tex/489baad08d379dd32f5e03f1c8bf9c9082.gif) ,
, 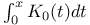 ,
, 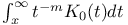 , m=0,1, CP
, m=0,1, CP 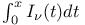 ,
, 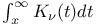 ,
,  , CP
, CP 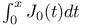 ,
,  ,
, 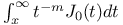 ,
, 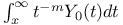 , m=0,1, CP
, m=0,1, CP 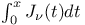 ,
, 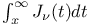 ,
, 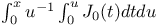 ,
, ![$\int_{0}^{x} u^{-1} \int_{0}^{u} t^{-1}[1-J_{0}(t)] dtdu$](/math_tex/7810fc2fc64b3f3cdd6bb90289c99ad582.gif) , CP
, CP 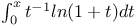 , CP
, CP 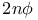
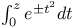 , BF
, BF 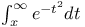 , CP
, CP 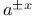 ,
, 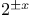 ,
, 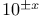 , BF
, BF 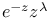 , BF
, BF 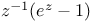 , BF
, BF 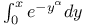 ,
, 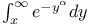
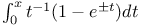 , BF
, BF  , P.V.
, P.V. 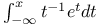 , CP
, CP  , CP
, CP