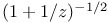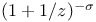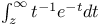|
|
 |
| јвторизаци€ |
|
|
 |
| ѕоиск по указател€м |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Luke Y.L. Ч Special Functions and Their Approximations. Volume II |
|
|
 |
| ѕредметный указатель |
Expansion, G-function, JP II 15 17Ч19 22Ч28
Expansion, G-function, LP II 16Ч19
Expansion, G-function, other G-functions I 147 148 152Ч157 183Ч189 II 14Ч24
Expansion, gamma function and derivatives see also "Expansion incomplete
Expansion, gamma function and derivatives,  , , 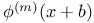 , CP I 28 29 II 287 , CP I 28 29 II 287
Expansion, gamma function and derivatives, ![$[\Gamma(x+1)]^{-1}$](/math_tex/59f8db6e920fddd47d8ad3cddb32e30682.gif) , , 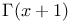 , CP I 28 29 II 287 300 , CP I 28 29 II 287 300
Expansion, gamma function and derivatives, ![$[\Gamma(z+3)]^{-1}$](/math_tex/30dc8080c17a09c05d6865fb60975b8182.gif) , , 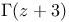 , powers of z I 26Ч28 II 299 , powers of z I 26Ч28 II 299
Expansion, gamma function and derivatives, 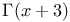 , ,  , ,  , CP I 28 29 II 287 301Ч303 , CP I 28 29 II 287 301Ч303
Expansion, gamma function and derivatives,  , series of , series of ![$[(z+1)_{n}(z+1)_{-n}]^{-1}$](/math_tex/9027dc3de902165c531ec80573eac88f82.gif) II 304 305 II 304 305
Expansion, hypergeometric function, confluent, the  -function, CP II 25 28 238Ч240 -function, CP II 25 28 238Ч240
Expansion, hypergeometric function, confluent, the  -function, G-functions II 25 -function, G-functions II 25
Expansion, hypergeometric function, confluent, the  , BF I 129Ч133 II 48 209 , BF I 129Ч133 II 48 209
Expansion, hypergeometric function, confluent, the  , CP II 35 , CP II 35
Expansion, hypergeometric function, confluent, the  , JP II 48 , JP II 48
Expansion, hypergeometric function, Gaussian, the  , ,  's I 130 's I 130
Expansion, hypergeometric function, Gaussian, the  , CP II 34 , CP II 34
Expansion, hypergeometric function, Gaussian, the  , JP II 62 , JP II 62
Expansion, hypergeometric function, Gaussian, the  , other , other  's II 62Ч64 's II 62Ч64
Expansion, hypergeometric function, generalized, the  , BF II 44 45 58Ч61 , BF II 44 45 58Ч61
Expansion, hypergeometric function, generalized, the  , CP I 296 II 30 31 , CP I 296 II 30 31
Expansion, hypergeometric function, generalized, the  , GP II 29 30 , GP II 29 30
Expansion, hypergeometric function, generalized, the  , JP II 1Ч8 13 29 44 45 , JP II 1Ч8 13 29 44 45
Expansion, hypergeometric function, generalized, the  , LP II 9Ч12 14 44 , LP II 9Ч12 14 44
Expansion, hypergeometric function, generalized, the  , other , other  's II 1Ч14 29Ч31 64 65 's II 1Ч14 29Ч31 64 65
Expansion, incomplete gamma function, 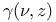 , BF II 55 56 289 , BF II 55 56 289
Expansion, incomplete gamma function, 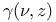 , CP II 40 41 55 56 see sine "Expansion error "Expansion exponential "Expansion Fresnel "Expansion hypergeometric "Expansion confluent" , CP II 40 41 55 56 see sine "Expansion error "Expansion exponential "Expansion Fresnel "Expansion hypergeometric "Expansion confluent"
Expansion, inverse tangent integral, t-1 arc tan t dt, CP II 310
Expansion, ker, kei, and derivatives I 216 see
Expansion, Laplace transform, inverse of, by expansion of desired function in series of Jacobi, Laguerre, and other orthogonal polynomials II 247Ч251
Expansion, Legendre polynomial, BF I 279
Expansion, logarithm, 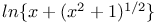 , CP II 312 , CP II 312
Expansion, logarithm, dilogarithm, 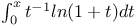 , CP II 310 , CP II 310
Expansion, logarithm, ln(1+0.16x), CP II 288
Expansion, logarithm, ln(1+x), CP II 308
Expansion, logarithm, ln(a+z),  Тs II 63 Тs II 63
Expansion, logarithm, ln(a+z), CP II 31 288 308
Expansion, logarithm, ln(a+z), JP II 13
Expansion, logarithm, ln{(1+z)/(1-z)}, series of ![$[1-(1-z^{2})^{1/2}]^{n}/z^{n}$](/math_tex/7312796fd21115c4920de80d551de68282.gif) II 63 II 63
Expansion, Lommel functions, BF II 39 54 55
Expansion, Lommel functions, CP II 27 39
Expansion, Lommel functions, GP II 39
Expansion, powers of z, 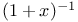 , ,  , CP II 307 , CP II 307
Expansion, powers of z, 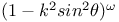 , ,  , and , and  's II 35 's II 35
Expansion, powers of z, 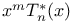 , CP I 301 , CP I 301
Expansion, powers of z, 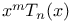 , CP I 298 , CP I 298
Expansion, powers of z,  , BF II 45 46 , BF II 45 46
Expansion, powers of z,  , JP I 285 , JP I 285
Expansion, powers of z, 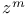 , m a positive integer or zero, , m a positive integer or zero,  's II 6 7 11Ч13 's II 6 7 11Ч13
Expansion, powers of z, 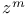 , m a positive integer or zero, CP II 295 298 , m a positive integer or zero, CP II 295 298
Expansion, powers of z, 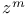 , m a positive integer or zero, JP I 277 291 II 6 7 14 , m a positive integer or zero, JP I 277 291 II 6 7 14
Expansion, powers of z, 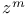 , m a positive integer or zero, LP II 11 , m a positive integer or zero, LP II 11
Expansion, powers of z, 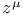 , ,  's II 2 5 9 14 31 's II 2 5 9 14 31
Expansion, powers of z, 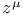 , BF II 45 46 , BF II 45 46
Expansion, powers of z, 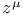 , JP I 284 285 II 2 5 20 31 , JP I 284 285 II 2 5 20 31
Expansion, powers of z, 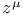 , LP II 9 , LP II 9
Expansion, Psi( )-function or logarithmic derivative of the gamma function see "Expansion gamma )-function or logarithmic derivative of the gamma function see "Expansion gamma
Expansion, sine and cosine integrals,  , , 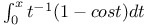 , CP II 42 289 325 , CP II 42 289 325
Expansion, sine and cosine integrals, 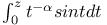 , , 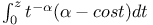 , a=0, 1 BF II 42 56 57 289 , a=0, 1 BF II 42 56 57 289
Expansion, sine and cosine integrals, 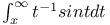 , , 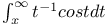 , CP II 27 289 326 327 , CP II 27 289 326 327
Expansion, sine and cosine,  , BF II 47 , BF II 47
Expansion, sine and cosine,  , CP II 34 , CP II 34
Expansion, sine and cosine, 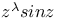 , ,  , BF II 32 33 46 47 , BF II 32 33 46 47
Expansion, sine and cosine, hyperbolic, CP II 313 314 363
Expansion, sine and cosine, hyperbolic, inverse of, CP II 312
Expansion, sine and cosine, inverse of, CP II 288 311
Expansion, sine and cosine, sin z, cos z, CP II 33 34 288 316Ч319 354
Expansion, sine and cosine, sin z, cos z, Legendre polynomials II 288
Expansion, Struve functions, 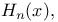 H_{n}(x)-Y_{n}(x}$, n=0, 1 CP 370 371 H_{n}(x)-Y_{n}(x}$, n=0, 1 CP 370 371
Expansion, Struve functions, 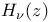 , , 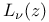 , BF II 40 55 , BF II 40 55
Expansion, Struve functions, 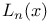 , n=0, 1, CP II 374 , n=0, 1, CP II 374
Expansion, Struve functions, integrals of, 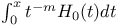 dt, m=0, 1, BF II 40 dt, m=0, 1, BF II 40
Expansion, Struve functions, integrals of, 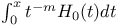 dt, m=0, 1, CP II 40 372 dt, m=0, 1, CP II 40 372
Expansion, Struve functions, integrals of,  , m=0, 1 CP II 375 , m=0, 1 CP II 375
Expansion, Struve functions, integrals of, ![$\int_{0}^{x} t^{-m} [H_{0}(t)-Y_{0}(t)] dt$](/math_tex/e82a92f69fcada258625aa988d26198282.gif) , m=0, 1, CP II 373 , m=0, 1, CP II 373
Expansion, tangent and cotangent, hyperbolic, inverse of, CP II 312
Expansion, tangent and cotangent, inverse of, arc tan x, CP II 32 288 309
Expansion, tangent and cotangent, inverse of, arc tan x, series of ![$[(1+x^{2})^{1/2}-1]^{n}/x^{n}$](/math_tex/42fc192992f004b6c0c0db54c4eb553a82.gif) II 63 II 63
Expansion, tangent and cotangent, inverse tangent integral, 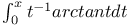 , CP II 310 , CP II 310
Expansion, tangent and cotangent, tan x, cot x, CP II 33 320
Expansion, tangent and cotangent, tan x, cot x, series of ![$[4m^{2}-z^{2}]^{-1}$](/math_tex/b01123f2d69607812ae3e612c42d9aef82.gif) II 33 II 33
Expansion, Weber function see "Anger Ч Weber"
Expansion, Whittaker functions see "Expansion hypergeometric confluent"
Expansion, Zeta function, 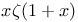 and related functions, CP II 287 306 and related functions, CP II 287 306
Exponential function I 38 40 48 209 II 195 257 292 see "Expansion"
Exponential integral I 3 7 135 221 222 II 201 see "Expansion"
Fermi Ч Dirac integrals II 291
Fresnel integrals I 135 224 227 see "Expansion"
G-function I 136Ч208
G-function, analytic continuation I 148 149 194
G-Function, approximation of, by polynomials and rational functions see "Approximation"
G-Function, asymptotic expansion, exponential, recurrence formula for coefficients in I 200 202 205Ч208
G-function, asymptotic expansion, large parameter II 23 24 26 27
G-Function, asymptotic expansion, large variable I 178Ч180 189Ч194
G-function, definition I 139 142Ч145
G-function, differential equation and solutions I 181 182
G-function, elementary properties I 149Ч152
G-Function, expansions of, in series of, G-functions I 147 148 152Ч157 183Ч189 II 24 see
G-Function, expansions of, in series of, generalized hypergeometric functions I 139 142 145Ч147 see
G-function, expressed as a named function I 230Ч234
G-function, hypergeometric functions and named functions, connection with I 225Ч234
G-function, integral representations I 59 143Ч145 159 164Ч175 177 II 23
G-Function, integrals involving Euler and related transforms I 170Ч177 II 23
G-Function, integrals involving Fourier transform I 166 169
G-Function, integrals involving Laplace and inverse Laplace transforms I 166Ч169
G-Function, integrals involving Mellin and inverse Mellin transforms I 157Ч159
G-Function, integrals involving other transform pairs I 177
G-Function, integrals involving product of two G-functions I 159Ч166
G-function, multiplication theorems I 152Ч157
G-function, named functions expressed in terms of I 225Ч230
Gamma function I 8Ч37 see
Gamma function, analytic continuation I 10 11
Gamma function, approximation see "Approximation"
Gamma function, asymptotic expansion, 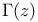 and and 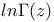 I 31Ч33 I 31Ч33
Gamma function, asymptotic expansion, ratio of products of gamma functions I 33Ч37
Gamma function, asymptotic expansion, recurrence formula for coefficients in asymptotic expansion for ratio of products of gamma functions I 205Ч208
Gamma function, definite integrals expressed in terms of I 15 16 60 61 157 177
Gamma function, definition and elementary properties I 8Ч10
Gamma function, expansion see "Expansion"
Gamma function, integral representations I 8 14Ч18
Gamma function, logarithmic derivative of see "Psi-function"
Gamma function, multiplication formula I 11 12
Gamma function, numerical values for certain fractional arguments II 293
Gamma function, power series and other expansions I 26Ч31
Gegenbauer polynomial I 273 279
GP see "Gegenbauer or ultraspherical polynomials"
h-transform I 165
Hankel functions I 135 204 213 215 230 234 II 27
Hankel transform I 165 287
Hermite polynomials I 135 273 II 245
Hypergeometric function (confluent),  see ЂG-function" "Hypergeometric "Hypergeometric "Incomplete see ЂG-function" "Hypergeometric "Hypergeometric "Incomplete
Hypergeometric function (confluent),  , ,  -function, Whittaker functions I 115Ч134 -function, Whittaker functions I 115Ч134
Hypergeometric function (confluent),  , approximation of, by polynomials and rational functions see "Approximation" , approximation of, by polynomials and rational functions see "Approximation"
Hypergeometric function (confluent),  , asymptotic expansion, large parameter(s) I 129 133 134 II 200 , asymptotic expansion, large parameter(s) I 129 133 134 II 200
Hypergeometric function (confluent),  , asymptotic expansion, large variable I 127 128 , asymptotic expansion, large variable I 127 128
Hypergeometric function (confluent),  , asymptotic expansion, uniform, large parameters and variable II 203 204 207 208 210 211 , asymptotic expansion, uniform, large parameters and variable II 203 204 207 208 210 211
Hypergeometric function (confluent),  , confluence principle I 48 , confluence principle I 48
Hypergeometric function (confluent),  , contiguous relations I 48 118 119 126 , contiguous relations I 48 118 119 126
Hypergeometric function (confluent),  , definition I 40 47 49 , definition I 40 47 49
Hypergeometric function (confluent),  , difference-differential properties I 48 117Ч119 125 126 , difference-differential properties I 48 117Ч119 125 126
Hypergeometric function (confluent),  , differential equation I 119 120 , differential equation I 119 120
Hypergeometric function (confluent),  , differential equation, solutions, complete I 121Ч124 , differential equation, solutions, complete I 121Ч124
Hypergeometric function (confluent),  , differential equation, solutions, degenerate I 120 121 , differential equation, solutions, degenerate I 120 121
Hypergeometric function (confluent),  , differential equation, solutions, logarithmic I 122 123 , differential equation, solutions, logarithmic I 122 123
Hypergeometric function (confluent),  , differential equation, solutions, ordinary I 119 , differential equation, solutions, ordinary I 119
Hypergeometric function (confluent),  , elementary relations I 117Ч119 , elementary relations I 117Ч119
Hypergeometric function (confluent),  , evaluation of a certain , evaluation of a certain  for special value of argument I 113 for special value of argument I 113
Hypergeometric function (confluent),  , expansions in series of Jacobi polynomials, Chebyshev polynomials, etc. see "Expansion" , expansions in series of Jacobi polynomials, Chebyshev polynomials, etc. see "Expansion"
Hypergeometric function (confluent),  , expressed as named function I 224 , expressed as named function I 224
Hypergeometric function (confluent),  , G-function, connection with I 225 226 228 231 234 , G-function, connection with I 225 226 228 231 234
Hypergeometric function (confluent),  , integral representations I 115Ч117 , integral representations I 115Ч117
| Hypergeometric function (confluent),  , Kummer relations I 121 124Ч126 , Kummer relations I 121 124Ч126
Hypergeometric function (confluent),  , Laguerre and Hermite polynomial, connection with I 273 , Laguerre and Hermite polynomial, connection with I 273
Hypergeometric function (confluent),  , named functions expressed in terms of I 211Ч213 220Ч224 , named functions expressed in terms of I 211Ч213 220Ч224
Hypergeometric function (confluent),  , other notations and related functions I 134 135 , other notations and related functions I 134 135
Hypergeometric function (confluent),  , products I 211 228 233 234 , products I 211 228 233 234
Hypergeometric function (confluent),  , Wronskians I 124 , Wronskians I 124
Hypergeometric function (confluent),  , zeros of , zeros of 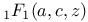 II 208Ч210 II 208Ч210
Hypergeometric function (Gaussian),  I 38Ч114 see I 38Ч114 see
Hypergeometric function (Gaussian),  , analytic continuation I 68Ч71 , analytic continuation I 68Ч71
Hypergeometric function (Gaussian),  , approximation of, by polynomials and rational functions see "Approximation" , approximation of, by polynomials and rational functions see "Approximation"
Hypergeometric function (Gaussian),  , asymptotic expansions for large parameter(s) I 235Ч242 , asymptotic expansions for large parameter(s) I 235Ч242
Hypergeometric function (Gaussian),  , confluence principle I 49 , confluence principle I 49
Hypergeometric function (Gaussian),  , contiguous relations I 47 89 , contiguous relations I 47 89
Hypergeometric function (Gaussian),  , convergence of series I 65 68 , convergence of series I 65 68
Hypergeometric function (Gaussian),  , definition I 39 41 , definition I 39 41
Hypergeometric function (Gaussian),  , difference-differential properties I 44Ч47 88 275 276 , difference-differential properties I 44Ч47 88 275 276
Hypergeometric function (Gaussian),  , differential equation I 64 93 , differential equation I 64 93
Hypergeometric function (Gaussian),  , differential equation, solutions, complete I 65 72Ч84 , differential equation, solutions, complete I 65 72Ч84
Hypergeometric function (Gaussian),  , differential equation, solutions, degenerate I 65 66 69 77Ч84 , differential equation, solutions, degenerate I 65 66 69 77Ч84
Hypergeometric function (Gaussian),  , differential equation, solutions, logarithmic I 75Ч84 , differential equation, solutions, logarithmic I 75Ч84
Hypergeometric function (Gaussian),  , differential equation, solutions, ordinary I 64 65 67Ч71 , differential equation, solutions, ordinary I 64 65 67Ч71
Hypergeometric function (Gaussian),  , elementary relations I 44Ч46 , elementary relations I 44Ч46
Hypergeometric function (Gaussian),  , evaluation of, for special values of argument I 99Ч103 114 , evaluation of, for special values of argument I 99Ч103 114
Hypergeometric function (Gaussian),  , expansions in series of Jacobi polynomials, other , expansions in series of Jacobi polynomials, other  's, etc. see "Expansion" 's, etc. see "Expansion"
Hypergeometric function (Gaussian),  , expressed as named function I 224 , expressed as named function I 224
Hypergeometric function (Gaussian),  , G-function, connection with I 139 , G-function, connection with I 139
Hypergeometric function (Gaussian),  , integral representations I 57 58 62 63 89Ч91 , integral representations I 57 58 62 63 89Ч91
Hypergeometric function (Gaussian),  , integrals involving I 170 172Ч174 , integrals involving I 170 172Ч174
Hypergeometric function (Gaussian),  , Kummer relations I 67 68 85Ч92 , Kummer relations I 67 68 85Ч92
Hypergeometric function (Gaussian),  , named functions expressed in terms of I 209Ч211 , named functions expressed in terms of I 209Ч211
Hypergeometric function (Gaussian),  , orthogonal polynomials, classical, connection with I 273 , orthogonal polynomials, classical, connection with I 273
Hypergeometric function (Gaussian),  , polynomial I 40 65 66 91 , polynomial I 40 65 66 91
Hypergeometric function (Gaussian),  , quadratic transformations I 92Ч98 , quadratic transformations I 92Ч98
Hypergeometric function (Gaussian),  , truncated series I 42 109 110 , truncated series I 42 109 110
Hypergeometric function (Gaussian),  , Wronskians I 84 85 , Wronskians I 84 85
Hypergeometric function (generalized),  I 38Ч114 115Ч134 135Ч208 see "Special I 38Ч114 115Ч134 135Ч208 see "Special
Hypergeometric function (generalized),  , analytic continuation I 149 , analytic continuation I 149
Hypergeometric function (generalized),  , approximation of, by polynomials and rational functions see "Approximation" , approximation of, by polynomials and rational functions see "Approximation"
Hypergeometric function (generalized),  , asymptotic expansion, exponential, recurrence formula for coefficients in I 200 202 205Ч208 , asymptotic expansion, exponential, recurrence formula for coefficients in I 200 202 205Ч208
Hypergeometric function (generalized),  , asymptotic expansion, large parameter(s) I 51 55 56 133 242Ч266 , asymptotic expansion, large parameter(s) I 51 55 56 133 242Ч266
Hypergeometric function (generalized),  , asymptotic expansion, large variable I 195Ч203 , asymptotic expansion, large variable I 195Ч203
Hypergeometric function (generalized),  , confluence principle and theorems I 49 51 53Ч56 , confluence principle and theorems I 49 51 53Ч56
Hypergeometric function (generalized),  , contiguous relations I 48 , contiguous relations I 48
Hypergeometric function (generalized),  , convergence of series I 43 44 , convergence of series I 43 44
Hypergeometric function (generalized),  , definition I 41 42 136 , definition I 41 42 136
Hypergeometric function (generalized),  , difference-differential properties I 44 48 , difference-differential properties I 44 48
Hypergeometric function (generalized),  , differential equation I 136Ч138 247 , differential equation I 136Ч138 247
Hypergeometric function (generalized),  , differential equation, solutions, complete I 147 148 181 182 , differential equation, solutions, complete I 147 148 181 182
Hypergeometric function (generalized),  , differential equation, solutions, logarithmic I 140Ч143 , differential equation, solutions, logarithmic I 140Ч143
Hypergeometric function (generalized),  , differential equation, solutions, ordinary I 137Ч139 , differential equation, solutions, ordinary I 137Ч139
Hypergeometric function (generalized),  , elementary relations I 44 , elementary relations I 44
Hypergeometric function (generalized),  , evaluation of , evaluation of 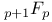 for special values of argument I 26 113 114 257Ч259 II 136 139 142Ч144 151 153 154 for special values of argument I 26 113 114 257Ч259 II 136 139 142Ч144 151 153 154
Hypergeometric function (generalized),  , evaluation of , evaluation of 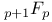 for special values of argument, p=1 I 99Ч103 114 for special values of argument, p=1 I 99Ч103 114
Hypergeometric function (generalized),  , evaluation of , evaluation of 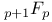 for special values of argument, p=2 I 103Ч111 113 259 II 142 for special values of argument, p=2 I 103Ч111 113 259 II 142
Hypergeometric function (generalized),  , evaluation of , evaluation of 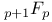 for special values of argument, p=3 I 112Ч114 II 140 for special values of argument, p=3 I 112Ч114 II 140
Hypergeometric function (generalized),  , evaluation of a certain , evaluation of a certain 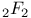 for special values of argument I 113 for special values of argument I 113
Hypergeometric function (generalized),  , expansion theorem for large parameter I 51 55 56 , expansion theorem for large parameter I 51 55 56
Hypergeometric function (generalized),  , expansions in series of other , expansions in series of other  's, Jacobi polynomials, etc. see "Expansion" 's, Jacobi polynomials, etc. see "Expansion"
Hypergeometric function (generalized),  , expressed as named function I 224 225 , expressed as named function I 224 225
Hypergeometric function (generalized),  , G-function, connection with I 139 142 145Ч147 225 230 231 , G-function, connection with I 139 142 145Ч147 225 230 231
Hypergeometric function (generalized),  , integral representations I 58Ч63 , integral representations I 58Ч63
Hypergeometric function (generalized),  , integrals involving I 58Ч62 164 168 169 , integrals involving I 58Ч62 164 168 169
Hypergeometric function (generalized),  , multiplication theorem I 155 , multiplication theorem I 155
Hypergeometric function (generalized),  , named functions expressed in terms of I 209Ч224 , named functions expressed in terms of I 209Ч224
Hypergeometric function (generalized),  , nearly poised I 103 , nearly poised I 103
Hypergeometric function (generalized),  , polynomial I 42 210 , polynomial I 42 210
Hypergeometric function (generalized),  , truncated series I 42 210 , truncated series I 42 210
Hypergeometric function (generalized),  , well poised I 103 104 , well poised I 103 104
Incomplete gamma function and related functions I 135 220Ч224 II 186Ч213 see "Expansion"
Inequalities for 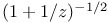 II 185 II 185
Inequalities for 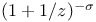 II 180 II 180
Inequalities for 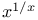 II 181 II 181
Inequalities for 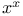 II 180 II 180
Inequalities for Bessel function ratio, 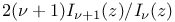 II 82 II 82
Inequalities for beta function (incomplete) II 178
Inequalities for error functions, 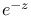 II 195 II 195
Inequalities for error functions, 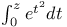 II 195 II 195
Inequalities for error functions, 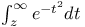 II 201 II 201
Inequalities for exponential integral, 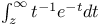 II 201 II 201
Inequalities for hypergeometric function, Gaussian, $_{2}F_{1}(1, \sigma ; \rho + 1; x) II 173 178
Inequalities for incomplete gamma function, 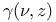 II 194 195 II 194 195
Inequalities for Jacobi polynomials I 280
Integral equations, solution of by expansion in series of Chebyshev polynomials of the first kind see "Expansion"
Integrals see "Bessel functions integrals see "Expansion"
Integration, numerical see "Approximation integrals"
Interpolation, inverse II 231
Inverse tangent integral II 310
Jacobi function I 274
Jacobi polynomials, application of in approximation of functions by polynomials and rational functions II 68 95 98 see
Jacobi polynomials, asymptotic expansion for large order I 53 54 237 250Ч259 278 279
Jacobi polynomials, Bessel function, connection with I 52
Jacobi polynomials, definition and basic properties I 273Ч275 280
Jacobi polynomials, difference-differential properties I 275 276 II 170Ч172 177 179
Jacobi polynomials, evaluation and estimation of coefficients of given f(x) when expanded in series of I 286Ч296
Jacobi polynomials, evaluation and estimation of coefficients of given f(x) when expanded in series of, asymptotic estimates of coefficients I 293Ч296
Jacobi polynomials, evaluation and estimation of coefficients of given f(x) when expanded in series of, coefficients as integral transform I 286Ч290
Jacobi polynomials, evaluation and estimation of coefficients of given f(x) when expanded in series of, coefficients when f(x) is defined by Taylor series I 290Ч293
Jacobi polynomials, expansion of functions in series of I 283Ч285 290Ч293 see
Jacobi polynomials, extended and generalized, asymptotic expansion for large order I 53 54 247Ч263 281Ч283
Jacobi polynomials, extended and generalized, definition I 247
Jacobi polynomials, extended and generalized, expansion of functions in series of I 291 292 see
Jacobi polynomials, extended and generalized, generating function I 254
Jacobi polynomials, extended and generalized, recurrence or difference formula II 134Ч142 145Ч147
Jacobi polynomials, generating functions I 254 278
Jacobi polynomials, inequalities I 280
Jacobi polynomials, integrals involving I 276 277 281Ч283 286
Jacobi polynomials, numerical integration and inversion of Laplace transforms II 244 245 247Ч249 251Ч253
Jacobi polynomials, orthogonal polynomials, connection with other I 273
Jacobi polynomials, orthogonality property I 276
JP see "Jacobi polynomials including extended and generalized Jacobi polynomials"
K-transform I 165
Ker and Kei functions I 216 see "Approximation" "Expansion"
Kontorovich Ч Lebedev transforms I 177
Kummer's formula I 57
Kummer's solution I 67
Laguerre polynomial, approximation of functions by use of see "Approximation"
Laguerre polynomial, asymptotic expansion for large order I 264Ч266 II 203 207
Laguerre polynomial, confluent hypergeometric function, connection with I 135
Laguerre polynomial, definition and orthogonality property I 273
Laguerre polynomial, difference-differential properties II 198Ч201
Laguerre polynomial, expansion of functions in series of I 291 292 see
Laguerre polynomial, generalized and extended, asymptotic expansion for large order I 263Ч266
Laguerre polynomial, generalized and extended, definition I 263
Laguerre polynomial, generalized and extended, expansion of functions in series of I 291 292 see
Laguerre polynomial, generalized and extended, recurrence or difference formula II 143 144 147 148
Laguerre polynomial, Jacobi polynomial, connection with I 273
Laguerre polynomial, numerical integration and inversion of Laplace transforms II 245 249Ч251
Laguerre polynomial, zeros of II 208Ч210
Laplace transform or integral, Abelian-type theorems II 246 247
Laplace transform or integral, approximation see "Approximation"
Laplace transform, inverse of, approximation and expansion see "Approximation" "Expansion"
Laplace transform, inverse of, Tauberian-type theorems II 247
Legendre functions I 178 211 II 165
Legendre polynomials I 273 279
Logarithm I 38 40 210 II 292 see "Expansion"
Lommel functions I 217 218 227 234 see "Expansion"
lp see "Laguerre polynomials including extended and generalized Laguerre polynomials"
Mathematical constants II 292 293
Mehler transforms I 178
Mellin Ч Barnes integrals, evaluation of by trapezoidal integration rule II 225
Named functions expressed as  's I 209Ч224 's I 209Ч224
Named functions expressed as G-functions I 225Ч230
Order symbols I 1
Orthogonal functions I 267Ч270
Orthogonal polynomials I 267Ч329 see "Chebyshev "Bessel
Orthogonal polynomials, application to approximation and expansion of functions see "Approximation" "Expansion"
Orthogonal polynomials, classical, orthogonal properties of I 273
Orthogonal polynomials, definition and basic properties I 267Ч272 II 145
P see "Polynomial"
Pade approximation see also "Approximation" "Continued
|
|
 |
| –еклама |
 |
|
|
 |
|
ќ проекте
|
|
ќ проекте



 ,
, 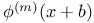 , CP
, CP ![$[\Gamma(x+1)]^{-1}$](/math_tex/59f8db6e920fddd47d8ad3cddb32e30682.gif) ,
, 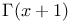 , CP
, CP ![$[\Gamma(z+3)]^{-1}$](/math_tex/30dc8080c17a09c05d6865fb60975b8182.gif) ,
, 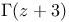 , powers of z
, powers of z 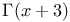 ,
,  ,
,  , CP
, CP  , series of
, series of ![$[(z+1)_{n}(z+1)_{-n}]^{-1}$](/math_tex/9027dc3de902165c531ec80573eac88f82.gif)
 -function, CP
-function, CP  , BF
, BF  ,
,  , BF
, BF 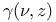 , BF
, BF 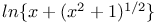 , CP
, CP 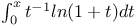 , CP
, CP ![$[1-(1-z^{2})^{1/2}]^{n}/z^{n}$](/math_tex/7312796fd21115c4920de80d551de68282.gif)
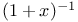 ,
,  , CP
, CP 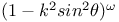 ,
,  , and
, and 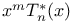 , CP
, CP 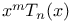 , CP
, CP  , BF
, BF 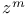 , m a positive integer or zero,
, m a positive integer or zero, 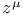 ,
,  ,
, 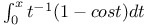 , CP
, CP 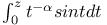 ,
, 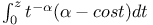 , a=0, 1
, a=0, 1 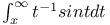 ,
, 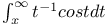 , CP
, CP  , BF
, BF 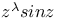 ,
,  , BF
, BF 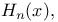 H_{n}(x)-Y_{n}(x}$, n=0, 1
H_{n}(x)-Y_{n}(x}$, n=0, 1 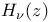 ,
, 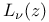 , BF
, BF 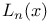 , n=0, 1, CP
, n=0, 1, CP 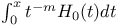 dt, m=0, 1, BF
dt, m=0, 1, BF  , m=0, 1
, m=0, 1 ![$\int_{0}^{x} t^{-m} [H_{0}(t)-Y_{0}(t)] dt$](/math_tex/e82a92f69fcada258625aa988d26198282.gif) , m=0, 1, CP
, m=0, 1, CP ![$[(1+x^{2})^{1/2}-1]^{n}/x^{n}$](/math_tex/42fc192992f004b6c0c0db54c4eb553a82.gif)
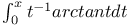 , CP
, CP ![$[4m^{2}-z^{2}]^{-1}$](/math_tex/b01123f2d69607812ae3e612c42d9aef82.gif)
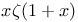 and related functions, CP
and related functions, CP 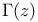 and
and 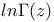
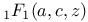
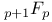 for special values of argument
for special values of argument 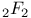 for special values of argument
for special values of argument