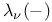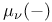Авторизация
Поиск по указателям
Cohn P.M. — Free Rings and Their Relations (London Mathematical Society Monographs)
Обсудите книгу на научном форуме Нашли опечатку?
Название: Free Rings and Their Relations (London Mathematical Society Monographs)Автор: Cohn P.M. Аннотация: In the development of ring theory there are two strands, each with its own problems and methods, and although with many points of contact, they have never merged completely. One of them is the theory of algebras; this was a non-commutative (indeed sometimes even non-associative) theory from the beginning but there were always heavy finiteness restrictions, which are only gradually being relaxed. Thus while there is a fairly substantial theory of Artinian rings, the theory of Noetherian rings is still in its early stages, and although it is being developed vigorously, it is clear that some type of maximum condition is essential for its development.
Язык: Рубрика: Математика /Серия: Сделано в холле Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Издание: 2nd editionГод издания: 1985Количество страниц: 608Добавлена в каталог: 15.05.2011Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
289 5 71f. 287 115f. xviii 449 3 74 19 63 125 382 390 382 Abelian category 546 Abelianizing a ring (or group) 341 448f. Absolute property 205 Abstract atomic factor 215 ACC = ascending chain condition 5 154 Additive category 54 Additive functor 549 Adjoint Associativity 552 Adjoint functor, pair 548 Admissible system, matrix 384 421 Affine automorphism 342 Affine scheme 487 Algebraic algebra 220 Algebraic element (left, right) 515 Algebraic matrix 506 Algebraic power series 134 291 Amitsur's theorem (on generalized polynomial identities) 281ff. Anti-ideal 322 334 376 379 Artin's problem 521 Artinian ring = ring with (left, right) DCC 22 ASSOCIATED 25 153 Atom, (n-) atomic 73 154 168 Augmentation ideal 61 Augmentation preserving automorphism 61 342 Baer's criterion 80 549 Basis theorem for abelian groups 494 Bergman — Dicks localization theorem 459 Bergman's centralizer theorem 340 378 Bergman's conjugacy theorem 529 Bezout domain (left, right) 12 69 Bezout identity 86 Bezout ring (weak) 86 Biased 357 Biassociated 422 Bicentralizer 282 Bidegree 344 Binomial extension 518 BirkhofTs representation theorem 180 544 Bordered matrix 405 421 Bound component, module 228f. Bound, strongly 234 Bounded element, module 312 379 Bounded linear transformation, matrix 505 Cancellation monoid 35 Capacity 22 Card(I) = cardinal of I, also |I| xx Category 544 Cayley — Hamilton theorem 505 539 Central extension 518 Chain ring 170 Characteristic of a module 24 Chase's lemma 175 Cleavage, cleft 189 Closed submodule, closure 245 251 Closed subring 102 Code 324 379 Cofinal 73 289 Cohen's theorem 310 Coherent family of matrix groups 74 Coherent ring 258 554 Coinduced extension 552 Cokernel 545 Column rank 247 Comaximal relation 28 161 Comaximal transposition 178 270 Comaximally transposable 171 Comma category 548 Comma-free code 379 Commensurable xix Companion matrix 505 Comparison theorem for numerators and denominators 429 Complete direct decomposition 186 Complete inversely filtered ring 126 Complete lattice 540 Completely primary ring 187 Completely reducible module 189 Completion (of a filtered ring) 126 Complex-skew polynomial ring 54 164 179f. 191 210 225 Computable matrix 15 Conductor 299 304 Conical monoid 19 153 321 conjugate 504 Connected (inversely filtered ring) 129 Connecting homomorphism 550 Constants 40 Content 107 Continuant polynomial 117 Convex 219 526 Coprime relation 161 Core 384 Cover (in a lattice) 540 Cramer's rule 384 Cyclic matrix 504 539 DCC = descending chain condition 10 de Jonquieres automorphism 342 379 Decomposable (left, right) 184 316 Dedekind lemma 372f. Defect theorem 329 Degenerate matrix 400 Degree 60 100 111 344 349 Degree of filtration 95 Degree, formal 104 Degree-function 51 139 Denominator 36 384 Dense subcategory 314 Dense submodule 245 Dependence number, 96 151 Dependence number, inverse, 125 Dependence relation 64 100 475 Dependence relative to a filtration 95 Dependence relative to a transfinite function 139 Depth 386 432ff. Derivation 39 Derivation, higher 137 358 523 Derived set 88 151 Determinantal sum 396 DFL = distributive factor lattice 199 207 Diagonal reduction 489 538 Diagonal sum xix 395 Diamond lemma 148 Dicks commutator test 355f. Dieudonne determinant 382 448 Differential equations 63 Differential operators 54 58 Differential transformations 503 Dimension, injective, projective 258 551 Direct product 544 Direct sum 545 Directed set 292 398 Distributive factor lattice, DFL 199 Distributive module 193 Distributiye lattice 542 Division algorithm 87ff. Division closure 335 387 Divisor group, D(-) 451 DL = category of finite distributive lattices 211 Duality = category anti-equivalence 548 Duality factorial 166 Duality for modules xviii 165 232ff. Duality in lattices 540 E-related 119 Eigenring, E(-) 30 63 192 Eigenring, scalar 220 Eigenvalue 504 510 Elementary divisor 495 Elementary divisor ring 492 539 Elementary embedding 287 Elementary matrix, operation 490 End of a graph 352 Epic R-field 388 Epic, epimorphism 545 Equivalence of categories 548 Equivalence of factors 215 Essential extension 235 Essential left factor 243 Euclidean algorithm 89 121f. 151 Euclidean ring 88 Euler's theorem (homogeneous functions) 62 Exact functor (left, right) 549 Exact sequence 546 Exchange Principle 126 Ext, extension of modules 551 Extremity of a graph 351 Factor closed 415 454 Factor complete 454 Factorial duality 166 Fibonacci numbers 117 Field of fractions 38 251 388 Field spectrum, X(-) 410 487 Filtered ring 95 Filtered ring, truncated 111 Filtration 94 Filtration, 125 Final object 545 Finitely generated (presented, related) 24 Fir (left, right), n-fir 67 71 85 Fir, one-sided 142 176 536 Firoid 86 Fitting's lemma 187 197 240 Five-lemma 547 Fixed ring 362 Flat module 49 552 Formal degree 104 Formal power series 55 Fox derivative 55 Fractions (monoid, group, ring, field) 34ff. Free algebra 59 106 Free ideal ring ( = fir) 71 Free monoid 59 Free product of groups 349 Frobenius inequality 261 Full matrix 159 192 Full operation 422 Full subcategory 545 Fully atomic 168 Fully invariant 195 Fully inverting homomorphism 415 Fully reducible 189 314 functor 547 Galois correspondence for free algebras 376ff. 380 Gauss's lemma 85f. 107 124 GE-related 119 GE-ring 76 86 Generalized polynomial identity, GPI 282 Gerasimov — Malcolmson localization theorem 484 GL-related 119 162 Global dimension 551 Global section (rational, integral) 487 Graded ring 100 Grothendieck category 239 Group of fractions 34 Hasse's criterion 94 HCF, HCLF, HCRF = highest common (left, right) factor (=greatest common divisor) 122 150 154 Height (of lattice element) 542 Hereditary ring 11 Hermitering 15 63 Higher derivation 137 358 523 Higman's trick 272 Hilbert basis theorem 53 63 Hilbert series 107 364ff. Hilbert theorem 90 521 HNN-construction 114 151f. 421 Hollow matrix 160 397 Homogeneous 345 Honest homomorphism 250 415 I-atom, I-prime 156 301 I-decomposable 316 IBN = invariant basis number 6 63 Idealizer, I(-) 30 63 Indecomposable 186 316 Index of a matrix, i(-) 28 Inert (totally, n-) 83 86 Inertia theorem 133 152 Initial object 545 Injective dimension 551 Injective hull 549 Inner derivation 40 Inner rank 248 487 Integral element, closure 298f. 303 Intersection theorem for firs 295 297 interval 540 Invariant 362 379 Invariant basis number (IBN) 6 63 Invariant elements, I(-) 154 156 300 379 Invariant factors 495 504 Invariant ring (monoid) 154 156 Inverse dependence number, 125 Inverse filtration 125 Inverse weak algorithm (n-term) 125 Inverting 34 382 Involution 294 Irreducible topological space 411 Irredundant decomposition 411 543 Isomorphism in a category 545 Isomorphism of factorizations 164 Isomorphism of idempotent matrices 20 Isotone (=order-preserving) mapping 211 Iterated skew polynomial ring 532 J-ring 532 Jacobian matrix, problem 355 380 Jacobson radical, J(-) 20 Join 540 543 Join-irreducible 212 543 Jordan — Hoelder theorem 168 198 214 542 Jung-van der Kulk theorem 348 379 Kaplansky's theorem (projective modules) 12 63 Kernel 45 545 Kharchenko — Galois correspondence 376 Kharchenko — Lane theorem 363f. 380 Klein's theorem 82 86 Kraft — McMillan inequality 325 379 Kronecker functional ring 308 Krull domain 302 Krull — Schmidt theorem 186 241 297 494 543 Kurosh — Ore theorem 185 543 L(cR, R) = lattice of principal right ideals between cR and R 80 179 Lane's lemma 357 380 Large (left, right) 36 Laurent series 56 521ff. LCM, LCRM, LCLM = least common (right, left) multiple 81 154 Leading form 344 Leading term 101 140 527 Leapfrog construction 117 Leibniz's formula 46 Length of a lattice 542 Length of an element, monomial 60 322 494 Level 174 Lifting property 77
Реклама
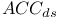= ACC on finitely generated submodules with dense inclusions 
= ACC on n-generator sub-modules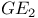 
, standard form 
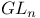= general linear group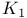 
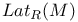= lattice of submodules of M 
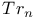= group of triangular matrices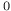 
-adic filtration 
-inverting homomorphism 
|
|
О проекте