Авторизация
Поиск по указателям
Mirsky L. — Transversal theory. An account of some aspects of combinatorial mathematics
Обсудите книгу на научном форуме Нашли опечатку?
Название: Transversal theory. An account of some aspects of combinatorial mathematicsАвтор: Mirsky L. Аннотация: "Transversal theory, the study of combinatorial questions of which Philip Hall's classical theorem on 'distinct representatives' is the fount and origin, has only recently emerged as a coherent body of knowledge. The pages that follow represent a first attempt to provide a codification of this new subject and, in particular, to place it firmly in the context of the theory of abstract independence. I have sought to make the exposition leisurely, systematic, and as nearly self-contained as possible; but since the length of the book had to be kept within conventional bounds, it has been necessary to exclude certain topics even though they impinge on my central theme. Thus I say nothing about the subject of 'flows in networks' initiated by Ford and Fulkerson; I pass in silence over the exciting possibilities of establishing combinatorial theorems by the method of linear programming; and I refer only occasionally to the theory of graphs. I hope that as a result my presentation has gained in care and clarity what it has undoubtedly lost in breadth of treatment.
Язык: Рубрика: Математика /Серия: Сделано в холле Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1971Количество страниц: 269Добавлена в каталог: 04.12.2010Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
90 17 34 7 154 204 183 33 5 2 74 13 7 148 33 1 206 6 193 6 6 5 2 33 193 130 26—27 3 3 3 76 206 139 2 2 8 35 34 2 2 1 12 13 'Qualitative' results 106 216 217 'Quantitative' results 106 217 A(J) 26 Adjunction 39 Admissible sets and mappings 33 Aigner, M. 146 236 Antichain 17 Ashe, D.S. 110 236 Axiom of Choice 8 18 19 23 A[N] 14 A|B, 119 Banach, S. 11 23 236 Bases (of independence space) 119—123 128 Beckmann, M.J. 211 241 Bell, J.L. 71 236 Berge, C. 23 146 236 Bijection 3 Bipartite family 189 190 Bipartite graph 21 Birkhoff's theorem 192 212 213 Birkhoff, G. 110 192 212 213 236 Bleicher, M.N. 110 236 Bollobas, B. 224 236 Boolean (restricted) polynomial 84 Boolean algebra 38 69 73 Boolean atoms 14 15 23 Brualdi, R.A. 23 72 110 128 129 142 143 146 167 168 182 213 216 218 221 224 226 231 232 234 236 237 Bushaw, D. 23 237 Cantor's theorem 13 cardinal number 12 Cardinal of family 13 Cartesian product 7 8 Chain 17 Chapman, H.W, 167 237 Characteristic set 225 Chase, P.J. 129 237 Choice function 8 Choice function, local, global 52 Chromatic number 22 64 72 Circuit 234 Cofinite set 100 Cohn, P.M. 128 237 Collection 1 Combinatorial equivalence 84 85 Common partial transversal (CPT) 147 151 176 177 Common system of representatives (CSR) 147—149 156 157 170—174 189 Common system of representatives (CSR) with defect 175 Common transversal (CT) 147 149 151 156 158 159 167 177 178 180 181 Common transversal (CT) of cosets 149 167 171 172 182 Common transversal (CT) of maximal subfamilies 181 182 Compact topological space 20 Comparability graph 71 Comparable elements 17 Complement 2 Complementary structure 140 141 146 Concurrence in graphs 21 Cover 20 Dantzig, G.B. 72 237 de Bruijn, N.G. 72 182 237 de Morgan identities 9 Decomposition theorem, Dilworth's 61—64 72 190 191 217 218 221 Defect form of Hall's theorem 40 50 Defect form of Rado's theorem 95 101 145 Defect, common system of partial transversal 25 Defect, common system of representatives with 175 Degree (of a node) 32 Deltoid 33 34 Denumerable set 13 Dependent set 90 Diagonal (of a matrix) 183 Difference (of sets) 2 Dilworth's decomposition theorem 61—64 72 190 191 217 218 221 Dilworth, R.P. 61 63 72 191 217 218 220 221 237 Direct sum of mappings 4 Disconnecting pair 143 Discrete topological space 20 Disjoint, partial transversals 74 75 Disjoint, paths 22 Disjoint, sets 2 Dlab, V. 110 237 Domain (of a mapping) 3 Doubly-stochastic (d.s.), matrix 183 185 192—204 213 Doubly-stochastic (d.s.), pattern 199—204 Dowling, T.A. 146 236 Dual, deltoid 34 Dual, family 35 184 Dual, statement 35 Duality 32—38 216 Dualization 39 Dulmage, A.L. 51 169 182 185 212 237 242 EDGE 21 Edmonds, J. 38 102 110 111 134 135 145 146 212 215 220 237 238 Egervary, E. 38 211 212 238 Elementary constructions 39 40 215 Elements, comparable and incomparable 17 Elements, linked 33 Elements, marginal 46 51 105 106 111 156 167 Elements, maximal 17 Empty set 1 Entringer, R.C. 212 238 Equicardinality of bases 57 72 121 128 Erdoes, P. 72 88 237 238 Everett, C.J. 38 71 72 182 238 Extension, of families 39 Extension, of partial order 19 68 Extension, of partial order on groups 68 Family 5 Family, associated with matrix 183 Family, bipartite 189 190 Family, dual 35 184 Family, restricted 34 Finite character 18 Flood, M.M. 212 238 Flows in networks 167 213 217 Folkman, J. 72 182 221 238 Ford and Fulkerson, criterion of 150—153 215 Ford, L.R.Jr. 88 150 152 156 166 167 180 182 213 215 217 238 Formal incidence matrix 101 110 184 Foster, B.L. 71 238 Fournier.J.-C. 128 238 Frobenius's theorem 189 212 214 Frobenius, G. 189 211 212 214 238 Fuchs, L. 72 238 Fulkerson's linking principle 206 213 216 Fulkerson, D.R. 38 72 88 102 110 134 135 146 150 152 156 166 167 180 182 206 213 215 216 217 226 232 238 239 Gale — Ryser criterion 76 88 206—209 213 Gale, D. 38 88 206 208 213 215 222 239 Gallai, T. 72 239 Global choice function 52 Gottschalk, W.H. 71 239 Graph(s) 21 23 Graph(s), bipartite 21 Graph(s), comparability 71 Graph(s), concurrence in graphs 21 Graph(s), incidence in graphs 21 Hall — Ore theorem 40 50 63 64 72 184 185 214 Hall — Rado condition 93 Hall's condition 26 27 55 Hall's condition 184 185 Hall's theorem 27 38 39 87 104 111 212 214 215 Hall's theorem, defect form of 40 50 Hall's theorem, deltoid form of 58 Hall's theorem, dual of 30 58 Hall's theorem, extensions of 44—48 Hall's theorem, transfinite form of 56 71 72 Hall, M.Jr. 38 56 72 88 128 212 216 239 Hall, P. 24 26 27 38 40 104 167 184 214 215 239 Halmos, P.R. 13 23 38 51 71 72 73 239 Halperin, I. 212 237 Hammer, P.L. 38 239 Harary, F. 23 146 239 Hardy, G.H. 88 212 239 Harper, L.H. 218 239 Henkin, L. 72 240 Hereditary structure 90 Heron, A.P. 145 Higgins, P.J. 74 88 240 Higgs, D.A. 225 240 Hoffman, A.J. 51 72 106 162 167 168 170 175 212 218 228 237 240 Horn, A. 146 240 Incidence in graphs 21 Incidence in matrices 183 Incidence matrix 76 184 Incidence matrix, formal 101 110 184 Incidence-bound collection 42 Incidence-free collection 42 inclusion 2 Inclusion, strict (proper) 2 Incomparable elements 17 Independence structure (space) 90 110 142 143 Independence structure (space), bases of 119—123 128 Independence structure (space), characterization of 99 Independence structure (space), models of 125—127 129 161 Independence structure (space), relation to linear structures 116 128 Independence structure (space), relation to transversal structures 102 103 128 Independence structure (space), restriction of 91 Independence structure (space), sum of independence structures 130 Independent, (partial) transversal 93 95—97 100 110 Independent, set 90 Index set 5 Infinity lemma, Koenig's 71 231 Ingleton, A.W. 111 118 128 233 240 Injection 3 Insertion theorems 36 49 159 167 172 intersections 2 7 Inverse mapping 4 Isbell, J.R. 213 240 Isotone mapping 9 Jackson, D.E. 212 238 Jacobson, N. 72 73 240 Jung, H.A. 59 72 221 Kaplansky, I. 88 238 Kellerer, H.G. 213 240 Kelley, J.L. 23 240 Kertesz, A. 128 129 240 Klarner, D.A. 230 Knaster, B. 23 240 Kneebone, G.T. 23 244 Kneser, H. 23 240 Koenig's Infinity Lemma 71 231 Koenig's theorem on graphs 22 23 30 145 217 Koenig's theorem on matrices 188 191 212 214 217 218 Koenig, D. 22 23 38 71 167 182 188 191 211 212 214 217 218 231 240 241 Koopmans, T.C. 211 241 Kreweras, G. 176 241 Kuhn, H.W. 50 51 106 162 167 168 170 175 218 228 240 241 Kuratowski's lemma 19 Kx 47 Latin rectangles 81—84 88 Latin squares 81—84 Lazarson, T. 110 128 241 Lindstroem, B. 233 Line (of a matrix) 183 Line-finite matrix 200 Line-sum 183 Linear programming 51 167 212 218 Linear structure 112—118 128 Linear structure, relation to independence structures 116 128 Linear structure, relation to transversal structures 113 114 116 128 Link (of two families) 147 Linked elements 33 Linked sets 34 36 43 Linking principle, Fulkerson's 206 213 216 Linking principles 216 Littlewood, J.E. 88 212 239 Local choice function 52 Locally finite deltoid 33 Loewig, H. 72 241 Lubel, D. 19 241 Lundina, D.S. 71 241 Luxemburg, W.A.J. 71 241 Maak, W. 38 167 212 241 MacLane, S. 110 128 241 Mann, H.B. 51 88 150 242 Mapping 3 Mapping theorems 9—12 Mapping, admissible 33 Mapping, bijective 3 Mapping, injective 3 Mapping, inverse 4 Mapping, isotone 9 Mapping, restriction of 3 Mapping, surjective 3 Mappings, direct sum of 4 Mappings, product (composition) of 4 Marcus, M. 213 242 Marginal elements 46 51 105 106 111 156 167 Mason, J.H. 110 146 225 242 Matrix, associated with a family 183 Matrix, contained in another 199 Matrix, doubly-stochastic (d.s.) 183 185 192—204 213 Matrix, formal incidence 101 110 184 Matrix, incidence 76 184 Matrix, line-finite 200 Maximal admissible set 33 Maximal element 17 Maximal independent set 91 Maximal subfamily 34 123 181 182
Реклама
 |
|
О проекте
|
|
О проекте




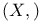 , (X,<)
, (X,<) 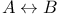
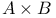
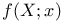
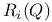 ,
, 

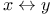
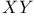
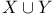 ,
, 
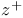
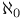
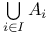 ,
, 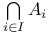

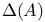 ,
, 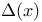
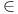 ,
, 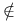
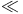
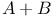

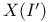
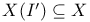
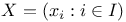
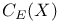 ,
, 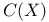 ,
, 
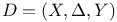
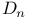


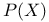
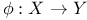
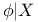

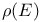
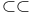
 ,
,  ,
, 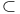

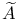
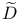
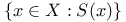
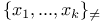
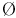
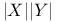 , |X|<|Y|
, |X|<|Y|