Авторизация
Поиск по указателям
Neusel M.D. — Invariant Theory of Finite Groups
Обсудите книгу на научном форуме Нашли опечатку?
Название: Invariant Theory of Finite GroupsАвтор: Neusel M.D. Аннотация: The questions that have been at the center of invariant theory since the 19th century have revolved around the following themes: finiteness, computation, and special classes of invariants. This book begins with a survey of many concrete examples chosen from these themes in the algebraic, homological, and combinatorial context. In further chapters, the authors pick one or the other of these questions as a departure point and present the known answers, open problems, and methods and tools needed to obtain these answers. Chapter 2 deals with algebraic finiteness. Chapter 3 deals with combinatorial finiteness. Chapter 4 presents Noetherian finiteness. Chapter 5 addresses homological finiteness. Chapter 6 presents special classes of invariants, which deal with modular invariant theory and its particular problems and features. Chapter 7 collects results for special classes of invariants and coinvariants such as (pseudo) reflection groups and representations of low degree. If the ground field is finite, additional problems appear and are compensated for in part by the emergence of new tools. One of these is the Steenrod algebra, which the authors introduce in Chapter 8 to solve the inverse invariant theory problem, around which the authors have organized the last three chapters. The book contains numerous examples to illustrate the theory, often of more than passing interest, and an appendix on commutative graded algebra, which provides some of the required basic background. There is an extensive reference list to provide the reader with orientation to the vast literature.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 2001Количество страниц: 371Добавлена в каталог: 28.11.2010Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Example, 56 Example, 38 64 223 Example, 7 39 43 147 223 Example, 224 Example, 226 Example, 46 50 213 216 Example, 208 Example, 65 161 173 Example, 164 Example, 226 Example, 80 Example, 80 213 Example, 230 Example, 230 Example, 213 Example, 104 Example, 273 Example, 213 Example, 215 Example, 215 Example, 110 Example, 216 Example, 54 Example, 131 Example, 82 94 98 216 274 Example, 173 Example, 9 225 Example, 5 35 Example, alternating group 5 Example, dihedral group 82 Example, Frobenius group 280 Example, orthogonal groups 308 Example, special orthogonal groups 308 Example, Stong's 164 Example, symmetric group 5 Examples, 207 Excess 237 Exponent sequence 69 Extended ideal 8 Extension 268 Exterior algebra 116 E[V] 116 Factorial 25 Faithful 5 Feshbach's transfer theorem 40 42 169 268 Feshbach, M. 171 Field of fractions 25 26 105 106 107 242 Fine Chern classes 91 92 Fine orbit Chern classes 16 98 Finite extension 31 Finite length 118 Finite p-group 102 103 Finite symplectic group 276 Finite type 45 316 Finite, noetherian 14 Finiteness, algebraic 12 Finiteness, combinatorial 14 Finiteness, homological 13 First Fundamental Theorem 25 First Main Theorem of Invariant Theory 86 Fixed point freely 11 Fixed-point set 173 Flag 164 Flag of subspaces 110 Flat 320 Forms 2 Forms, as functions 3 Fractal of the Dickson algebra 270 278 Fractal property 155 271 Fractal property of the Dickson algebra 271 Fractal property of the Steenrod algebra 271 Free R-module on a set 57 Freyd's adjoint functor theorem 284 Frobenius homomorphism 21 227 Frobenius map 271 Frobenius subgroup 280 Fundamental class 124 194 Fundamental theorems 24 G-invariant configuration 103 G-set 57 G-stable 6 Galois embedding theorem 245 Galois field 2 Generalized Landweber — Stong conjecture 254 Generalized quaternion group 131 173 Generalized quaternion groups 217 Global dimension 118 Global dimension d 118 Goebel's bound 74 149 Goebel's Theorem 46 69 73 Goebel, M. 18 Going down 323 Going up 323 Good primes 187 Gorenstein 54 213 Gorenstein ring 146 Gorenstein rings 143 Graded algebra 316 Graded algebra of maps 287 Graded algebra of polynomial functions 2 Graded complete intersection 68 Graded field 105 Graded field of fractions 105 317 Graded module 317 Graded vector space 316 Grading, negative 248 Gradings 315 Grassmann variety 269 Group action 1 Group algebra 6 Group of odd order 27 Height 42 Herbrand's lemma 178 Higher center 209 Higher-order differential operator 229 Hilbert function 14 Hilbert ideal 9 167 Hilbert — Serre theorem 66 67 Hilbert's basis theorem 1 30 Hilbert's Nullstellensatz 1 326 Hilbert's syzygy theorem 1 118 hom-codim(-) 139 hom-dim-(-) 118 Homological algebra 13 Homological codimension 19 129 173 Homological degree 116 Homological dimension 118 Homological finiteness 13 Homological properties 278 Hopf algebra 231 Hyperplane 156 186 Hyperplane of a pseudoreflection 186 Hyperplane of a transvection 156 Hypersurface 146 310 Icosahedral group 54 191 220 Ideal of stable invariants 11 Ideal, maximal 322 Ideal, primary 322 Idempotent 294 Ifp-basis 102 Ifp-dimensions 102 Imbedding property 242 Imbedding theorem 245 270 278 291 Indecomposable elements 318 INDEX 33 Index sequence 234 Injective hull 247 248 251 Integer representation 221 Integral extension 31 320 Integral extensions and the functor T 292 Integrally closed 25 Internal degree 117 174 Intertwining action 287 Invariant ideal 23 259 260 Invariant ideals 23 Invariant prime ideal spectrum 266 Invariants of 160 inverse invariant theory problem 22 Irreducible ideal 143 Irreducible representation 75 Irredundant primary decomposition 322 Isolated prime 322 Isometries of a square 82 Isometries of the square 127 Isotropy group 35 57 60 Isotropy subgroup 75 308 Isotypic component 6 Iterated fixed-point filtration 101 Iterated fixed-point length 101 Iterated fixed-point set 101 Ith polarized Chern class 87 Ith polarized elementary symmetric polynomial 87 Jacobian determinant 126 194 Jordan block 161 Jordan canonical form 160 Kaehler differentials 303 Koszul complex 114 116 117 173 250 Koszul complex, modified 178 Koszul's Theorem 117 Krull dimension 25 42 115 324 Krull dimension and the functor 292 297 Krull relations 268 320 322 Kth doubly polarized Chern class 91 Lam's 260 Landweber — Stong conjecture 246 247 283 Landweber's Theorem 270 Landweber, P.S. 158 Lannes — Zarati structure theorem 249 Lannes's 284 Lasker — Noether Theorem 1 268 322 Leibniz rule 35 Length 234 Local cohomology 176 Local cohomology spectral sequence 176 Lying over 323 Lying-over 271 Macaulay's theorem 278 Maximal 322 Maximal ideal 322 Maximal regular sequence 129 Minimal polynomial 203 Minimal resolution 121 MOD/A 118 Modified Koszul complex 178 Modular case 2 Modular invariant theory 151 Module of indecomposable elements 120 Molien's theorem 46 49 moment 234 Monic polynomial 31 Monomial 3 Monomial basis 59 Moore, J.C. 315 Morphism of degree d 316 Multiindex 3 Multiplicity 6 63 Multiplicity function 63 Multipolarized Chern classes 91 92 Multipolarized elementary symmetric polynomials 92 Multiset 63 n-fold Cartesian product 61 Nakajima — Stong Theorem 109 Nakajima's Theorem 307 Nakajima, H. 159 164 Nakayama's lemma 318 Negatively graded 248 Newton's formula 81 Nilpotent group 210 Nilpotent groups 23 Nilradical 255 Noether map 29 85 Noether normalization 324 Noether Normalization Theorem 14 323 Noether problem 26 Noether's bound 17 36 149 Noether's finiteness theorem 31 Noetherian 25 Noetherian finiteness 14 Noetherian module 30 Noetherian ring 30 Noetherianess 290 291 294 Nonassociates 27 Nondiagonalizable pseudoreflection 156 Nonmodular case 2 Nonnegatively graded 45 Norm 79 Normal extension 323 Octahedral group 220 Optimal system of parameters 18 Orbit 1 57 Orbit Chern classes 16 78 255 Orbit polynomial 78 Orbit sum 58 Orbit sums 58 Order of the pole 69 Orthogonal group 308 309 P(M,t) 45 p-group 27 210 p-groups 23 152 160 193 p-Sylow subgroup of 110 p-Sylow subgroup of 110 Palindromic polynomial 145 Parabolic Group 216 Parameter ideal 143 Permutation invariants 69 Permutation representation 8 17 23 46 57 149 Pigeonhole Principle 207 Poincar series 46 Poincar'e duality algebra 194 Poincar'e series 67 Poincare duality algebra 124 126 Poincare series 14 45 57 59 66 Pointwise stabilizer 20 146 192 283 288 289 307 Polar axis 54 191 192 Polarized Chern classes 213 Polarized elementary symmetric polynomials 87 Poles 54 Polynomial algebra 2 146 164 Polynomial algebra problem 19 Polynomial algebras and the 302 Polynomial functions 2 4 Positively graded 45 Pre-Euler class 104 213 267 Primary decomposition 320 Primary ideal 322 Prime avoidance lemma 320 Prime field 49 Prime Filtration Lemma 330 Prime ideal spectrum 259 Primitive derivation 244 Principal ideal 35 Proj(-) 259 proj-dim_(-) 118 Projective 10 320 Projective dimension 18 25 118 Projective resolution 13 174 Pseudo-optimal 18 Pseudoreflection 51 124 186 Pseudoreflection group 186 193 Pseudoreflection groups 186 194
Реклама
 |
|
О проекте
|
|
О проекте




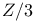
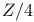
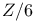
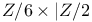
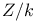
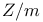
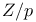

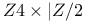
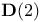
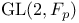
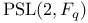
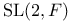

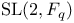
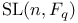
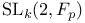


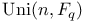
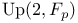
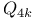

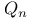
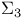


 -construction
-construction