Àâòîðèçàöèÿ
Ïîèñê ïî óêàçàòåëÿì
Weinberg S. — The Quantum Theory of Fields. Vol. 1 Foundations
Îáñóäèòå êíèãó íà íàó÷íîì ôîðóìå Íàøëè îïå÷àòêó?
Íàçâàíèå: The Quantum Theory of Fields. Vol. 1 FoundationsÀâòîð: Weinberg S. Àííîòàöèÿ: In The Quantum Theory of Fields, Nobel Laureate Steven Weinberg combines his exceptional physical insight with his gift for clear exposition to provide a self-contained, comprehensive, and up-to-date introduction to quantum field theory.
ßçûê: Ðóáðèêà: Ôèçèêà /Êâàíòîâàÿ òåîðèÿ ïîëÿ /Ó÷åáíèêè /Ñòàòóñ ïðåäìåòíîãî óêàçàòåëÿ: Ãîòîâ óêàçàòåëü ñ íîìåðàìè ñòðàíèö ed2k: ed2k stats Ãîä èçäàíèÿ: 1996Êîëè÷åñòâî ñòðàíèö: 609Äîáàâëåíà â êàòàëîã: 09.10.2005Îïåðàöèè: Ïîëîæèòü íà ïîëêó |
Ñêîïèðîâàòü ññûëêó äëÿ ôîðóìà | Ñêîïèðîâàòü ID
Ïðåäìåòíûé óêàçàòåëü
Hepp, K. 512 532 Herzberg, G. 45 Heydenberg, N. 167 Hibbs, A. R. 423 Hilbert space, defined 49 Hocking, J. G. 106 Hoddeson, L. 39 40 46 167 Hole theory 12 29 31 Homotopy groups and classes 89—90 96—100 419—20 Homotopy groups and classes, defined 100 Howard, J. C. 470 Hubbard — Stratonovich transformation 461 Hubbard, J. 461 470 Huber, A. 596 Huff, R. W. 596 Hugenholtz, N, M. 178 790 Hypercharge 123 Hyperons 123 Hyperons, discovered 30 Induced emission of photons 18 Induced representations 65 Infield, L. 46 Infinities (see “Ultraviolet divergences” “Infrared Infrared divergences 32—3 491 496 539—53 Inoenue — Wigner contraction 62 Inoeu, T. 45 Inonue, E. 62 105 Interaction picture 143 287 Interaction picture for derivative coupling 318—20 Interaction picture for Dirac field 323—5 Interaction picture for electrodynamics 353 Interaction picture for interacting scalar field 304—5 Interaction picture for vector field 320—3 Internal symmetries 121—2 Invariant subgroups and subalgebras 70 88 Irreducible representations, defined 64 Irrelevant couplings 503 Isospin (isotopic spin) 123 Israel, W. 533 Ito, D. 45 Jackiw, R. 40 424 Jackson, J. D. 471 Jacobi identity 83 Jaffe, A. 423 Jammer, M. 39 Jauch, J. M. 257 Jets 550 552 Johnson, W. R. 596 Joliot, F. 45 Joos, H. 257 Jordan, P. 3 10 15 16 17 18 19 23 25 40 41 431 292 Jost, R. 258 Jouvet, B. 470 Julian, R. S. 48 K mesons 123 132—3 K mesons, discovered 30 Kabir, P. 530 532 Kaellen, G. 457 470 Kaellen-Lehmann representation 457—62 Kahn, B. 48 Kaiser, G. 257 Kamefuchi, S. 424 532 Kanesawa, S. 48 Kemmer, N. 31 46 Kinetic theory 150—1 Kinosbita, T. 563 596 Klein — Gordon — Schrodinger equation 1 13 21 Klein, O. 4 7 13 25 27 29 41 44 200 211 239 277 368 375 Koba, Z. 46 48 Kockel, B. 31 46 533 Kollath, R. 168 Kramers degeneracy 81 Kramers — Kronig relation 469 Kramers, H. A. 35 47 81 106 168 469 471 Kroll, N. M. 38 47 593 596 Kubo, R. 190 Kusch, P. 48 Lagrangians 20 297—306 Lagrangians for complex scalar field 21 Lagrangians for interacting scalar field 302 Lagrangians integration by parts 305—6 Lagrangians irregular 326 Laidlaw, M. G, G. 418 424 Lamb shift 34—6 484 578—94 Lamb, W. E. 35 36 38 47 48 593 596 Land 41 Landau, L. D. 106 168 418 452 Lang, S. 424 Langer, J. S. 596 Lattes, C. 30 Lederman, L. 106 167 Lee — Nauenberg theorem 549—53 Lee, T D. 106 127 132 167 178 189 228 424 549 563 Legendre transformations 297 301 Lehmann, H. 438 457 463 470 Leinaas, J. M. 424 Lepton number 122 530 Leptons 472 Leutwyler, H. 533 Lewis, H. W. 46 Lie groups 53—5 Liebfried, D. 596 Lifshitz, E. M. 106 168 linear polarization 359 Lippmann — Schwinger equation 111 142 Lippmann, B. 111 166 Little groups 64-6 Little groups, 69—73 Little groups, 68—9 Liu, H. H. T. 498 Local Symmetries 342 (also see “Gauge transformations”) Local symmetries for different momenta 65—6 Local symmetries for massless particles 248 Local symmetries, defined 64 London, F. 375 Loops 186—7 270 282—3 358 413 Lorentz (or Landau) gauge 212 346 418 Lorentz transformations action on creation operators 177 Lorentz transformations action on general states 57 Lorentz transformations action on one-particle states 62—73 Lorentz transformations in canonical formalism 314—18 Lorentz transformations in off-shell matrix elements 427 Lorentz transformations in perturbation theory 144—5 277—9 259 Lorentz transformations of cross-sections and decay rates 138 Lorentz transformations of S-matrix 116—21 Lorentz transformations, defined 55—7 Lorentz transformations, homogeneous Lorentz group, defined 57 Lorentz transformations, homogeneous Lorentz group, general representations 229—33 Lorentz transformations, Poincare algebra 58—61 Lorentz transformations, Poincare group, defined 57 Lorentz transformations, proper orthochronous Lorentz group 58 Low, F. E. 291 556 563 LSZ theorem (see “Reduction formula”) Ludwig, G. 40 Lueders, G. 245 257 Lundeen, S. R. 596 Lyubarski, G. Ya. 257 M-matrix, defined 117 Mackey, G. W. 105 Magnetic moments of spin 1/2 particles 456—7 485—90 520 555—6 “Muon”) Mailer, C. 29 45 46 166 Majorana fermions 226 242 Mandelstam variables 515 Mandelstam, S. 424 Many time formalism 22 Marginal couplings 503 Marshak, R, E. 38 45 Maskawa, T. 329 330 338 Mass renormalization 439—42 473 495—6 587—9 Matrix mechanics 3—4 Matthews, P. T. 532 Maxwell equations 1 252 339 342 370 Mayers, D. F. 596 Mehra, J 39 42 Meller (electron-electron) scattering 29 Michelson, A. A. 41 Miller, A. L. 39 44 498 Miyamoto, Y. 47 Momentum operator 61 310—11 Muon 38 Muon, discovered 30 Muon, magnetic moment 489—90 520 Muonic atoms 483—4 Myrheim, J. 424 Nafe, J. E. 48 Nakajima, H. 329 330 338 Nappi, C. R. 257 Nash, C. 106 Nauenberg, M. 549 563 Neddermeyer, S. H. 30 45 Negative energy ‘states’ 10—14 23—7 567 Nelson, E. B. 48 Neutron 29 Neutron, neutron scattering on complex nuclei 157 160 Neutron-proton scattering 158 Newton, R. G. 166 Ne’eman, Y. 123 167 Nishina, Y. 29 44 368 375 Noether’s theorem 307 Non-compact groups (see “Compact and non-compact groups”) Non-linear 337 377 392—3 Nordheim, L. 30 45 Nordsieck, A. 33 46 562 Normal ordering 175 200 262 Novikov, V. 533 Nuclear forces 29—30 434—6 Occhialini, G. P. S. 13 30 45 Ohnuki, Y. 424 Old-fashioned perturbation theory (see “Perturbation theory”) Omnes, R. 471 One-particle-irreducible, defined 439 Oppenheimer, J. R. 12 23 27 28 29 30 31 35 37 38 43 44 45 46 Optical theorem 148 Osterwalder — Schrader axioms 384 424 Osterwalder, K. 424 Overlapping divergences 511—15 p-forms 369—72 Pachucki, K. 596 Pair production 29 Pais, A. 39 132 167 Pancini, E. 30 45 Parasiuk, O. 512 532 Parastatistics 420 Parity and space inversion (P) of bound electron states 568—9 586 Parity and space inversion (P), accidental symmetry 521 530—1 Parity and space inversion (P), defined 58 (see also “Specific particle types”) Parity and space inversion (P), intrinsic parities 125—7 (see also “Specific particle types”) Parity and space inversion (P), non-conservation in weak interactions 127 (see also “Specific particle types”) Parity and space inversion (P), transformation of 74—6 (see also “Specific particle Types”) Parity and space inversion (P), transformation of creation operators 177 (see also “Specific particle types”) Parity and space inversion (P), transformation of Dirac fields 221 224—5 Parity and space inversion (P), transformation of general irreducibie fields 239—40 (see also “Specific particle Types”) Parity and space inversion (P), transformation of one-particle states 76—9 103 Parity and space inversion (P), transformation of scalar fields 205—6 (see also “Specific particle types”) Parity and space inversion (P), transformation of vector fields 213 (see also “Specific particle types”) Partial wave expansions 151—9 Paschen, F. 41 Pasternack, S. 47 Path integrals 259 376—7 524 Path integrals for derivative coupled scalars 391—2 Path integrals for fermions 399—13 Path integrals for massive vector fields 393—5 Path integrals for non-linear 392—3 Path integrals for quantum electrodynamics 413 18 Path integrals for S-matrix 385—9 Path integrals Lagrangian version 389—95 Path integrals used to derive Feynman rules 395—8 Path integrals, derivation 378—84 Pauli matrices, defined 217 Pauli term 14 517 520 Pauli — Villars regulator 486 494 Pauli, W. 3 11 14 19 20 21 22 23 24 26 28 31 40 42 45. 47 245 257 292 494 495 517 Peierls, R. E. 44 46 47 168 Pendleton, H. 563 Perlmutter, A. 257 Perturbation theory, old-fashioned 142 259 549 564 585—6 593 Perturbation theory, time-dependent 142—5 (also see “Dyson series” “Feynman “Path Petermann, A. 498 Phase shifts 129—30 155 Phase space factors 139—41 Photon 3 Photon, charge conjugation phase 229 Photon, helicity and polarization 73—4 250—1 359—61 368 Photon, masslessness 343 452 477 Photon, photon-photon scattering 32 509 523—5 Photon, propagator 353—5 (also see “Soft photons” “Quantum Piccioni, O. 30 45 167 Pion-nucleon coupling constant 435 Pions 13 38 435—6 521 Pions, 131 Pions, 127 7 Pions, intrinsic charge-conjugation phase of 131 229 Pions, intrinsic parity 127 Pions, prediction and discovery 30 Pions, scattering on nucleons 463 469 Pipkin, F. M. 596 PJaczek, G. 168 Podolsky, B. 22 44 Poincare group and algebra (see “Lorentz transformations”) Poincare‘s theorem 99 370 Poisson brackets, defined 327 Polar decomposition theorem 88 530 Polarization, massive spin one particle 209—10 212 Polarization, masslessness 343 Polarization, photon 73—4 Polchinski's theorem 526—8 Polchinski, J. 424 526 533 Poles in scattering amplitudes 428—36 554 564 Pomeranchuk's theorem 468 Pomeranchuk, I. Ja. 468 469 477 Popov, V. N. 377 424 Positivity of energy 75—6 302 Positron 12—13 567—8 585 Positronium 227 Powell, C. F. 30 45 Power counting theorem 505 Present, R. S. 767 Primary constraints (see “Constraints”) Principal value function 113 Probabilities in quantum mechanics 7 27—8 33 50 Projective representations 53 81—91 96—100 Propagators 262 274—80 397—8 506 574—8 Proton charge 445 Proton charge radius 38 594 Proton form factors 457 Proton identified as holes 12 Proton in nuclei 29 PT-non-conservation 130—1 Quantum chromodynamics 123 531 549 Quantum electrodynamics 29 31—8 339—62 413—18 472—97 503 507—15 529—31 564—94 “Electron” “Photon” “Gauge “Ultraviolet “Infrared Quantum fields, Dirac 23—4 219—29 Quantum fields, early theory 15—31 Quantum fields, free fields 191—200 Quantum fields, general quantum fields 233—44 Quantum fields, massless particles 246—55 Quantum fields, redefinitions 331—2 Quantum fields, scalar 21—2 24—8 201—6 Quantum fields, uniqueness of irreducible fields 238 Quantum fields, vector 207—12 quantum mechanics 49—50
Ðåêëàìà
, A. 
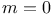
-model 
and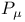 
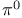decay 
decay 
|
|
Î ïðîåêòå