Àâòîðèçàöèÿ
Ïîèñê ïî óêàçàòåëÿì
Weinberg S. — The Quantum Theory of Fields. Vol. 1 Foundations
Îáñóäèòå êíèãó íà íàó÷íîì ôîðóìå Íàøëè îïå÷àòêó?
Íàçâàíèå: The Quantum Theory of Fields. Vol. 1 FoundationsÀâòîð: Weinberg S. Àííîòàöèÿ: In The Quantum Theory of Fields, Nobel Laureate Steven Weinberg combines his exceptional physical insight with his gift for clear exposition to provide a self-contained, comprehensive, and up-to-date introduction to quantum field theory.
ßçûê: Ðóáðèêà: Ôèçèêà /Êâàíòîâàÿ òåîðèÿ ïîëÿ /Ó÷åáíèêè /Ñòàòóñ ïðåäìåòíîãî óêàçàòåëÿ: Ãîòîâ óêàçàòåëü ñ íîìåðàìè ñòðàíèö ed2k: ed2k stats Ãîä èçäàíèÿ: 1996Êîëè÷åñòâî ñòðàíèö: 609Äîáàâëåíà â êàòàëîã: 09.10.2005Îïåðàöèè: Ïîëîæèòü íà ïîëêó |
Ñêîïèðîâàòü ññûëêó äëÿ ôîðóìà | Ñêîïèðîâàòü ID
Ïðåäìåòíûé óêàçàòåëü
127 130—1 29 207 159—60 207 88 165 202 276 225 227 165 225 227 469 127 Aaron, R. 470 Abelian groups, defined 55 Absorption of photons 18 Accidental symmetries 529—31 action 299 307 Aharony, A. 168 Aitken, A. C. 106 Alpha decay 160 Amado, R. D. 470 Anderson, C. D. 12 30 43 45 Annihilation and creation operators 16 19—20 23—4 26—8 169 173 Anomalous Zeeman effect 5 Antiparticles 13—14 23—8 104 149—50 199 567 Antiunitary and antilinear operators, defined 51 Anyons 420 Aramaki, S. 39 Argyres, P. C. 257 Artin, E. 424 Auxiliary fields 302—3 314 Axial gauge 346 Bagger, J. 338 532 Bailey, J. 498 Bakamijian, B. 189 Bargmann, V. 106 Baryon number, defined 122 Belinfante tensor 316 Belinfante, F. J. 44 316 338 Berezin integration, defined 403 Berezin, F. A. 403 404 424 Bergmann, P. G. 335 Beta decay 23 29 127 146 228 519 Bethe — Salpeter equation 560 Bethe, H. 29 33 36 45 47 560 563 593 596 Beyer, R. T. 39 43 Bhabha (electron—positron) scattering 29 Bhabha, H. J. 29 45 Bjorken, J. D. 532 Blackett, P. M. 13 Bloch, F. 33 46 562 Bogoliubov, N. N. 512 532 Bohr, N. 3 11 19 32 42 44 168 198 256 Boltzmann H-theorem 151 Boosts, defined 61 Born approximation 115 156 Born, M. 3 15 16 17 18 19 23 25 40 42 43 46 115 166 292 Bose — Einstein statistics 11 Bound states (see “Composite particles”) BPHZ prescription 512—13 Braid group 420 Breit — Wigner formula 162—3 Breit, G. 36 48 162 167 168 Bremstrahlung 29 Broken symmetry 443 451 Brown, G. E. 596 Brown, L. 39 40 45 46 167 532 Burgoyne, N. 257 Butler, C. C. 30 45 123 167 Canonical commutation and anticommutation relations 16 19—22 293—8 529 Canonical transformations 329 Cao, T. Y. 39 Carlson, J. F. 29 30 32 45 Cartan, E. 256 Casimir effect 297 Casimir, H. B. G. 338 Cassen, B. 167 Causality 145 198 463 Center-of-mass frame 139 Central charges 83 Chadwick, J. 45 Charge (see “Electric charge”) Charge conjugation (C) for photons 427—8 (also see “Specific particle types”) Charge conjugation (C), accidental symmetry 521 530—1 Charge conjugation (C), defined 121 131—2 Charge conjugation (C), intrinsic charge conjugation phases 131 (also see “Specific particle types”) Charge conjugation (C), non-conservation 132 (also see “Specific particle types”) Charge conjugation (C), transformation of creation operators 177 (also see “Specific particle types”) Charge conjugation (C), transformation of Dirac fields 226—7 (also see “Specific particle types”) Charge conjugation (C), transformation of fermion bilinears 229 (also see “Specific particle types”) Charge conjugation (C), transformation of general irreducible fields 241—2 (also see “Specific particle types”) Charge conjugation (C), transformation of scalar fields 206 (also see “Specific particle types”) Charge conjugation (C), transformation of vector fields 213 (also see “Specific particle types”) Chew, G. 47 471 Chew-Frautschi plot 469 471 Chinowsky, W. 167 Chiral transformation 520 Christenson, J, H. 106 167 circular polarization 359 Clebsch—Gordan coefficients 124 152 154 156 233—4 242 569 Clifford aigebra 214 Closed p-forms, defined 369 Cluster decomposition principle 169 177—89 197 259 Cohen, R. S. 44 256 Coherent states 189 Collins, R. D. B. 471 532 COLOR 549 Compact and non-compact groups 231 Composite particles 110 461—2 Compton (electron-photon) scattering 29 362—9 Compton, A. H. 41 362 364 504 Condon, E. U. 167 Connected amplitudes 178—82 270 282 286 389 413 Conservation laws for angular momentum 118 (also see “Specific symmetries and conserved quantities”) Conservation laws for charge 119 199 427 Conservation laws for current 212 307 478 586 Conservation laws for energy and momentum 117—18 425—7 Conservation laws limitations 253 537—8 Constraints 325—31 Constraints in electrodynamics 344 346—7 Conversi, M. 30 45 Cosmic rays 29 123 Coulomb energy 350 353 355—6 560 Coulomb gauge 251 346—50 365 Counterterms in quantum electrodynamics 472—3 CP-invariance for degenerate multiplets 104 CP-invariance, non-conservation in 132—3 CPT-invariance 104 133 244—6 459 Creation operators (see “Annihilation and creation operators”) Crichton, J. R. 189 Cronin, J, W. 106 132 167 Cross sections, defined 137—9 Cross sections, high energy limit 158—9 Cross sections, partial wave expansions 155—6 Crossing symmetry 269 467 554 Cumulants 178 Curie-Joliot, I. 45 Dalitz Plot 141 Dalitz, R, H. 141 168 563 Dancoff, S. M. 33 34 46 Dangerous states 550—2 Darwin, C. G. 10 41 42 596 Davisson, C. J. 3 40 de Broglie, L. 3 40 de Kronig, R. 469 471 De Rham cohomology 370 De Witt, C. M. 377 418 424. Deans, W, M. 41 Decay rates, general formula 136—7 Dedijer, S. 257 Density matrix 360 Deser, S. 563 Desiderio, A. M. 596 DeWitt, B. S. 39 424 Differential forms (see “p-forms”) Diffraction scattering 148—9 158 Dimensional regularization 449 477—80 497 Dimensionality, of fields and couplings 502 519 525—7 Dirac brackets 328—31 332—7 Dirac brackets in electrodynamics 347—9 Dirac equation 1 6—14 225 565—72 Dirac matrices 8—9 214—19 Dirac matrices, slash notation, defined 358 Dirac matrices, traces 361 372—4 Dirac representation of homogeneous Lorentz group 213—19 Dirac, P. A. M. 4 5 6 7 8 9 10 11 12 13 14 18 19 22 23 24 27 28 29 32 33 34 39 40 41 42 43 44 46 47 49 105 200 213 218 256 292 325 328 329 330 335 345 376 424 457 470 489 565 566 567 596 Dispersion relations 460 462—9 Distorted wave Born approximation 146—7 Donoghue, J. F. 533 Dotted and undotted indices 230 Drell, S. D. 532 Dresden, M. 61 Drinkwater, J. W. 47 Druehl, K. 424 Duality 232 371 Dyson series 144 259—60 Dyson, F. J. 37 48 106 144 168 258 259 287 29/ 376 499 532 Eckart 153 156 162 165 Eclipsing binaries 368 Edmonds, A. R. 106 167 165 257 596 Effective field theories 499 523—5 Ehrenfest, P. 15 29 43 45 Einstein, A. 12 13 18 19 43 55 518 Electric charge 341 Electric charge radius, defined 493 Electric charge, conservation 122 537 Electric charge, renormalization 342 442—8 473 480—3 Electric dipole moments 81 521 Electron, charge radius 493 Electron, classical theory 31 369 496 Electron, magnetic moment 6 10 14 36 457 468 520 Electron, spin 6—9 Elliptic polarization 360 Elsasser, W. 3 40 Energy shifts of atomic states 31—2 574 Energy shifts of atomic states, ls energy shifts 594 (also see “Lamb shift” “Uehling “Muonic Energy-momentum tensor 310—12 entropy 151 Epstein, S. 46 Equivalence principle 537 Erickson, G. W. 498 Euclidean path integrals 384 Euler constant 479 497 Euler — Lagrange equations 300 Euler, H. 32 46 523 524 526 533 Exact p-forms 369 Excitation energies, in hydrogen 592—3 Exclusion principle 11 Exterior derivatives 369 External fields 266 287—90 412—13 556—62 572—8 Fabri, E. 168 Faddeev equations 188 Faddeev, L. D. 190 376 378 424 Feenberg, E. 167 168 Feinberg, G. 167 530 533 Feinberg, J. 335 Fermi — Dirac statistics 12 171—2 267—70 418—20 Fermi, E. 11 19 23 39 421 44 292 Ferretti, B. 767 Feynman diagrams 36—7 259—91 Feynman diagrams for electrodynamics 355—8 Feynman gauge 355 417 Feynman parameters 474 486 497 Feynman, R. P. 33 36 37 38 47 45 259 276 280 286 353 354 355 360 375 376 377 400 411 413 417 423 424 426 430 459 472 474 486 495 559 572 Field equations 200 211—12 239 Field renormalization 331—2 436—42 452 461 473 479 484 543—4 Field-translation-invariant scalar theory 521—3 Fields (see “Quantum fields”) Fierz, M. 20 40 44 46 47 257 Fine structure 4—6 570 Fine structure constant 2 5 First class constraints (see “Constraints”) Fitch, V. L. 106 133 167 Flanders, H. 106 375 Flavors (of leptons) 529 Floating cutoff 525—8 Fock, V. 22 23 41 44 375 Foley, H. M. 45 Forests 512—13 form factors 452—7 485—93 580—2 Frautschi, S. C. 471 562 Fredenhagen, K. 424 French, J. B. 35 38 47 593 596 Friedman, J. I. 106 167 Froehlich, H. 48 Froissart bound 159 Froissart, M. 168 Fukuda, H. 47 Functionals, notation 299 Furry's theorem 428—9 509 Furry, W. H. 23 27 28 32 44 46 428 470 Gaberdiel, R. 424 Galilean invariance 62 145 217—18 Gamow, G. 7 39 42 Garwin, R. 106 167 Gasser, J. 533 Gauge transformations 251—2 339—43 345 370 448—52 “Coulomb “Temporal “Axial “Unitarity “Feynman Gaussian Integrals 420—3 Gell-Mann, M. 123 132 167 291 477 556 563 General relativity 255 312 316 369 518—19 521 Generators of symmetries 307—14 Georgi, H. 257 Germer, L. H. 3 40 Gerstein, I. S. 424 Glimm, J. 423 Global symmetries, defined 307 Gluons 549 Goldberger, M. L. 166 463 471 556 563 Goldstone, J. 178 189 Gordon, W. 4 7 10 13 25 27 41 42 200 211 239 277 596 Goudsmit. S. 5 10 41 Grassmann variables, defined 401 Graviton 73—4 253 521 537 548 Green, M. B. 375 Grisaru, M. 563 Grotch, H. 596 Groups, defined 52 (also see “Abelian groups” “Homotopy “Lie “Little “Representations” “Semi-simple Gudehus, T. 257 Guersey, F. 106 Haag, R. 424 Haenscb, T. W. 596 Hafstad, L. D. 767 Hahn, Y. 532 Halter, J. 533 Hamiltonian for complex scalar field 22 Hamiltonian for Dirac equation 8 Hamiltonian for electrodynamics 349—50 Hamiltonian for free particles 176 Hamiltonian for interacting Dirac field 323 Hamiltonian for interacting scalar fields 199 302 Hamiltonian for interacting vector field 321 Hamiltonian for one-dimensional scalar field 15—17 Harvey, J. 533 Hawking, S. W. 533 Heisenberg picture 109 288 297 425 Heisenberg, W. 3 4 10 15 16 17 20 21 24 25 29 32 33 40 41 42 43 44 45 46 109 766 292 519 532 533 Heitler, W. 29 45 45 Helicity, defined 72 Helicity, limitations for massless particle fields 253—4 Helicity, limited to integers and half-integers 90
Ðåêëàìà
decay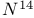 
molecular spectrum 
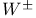particles 
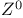particle 
group 
baryon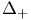 
function 
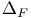function 
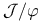meson 
meson 
-mesons 
problem 
decay 
|
|
Î ïðîåêòå