|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Berger M., Cole M. (translator) — Geometry I (Universitext) |
|
|
 |
| Предметный указатель |
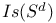 , finite subgroups of 18.5.10 , finite subgroups of 18.5.10
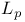 -spaces 11.8.11.12 -spaces 11.8.11.12
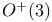 1.8.1 4.3.9.2 1.8.1 4.3.9.2
 , connectedness of 8.4.3 , connectedness of 8.4.3
Abelian group 1.4.4.1 8.3.3
Accumulation point 9.11.4
Acerbas 12.11.7
action see “Group action”
Action of permutations on cross-ratios 6.3
Acute angle 10.1.8
Acute triangle 9.4.1.3
Adjacent 12.1.12 12.1.8 12.8.6.2
Adjoint of an endomorphism 8.1.8.6 13.2.4
Aerial photography 4.7
Affine automorphism 2.3.1
Affine conic 16.7
Affine coordinates 2.2.9
Affine Euclidean spaces see “Euclidean affine spaces”
Affine form 2.4.8.3 11.8.7.2
Affine frame 2.2.9 3.2.5 4.4 2.2
Affine frame, convention about 2.2.8
Affine geometry vs. projective geometry 5.4.2
Affine geometry, fundamental theorem of 2.6
Affine geometry, shortcomings of 5.0.1
Affine group 2.3.3.4 1.2.7
Affine independent set 2.4.3
Affine isomorphism 2.3.1
Affine map see “Affine morphism”
Affine morphism 2.3 2.3.1 3.2 3.5
Affine polynomial 3.3.5
Affine quadratic form 3.3.6 15.2
Affine quadric 15.1.1 15.3 20.1.2
Affine space 2.1.1
Affine space, complex 2.8.7
Affine space, complexification of 7.6.2
Affine space, dimension of 2.1.8
Affine space, orientation of 2.7.2
Affine spaces, differential calculus in 2.7.7
Affine spaces, isomorphism between 2.2.6
Affine spaces, product of 2.2.2
Affine subspace 2.4.1 5.3
Affine subspaces, intersection of 2.4.2.4
Alexandroff 12.11.5.3
Alexandroff compactification 5.2.6 18.1.3.2 20.2.4
Algebra 0.2
Algebraic curves 16.4.9
Algebraic dual 0.2
Algebraic equations, solution by radicals 16.4.11.1
Algebraic geometry 4.0.5 4.2.6 12.7.5.1 17.1.6
Algebraic group 12.6.9
Algebraic hypersurface 14.1.4.2
Algebraic number field 13.1.4.5
Algebraic topology 4.0.5 4.3.7 4.3.9 12.7.5.3 II.86 18.8.7 19.1.3.3
Algebraic topology of orthogonal groups 8.10
Algebraic topology of projective spaces 4.3 4.8.2
Algebraic topology of the sphere 18.2.1
Algebraic varieties 14.1.6.2
Algebraically closed base field 6.4.2 6.5.1 6.7.2 6.7.4 13.4.3 13.5.3 14.1.5.3 14.1.6.2 16.2.10
Alhambra 1.7.1
Alternating bilinear form 2.7.2.1 14.8.12.2
Alternating group 0.2 1.8.4 6.3.2 12.5.5.6
Altitude of a spherical triangle 18.11.5
Altitude of a tetrahedron 10.6.6
Altitude of a triangle 9.4.1.3 10.1.4 10.13.1
Analysis 6.6 11.8.11.12 13.5.6 19.7.3
Analytic geometry 2.4.8 10.7.6 14.1.4 14.5.3 17.1.6
Analytic spheres 10.7.7 20.4.2
Angle between tangent vectors to a sphere 18.1.2.4
Angle of a rotation 1.7.5 9.3.4 9.3.5
Angle of an orientation-preserving similarity 9.6.3
Angle, dihedral 12.1.12
Angle, non-oriented 8.6
Angles and similarities 8.8.5
Angles in Artinian plane 13.8.5
Angles in Euclidean affine space 9.2.1
Angles in hyperbolic space 19.2.7
Angles of a spherical triangle 18.6.6
Angles of a triangle 10.1.2
Angles of a triangle, sum of 10.2.4
Angles of an elliptic triangle 19.1.8.1
Animals 12.5.5.7 11.86
Anisotropic 18.2.1 13.9.4
Antihomographies 18.10.2.4
Antir Euclidean 19.2.7
Appolonius 17.1.5 17.3
Appolonius’ formula. 9.7.6.1
Appolonius’ hyperbola 17.5.5.6
Appolonius’ problem 10.11.1
Appolonius’ Theorem 15.6.4
Approximation lemmas 12.9.2
Apulia, Italy II.22
Arc cosine 8.3.11 9.9.7.1 18.6.6
Arc of circle 8.7.5.4
Archimedean field 8.5.2
Archimedes 15.7.6
Architecture II.22 (see also “Shell architecture”)
Area 9.12.4.1 9.12.7
Area and Steiner symmetrization 12.10.10
Area of a compact convex set 12.10.2
Area of a triangle 9.7.3.8 9.12.4.4 10.1.5
Area of ellipse 17.7.5
Area of pedal triangles 10.4.5
Area of polytopes 12.8
Area of the sphere 12.10.4.1
Areas on the sphere 18.8.8.1
Areas, calculation of 9.12.6.3
Argument 8.7.9
Arithmetic 6.6 10.13.29 12.4.6 13.7.2 19.7.3
arrow 3.1.2
ART I.ix 1.7.1
Artin 13.7.2
Artinian plane 13.1.4.4 13.8 19.2.5
Artinian space 18.1.4.4
Artinian space, quadrics in 14.4
Associativity of barycenters of punctual masses 3.4.8
Associativity of the base field 2.5.5 5.4.4
Asteroids 17.2.1.7
Astroid 9.14.34.8 17.7.4
Astronomy II.146 18.6.1 18.6.11 18.6.13.8
Asymptote 15.5.11 17.8
Asymptotic cone 15.5.11
Atlas 4.2.1
automobile 18.6.11
Automorphic function 6.6.7
Axiomatic point of view 5.0.1
Axiomatization 2.6.7 2.5.5
Axiomatization of affine geometry 2.6.7
Axiomatization of Euclidean geometry 9.2.3
Axiomatization of projective geometry 4.5.12 4.6.7
Axis of a rotation 8.4.7.1 9.3.5
Axis of a screw motion 9.3.5
Axis of an ellipse 16.3.10.2
Axis of inertia 13.5.6
Bailing-out maxim 11.1.2.2
Balls, volume of 9.12.4.6
Barycenter 3.4.1 3.4.5 9.7.6 9.12.6.1 11.1.8.3
Barycenter coordinates 3.6.2 10.6.8 10.13.26
Barycenter subdivision 3.6.5 3.7.8
Barycenter, calculation of 3.4.6.6
Base field see “Associativity” “Commutativity” etc.
Base of a pencil 14.2.7.3
Basel cathedral 9.6.9 9.14.32
Basis of a complexification 7.1.3
Beltrami 19.7.2
Bernoulli, Jakob 9.6.9
Bernoulli’s tomb 9.6.9 9.14.32
Betti numbers 4.3.9.4
Bezout’s Theorem 16.4
Bicircular quartics 9.6.8
Bieberbach’s isodiametric inequality 9.13.8
| Bieberbach’s theorem 1.7.7.3
Bijections 0.2
Bilinear form 8.1.1 II.86
Billiards 9.4.1 9.14.9 17.9.2
Binet’s formula 17.2.1.7
Binomial coefficients 0.2
bisector 8.7.3.2 8.7.7.4 8.12.10 9.14.3 18.11.5 20.4.4 20.8.2
Bistochastic matrices 11.6.6.3 11.9.7
Bitangent conics 16.4.10.3
Blaschke Kugelungsatz 9.13.6 9.14.24
Blaschke rolling Theorems 12.12.14
Blaschke selection theorem 9.11.4
Blaschke — Lebesgue 12.10.5
Bolyai 19.7.2
Bonnesen’s theorem 12.11.9.4 12.12.15
Borsuk — Ulam, theorem of 18.2.7
Bound vector 2.1.3
box 9.12.4.2
Boy’s surface 4.3.9.1
Brahmagupta’s formula 10.13.7
Braid lemma 9.5.4.9
Brianchon’s theorem 16.2.13 19.3.5
British Museum 12.5.5.7
Bruhat — Tits lemma 9.8.6.5
Brunn — Minkowski theorem 11.8.8.1 12.11.3
Calculation in affine spaces 2.3.8 9.2.6
Calculation in projective spaces 4.2.1
Calculation of areas 9.12.6.3 12.10.11
Calculation of barycenters 3.4.6.6
Calculation of cross product 8.11.10
Calculation of cross-ratios 6.2
Calculation of duals 14.5.3
Calculation of projective morphisms 4.5.13
Calculation of quadrics 14.1.4
Calculation with affine polynomials 3.3.3
Calculation with vectors 3.1
Canonical density 8.11.8
Canonical measure of affine spaces 2.7.4.2
Canonical measure of elliptic spaces 19.1.1.7
Canonical measure of Euclidean affine spaces 9.12
Canonical measure of hyperbolic space 19.5.1
Canonical measure of spheres 12.3.2 18.3.7
Canonical norm 9.2.6.4
Canonical projection onto a projective space 4.1.1
Canonical topology of a projective space 4.8.1
Canonical topology of an affine space 2.7.1.1
Canonical volume 9.12
Canonical volume form 8.11.3 18.3.4
CAR 18.6.11
Caratheodory’s theorem 11.1.8.6 11.5.8 11.7.4.2
Cardboard polyhedra 12.1.3.2 12.8
Cardinality of a set 0.1
Cardioid 9.6.8 9.14.34.3 9.14.34.5
Caron, Joseph I.xi
Cartan — Dieudonne Theorem 13.7.12
Carthage 12.11.8
Cartography 4.7.5 18.1 18.1.5.4
Cartography of France 18.1.8.5
Cartography of Switzerland 18.1.8.3
Cash prize 10.6
Castel del Monte, Italy II.22
Castillon’s problem 10.11.4 16.3.10.3
Cauchy — Schwarz inequality 12.11.5.4
Cauchy’s formula 12.3.3 12.8.1 12.10.2 12.11.9.6
Cauchy’s Lemma 12.6.6.1 18.6.11 18.7.16
Cauchy’s Theorem 12.8.1
Caustic 9.14.34.3
Cayley 5.2.2 I.122 8.8.7.5 16.6.1 16.6.12.4
Cayley numbers see “Octonions”
Cayley — Menger determinant 9.7.3.1 9.7.4 9.14.23
Cayley’s formulas 17.6.7
Celestial charts 18.1.8.6
Cell decompositions 4.3.9.4
Center of a compact set 2.1.5.5
Center of a group 1.6.7.2
Center of a quadric 15.5.3
Center of a regular polygon 12.4.3
Center of a regular polytope 12.5.2.1
Center of a rotation 1.7.5 9.8.4
Center of a similarity 9.5.2
Center of a sphere 10.7.1
Center of an isometry 9.3.3
Center of curvature see “Curvature”
Center of mass 2.7.5.3 3.4.2 3.4.6.1 12.5.2.1
Center of the ortogonal group 8.2.16
Central field 8.1.2.2
Central quadric 15.5.3
Centrifugal "force" 17.5.5.5
Centroid 2.7.5.2 3.4.2 9.12.6 11.5.9.3 11.7.6
Ceva, theorem of 2.8.1 3.7.12 10.13.1.2
Chain of theorems 10.9.8 10.13.19
Change of variable theorem 0.6 2.7.4.2
Characteristic classes 14.3.7
Characteristic function 0.6
Chart 4.2.1 18.1.5.1 18.2.1 18.11.2
Chart, what you want in a 18.1.7
Charts in P(E) 4.5.13
Chasles circles 17.9.11
Chasles’s relation 2.1.5 8.7.2.4 10.9.7.2 16.6.1
Cheeger — Simons, conjecture by 18.3.8.6
Chinese lantern 9.12.7
chords 10.9.2.1
circle 8.3.3 8.12.4 9.4.4 10.7.1 11.6.1 18.1.8.1
Circle as a conic 17.4.2
Circle Limit II.342
Circle, classical problems on 10.11
Circles in hyperbolic space 19.3.3.2 19.6.8.2
Circles in the plane 10.9
Circular group 20.6.1
Circular sections 15.7.14
Circumscribed 12.5.2.1
Class formula 1.6.6 1.8.3.1
Classical geometry 2.7 I.200
Classical groups 16.3.9
Classification 1.6.5
Classification of affine quadrics 15.3
Classification of crystallographic groups 1.9.9
Classification of Euclidean affine quadrics 15.6.1
Classification of quadratic forms 13.1.4.1 13.4
Classification of quadrics 14.1.5
Clifford algebra 8.9.13 8.10.3 13.6.8
Clifford parallelism 4.3.7 18.8.1.2 18.8.4 18.9.3 18.11.17 19.1.4
Clifford parallelism, generalizations of 18.9.4.4
Clifford parallelogram 18.8.4
Closed ball 0.3
Closed convex hull 11.2.3
Closed hyperplane 2.1.3.2
Closedness of stabilizers 9.8.3
Cobordism 4.0.5
Cocoon 11.3.6.0
Cocube 12.1.2.5 12.5.4.3
Cogwheel design 9.14.34.6
Cohomology rings 4.3.9.4
Combinations 1.5.2
comets 17.2.1.7
Common perpendicular 9.2.6.5
Commutative algebra 16.4.9
Commutativity of base field 2.3.3.9 2.5.5 4.5.6 4.9.8
Compact convex set 9.12.7 12.9
Compact manifolds 4.0.5
Compact subgroups of GA(X) 2.7.5
Compactness of orthogonal group 8.2.3.3
Compactness of projective spaces 4.3.3
Compactness of sphere 18.2.1
Compactness of stabilizers 9.8.6
compass 10.8.3 10.9 10.11.2
Complementary affine subspaces 2.4.9.4
Complete quadrilateral 3.7.18 6.4.4 17.9.19
Completely faithful 5.1.3
Completely singular 13.2.1
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



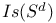 , finite subgroups of
, finite subgroups of 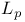 -spaces
-spaces 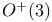
 , connectedness of
, connectedness of