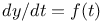|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Blanchard P., Devaney R.L. — Differential Equations |
|
|
 |
| Предметный указатель |
Predator-prey system, modified 157
Predator-prey system, pesticides 167
Predator-prey system, Runge — Kutta method 658
Predator-prey system, three species 525
Qualitative Methods 6 13 62
Qualitative methods, systems 164 181
Radioactive decay 16
Ramp function 579
RC circuit 44 61 568 577
RC circuit, constant input voltage 45
RC circuit, on-off input voltage 46
RC circuit, periodic input voltage 47
RC circuit, zero input voltage 45
Red Sox 299 1919—2003
Renormalization 699
Resonance 415
Resonance, Millennium Bridge 417
Restoring force 159
Return map 731; see Poincar return map return map
Riemann Sums 642
RLC circuit 375 391 447
Robo-lobster 513
Rock and Roll see Paul
ROPE 77
Round-off error see Finite arithmetic
Rubber band 229 448
Runge — Kutta method 649—658
Runge — Kutta method, definition 650
Runge — Kutta method, error 653
Runge — Kutta method, finite arithmetic 660
Runge — Kutta method, Mathematica program 655
Runge — Kutta method, order 653
Runge — Kutta method, step size 653
Runge — Kutta method, systems 657
Runge — Kutta method, TI calculator program 654
Runge — Kutta method, С program 656
Saddle 274 277 362
Saddle node bifurcation see Tangent
Saddle, connection 487
Saddle, nonlinear 463
Saddle, separatrix 287 463
Salvadori, Mario 208 433
Saving model 28
Sawtooth function 581
Second-order differential equation 161
Second-order differential equation, forced 383
Second-order differential equation, Hermite equation 739
Second-order differential equation, homogeneous 383
Second-order differential equation, Legendre equation 743
Second-order differential equation, linear 325
Second-order differential equation, nonhomogeneous 383
Second-order differential equation, power series 739
Second-order numerical methods 646
See Harmonic oscillator, Springs, hard 552
Seed 673
Sensitive dependence on initial conditions 703 706
separable equations 23—28
Separable equations, missing solutions 27
Separation of variables 20—32
Separatrix 287 463
Separatrix, stable 463
Separatrix, unstable 463
Shock absorbers 339
Shock absorbers, active 231 426
Shock absorbers, truck seats 231
Shock absorbers, washing machines 426
simple harmonic motion 331
Simpson’s rule 650
Singular matrix 242
Sink, first-order equation 86
Sink, linear systems 279 362
Sink, nonlinear 463
Sink, spiral 299 463
Sinusoidal forcing 397 413 421 589
Skyscraper, P — Delta effect 207
Skyscraper, swaying 206
Skyscraper, tuned-mass damper 509
Slope fields 36—43
Slope fields, sketching 37
Slope fields, special cases, 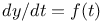 39— 40 39— 40
Slope fields, special cases, autonomous equations 40
Smale, Stephen 63
Sock absorbers see washing machines
Solution 6—7 20
Solution curve 155
Solution, asymptotic 82
Solution, checking 21 188
| Solution, domain of definition 66 82
Solution, existence and uniqueness 65
Solution, general 7 23 190
Solution, implicit 28
Solution, initial-value problem 7 22—23 162 188
Solution, linearly independent 355
Solution, missing 27
Solution, periodic 154 299
Solution, separatrix 287
Solution, system 13 154
Source, first-order equation 86
Source, linear systems 283 362
Source, nonlinear 463
Source, spiral 299 463
speed bumps 421
Spiral saddle 360
Spiral sink 299 463
Spiral source 299 463
Spring constant 160
springs 160
Springs, soft 552
Square wave 581
Stable equilibrium point 86 285
Stable separatrix 463
Steady-state response 387
Steady-state solution 120
Step function see Heaviside function
Step size 54
Straight-line solutions 267
Superposition, principle of see Linearity Principle
Suspension bridge 417 433 448
Suspension bridge, Lazer — McKenna light flexible long span 441
Suspension system model 339
systems 152
Systems, autonomous 181
Systems, checking solutions 188
Systems, coupled 12
Systems, decoupled 189
Systems, dimension 240
Systems, direction field 173
Systems, Euler’s method 198
Systems, Existence and Uniqueness Theorem 205
Systems, first-order 12
Systems, general solution 190
Systems, linear 237
Systems, Metaphor of the parking lot 175
Systems, nullcline 472
Systems, phase plane 156
Systems, phase portrait 156
Systems, Runge — Kutta method 657
Systems, three-dimensional 354—365
Systems, vector field 171
Tacoma Narrows bridge 433—440 448
Tangent bifurcation 692
Taylor polynomial 459
Taylor polynomial, power series methods 736
Taylor’s Theorem 457 631 641
Template, Lorenz 711
Tent function 716
Time-series 676
Trace 342
Trace-determinant plane 342
Trace-determinant plane, critical loci 347
Trapezoid Rule 642 650
Trolley cars 95
Truck seats 231
Tuned-mass damper 509
Undamped harmonic oscillator 330
Underdamped harmonic oscillator 331
Undetermined coefficients, method of 118 388
Unforced equation 383
Uniqueness Theorem, first-order equations, applications 70—73
Uniqueness Theorem, systems 205
Unstable equilibrium point 285 299
Unstable separatrix 463
van der Pol equation 201 452
Vector field 171 173
Volterra — Lotka system 482
Volterra, V. 13
Washing machines 426
Weather 214 539
Web diagram 683
WEEI 299
World Series 2004
wronskian 258
Wytham Woods 18
Xia, Zhihong 495
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 return map
return map