Àâòîðèçàöèÿ
Ïîèñê ïî óêàçàòåëÿì
Chaikin P.M., Lubensky T.C. — Principles of condensed matter physics
Îáñóäèòå êíèãó íà íàó÷íîì ôîðóìå Íàøëè îïå÷àòêó?
Íàçâàíèå: Principles of condensed matter physicsÀâòîðû: Chaikin P.M., Lubensky T.C. Àííîòàöèÿ: This book provides an overview of the physics of condensed matter systems. Assuming a familiarity with the basics of quantum mechanics and statistical mechanics, the book establishes a general framework for describing condensed phases of matter based on symmetries and conservation laws. After surveying the structure and properties of materials with different symmetries, it explores the role of spatial dimensionality and microscopic interactions in determining the nature of phase transitions. Particular attention is given to critical phenomena and renormalization group methods. The properties of liquids, liquid crystals, quasicrystals, crystalline solids, magnetically ordered systems and amorphous solids are investigated in terms of their symmetry, generalized rigidity, hydrodynamics and topological defect structure. In addition to serving as a course text, this book is an essential reference for students and researchers in physics, applied physics, chemistry, materials science and engineering, who are interested in modern condensed matter physics.
ßçûê: Ðóáðèêà: Ôèçèêà /Ôèçèêà òâ¸ðäîãî òåëà /Ñòàòóñ ïðåäìåòíîãî óêàçàòåëÿ: Ãîòîâ óêàçàòåëü ñ íîìåðàìè ñòðàíèö ed2k: ed2k stats Ãîä èçäàíèÿ: 1995Êîëè÷åñòâî ñòðàíèö: 699Äîáàâëåíà â êàòàëîã: 12.08.2005Îïåðàöèè: Ïîëîæèòü íà ïîëêó |
Ñêîïèðîâàòü ññûëêó äëÿ ôîðóìà | Ñêîïèðîâàòü ID
Ïðåäìåòíûé óêàçàòåëü
Diffusion, on a lattice, master equation 378—381 Diffusion, particle 353 Diffusion, permeation 617 Diffusion, phason 617 Diffusion, response function 371—373 Diffusion, spin 438 Diffusion, thermal 425 429 438 451 454 Diffusion, vacancy 459 Dilation 317 Dilation symmetry 90 668 Dirac delta function 204 Direct lattice 43—45 668 Direct pair correlation function 195 Directed diffusion 415 668 Director 59 168 420 506 668 Disclinations 495 668 Disclinations, and the KT transition 558 Disclinations, density 534 Disclinations, in crystal 517 519 534 Disclinations, in hexatic liquid crystals 526 Disclinations, in nematic liquid crystals 524—526 Disclinations, in smectic-C films 506 509 Disclinations, loops 527 Disclinations, twist 519 Disclinations, Volterra construction 517 519 Disclinations, wedge 519 Discommensurations 590 602—603 613 668 Disconnected diagram 279 Discontinuous transition 13 (see also “First-order phase transition”) Discotic liquid crystals 15 68 668 Discrete Gaussian Model 578 584 645 668 Discrete symmetry, group 13 135—137 590 668 Dislocations chapter 9 12 13 49 495 510 520 531 640—643 668 Dislocations, and CI transition 640—643 Dislocations, and grain boundaries 522—524 Dislocations, Burgers vector 508—509 513 534 Dislocations, density 521 Dislocations, in crystals 531 Dislocations, in crystals, edge 514—515 Dislocations, in crystals, energies 531—536 Dislocations, in crystals, screw 514—515 Dislocations, in hexagonal and close-packed lattices 515 Dislocations, in periodic solids 512—517 Dislocations, in smectic liquid crystals 507 308 507—512 561—573 Dislocations, mediated melting 555 555—559 Dislocations, Volterra construction 515 683 Dissipation 361 365—366 394—395 419 446—448 668 Dissipationless flow 445—448 668 Dissipative dynamics 466—469 domain walls chapter 10 15 590 668 Domain walls, fluctuating 635 Domain walls, in mean-field theory 595—601 Dual lattice 50 580 668 Duality, lattice Coulomb-gas model 582—584 Duality, Potts models 579—582 Duality, Villain model 578—579 582—584 Duality, xy-model 582—584 Dynamic models see “Models stochastic” Dynamic modes 2 359 362—368 diffusion sound”) Dynamic scaling 469—472 Dynamic upper critical dimension 471 Easy-plane ferromagnet 138 Edge dislocations 13 510 520 640—643 Edge dislocations, loops 512 Effective critical exponent 273 Einstein relation 373—375 385 668 Elastic constants, tables of 319 320 Elastic continuum 366 Elastic variable 288 Elasticity chapter 6 Elasticity of sterically stablized phases 638—640 Elasticity, 298—306 Elasticity, Eulerian stress tensor 338—341 Elasticity, fluctuations 293—295 Elasticity, fluctuations, and light scattering 306—308 Elasticity, frank free energy, of nematic liquid crystals 298—300 Elasticity, free energy, xy-model 289—290 Elasticity, Josephson scaling relation 292—293 Elasticity, Lagrangian elasticity 330—334 Elasticity, Lagrangian stress tensor 334—337 Elasticity, nonlinear sigma model 341—347 Elasticity, of classical harmonic lattices 332 Elasticity, of solids 316 Elasticity, of solids, bond-angle and translational order 328 Elasticity, of solids, density functional theory 329 Elasticity, of solids, elastic free energy 318—319 Elasticity, of solids, fluctuations 321—322 Elasticity, of solids, isotropy, and cubic solids 319—321 Elasticity, of solids, one-dimensional crystals 322—324 Elasticity, of solids, two-dimensional crystals 324—326 Elasticity, of solids, vacancies and interstitials 325—328 Elasticity, polymerized membranes 632 Elasticity, smectic liquid crystals 308—316 Elasticity, stress-strain relations 337—338 Elasticity, xy-model 289—297 Electron scattering 409 Energy density 240 Energy of a surface 625—626 Enthalpy 113 entropy 3 111 119 146 424—425 Entropy production equation 444—445 Entropy, density 424 427 Entropy, of mixing 146 Entropy, steric 635 Equations of state 116—117 Equations of state, mean-field 153 Equations of state, scaled 234—235 Equations of state, Van der Waals 683 Equipartition theorem 10 123 223 Escape, to third dimension 501 Euclidean group 39 668 Euler characteristic 626 669 Euler — Lagrange equation 301—302 485 538 597 671 Eulerian coordinates 331 446 Eulerian elasticity 338—341 Euler’s equation 448 669 exchange interaction 21—25 Excluded volume 46 94 Exclusion principle 7 23 Extensive Variable 111 External Hamiltonian 134 f-sum rule 372 670 Face-centered cubic structure 9 41 53—57 190—194 669 Face-centered cubic structure, defects 515—517 Facets and faceting 8 648—655 Fan spin order 87 FCC see “Face-centered cubic structure” Fermi gas 122 Fermi pseudopotential 402—404 Fermi’s Golden Rule 30 400—402 669 Ferrimagnet 87 130 Ferroelectric phase 81 138 Ferromagnet, conjugate field 128 Ferromagnet, ferromagnetic phase 87 128 Ferromagnet, hedgehog defect for 500 Ferromagnet, Heisenberg model 127 435 Ferromagnet, hydrodynamics, isotropic 439—440 Ferromagnet, model J 477 Ferromagnet, order parameter 128 Ferromagnet, spin waves 440 Ferromagnetic exchange 25 Fick’s law 369—370 373 669 Field theory 213—283 Field theory, construction 217—226 Field theory, gaussian integrals 221—223 Field theory, Ising model, hypercubic lattice 248—252 Field theory, Ising model, one-dimensional Ising model 242—245 Field theory, Landau — Ginzberg — Wilson 465 Field theory, lattice field theories, continuum limit 219—221 Field theory, mean-field theory, from functional integrals 223—225 Field theory, n-vector model 228—230 Field theory, self-consistent field approximation 226—230 First homotopy group 504 First law of thermodynamics 109—111 First-order phase transition 144 First-order phase transition, and harmless staircase 605 First-order phase transition, and nucleation 480—482 First-order phase transition, bicritical point 183 First-order phase transition, liquid-to-solid 187—198 First-order phase transition, nematic-isotropic transition 168—172 First-order phase transition, Potts model 202 First-order phase transition, tricritical point 174 Five-fold symmetry 50 82—85 Fixed point 245 251 262 267—269 671 548 573 634 669 Fixed point, cubic 268 Fixed point, Gaussian 262 268 Fixed point, Heisenberg 266 268 Fixed point, high T 245 262 Fixed point, Ising, 251 Fixed point, Ising, 268 Fixed point, Ising, 1D 245 Fixed point, Kosterlitz — Thouless 548 573 Fixed point, polymerized membrane 634 Fixed point, Potts lattice gas 256—257 FK see “Frenkel — Kontorowa” Floating phase 601 Flory parameter 671 Flory theory, radius of gyration of a polymer 94 669 Fluctuating walls 635—640 Fluctuation-dissipation theorem 353 374 387 397—399 664 Fluctuations 15 Fluctuations, and break-down of mean field theory (Ginzburg) 214—217 225—226 Fluctuations, and corrections to mean-field theory 294 Fluctuations, and correlation functions 35 125 131 Fluctuations, and Debye — Waller factor 294 322 407 666 Fluctuations, and destruction of long-range order 294 Fluctuations, and scattering 405—408 Fluctuations, and susceptibilities 120 132 Fluctuations, dynamic 355 387 397 Fluctuations, in harmonic approcimation 626—629 Fluctuations, in mean-field theory 154 157 Fluctuations, of elastic variables 293 306 312 321 Fluctuations, of fluid membranes 626 Fluid hydrodynamics 441—453 Fluids 38—43 Fluids, internal energy 109 Fluids, thermodynamics 108—117 Fluids, two-component 453 (see also “Superfluids” “Water”) Fokker — Planck equation 388—389 473 Four-state Potts model 256 Fourier transforms 10 97—100 Fourier transforms, transforms on a lattice 100 Fractals 91—97 Frank director 168 302 311 328 Frank free energy 298—300 311 316 455 567 669 Free standing smectic-C films 506 Freedericksz transition 302—304 670 Freezing 8—15 Freezing, Hansen — Verlet criterion 192 671 Frenkel — Kontorowa model 601—620 Frustration 7 112 670 Functional derivatives 140—141 672 Functional integral 218 Fundamental group 504 670 Galilean transformation 441—442 Gap exponent 234 Gas partition function, Coulomb 588—589 Gaseous state 3—5 38—43 Gauge symmetry 135 670 Gauss — Bonnet theorem 626 670 Gaussian critical point 262 670 Gaussian curvature 624 625 670 Gaussian fluctuations 224 670 Gaussian Integrals 221—223 Gaussian model, momentum shell renormalization group 261—263 Generalized force 110 Generalized Heisenberg models 435—436 Genus 626—627 Gibbs free energy 112 114 Gibbs’ paradox 119 670 Ginzburg criterion 214—217 225 670 Ginzburg length 215 228 Ginzburg parameter 572 Ginzburg temperature 215 225—226 228 272 Ginzburg — Landau model, time-dependent 466 682 Glaciers 12 Glass 112 671 Glauber model 466 671 Glide 12 520 Glide plane, lattice 57 Global packing constraints 7 Global symmetry 135 671 Glossary 662—684 Gold, fractal aggregate 95 Golden mean 51 83 671 Goldstone mode 432 471 Goldstone theorem 432 434 Good solvent 93 673 Grain boundaries 522—523 589 671 grand canonical ensemble 120 125 Grand potential 113 Graphite, lattice 78—79 Gravity 335 Green function 477—478 Green function, and dynamic response 370—371 Green function, and mode-mode coupling 477—478 Green function, diffusion 370—371 376 531 Ground state manifold 502 Groups, first fundamental group 504 672 Groups, homotopy 504—505 524 Gyration, radius of 91 680 Hameker constant 553 Hansen — Verlet criterion 192 671 Hard cutoff 219 671 Hard-sphere, close-packing 671 Hard-sphere, fluid 38 Hard-sphere, interaction 40 Hard-sphere, model 7 Hard-sphere, solid-liquid phase transition 43 Harmless devil’s staircase 605 Harmonic lattice, acoustic phonons 367—368 harmonic oscillator 359—366 Harmonic oscillator, fluctuation-dissipation 387 Hartree approximation 226 672 Hausdorff dimension 97 672 Heat 109 heat capacity 114 Heat current 424 427 445 Heaviside unit step function 355 Heavy wall 602 672 Hedgehog 500—503 527 585 674 Heisenberg fixed point 266 269 Heisenberg model 14 127—129 140 202 435 672 Heisenberg operator 392 Heitler — London theory 20 25 672 Helical magnetic phase 91 Helical spin order 87 Helicity modulus 290—291 Helium, 117 179 Helium, 179 . Helium, 177 179 Helium, helium films 551—555 Helium, Kosterlitz — Thouless transition 551—552 Helium, superfluids 138 289 460—464 Helmholtz free energy 112 194 200 672 Helmholtz free energy, density 115 122 126—127 327 Hexagonal close-packed solid (HCP) 41 56—57 105 515—517 672 Hexagonal lattice 57 69 191 515—517 Hexatic phases 65—68 672 Hexatic phases, 2D crystals 78 558
Ðåêëàìà
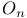symmetry and nematic liquid crystals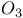 
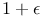Migdal-Kadanoff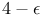 
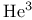
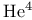mixtures 
|
|
Î ïðîåêòå