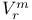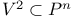Авторизация
Поиск по указателям
M.A.Akivis, V.V.Goldberg — Projective Differential Geometry of Submanifolds
Обсудите книгу на научном форуме Нашли опечатку?
Название: Projective Differential Geometry of SubmanifoldsАвторы: M.A.Akivis, V.V.Goldberg Аннотация: In this book, the general theory of submanifolds in a multidimensional projective space is constructed. The topics dealt with include osculating spaces and fundamental forms of different orders, asymptotic and conjugate lines, submanifolds on the Grassmannians, different aspects of the normalization problems for submanifolds (with special emphasis given to a connection in the normal bundle) and the problem of algebraizability for different kinds of submanifolds, the geometry of hypersurfaces and hyperbands, etc. A series of special types of submanifolds with special projective structures are studied: submanifolds carrying a net of conjugate lines (in particular, conjugate systems), tangentially degenerate submanifolds, submanifolds with asymptotic and conjugate distributions etc. The method of moving frames and the apparatus of exterior differential forms are systematically used in the book and the results presented can be applied to the problems dealing with the linear subspaces or their generalizations.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1993Количество страниц: 362Добавлена в каталог: 11.12.2009Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Submanifold(s), 2-dimensional 59 64—66 158 207 289 Submanifold(s), 2-dimensional of 4th order 30 Submanifold(s), 2-dimensional, 2nd fundamental form of 59—61 64—66 158 289 Submanifold(s), 2-dimensional, asymptotic direction of 60—61 64—66 Submanifold(s), 2-dimensional, conjugate net of 60 Submanifold(s), 2-dimensional, infinitesimal displacement of moving frame of 287 Submanifold(s), 2-dimensional, invariant normalization of 207 Submanifold(s), 2-dimensional, moving frame of 287 Submanifold(s), 2-dimensional, osculating subspace of 289 290 Submanifold(s), 2-dimensional, semi-asymptotic net of 61 Submanifold(s), 2-dimensional, smooth 285 Submanifold(s), 2-dimensional, tangent subspace to 290 Submanifold(s), 2-dimensional, tangentially degenerate 67 Submanifold(s), 2-dimensional, Veronese mapping of 64—66 Submanifold(s), 2-ruled 72 Submanifold(s), 2nd fundamental form of 40 42 47 62 71 165 169 277 Submanifold(s), 2nd fundamental form of, 2-dimensional 59—61 64—66 Submanifold(s), 2nd fundamental form of, 3-dimensional 56—58 67—70 Submanifold(s), 2nd fundamental form of, carrying conjugate net 73 Submanifold(s), 2nd fundamental tensor of 39 73 148 149 152 165 269 277 Submanifold(s), 2nd fundamental tensor of, rank of 56 Submanifold(s), 2nd osculating subspace of 41 62 Submanifold(s), 3-dimensional 56—58 72 Submanifold(s), 3-dimensional with 6-dimensional osculating sub-space 72 Submanifold(s), 3-dimensional with complete system of one-dimensional asymptotic distributions 151 Submanifold(s), 3-dimensional, 2nd fundamental form of 56—61 64—66 Submanifold(s), 3-dimensional, asymptotic direction of 57—58 67—70 Submanifold(s), 3-dimensional, carrying net of asymptotic lines 151 Submanifold(s), 3-dimensional, conjugate directions of 57—58 67—70 Submanifold(s), 3-dimensional, conjugate net of 57 Submanifold(s), 4th order neighborhood of 199 Submanifold(s), 5-dimensional 72 Submanifold(s), bundle of 1st order frames of 93 Submanifold(s), carrying complete system of conjugate directions 165 Submanifold(s), carrying conjugate net 73 85 99 104 111 188 190 200 207 Submanifold(s), carrying conjugate net, 2nd fundamental form of 62 73 165 Submanifold(s), carrying conjugate net, existence of 85 Submanifold(s), carrying conjugate net, invariant normalization of 199—205 207 Submanifold(s), carrying conjugate net, quasi-Laplace transformation of 100 112 Submanifold(s), carrying family of rectilinear generators 171 Submanifold(s), carrying m-conjugate system 101 Submanifold(s), carrying net 207 Submanifold(s), complete parabolic 134 141 Submanifold(s), complete regular 137 Submanifold(s), conic 104 112 168 Submanifold(s), conic, 2-dimensional 109 110 Submanifold(s), conic, equations of 104 110 Submanifold(s), conic, existence of 105 Submanifold(s), conic, generalized 107—110 Submanifold(s), conic, vertex of 168 Submanifold(s), determinant 29 30 67 130 131 Submanifold(s), determinant, algebraic 53 Submanifold(s), doubly foliated 83 Submanifold(s), equipped 174 Submanifold(s), filtration of 62 67 Submanifold(s), generator of 116—118 132 134 148 150 Submanifold(s), infinitesimal displacement of frame of 74 Submanifold(s), integral 144 170 Submanifold(s), moving frame of 34 Submanifold(s), normalized 174 205 206 266 Submanifold(s), normalized of codimension 2 205 Submanifold(s), normalized, element of 174 Submanifold(s), normalized, infinitesimal displacement of frame of 174 282 Submanifold(s), normalized, tangentially degenerate 205 Submanifold(s), parabolic 132—135 Submanifold(s), parabolic, 3-dimensional of rank 2 14 Submanifold(s), parabolic, complete 134 141 Submanifold(s), Peterson 104 112 Submanifold(s), Peterson, generalized 108 109 111 Submanifold(s), point 269 Submanifold(s), quasiasymptotic 71 111 Submanifold(s), regular 134 137 Submanifold(s), regular, complete 137 Submanifold(s), Riemannian 133 Submanifold(s), ruled 94 171 Submanifold(s), shadow 112 170 Submanifold(s), singular 269 Submanifold(s), smooth 285 Submanifold(s), support 255 261 Submanifold(s), support, plane generator of 255 257 260 261 Submanifold(s), system of 277 278 Submanifold(s), system of, algebraic 277 278 Submanifold(s), tangent subspace to 34 36 62 145 174 Submanifold(s), tangentially degenerate 38 96 103 113 116—118 121 122 124 126 129 141 166 Submanifold(s), tangentially degenerate of codimension 3 101 Submanifold(s), tangentially degenerate of rank 2 118 Submanifold(s), tangentially degenerate of rank one 116 118 122 123 Submanifold(s), tangentially degenerate, 2nd osculating subspace of 119 120 124 127 128 Submanifold(s), tangentially degenerate, 3-dimensional 57 58 67—70 Submanifold(s), tangentially degenerate, 3-dimensional of rank 2 137 Submanifold(s), tangentially degenerate, autodual 117 255 Submanifold(s), tangentially degenerate, carrying holonomic focal net 125 Submanifold(s), tangentially degenerate, generator of 116 118 132 134 148 150 Submanifold(s), tangentially degenerate, infinitesimal displacement of frame of 114 Submanifold(s), tangentially degenerate, normalized 205 Submanifold(s), tangentially degenerate, self-dual 117 255 Submanifold(s), tangentially degenerate, tank of 96 166 Submanifold(s), tangentially degenerate, without singularities 118 Submanifold(s), tangentially nondegenerate 38 73 75 103 113 116 Submanifold(s), with special projective structure vi Subnormal of 1st normal 174 175 193 Subnormal of 1st normal of hyperquadric 177 Subspace(s) 169 Subspace(s), (r-1)-secant 29 30 Subspace(s), 1st normal of 36 40 53 61 147 167 Subspace(s), asymptotic 145 147 Subspace(s), characteristic 49 52 53 120 Subspace(s), conjugate 215 Subspace(s), conjugate, complementary 44 277 Subspace(s), differential equations of 143 Subspace(s), infinitesimal displacement of frame of 26 143 Subspace(s), invariant 147 Subspace(s), linear v Subspace(s), normal 36 42 43 45 61 62 147 Subspace(s), normal, basis of 43 Subspace(s), normal, dimension of 43 Subspace(s), normal, reduced 45 81 Subspace(s), osculating of 2-submanifold 288—290 Subspace(s), osculating of curve 53 Subspace(s), osculating of Grassmannian 50 Subspace(s), osculating of hypersurface 54 Subspace(s), osculating of order k of image of Grassmannian 52 Subspace(s), osculating of order q to submanifold 46 48 151 Subspace(s), osculating, 1st of submanifold 41 Subspace(s), osculating, 2nd of 119 120 124 127 128 Subspace(s), osculating, 2nd of Segre variety 41 Subspace(s), osculating, 2nd of submanifold 41 42 47 55 56 62 82 85 119—128 145 147—150 152 165 167 Subspace(s), osculating, 2nd of Veronese variety 42 Subspace(s), osculating, 3rd 45 Subspace(s), pencil of 28 Subspace(s), projective 116 143 Subspace(s), stationary subgroup of 25 26 Subspace(s), stationary subgroup of, invariant form of 26 Subspace(s), tangent 5 34 35 61 145 Subspace(s), tangent, to 2-submanifold 290 Subspace(s), tangent, to curve 53 Subspace(s), tangent, to hypersurface 291 Subspace(s), tangent, to image of Grassmannian 35 Subspace(s), tangent, to Segre variety 37 Subspace(s), tangent, to submanifold 34 35 61 145 Subspace(s), tangent, to tangentially degenerate submanifold 115 Subspace(s), tangent, to Veronese variety 138 Summation 1 Support submanifold 255 261 Support submanifold, plane generator of 255 257 260 261 Surface(s) 90 91 173 209 245 Surface(s), 2nd fundamental form of 91 92 Surface(s), asymptotic line of 251 Surface(s), canonical tangent of 250 251 Surface(s), conic 108 Surface(s), conjugate net on 55 91 92 Surface(s), Darboux line of 251 Surface(s), degeneracy of 251 Surface(s), Fubini linear element of 214 251 Surface(s), Fubini net of 251 Surface(s), invariant of 245 Surface(s), Laplace transform of 90 91 111 Surface(s), Laplace transform of, 2nd fundamental form of 98 Surface(s), moving frame of 90 91 245 252 Surface(s), parabolic 135 Surface(s), projective theory of 267 Surface(s), projectively deformable 267 Surface(s), ruled 29 156 158 235 251 Surface(s), ruled of 2nd order 30 Surface(s), with common Lie quadrics 252 253 Surjective mapping 7 svec v 266 267 326 Svoboda 171 327 Symmetric double transformation 62 Symmetric embedding 30 Symmetric matrix 113 138 Symmetric tensor 130 177 232 243 Symmetric tensor, nondegenerate 177 Symmetric tensor, product 37 Symmetrization 27 Symmetroid, cubic 31 67—69 286 287 System of 2nd fundamental forms 169 System of 2nd fundamental forms of 64—67 System of 2nd fundamental forms of 67—70 System of hypersurfaces 271 System of Pfaffian equations in involution 12 31 87 89 105 108 126 139 141 153 191 253 System of Pfaffian equations, completely integrable 11 System of points, rank of 81 83 201 System of submanifolds 277 278 System of submanifolds, algebraizable 277 278 System of tensors 240 System, closed 109 System, complete 2-component 148 System, complete 2-component, doubly foliated 149 150 System, complete 2-component, integrable conjugate 169 System, complete multicomponent 150 System, completely integrable 11 System, conic 105 107 205 System, conjugate 78—80 System, conjugate of distributions 165 System, conjugate, 2-component 112 148 165 169—171 System, conjugate, conic 205 System, conjugate, conic 2-component 169 System, conjugate, multicomponent 171 System, m-conjugate 78—80 82 83 98—101 104 106 System, m-conjugate, 2nd Laplace transform of 101 System, m-conjugate, conic 105 108 205 System, m-conjugate, existence of 85 System, m-conjugate, Laplace transform of 100 111 System, m-conjugate, R 112 T-pair of complexes 112 Tangency of 1st order 216 Tangency of 2nd order 211 216 Tangency of 3rd order 217 218 220 Tangency, double 69 Tangent bundle 5 178 183 Tangent bundle, affine connection in 179 183 193 Tangent bundle, element of 5 Tangent canonical 250 251 Tangent hyperplane 49 136 284 Tangent hyperplane, to hypercone 284 Tangent hyperplane, to hyperquadric 219 Tangent hyperplane, to hypersurface 211 216 272 Tangent plane, double 249 Tangent space 5 19 Tangent space, basis of 19 Tangent subspace to 2-dimensional submanifold 290 Tangent subspace to curve 53 Tangent subspace to hypersurface 291 Tangent subspace to image of Grassmannian 35 Tangent subspace to Segre variety 37 Tangent subspace to submanifold 34 35 61 145 Tangent subspace to tangentially degenerate submanifold 115 Tangent subspace to Veronese variety 138 Tangent vector 5 Tangent vector, coordinates of 14 48 Tangential coordinates 20 49 261 Tangential frame 20 Tangential frame of hyperband 257 Tangential frame, infinitesimal displacement of 20 Tangentially degenerate hypersurface 256 260 Tangentially degenerate hypersurface of rank one 116 Tangentially degenerate hypersurface of rank two 291 Tangentially degenerate submanifold 38 96 113 116—118 121 122 124 126 129 141 166 Tangentially degenerate submanifold of codimension 3 101 Tangentially degenerate submanifold of rank 2 118 Tangentially degenerate submanifold of rank one 116 118 122 123 Tangentially degenerate submanifold, 2-dimensional 67 Tangentially degenerate submanifold, 2nd osculating subspace of 119 120 124 127 128 Tangentially degenerate submanifold, 3-dimensional 57 58 67—70 Tangentially degenerate submanifold, 3-dimensional of rank 2 137 Tangentially degenerate submanifold, autodual 117 255 Tangentially degenerate submanifold, carrying holonomic focal net 125 Tangentially degenerate submanifold, generator of 116—118 132 134 148 150 Tangentially degenerate submanifold, infinitesimal displacement of frame of 114 Tangentially degenerate submanifold, normalised 205 Tangentially degenerate submanifold, rank of 96 166 Tangentially degenerate submanifold, self-dual 117 255 Tangentially degenerate submanifold, tangent subspace to 115 Tangentially degenerate submanifold, without singularities 118 Tangentially degenerate surface 67 Tangentially nondegenerate hypersurface 222 Tangentially nondegenerate submanifold 38 73 75 103 113 116 Teixidor 271 327 Tensor(s) 176 197 Tensor(s) of projective curvature 180 181 Tensor(s), 1st canonical 230 Tensor(s), 2nd fundamental of, hypersurface 54 218 221 Tensor(s), 2nd fundamental of, submanifold 39 73 148 149 152 165 269 277 Tensor(s), 3rd fundamental 44 152 Tensor(s), apolar 233 Tensor(s), curvature 17 179 Tensor(s), Darboux 112 171 213 218 218—220 222 225—228 244 266 269 Tensor(s), Darboux of hyperband 265 Tensor(s), Darboux, degenerate 227 Tensor(s), Darboux, nondegenerate 236 Tensor(s), determinant of 236 Tensor(s), differential equations of 3 Tensor(s), field vii 6 Tensor(s), fundamental of order q 48 Tensor(s), inverse 178 199 203 211 214 227 258 Tensor(s), law of transformation of 4 115 Tensor(s), mixed 39 Tensor(s), mutually inverse 203 214 258 Tensor(s), non singular 197 Tensor(s), nondegenerate 257 258 Tensor(s), nondegenerate, Darboux 226 Tensor(s), nondegenerate, symmetric 177 Tensor(s), rank of 295 Tensor(s), relative 4 212 213 242 246 259 Tensor(s), Ricci 181 185 186 Tensor(s), Ricci, alternated 185 Tensor(s), symmetric 130 232 233 243 Tensor(s), symmetric, nondegenerate 177 Tensor(s), symmetric, product 37 Tensor(s), system of 240 Tensor(s), torsion 17 179 Tensor(s), trace of 225 Tensorial method vii Tensorial square 30 Terentjeva 268 327 Terrachini 71 Theorem, Abel 270 278 295 Theorem, Abel, generalization of 295 Theorem, Euler 195
Реклама
 |
|
О проекте
|
|
О проекте