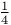|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Oldham K., Spanier J. — The fractional Calculus: Theory and applications of differentiation and integration to arbitrary order |
|
|
 |
| Предметный указатель |
Semidifferential equations, used to relate ground-level pollution concentrations to pollution generation rate 208
Semidifferentiation 14
Semidifferentiation and semiintegration, analog circuits for 148—154
Semidifferentiation, used to relate surface temperature to heat flux 203
Semiintegral electroanalysis 204
Semiintegrals 16 185 see
Semiintegrating circuits 152
Semiintegrating circuits, accuracy of 149
Shermergor, T.D. 2 222
Shilov, G.E. 13
Shinbrot, M. 12 14
Sine integrals 128
Sine integrals as hypergeometrics of complexity 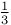 165 165
Sine integrals as reducible transcendentals 161
Sines and Cosines see "Hyperbolic sines"
Sines and cosines as periodic functions, differintegration of 110
Sines and cosines of  , as hypergeometrics 161 163 , as hypergeometrics 161 163
Sines and cosines of  , as hypergeometrics, differintegration of 96 , as hypergeometrics, differintegration of 96
Sines and cosines, differintegration as examples of cyclo-differential functions 110—112
Sines and cosines, semiderivatives and semiintegrals 124—126
Sneddon, I.N. 12 13
Somorjai, R.L. 2 14 222
Spanier, J. 2 14 15 200 204 222
Special functions of mathematical physics, as K=L-2 transcendentals 177
Special functions of mathematical physics, interrelations among 79 81
Stegun, I.A. 219
Stephens, E. 10
Stirling numbers of first kind 19
Stirling numbers of first kind, table of values 18
Stirling numbers of second kind 22 37 81
Stirling numbers of second kind, notation for 15
Stirling numbers of second kind, table of values 22
Struve functions 124 125 177—178
Struve functions as hypergeometrics of complexity  164 164
Struve functions as reducible transcendentals 161
Struve functions, notation for 15
Stuloff, N. 12
Symbolic methods 2
Synthesis diagrams 169
Synthesis diagrams for associated Legendre functions 173
Synthesis diagrams for K=L transcendentals 172—174
Synthesis diagrams for K=L-1 transcendentals 175—176
Synthesis diagrams for K=L-2 transcendentals 177—180
Synthesis diagrams Legend re line 173
Synthesis diagrams logarithm line 175
Synthesis diagrams, Bessel — Struve line 178
Synthesis diagrams, example illustrating principles 170
| Synthesis diagrams, involving steps of 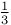 and and 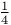 179—180 179—180
Tautochrone 2 4 5 183
Tautochrone problem, solution as a semiintegral 185
Tautochrone, coordinate system for 184
Tautochrone, equations for 185
Taylor's series 14 15
Techniques in fractional calculus 133—160
Terentev, N.M. 209 220
Term-by-term differentiation 38 74—75
Term-by-term differintegration 69—75
Term-by-term differintegration of arbitrary differintegrable series 71—74
Term-by-term differintegration, to negative order 71—72
Term-by-term differintegration, to positive order 74—75
Term-by-term integration 38
Titchmarsh, E.C. 79 222
Transcendental functions, interrelationships among 167
Transcendental functions, representation as hypergeometrics 162—165
Transcendental functions, representations of 161—180
Transmission line, impedance of 212 213
Transmission line, optimum termination of 212
Transmission line, simulation by discrete components 216
Transmission line, symbolism, diagram explaining 211
Transmission line, terminations, diagram depicting four alternatives 214
Transmission line, theory 2
Transport in semiinfinite medium 198—200
Transport problems 2
Transport theory 197
Tricomi, F.G. 220
Veinoglu, B.C. 222
von Wolfersdorf, L. 13
Wall, H.S. 153 223
Wallis' infinite product for  3 3
Wallis, J. 3
Wastchenxo, Z. 7
Watanabe, Y. 10 79 223
Wave equation 11
Weakly singular intergral equation see "Abel's integral equation"
Wetland, G.V. 13
Weyl differintegrals 95
Weyl integral 53
Weyl, H. 2 8 10 53 95 223
Widder, D.V. 11 38 223
Wiener, N. 2 223
Withers, R.F.J. 220
Young, L.C. 11
Zero, differintegration of 63
Zeta function 142—143
Zygmund, A. 10 11 14
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



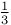
 , as hypergeometrics
, as hypergeometrics