Авторизация
Поиск по указателям
Mcmullen P., Schulte E. — Abstract Regular Polytopes
Обсудите книгу на научном форуме Нашли опечатку?
Название: Abstract Regular PolytopesАвторы: Mcmullen P., Schulte E. Аннотация: geometrical research, which began with regular polygons and polyhedra. They are highly symmetric combinatorial structures with distinctive geometric, algebraic or topological properties; in many ways more fascinating than traditional regular polytopes and tessellations. The rapid development of the subject in the past 20 years has resulted in a rich new theory, featuring an attractive interplay of mathematical areas, including geometry, combinatorics, group theory and topology. Abstract regular polytopes and their groups provide an appealing new approach to understanding geometric and combinatorial symmetry. This is the first comprehensive up-to-date account of the subject and its ramifications, and meets a critical need for such a text, because no book has been published in this area of classical and modern discrete geometry since Coxeter's Regular Polytopes (1948) and Regular Complex Polytopes (1974). The book should be of interest to researchers and graduate students in discrete geometry, combinatorics and group theory.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 2002Количество страниц: 551Добавлена в каталог: 23.11.2009Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Polygon, regular see Regular polygon Polygon, star-polygon 16 Polyhedron 25 Polyhedron, abstract 25 Polyhedron, convex 8 Polyhedron, infinite see Apeirohedron Polyhedron, regular see Regular polyhedron Polyhedron, toroidal 18 Polytopal 26 Polytope 22 Polytope, 25 Polytope, 22 25 Polytope, 7 Polytope, abstract 22-31 Polytope, chiral 38 Polytope, complex regular 293 Polytope, convex 7 Polytope, cubical 268 Polytope, dual 9 28 Polytope, finite 25 Polytope, finite, locally 25 Polytope, flat 109 115-120 275 372 508 514 Polytope, flat, 109 Polytope, geometric 122 Polytope, group of 27 Polytope, incidence polytope 22 Polytope, infinite see Apeirotope Polytope, locally of topological type 361 Polytope, neighbourly 249 268 271 404 Polytope, neighbourly, weakly 267 Polytope, projective 162-165 287 502 Polytope, projective, locally 284 461 463 467 502-509 516 Polytope, regular see Regular polytope Polytope, self-dual 28 Polytope, simplicial 268 Polytope, spherical 153 Polytope, spherical, locally 152-162 Polytope, symmetric 123 Polytope, symmetry group of 9 123 Polytope, topological model of 161 367 Polytope, toroidal 154 165-175 Polytope, toroidal, higher 445 450-470 Polytope, toroidal, locally see Locally toroidal regular polytope POSET 22 Pre-polytope 43 primitive 291 Proclus 2 Proper central involution 249 255 Pythagoras 2 Quotient 42-49 Quotient, criterion 56 Quotient, lemma 56 Quotient, polytope 44 58-60 272 Quotient, space 149 Rad 74 Radical 298 Rank 22 Rank 23 Rank, co-rank 23 Rank, of Coxeter group 65 Rank, rank function 23 Rap-map 43 Realization 121-127 Realization, 261-264 Realization, blend 122 125 Realization, centre of 127 Realization, cone 127-140 Realization, cross-polytope realization 135 Realization, degenerate 122 Realization, dimension of 122 Realization, discrete 142 Realization, faithful 122 Realization, linear combination 122 126 Realization, of 136 Realization, of 484-490 Realization, of 259-261 Realization, of 417 Realization, of 396-398 Realization, of 24-cell 138 Realization, of dodecahedron 137 Realization, of finite regular polytope 127-140 Realization, of hemi-dodecahedron 138 Realization, of icosahedron 137 Realization, of Klein's map 139 Realization, of regular apeirogon 140 Realization, of regular apeirotope 140-147 Realization, of regular polygon 135 Realization, pure 126 Realization, scalar multiple of 122 126 Realization, simplex realization 128 Realization, symmetries of 123 Realization, symmetry group of 123 Realization, translation-free 143 Realization, trivial 122 Realization, vertex-faithful 122 Realization, vertex-set of 121 Rec 142 Reduced sequence 98 Reflexion group 64 290 298 Reflexion group, complex, locally finite 306 Reflexion group, complex, locally unitary 306 Reflexion group, complex, strongly locally finite 327 334 Reflexion group, complex, with involutory generators 298-319 Reflexion group, Coxeter see Coxeter group Reflexion group, euclidean, discrete infinite 73 89 Reflexion group, euclidean, finite 71-72 83-94 Reflexion group, euclidean, irreducible 73 Reflexion group, euclidean, preserving figure see Symmetry group Reflexion group, euclidean, reducible 75 Reflexion group, unitary, 293 Reflexion group, unitary, blend of 415 Reflexion group, unitary, completely reducible 290 Reflexion group, unitary, finite 290-298 321-327 334-336 339-341 343-344 347-355 357-359 Reflexion group, unitary, imprimitive 291 Reflexion group, unitary, infinite discrete 309-312 327-331 344 350 354-355 Reflexion group, unitary, irreducible 290 Reflexion group, unitary, primitive 291 Reflexion group, unitary, real 294 Reflexion group, unitary, with involutory generators 294-298 Reflexion group, Weyl see Weyl group Reflexion in hyperplane, complex 298 Reflexion in hyperplane, complex, unitary 290 Reflexion in hyperplane, real 68 Reflexion in hyperplane, real, euclidean 10 Reflexion in subspace 10 123 Regular 9 31 Regular apeirogon 15 25 27 Regular apeirogon, helical 217 222 Regular apeirogon, linear 217 221 Regular apeirogon, realizations of 140 Regular apeirogon, zigzag 217 222 Regular apeirohedron, in 220-236 Regular apeirohedron, in 221-223 226-229 Regular apeirohedron, in 221 Regular apeirohedron, in 223-226 230-236 Regular apeirotope, 4-apeirotopes in 236-243 Regular apeirotope, realizations of 140-147 Regular honeycomb, euclidean 15 81 254 Regular honeycomb, euclidean, symmetry group of 67 73 Regular honeycomb, hyperbolic 78 81 204 254 362 384 431-437 445-449 Regular honeycomb, hyperbolic, symmetry group of 67 78 Regular honeycomb, with finite cells see regular tessellation Regular map 17-20 179 Regular map, Dyck's map 18 140 399 474 Regular map, Klein's map 18 139 474 Regular map, non-orientable 180 Regular map, of hyperbolic type 179 Regular map, polyhedral models of 140 268 Regular map, polytopal see Regular polyhedron Regular map, toroidal 18 364 390-391 Regular polygon, blended 135 222 Regular polygon, convex 3 6 11 27 Regular polygon, helical 217 Regular polygon, in 217 Regular polygon, infinite see Regular apeirogon Regular polygon, pure 217 Regular polygon, realizations of 135 Regular polygon, skew 217 222 Regular polygon, star-polygon 5 16 135 Regular polyhedron, convex 1-4 11 217-220 Regular polyhedron, Coxeter's 256 259 264 Regular polyhedron, Coxeter's 140 184 196 256 260 Regular polyhedron, Coxeter's skew 140 196 Regular polyhedron, history of 1-7 Regular polyhedron, in 217-236 Regular polyhedron, infinite see Regular apeirohedron Regular polyhedron, Kepler - Poinsot 5 16 212 217-220 Regular polyhedron, on surface see Regular map Regular polyhedron, Petrie - Coxeter 18 196 Regular polyhedron, polyhedral models of 140 268 Regular polyhedron, related to a linear group 471-490 Regular polyhedron, skew 7 140 196 Regular polyhedron, star-polyhedron 5 16 212 217-220 Regular polyhedron, toroidal 18 364 390-391 Regular polytope regular polytope, universal 78-83 Regular polytope, abstract 31-38 Regular polytope, abstract, group of 31-38 49-58 Regular polytope, amalgamation of 96-101 Regular polytope, classical 206 Regular polytope, complex regular 293 Regular polytope, convex 7-15 31 208 Regular polytope, convex, symmetry group of 10 67 71 Regular polytope, free extension of 106-109 Regular polytope, higher toroidal 445 450-470 Regular polytope, history of 1-7 Regular polytope, infinite see Regular apeirotope Regular polytope, locally of topological type 361 Regular polytope, projective 162-165 287 502 Regular polytope, projective, locally 284 461 463 467 502-509 516 Regular polytope, quotient polytopes of 58-60 Regular polytope, realizations of see Realization Regular polytope, related to a linear group 490-501 Regular polytope, self-dual 37 Regular polytope, spherical 153 Regular polytope, spherical, locally 152-162 Regular polytope, star-polytope 16 31 206-217 Regular polytope, symmetries of see Symmetry group Regular polytope, topological model of 161 367 Regular polytope, toroidal see regular toroid Regular polytope, toroidal, locally see Locally toroidal Regular polytope, universal, in class 96-101 Regular polytope, universal, of type 78-83 Regular polytope, universal, with given facet and vertex-figure 97 Regular solids 2 11 Regular tessellation, euclidean 15 81 254 Regular tessellation, euclidean, planar 2 15 Regular tessellation, euclidean, symmetry group of 67 73 Regular tessellation, hyperbolic 81 Regular tessellation, hyperbolic, symmetry group of 67 78 Regular tessellation, on space-form 151 Regular tessellation, on space-form, euclidean 165-177 Regular tessellation, on space-form, hyperbolic 178-182 Regular tessellation, on space-form, spherical 162-165 Regular tessellation, on surface see Regular map Regular tessellation, on surface, polytopal see Regular polyhedron Regular tessellation, on torus see Regular toroid Regular tessellation, spherical 162-165 Regular tessellation, with finite or infinite cells see Regular honeycomb Regular toroid 154 Regular toroid, cubic 165-169 172-175 258 269 Regular toroid, on 2-torus 18 364 390-391 Regular toroid, other 170-175 Regular, combinatorially 11 31 152 Regular, directly 38 Regular, geometrically 156 Regular, semi-regular 15 Reinhardt, C. 17 Representation, character norm 131 Representation, character of 131 Representation, complex conjugate 130 Representation, contragredient 68 302 Representation, degree of 129 133 Representation, isomorphic 131 Representation, of Coxeter groups 64-70 Ridge of abstract polytope 23 Ridge of convex polytope 8 Ronan, M. A. 21 Root 75 Root, lattice 75 Root, lattice, 167 170 Root, system 75 Schl 208 306 314 Schl 30 Schl 293 Schl 11 Schl 17 Schl 207 Schl 6 16 85 Schulte, E. xiii 21 Section 9 23 Section, 23 Section, proper 23 Self-Petrie 193 Semi-regular 15 Sggi 35 Shephard, G. C. 289 simplex 8 11 Simplex, adjacent 39 Simplex, base 40 Simplex, dissection 432 505 Simplex, type of 39 Simplicial complex of chains see Order complex Simplicial complex of chambers see Chamber complex Skewing operation 199 Sloane, N. J. A. 170 Sommerville, D. M. Y. 86 Space, complex projective 291 Space, Euclidean 148 Space, hyperbolic 148 Space, real elliptic 150 Space, real projective 149 Space, spherical 148 Space, unitary 290 Space-form 148-152 Space-form, affinely equivalent 151 Space-form, euclidean 149 175-177 Space-form, hyperbolic 149 178-182 Space-form, isometric 150 Space-form, isometry type of 150 Space-form, orientable 150 Space-form, similar 151 Space-form, spherical 149 162-165 Space-form, tessellation on 151 Space-form, volume of 151 Star 113 Starry 207 213 Stringham, W. I. 6 Subfacet 23 Supporting hyperplane 8 Symmetries of geometric polytope 123 Symmetry group, of polytope, complex regular 293 Symmetry group, of polytope, convex regular 9 Symmetry group, of polytope, geometric 123 Symmetry group, of realization 123 Symmetry group, of regular 4-apeirotope in 236-243 Symmetry group, of regular honeycomb or tessellation euclidean 67 73 Symmetry group, of regular honeycomb or tessellation euclidean, hyperbolic 67 78 431-432 445-449 Symmetry group, of regular honeycomb or tessellation euclidean, on space-form 156 Symmetry group, of regular polyhedron in 217-220 226-236 Tessellation, chiral 178
Реклама
 |
|
О проекте
|
|
О проекте



 -polytope
-polytope  -flat
-flat 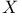


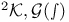

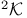
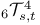

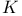
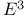

![$\{4, 2p|4^{[p/2]-1}, 2s\}$](/math_tex/f5fc7535d0a660d19f636b207ae8b3e982.gif)


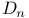
 fli determinant
fli determinant  -section
-section 