|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Eisenbud D., Harris J. — The Geometry of Schemes |
|
|
 |
| Предметный указатель |
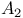 singularity 204 singularity 204
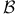 -sheaf 16 -sheaf 16
Absolutely irreducible 56
Acnode 57
Affine line 48
Affine locally 21
Affine pair 22
Affine plane 48
Affine scheme 7 8 21
Affine scheme, primary 68
Affine schemes and commutative rings 36
Affine space 33 34 47 84
Affine tangent space 104 105
Affine variety 47
Algebra, Azumaya 206
Algebra, category of -s 253 258
Algebra, coordinate 28 47
Algebra, dimension of 32 60
Algebra, division 207
Algebra, graded 91 95 100 101
Algebra, Noetherian 81
Algebra, quasicoherent sheaf of -s 40 101
Algebra, reduced 47 53
Algebra, Rees 172 173
Algebra, spectrum of 40 111
Algebra, symmetric 102 105 122 173
Algebra, tensor product of -s 37
Algebraic geometry see “Classical”
Algebraic spaces 262
Algebraic stack 276
Algebraically closed 8—11 47 48 50 58 62 65 83 104 119 140 141 151 153—156 160 163 191—193 195 203 207 213 228 231 239 243 245 248 272 276
Alonso, Leo 5
Altman, Allen 230
Analytic branch 52
Analytic functions 27
Analytic functions, sheaf of 14
Analytic manifold 8 51
Analytic spaces 116
Annihilator sheaf 220
Arithmetic genus 129 138
Arithmetic scheme 81 110 162 180 185 188 245 249
Artin, Michael 53 261
Artinian ring 65 78
Associated closed ideal 100
Associated prime 67
Associated scheme 68
Associated to a variety 47
Axiom of Choice 12
Azumaya algebra 206
Base of open sets 16
Base, change 38 54 152 154 168 209 236 237 241
Base, Grobner 75
Base, scheme 45 95 122 193 222
Basic open set 10 14 16 30
Bayer, David 138
Behrend, Kai A. 277
Betti number 127 128 130
Bezout’s Theorem 141 146 148 154
Blow-up 103 162—192
Bombieri, Enrico 1
Bourbaki, Nicolas 76 112
Brauer group 206
Brieskorn, Egbert. 52
Brill — Noether — Petri problems 1
Brown, Edgar H. 261
Buhler, Joe 5
Burch, Lindsay 133
Cartier, divisor 110 117 166 167 174
Cartier, subscheme 165 167 168 175 176 178 182
Cassels, J. W. S. 206
Castelnuovo regularity 264
Categorical, definition of morphism of sheaves 15
Categorical, definition of presheaf 12
Categorical, equivalence of affine schemes and rings 30
Category, opposite 30
Center of blow-up 165
Chevalley, Claude 210
Chinese remainder theorem 60
Choice, axiom of 12
Clemens, Herbert 5 179
Closed point 11 22 26 31 42 48 50—52 54—56 60 63 70 71 75 76 82 83 85 88 93 105 111 132 160 191 216 239 247 255 256 274 275
Closed subfunctor 255
Closed subscheme 23 24 71 100 211
Closed, locally 26
Closed, universally 95
Closure 26
Coarse moduli space 276
Cohen — Macaulay 32 146 151
Coherent sheaf 24 25 103
Cokernel 16
Collino, Alberto 265
Commutative ring see “Ring”
Compactness 19 91 93—95 229
Compatibility condition 33
Complement of a subfunctor 255
COMPLEX 8 20
Component, embedded 66 68
Component, isolated 67 68
Component, length of 68
Component, multiple 156
Component, primary 67 68
Cone over a cubic 204
Cone over a quartic 148
Cone, cubic 63
Cone, quadric 173 175 197—199
Cone, tangent 106—110 171 178 190 192 203 204
Cone, tangent, projectivized 107
conic 63 86 141 142 160 179 185 186 190 196 198 202
Conic and quartic 160
Conic, double 138 156 157
Conic, intersection of -s 143
Conic, intersection with line 59
Conic, reducible and nonsingular 87
Conic, universal 207 208
Constructible subset 209
Containment of schemes 23
Continuous functions, sheaf of 13
Coolidge, Julian L. 152 242
Coordinate ring 47
Cotangent sheaf, relative 230 231
Cotangent space, Zariski 27
Cox, David 229
Crunode 57
Cubic surface 191
Cubic universal 203 204
Cubic, lines on 202
Curvilinear scheme 65
Cusp 156 239
Decomposition, primary 67 68
Dedekind domain 71 75
Deformation, first-order 267
Degree of affine scheme 62 65
Degree of scheme 129 141
Degree of subscheme 125
Dehomogenization 98 153
Deligne, Pierre 1
Demazure, Michel 261
Diagonal subscheme 93
Dieudonne, Jean 5
Different of a polynomial 234
Dimension of scheme 27
Dimension, Krull 27 65
Dimension, relative 231
Direct limit 14
Discriminant of a polynomial 233
Discriminant, scheme 231
Distinguished open set 10 23
Division algebra 207
Divisor, Cartier 117
Divisor, exceptional 164 165
| Dominant morphism 211
Double conic 138
Double line 136
Double point 58 62 88 178 180 246
Dual curve 162 237 240
Dual projective space 103
Effective Cartier divisor 117
Elemination theory 210
Eliot, Thomas Stearns v
Elliptic curve 161
Embedded component 66 68
Embedded point 66
Equalizer 35
Equations with parameters 70
Etale, equivalence relations 262
Etale, topology 53
Euler’s relation 153
Exceptional divisor 164 165
Faithful functor 43
Faltings, Gerd 1
Family of closed subschemes 71
Family of curves 156
Family of schemes 70
Family, flat 75
Family, limit of 71
Family, universal 222
Fano, scheme 120 193—205 266 273
Fano, scheme, tangent space to 271
Fano, scheme, universal 203
Fano, variety, universal 204
Fantechi, L. 277
Fiber 31 70
Fibered coproduct 37
Fibered product 35 36 254 260
Fibered sum 37
Finite morphism 92
Finite scheme 62
Finite type 76 210 231
Finite type, morphism of 92
Finitely generated 8 47 50
First-order deformation 267
First-order neighborhood 58 67
Fitting ideal 220 221 225 231 232 236
Fitting image 219 221—224
Fitting scheme 220
Fitting’s Lemma 219
Flat, family 75 125
Flat, limit 77
Flat, module 75
Flat, scheme 32
Flatness, geometric interpretation of 125
Flatness, theorem 79
Flex 151 152
FLOP 179
Fong, Lung-Ying 138
Form (type of scheme) 205
Forster, Otto 18
Four Quartets v
Free resolution 127 128 133 134 144
Frobenius automorphism 56
Frohlich, Albrecht 206
Fulton, William 148 277
Functor of nonsingular curves 274
Functor of points 42 43 252
Functor, faithful 43
Functor, injective 254
Functor, represent able 44
Functor, tangent space to 256
Gabriel, Pierre 261
Galois, cohomology 206
Galois, group 40 56 85 87
Gashorov, Vesselin 5
Gathmann. Andreas 5
Generic, flatness theorem 79
Generic, hypersurface 123
Generic, point 9 48
Genus, arithmetic 129 138
Geometrically irreducible 56
Germ of analytic function 14
Germ of variety 50
Global Proj 102 170
Global regular function 22
Global section 12
Global Spec 40 41
Gluing 33
Godement, Roger 18
Gottsche, Lothar 277
Graber, Tom 5
Graded algebra 91 95 100 101 170
Graded Betti number 127 128 130
Graded module 118
Graded ring, Proj of 95
Graded sheaf 118
Grading 34 96
Grassmannian 63 119 193 195 201 206 207 261 263 264 272 273
Grassmannian, functor 261
Green, Mark L. 130
Griffiths, Phillip 1 155 192 203
Grobner bases 75 229
Gross, Benedict 5
Grothendieck, Alexandre 1 5 18 79 261
Group scheme 258
Gruson, Laurent 77
Gunning, Robert C. 18
Hartshorne, Robin 4 5 18 75 95 101 110 125 126 129 155 162 210 218
Hassett, Brendan 5
Hausdorff 11 19 91 93 95
Henselization 52
hessian 153—155 157
Hilbert, David 48 125 128
Hilbert, function 124—130
Hilbert, functor 262
Hilbert, polynomial 125 129. 143 262—264
Hilbert, scheme 120 129 259 262 264 266
Hilbert, scheme, tangent space to 267
Hilbert, series 149 150
Hilbert, theorem 125
Hilbert, theorems 128
Hironaka, Heisuke 261
Homogeneous, coordinate ring 96
Homogeneous, element 96
Homogeneous, ideal 96
Homogenization 98
Hyperplane, universal 124
Hypersurface 122 141
Hypersurface, generic 123
Hypersurface, universal 123
Iarrobino, Anthony 64 265
Ideal see “Prime maximal minimal”
Ideal of minors 219
Ideal, Fitting 220 221 225 231 232 236
Ideal, sheaf 24
Image of morphism 209
Image, Fitting 219 221—224
Image, reduced 222
Image, scheme-theoretic 222 223
Image, set-theoretic 209 222
Infinitely near point 59
Injective functor 254
Intersection of schemes 24
Intersection, multiplicity 141 148 151
Invariance under base change 209
Invariants of projective scheme 124
Inverse of a sheaf 117
Inverse, image see “Preimage”
Inverse, limit 17 53
Invertible, module 113
Invertible, sheaf 112 116 117
Irreducible scheme 25
Irreducible scheme, absolutely 56
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



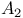 singularity
singularity 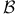 -sheaf
-sheaf