|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Keller K. — Invariant Factors, Julia Equivalences and the (Abstract)Mandelbrot Set |
|
|
 |
| Предметный указатель |
 (renormalization) 162 (renormalization) 162
 5 5
 (sequence tuning) 92 (sequence tuning) 92
 (space of compact subsets of (space of compact subsets of  ) 32 ) 32
 (diameter of an equivalence relation (diameter of an equivalence relation 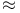 on on  ) 63 ) 63
 32 32
 (factor map) 5 (factor map) 5
 (infinite gap in (infinite gap in 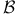 related to related to  ) 86 ) 86
 (critical value gap of (critical value gap of  ) 91 ) 91
 (impression of (impression of  ) 155 ) 155
 (impression of (impression of  ) 155 ) 155
 (Julia set corresponding to a renormalization of (Julia set corresponding to a renormalization of  ) 14 ) 14
 (filled-in Julia set corresponding to a renormalization of (filled-in Julia set corresponding to a renormalization of  ) 14 ) 14
 ('periodic' parameter chord associated to a dynamic pair ('periodic' parameter chord associated to a dynamic pair  ) 129 ) 129
 (X factorized by an equivalence relation (X factorized by an equivalence relation  ) 4 ) 4
![$[;{k}_{1},\;{k}_{2},{k}_{3},...(,{k}_{n})]$](/math_tex/1914278b6f5a3ec8a72839279d042bac82.gif) (continued fraction) 103 (continued fraction) 103
 structure graph 41 structure graph 41
 -tuning 99 -tuning 99
 (substantially equal) 26 (substantially equal) 26
 (preperiodic preimage of (preperiodic preimage of  ) 79 ) 79
 (preperiodic preimage of (preperiodic preimage of  ) 30 ) 30
 (periodic preimage of (periodic preimage of  ) 30 ) 30
 ( ( for for  ) 86 ) 86
 (set of accumulation chords of (set of accumulation chords of  ) 45 ) 45
 -sublimb 20 -sublimb 20
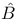 (kneading sequence of a 'periodic' parameter chord) 79 (kneading sequence of a 'periodic' parameter chord) 79
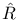 ('generalized' kneading sequence) 124 ('generalized' kneading sequence) 124
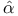 (kneading sequence of (kneading sequence of  ) 6 ) 6
 (ratio between 0-length and 1-length of (ratio between 0-length and 1-length of  ) 102 ) 102
 (1-frequency of an (1-frequency of an  ) 102 ) 102
 (with periodic a associated point) 53 (with periodic a associated point) 53
 (counter-clockwise itinerary) 26 (counter-clockwise itinerary) 26
 (clockwise itinerary) 26 (clockwise itinerary) 26
 (uniformization map for (uniformization map for  ) 15 ) 15
 (order on the set of Julia equivalences) 91 (order on the set of Julia equivalences) 91
 (Tent map) 185 (Tent map) 185
 (equivalence relation defining the Abstract Mandelbrot set) 16 (equivalence relation defining the Abstract Mandelbrot set) 16
 (specified itinerary) 62 (specified itinerary) 62
 (special subset of the circle) 30 (special subset of the circle) 30
 (special subset of the disk) 41 (special subset of the disk) 41
 (special chord in (special chord in  ) 81 ) 81
 (number of components of (number of components of  ) 41 ) 41
 (combinatorial class of M) 17 (combinatorial class of M) 17
 (landing point of (landing point of  ) 149 ) 149
 (characteristic symbol of a chord (characteristic symbol of a chord 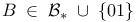 ) 79 ) 79
 (characteristic symbol of a periodic (characteristic symbol of a periodic  ) 51 ) 51
 (special infinite gap in (special infinite gap in  ) 56 ) 56
 (itinerary) 6 (itinerary) 6
 (Julia set) 2 (Julia set) 2
 (small filled-in Julia set corresponding to a renormalization) 14 (small filled-in Julia set corresponding to a renormalization) 14
 (filled-in Julia set) 2 (filled-in Julia set) 2
 (special maps on the circle) 30 (special maps on the circle) 30
 (special maps on the circle) 30 (special maps on the circle) 30
 (multiplier map on clW) 19 (multiplier map on clW) 19
 (internal address) 81 (internal address) 81
 (quadratic polynomial) 1 (quadratic polynomial) 1
 (inversion of binary expansion) 91 (inversion of binary expansion) 91
 (generalization of (generalization of  ) 92 ) 92
 (map on (map on  associated with associated with  ) 93 ) 93
 (preperiodic chord associated with an immediately visible chord in {\mathcal B}_{*}) 127 (preperiodic chord associated with an immediately visible chord in {\mathcal B}_{*}) 127
 (set of recurrent Sturmian sequences of 1-frequency (set of recurrent Sturmian sequences of 1-frequency  ) 104 ) 104
 (special set of Sturmian sequences) 101 (special set of Sturmian sequences) 101
 109 109
 36 36
 (chord corresponding to a component K of (chord corresponding to a component K of  ) 42 ) 42
 38 38
 36 36
 (hyperbolic component related to (hyperbolic component related to  ) 19 ) 19
 (periodic/preperiodic point map) 18 (periodic/preperiodic point map) 18
 (landing point of (landing point of  ) 10 ) 10
![${[A]}_{\equiv}$](/math_tex/9cb6fc66d02a2360f3c6526693f82db982.gif) (transitive hull of A with respect to (transitive hull of A with respect to  ) 4 ) 4
![${[x]}_{\equiv}$](/math_tex/2b59e0083dee70c35e4778b92dd364bd82.gif) (equivalence class) 4 (equivalence class) 4
 (Julia equivalence) 59 (Julia equivalence) 59
 clockwisely ordered points in clockwisely ordered points in 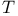 ) 33 ) 33
 clockwisely ordered points in clockwisely ordered points in 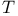 ) 33 ) 33
 (chord with end points (chord with end points  ) 32 ) 32
 (0—1-word a after interchanging 0 and 1) 27 (0—1-word a after interchanging 0 and 1) 27
 (binary expansion of (binary expansion of  ) 5 ) 5
 (curve associated to a 'parameter' chord) 149 (curve associated to a 'parameter' chord) 149
 (curve associated to a 'dynamic' chord) 146 (curve associated to a 'dynamic' chord) 146
 (curve defined by two parameter rays) 149 (curve defined by two parameter rays) 149
 (curve defined by two dynamic rays) 146 (curve defined by two dynamic rays) 146
 (equipotential curve in the dynamic plane) 157 (equipotential curve in the dynamic plane) 157
 (special periodic sequence related to renormalization) 109 (special periodic sequence related to renormalization) 109
 (parameter ray) 15 (parameter ray) 15
 (standard dynamic ray) 7 (standard dynamic ray) 7
 (dynamic ray) 9 (dynamic ray) 9
 (special symbol sequence) 107 (special symbol sequence) 107
 (0—1-sequence associated with a gap G in (0—1-sequence associated with a gap G in  ) 84 ) 84
 (initial subword of (initial subword of 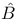 of length PER(B) - 1) 79 of length PER(B) - 1) 79
 (initial subword of (initial subword of 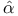 of length PER(a) - 1) 26 of length PER(a) - 1) 26
 (wake associated to (wake associated to  ) 17 ) 17
 (n-fold concatenation of a word w) 25 (n-fold concatenation of a word w) 25
 (successor function) 41 (successor function) 41
 (special subset of the disk) 39 (special subset of the disk) 39
 (periodic preimage of (periodic preimage of  ) 79 ) 79
 (identification associated with binary expansion) 5 (identification associated with binary expansion) 5
 ('composition' of two equivalence relations) 183 ('composition' of two equivalence relations) 183
 ('quotient' of two equivalence relations) 115 184 ('quotient' of two equivalence relations) 115 184
 ('composition' of two equivalence relations) 175 ('composition' of two equivalence relations) 175
 (special equivalence relation on (special equivalence relation on  ) 173 ) 173
 (special equivalence relation on (special equivalence relation on  ) 173 ) 173
 (kneading sequence just behind B) 80 (kneading sequence just behind B) 80
 (kneading sequence just before B) 80 (kneading sequence just before B) 80
 (counter-clockwise kneading sequence) 26 (counter-clockwise kneading sequence) 26
 (clockwise kneading sequence) 26 (clockwise kneading sequence) 26
 (empty word) 25 (empty word) 25
 ( ( less some special parameter rays) 148 less some special parameter rays) 148
 (unit disk) 32 (unit disk) 32
 40 40
 39 39
 110 110
 (points with periodic kneading sequence) 26 (points with periodic kneading sequence) 26
 (points being periodic with respect to h) 26 (points being periodic with respect to h) 26
 (points in (points in  , of period dividing n) 77 , of period dividing n) 77
 (non-periodic points with periodic kneading sequence) 27 (non-periodic points with periodic kneading sequence) 27
 47 47
 (words over the alphabet (words over the alphabet  ) 25 ) 25
 (words in (words in  of length n) 25 of length n) 25
 (sequences over the alphabet (sequences over the alphabet  ) 25 ) 25
 (circle) 4 (circle) 4
 91 91
 (special subset of the circle) 30 (special subset of the circle) 30
 (special set of the circle) 30 (special set of the circle) 30
 (n-renormalization domain) 95 108 (n-renormalization domain) 95 108
 (closure of (closure of 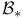 ) 84 ) 84
 ( ( for for  ) 123 ) 123
 (invariant lamination constructed from a 'long' chord (invariant lamination constructed from a 'long' chord  ) 40 ) 40
 (set of chords 'generating' (set of chords 'generating'  ) 55 ) 55
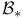 ('periodic' parameter chords) 80 ('periodic' parameter chords) 80
 (set of chords for the construction (set of chords for the construction  ) 39 ) 39
 (set of chords for the construction (set of chords for the construction  ) 36 ) 36
 (sets of chords for the construction of (sets of chords for the construction of  ) 36 ) 36
 (visibility tree) 22 (visibility tree) 22
 ( ( structure graph) 41 structure graph) 41
 ('rotation number') 105 ('rotation number') 105
 25 25
 (Riemann sphere) 7 (Riemann sphere) 7
 (Boetkher map) 7 (Boetkher map) 7
 (projection onto the factor) 4 (projection onto the factor) 4
 (shift map) 6 25 (shift map) 6 25
 (Lavaurs' equivalence relation) 78 (Lavaurs' equivalence relation) 78
 (backward-shifts) 184 (backward-shifts) 184
 (conjugacy related to renormalization) 115 (conjugacy related to renormalization) 115
' (rotation by 180°) 4
0—1-sequence, recurrent 102
1-frequency 102
1-length 30
A(k) (0—1-word/0—1-sequence derived from  27 27
Abstract Julia set 12
| Abstract Mandelbrot set 16
Address of a point 186
ALPHA fixed point 163
Angle, external 9
Angle, internal 20
Angle, n-renormalizable 108
Angle, n-renormalizable, simply 112
Backward iterate 7
Backward orbit 7
Basin, immediate 141
Behind, a chord 32
Behind, a hyperbolic component 20
Behind, the chord 01 80
BETA fixed point 163
Between, chords 32
Between, points 32
Bifurcate 20
Binary expansion 5
Boetkher map 8
Bound a gap 34
Boundary chord of a gap 34
Brjuno number 170
Bucket handle 189
Cantor set 49
Center of a hyperbolic component 169
Characteristic symbol 51
Characteristic symbol of a chord in  79 79
Chord 32
Chord, degenerate 32
Chord, narrow 137
Chord, non-degenerate 32
Chord, separating 32
Combinatorial class 17
Combinatorially smaller 78
Composant 189
Conjugacy 4
conjugate 4
Continuum 71
Contraction 185
Cremer point 142
Crossing chords 32
D (distance on  ) 32 ) 32
D(S) (length of a chord S) 32
Dendrite 71
Diameter of 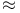 63 63
DPair (Q) (dynamic pair associated to a 'periodic' parameter chord Q) 130
Dynamic pair 123
Dynamic pair, parameter-like 123
Dynamic pair, semi-visible 131
Dynamic pair, visible 123
Equivalence relation,  degenerate 12 degenerate 12
Equivalence relation,  non-degenerate 12 non-degenerate 12
Equivalence relation,  planar 11 planar 11
Equivalence relation, closed 182
Equivalence relation, closed as a subset of the product space 4
Equivalence relation, completely f-invariant 4
Equivalence relation, completely invariant 5
Equivalence relation, f-invariant 4
Equivalence relation, invariant 5
Equivalence relation, invariant, generated 184
Factor map 5
Factor, (completely) invariant 5
Fatou component 141
Fatou component, bounded 141
Fatou set 141
Filled-in Julia set 2
Filled-in Julia set of a quadratic-like map 13
Filled-in Julia set small 14
Flow 188
Gap 34
Gap(B) (infinite gap in 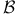 related to related to 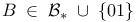 ) 86 ) 86
Gap, critical 34
Gap, critical value 34
Gap, infinite 34
Gap, polygonal 34
Gap<S> (critical value gap in  ) 123 ) 123
generation rules 184
Generation, algebraic 187
Horseshoe 189
Hybrid-equivalent 13
Hyperbolic component 18
Hyperbolic component, main 19
Impression, of a dynamic ray 155
Impression, of a parameter ray 155
Internal address 81
Internal address, angled 161
Iterate 7
Itinerary 6
Itinerary, specified 62
J(f) (Julia set of a quadratic-like map f) 13
Julia set 2
Julia set of a quadratic-like map 13
K(f) (filled-in Julia set of a quadraticlike map f) 13
Kneading sequence 6
Kneading sequence, admissible 83
Kneading sequence, just before a chord in 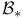 80 80
Kneading sequence, just behind a chord in  80 80
Kneading sequence, of a chord in  79 79
Lamination 34
Lamination, invariant 34
Land 9
Landing pattern 143
Landing point 9 15
Locally connected 10
Locally connected, at a point 10
M (Mandelbrot set) 2
Main point 65
Mandelbrot set 2
Map, angle-doubling 4
Map, hyperbolic 162
Map, polynomial-like 13
Map, quadratic-like 13
Match 189
Matchbox 189
Matchbox manifold 189
Misiurewicz point 142
Multiplier 8
Multiplier of a parameter 142
N-renormalization domain 108
Orbit 7
Orbit, periodic 7
Orbit, periodic, attractive 8
Orbit, periodic, indifferent 8
Orbit, periodic, irrationally indifferent 8
Orbit, periodic, parabolic 8
Orbit, periodic, repelling 8
Orbit, periodic, superattractive 8
Orbit, postcritical 8
Parameter, hyperbolic 18 142
Parameter, irrationally indifferent 142
Parameter, parabolic 142
Parameter, superattractive 142
Partition 27
Partition,  -regular 28 -regular 28
Partition, n-regular 28
Partition, n-regular derived 28
PER(B) (period of a parameter chord B 79
PER(x) (period of a point x) 7
Period, of a chord in  79 79
Period, of a hyperbolic component 18
Period, of a parameter 142
Period, of a point 7
Period, of a preperiodic point 7
Period, of an orbit 7
Point, critical 8
Point, fixed 7
Point, periodic 7
Point, periodic, associated 53
Point, periodic, attractive 8
Point, periodic, indifferent 8
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 (renormalization)
(renormalization) 
 (sequence tuning)
(sequence tuning)  (space of compact subsets of
(space of compact subsets of  )
)  (diameter of an equivalence relation
(diameter of an equivalence relation 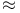 on
on  )
) 
 (factor map)
(factor map)  (infinite gap in
(infinite gap in 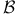 related to
related to  )
)  (critical value gap of
(critical value gap of  )
)  (impression of
(impression of  )
)  (impression of
(impression of  )
)  (Julia set corresponding to a renormalization of
(Julia set corresponding to a renormalization of  )
)  (filled-in Julia set corresponding to a renormalization of
(filled-in Julia set corresponding to a renormalization of  ('periodic' parameter chord associated to a dynamic pair
('periodic' parameter chord associated to a dynamic pair  )
)  (X factorized by an equivalence relation
(X factorized by an equivalence relation  )
) ![$[;{k}_{1},\;{k}_{2},{k}_{3},...(,{k}_{n})]$](/math_tex/1914278b6f5a3ec8a72839279d042bac82.gif) (continued fraction)
(continued fraction)  structure graph
structure graph  (substantially equal)
(substantially equal)  (preperiodic preimage of
(preperiodic preimage of  )
)  (preperiodic preimage of
(preperiodic preimage of  )
)  (periodic preimage of
(periodic preimage of  for
for  )
)  (set of accumulation chords of
(set of accumulation chords of  )
)  -sublimb
-sublimb 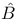 (kneading sequence of a 'periodic' parameter chord)
(kneading sequence of a 'periodic' parameter chord) 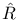 ('generalized' kneading sequence)
('generalized' kneading sequence) 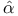 (kneading sequence of
(kneading sequence of  )
)  (ratio between 0-length and 1-length of
(ratio between 0-length and 1-length of  )
)  (1-frequency of an
(1-frequency of an  (with periodic a associated point)
(with periodic a associated point)  (counter-clockwise itinerary)
(counter-clockwise itinerary)  (clockwise itinerary)
(clockwise itinerary)  (uniformization map for
(uniformization map for  )
)  (order on the set of Julia equivalences)
(order on the set of Julia equivalences)  (Tent map)
(Tent map)  (equivalence relation defining the Abstract Mandelbrot set)
(equivalence relation defining the Abstract Mandelbrot set)  (specified itinerary)
(specified itinerary)  (special subset of the circle)
(special subset of the circle)  (special subset of the disk)
(special subset of the disk)  (special chord in
(special chord in  )
)  (number of components of
(number of components of  )
)  (combinatorial class of M)
(combinatorial class of M)  (landing point of
(landing point of  )
)  (characteristic symbol of a chord
(characteristic symbol of a chord 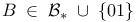 )
)  (characteristic symbol of a periodic
(characteristic symbol of a periodic  (special infinite gap in
(special infinite gap in  (itinerary)
(itinerary)  (Julia set)
(Julia set)  (small filled-in Julia set corresponding to a renormalization)
(small filled-in Julia set corresponding to a renormalization)  (filled-in Julia set)
(filled-in Julia set)  (special maps on the circle)
(special maps on the circle)  (special maps on the circle)
(special maps on the circle)  (multiplier map on clW)
(multiplier map on clW)  (internal address)
(internal address)  (inversion of binary expansion)
(inversion of binary expansion)  (generalization of
(generalization of  (preperiodic chord associated with an immediately visible chord in {\mathcal B}_{*})
(preperiodic chord associated with an immediately visible chord in {\mathcal B}_{*})  (set of recurrent Sturmian sequences of 1-frequency
(set of recurrent Sturmian sequences of 1-frequency  )
)  (special set of Sturmian sequences)
(special set of Sturmian sequences) 

 (chord corresponding to a component K of
(chord corresponding to a component K of 

 (hyperbolic component related to
(hyperbolic component related to  (periodic/preperiodic point map)
(periodic/preperiodic point map)  (landing point of
(landing point of  )
) ![${[A]}_{\equiv}$](/math_tex/9cb6fc66d02a2360f3c6526693f82db982.gif) (transitive hull of A with respect to
(transitive hull of A with respect to ![${[x]}_{\equiv}$](/math_tex/2b59e0083dee70c35e4778b92dd364bd82.gif) (equivalence class)
(equivalence class)  (Julia equivalence)
(Julia equivalence)  clockwisely ordered points in
clockwisely ordered points in 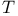 )
)  clockwisely ordered points in
clockwisely ordered points in  (chord with end points
(chord with end points  )
)  (0—1-word a after interchanging 0 and 1)
(0—1-word a after interchanging 0 and 1)  (binary expansion of
(binary expansion of  )
)  (curve associated to a 'parameter' chord)
(curve associated to a 'parameter' chord)  (curve associated to a 'dynamic' chord)
(curve associated to a 'dynamic' chord)  (curve defined by two parameter rays)
(curve defined by two parameter rays)  (curve defined by two dynamic rays)
(curve defined by two dynamic rays)  (equipotential curve in the dynamic plane)
(equipotential curve in the dynamic plane)  (special periodic sequence related to renormalization)
(special periodic sequence related to renormalization)  (standard dynamic ray)
(standard dynamic ray)  (dynamic ray)
(dynamic ray)  (special symbol sequence)
(special symbol sequence)  (0—1-sequence associated with a gap G in
(0—1-sequence associated with a gap G in  )
)  (initial subword of
(initial subword of  (initial subword of
(initial subword of  (wake associated to
(wake associated to  )
)  (n-fold concatenation of a word w)
(n-fold concatenation of a word w)  (successor function)
(successor function)  (special subset of the disk)
(special subset of the disk)  (periodic preimage of
(periodic preimage of  (identification associated with binary expansion)
(identification associated with binary expansion)  ('composition' of two equivalence relations)
('composition' of two equivalence relations)  ('quotient' of two equivalence relations)
('quotient' of two equivalence relations)  ('composition' of two equivalence relations)
('composition' of two equivalence relations)  (special equivalence relation on
(special equivalence relation on  )
)  (special equivalence relation on
(special equivalence relation on  (kneading sequence just behind B)
(kneading sequence just behind B)  (kneading sequence just before B)
(kneading sequence just before B)  (counter-clockwise kneading sequence)
(counter-clockwise kneading sequence)  (clockwise kneading sequence)
(clockwise kneading sequence)  (empty word)
(empty word)  (
( less some special parameter rays)
less some special parameter rays) 


 (points with periodic kneading sequence)
(points with periodic kneading sequence)  (points being periodic with respect to h)
(points being periodic with respect to h)  (points in
(points in  (non-periodic points with periodic kneading sequence)
(non-periodic points with periodic kneading sequence) 
 (words over the alphabet
(words over the alphabet  )
)  (words in
(words in  (sequences over the alphabet
(sequences over the alphabet 
 (special subset of the circle)
(special subset of the circle)  (special set of the circle)
(special set of the circle)  (n-renormalization domain)
(n-renormalization domain) 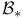 )
)  (
( for
for  )
)  (invariant lamination constructed from a 'long' chord
(invariant lamination constructed from a 'long' chord  )
)  (set of chords 'generating'
(set of chords 'generating'  )
)  (set of chords for the construction
(set of chords for the construction  (set of chords for the construction
(set of chords for the construction  (sets of chords for the construction of
(sets of chords for the construction of  (visibility tree)
(visibility tree)  ('rotation number')
('rotation number') 
 (Riemann sphere)
(Riemann sphere)  (Boetkher map)
(Boetkher map)  (projection onto the factor)
(projection onto the factor)  (shift map)
(shift map)  (Lavaurs' equivalence relation)
(Lavaurs' equivalence relation)  (backward-shifts)
(backward-shifts)  (conjugacy related to renormalization)
(conjugacy related to renormalization) 
 degenerate
degenerate  -regular
-regular