Quadratic equations in Diophantus 7—8 66
Quadratic equations in Euclid 63
Quadratic equations in Heron 63 64
Quadratic equations in Hippocrates of Chios 63
Quadratic inequalities in Diophantus 60—63 5
Quadratic inequalities, limits to roots 60—63 65 95
Qust b. L b. L q q 19 19
Radice (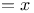 ) 40 ) 40
Radix (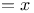 ) 38 ) 38
Rahn 50 n. 286
Ramus 16 n.
Rationality, Diophantus’ view of 52—53
Recorde, Robert 50 n.
Regiomontanus 5 17 30 33 49
Relato, Italian term for certain powers of unknown 41
Res, alternative for radix, in sense of unknown quantity 38
Rhind papyrus 113
Right-angled triangles in rational numbers in Diophantus 93—94 105—106
Right-angled triangles in rational numbers in Diophantus, Euclid’s formula for 117 120
Right-angled triangles in rational numbers in Diophantus, Fermat’s theorems and problems on 304—205 n. 218—219 220 229 230 231—232 235 236 239—240 293—318 364—371
Right-angled triangles in rational numbers in Diophantus, Greek indeterminate problems on, other than those of Dioph. 119—131
Right-angled triangles in rational numbers in Diophantus, method of “forming” 93—94
Right-angled triangles in rational numbers in Diophantus, other methods of forming attributed to Pythagoras 116—117
Right-angled triangles in rational numbers in Diophantus, other methods of forming attributed to to Plato 116—117
Right-angled triangles in rational numbers in Diophantus, Pythagorean formula once used by Diophantus 342
Rodet 34 35
Rosen 50
Rudio 63 n.
Rudolff, Christoff 23 50
Salmasius, Claudius 17
Sand-reckoner of Archimedes 122
Saunderson, N. 27 n.
Sch ne 43 45 118 ne 43 45 118
Schaewen, P. v 327 328
Schmeisser 31
Schreiber, H. see Grammateus
Schuler, Wolfgang 24
Schulz 9 11 18 30 31 108 140 219
Sebastian Theodoric 24
Serenus 12
Simon Simonius Lucensis 25
Simplicius 63 n.
Sirmondus, J. 27
Smith, H. J. S. 292
Speusippus on polygonal numbers 125
Square root,  (side) 65 n. (side) 65 n.
Square root, sign 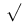 for 50 n. for 50 n.
Squares, numbers as sum of two 105—107 268—271
Squares, numbers as sum of two, three, or four 110 273 274
Squares, numbers as sum, not of three 108 109 273
Squares, numbers as sum, not of two 107—108 271—272
Squares, numbers as sum, of three 272—273
Stevin, Simon 29 30
Stifel, M. 23 49 50
Submultiples, decomposition of fractions into 46 112
Submultiples, sign for 45—47
Submultiples, submultiples of unknown and powers 47
| Subtraction, symbol for 41—44
Suidas 1 18 22
Surdesolides, sursolida or supersolida 41
Surds 23—24
Suter, H. 19 n.
Tannery, P. 2 n. 3 5 6 8 10—12 14—19 25 28 31 32—37 43—44 45 108 111 118 125 135 138 144 148 150 156 160 198 219 234 256 278 279 280 281 290 308
Tanto, unknown quantity, in Bombelli 22
Tartaglia 21 40
Theaetetus 124
Theon of Alexandria 2 18
Theon of Smyrna 2 36 117 126 310
Theudius 124
Thompson, D’Arcy W. 37
Thymaridas, Epanthetna of 114—116
Unknown quantity (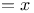 ), ), 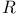 (Radix or Res) 38 (Radix or Res) 38
Unknown quantity (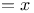 ), ),  first used by Descartes 50 n. first used by Descartes 50 n.
Unknown quantity (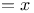 ), called in Diophantus, ), called in Diophantus, 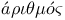 “number”, definition of 32 115 130 “number”, definition of 32 115 130
Unknown quantity (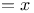 ), called in Diophantus, ), called in Diophantus, 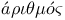 “number”, symbol for 32—37 130 “number”, symbol for 32—37 130
Unknown quantity (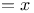 ), Egyptian scale 41 ), Egyptian scale 41
Unknown quantity (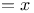 ), Italian — Arabian and Diophantine scales of powers 40 41 ), Italian — Arabian and Diophantine scales of powers 40 41
Unknown quantity (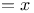 ), other signs for, ), other signs for, 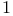 , used by Bombelli 22 38 , used by Bombelli 22 38
Unknown quantity (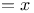 ), Radice, Lato, Cosa 40 n. ), Radice, Lato, Cosa 40 n.
Unknown quantity (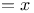 ), signs for powers of 38 129 ), signs for powers of 38 129
Unknown quantity (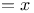 ), signs for submultiples of unknown and powers 47 130 ), signs for submultiples of unknown and powers 47 130
Vacca, G. 106 n.
Valla, Georgius 48
Vatican MSS. of Diophantus 5 15 16 17
Vergetius, Angelus 16
Vieta 27 38—39 49
Vieta, son 101 102 214 285 329 331
Vossius 31
Wallis 40286 287 288 289
Weber and Wellstein 107 n. 145
Weber, Heinrich 3 n.
Wertheim 30 110 137 138 145 151 161 209 211 212 216 217 254 256 257 286 294 295
Westermann 125 n.
Widman 49 n.
Wieferich 145 n.
Woepcke 5 n.
Xylander 17 22—26 27 28 29 35 38 107—108 140
Xylander, Xylander’s MS. of Diophantus 17 25 36
Zensus (—Censo), term for square of unknown quantity 38
Zetetica of Vieta 27 101 285
Zeuthen 118—121 205 278 281 290 294—295
“Cube-cube” (=sixth power of unknown, or 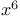 ), sign for 38 129 ), sign for 38 129
“Diagonal-” numbers 117 118 310
“False supposition”, use of, in Egypt 112—113
“Pellian” equation, origin of this erroneous term 386
“Regula falsi” in Egypt 113—113
“Side-” and “diagonal-” numbers, Pythagorean solution of 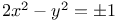 by means of 117—118 278 310 by means of 117—118 278 310
“Side” =square root 65 n.
“Species” (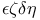 ) of algebraical quantities 7 130 131 ) of algebraical quantities 7 130 131
“Square-cube” ( 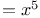 ), sign for 38 129 ), sign for 38 129
“Square-square” (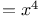 ), sign for 38 129 ), sign for 38 129
“Triple-equations” of Fermat 163 n. 179 182 202 223 224 246 321—328
“Units” (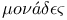 )=absolute term 39—40 )=absolute term 39—40
“Units” (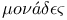 )=absolute term, abbreviation for 39 130 )=absolute term, abbreviation for 39 130
“Wurm’s problem” 123
|
 |
|
О проекте
|
|
О проекте



 b. L
b. L q
q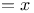 )
)  ne
ne  (side)
(side) 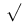 for
for 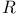 (Radix or Res)
(Radix or Res)  first used by Descartes
first used by Descartes 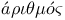 “number”, definition of
“number”, definition of 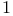 , used by Bombelli
, used by Bombelli 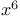 ), sign for
), sign for 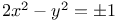 by means of
by means of 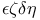 ) of algebraical quantities
) of algebraical quantities 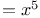 ), sign for
), sign for 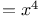 ), sign for
), sign for  )=absolute term
)=absolute term