|
|
 |
| јвторизаци€ |
|
|
 |
| ѕоиск по указател€м |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Burn R.P. Ч Numbers and Functions: Steps to Analysis |
|
|
 |
| ѕредметный указатель |
 , countably infinite 4.25 , countably infinite 4.25
 , defined 2.1 , defined 2.1
Abel 5.91 5H 12.40 12H
Absolute convergence 5.66Ч5.67 2.77
Absolute value 2.52Ч2.64 3.33 3.54
Accumulation point (cluster point) 4.48
Alternating Series Test 5.62Ч5.63
Anti-derivative 10.52 10.54
Arc cosine 10.49Ч10.50
Arc length 10.43Ч10.48
Arc sine 8.44
Arc tangent 1.8 5.84 8.45 11.60Ч11.62
Archimedean order 3.19 3H
Archimedes 1H 3H 5H 10H
Area 10.1Ч10.9
Area under  10.6 10.9 10.6 10.9
Area under  10.1 10.1
Area under 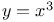 10.2 10.2
Area under  10.3Ч10.5 10.3Ч10.5
Arithmetic mean 2.30Ч20.39
Ascoli 4H
Associative law 5.1
Asymptote 7.4
Barrow 10H
Berkeley, Bishop 8H
Bernoulli, Jacques 1H
Bernoulli, Jacques, inequality 2.29 3.21
Bernoulli, Johann 8H 9H
Bijection 6.5 6.6
Binomial theorem 1.5 5.98 5.112Ч5.113 8H 11H 12.45 12H
Blancmange function 12.46
Bolzano 2H 4.53 4H 5H 6H 7.12 7H 12H
Bolzano Ч Weierstrass theorem 4.46 4.53
Bound 3.48
Bound, lower: for sequence 3.5Ч3.7
Bound, lower: for set 4.53 7.8
Bound, upper: for sequence 3.5Ч3.7
Bound, upper: for set 4.53 7.8
Bounded sequence 3.5 3.13Ч3.14 3.62 4.43Ч4.47 4.83Ч4.84 5.25
Bounded sequence, convergent subsequence 3.80 4.46Ч4.47 4.83Ч4.84
Bounded sequence, eventually 3.13 3.62
Bounded sequence, monotonic 3.80 4.33Ч4.35
Bouquet and Briot 5H
Bourbaki 6H
Briggs 11H
Burkill 11.1 11.29
Cantor 4.53 4H 6H 9H App. App.
Cauchy 2H 3H 4H 5H 6.7 6.55 6H 7H 8.20 8H 9H 10.39 10.54 10.59 10H 11.32 11H 12.45 12H App. 3.25
Cauchy product 5.108Ч5.113 5H
Cauchy sequence 4.32 4.55Ч4.57 4H 5.18 7H
Cauchy Ч Hadamard formula 5.99Ч5.102
Cauchy's mean value theorem 9.25
Cauchy's ntr root test 5.35Ч5.39 5.80Ч5.83
Cavalieri 9H 10H
Chain rule 8.18 8.33 8H
Chinese Box Theorem 4.42
Circle of convergence 5.94 5.103Ч5.105 12.40
Circle, circumference of 2.39 12.48
Circular functions 10.39Ч10.62
Closed interval 3.78 7.8
Closed interval, nested 4.42
Cluster point 4.48 4.49Ч4.51 4.53 8.5
Comparison test, first 5.26
Comparison test, limit form of second 5.55
Comparison test, second 5.53
Completeness principle 4.31
Completing the square 2.40Ч4.42
Concave 9.24
Conditional convergence 5.71Ч5.76
Connected sets 7.8 7.21
Contiguous functions 6.96
Continued fraction 4.58
Continuity of composite functions 6.40
Continuity of contiguous functions 6.96
Continuity of differentiable functions 8.12
Continuity of mono tonic functions 7.7
Continuity of polynomials 6.29
Continuity of products of functions 6 26
Continuity of quotients of functions 6.54
Continuity of reciprocals of functions 6.52
Continuity of sums of functions 6.23
Continuity on closed intervals 7.29Ч7.35 7.43
Continuity on intervals 7.8Ч7.20 7.38
Continuity or limits of functions 12.20Ч12.23
Continuity, by limits 6.86 6.89
Continuity, by neighbourhoods, defined 6.64
Continuity, by sequences, defined 6.18
Continuity, by sequences, equivalent to neighbourhoods 6.67Ч6.68
Continuity, squeeze rule 6.36
Continuity, uniform 7.37Ч7.45
Continuous at one point 6.35 8.24
Continuous at one point, bounded in neighbourhood 6.69
Continuous at one point, positive in neighbourhood 6.70
Continuous everywhere, differentiable nowhere 12.46
Continuous function on closed intervals 7.29Ч7.35 7.43
Continuous function on intervals 7.8Ч7.10 7.37
Continuous function, absolute value of 6.41
Continuous function, invertible 7.22Ч7.27
Contraction mapping 9.18
Contradiction 2.12 4.9 4.18Ч4.21 5.93
Contrapositive 5.11 5.26 5.60 5.93
Convergence of absolutely convergent series 5.66Ч5.70 5.77
Convergence of bounded monotonic sequences 4.34Ч4.35
Convergence of Cauchy sequences 4.55Ч4.58
Convergence of geometric series 5.2 5.9 5.12 5.20
Convergence of infinite decimals 4.11Ч4.14 4.31
Convergence of sequences of functions: point wise 12.11Ч12.14
Convergence of sequences of functions: uniform 12.15Ч12.46
Convergence of sequences, definition 3.48 3.60
Convergence of series, definition 5.5
Convergence of Taylor scries 9.43 5.44
Convergence, conditional 5.71Ч5.75
Convergence, general principle of 4.57 5.18
Convergent sequences, absolute value rule 3.54
Convergent sequences, are bounded 3.62
Convergent sequences, difference rule 3.54
Convergent sequences, inequality rule 3.76
Convergent sequences, products of 5.54 5.55
Convergent sequences, quotients of 3.65Ч3.67
Convergent sequences, reciprocals of 3.65Ч3.66
Convergent sequences, scalar rule 3.54
Convergent sequences, shift rule 3.52
Convergent sequences, squeeze rule 3.54
Convergent sequences, subsequence rule 3.54
Convergent sequences, sums of 3.54 3.55
CONVERSE 2.4
Convex functions 9.24
Cosine, definition 9.40 11.50
D'Alembert 3H 5H 8H
d'Alembert, test for sequences 3.40Ч3.41 3.70Ч3.73 3H 11.35
d'Alembert, test for series 3H 5.40Ч5.50 5.68Ч5.69 5.79 5.95 5.97 5H
Darboux 7H 9.12 10.20 10.50Ч10.51 10H
de l'Hopital 8H 9.26 9H 11.32
de la Chapelle 3H
De Morgan 1H
De Sarasa 10H 11H
Decimals 3.51 4.11Ч4.17 4.22 4.31
Decimals, infinite 3.51 4.14 4.23 4.30
Decimals, non-recurring 4.17
Decimals, recurring 4.11Ч4.16
Decimals, terminating 3.51 4.9 4.11 4.22 4.31
Decreasing function 5.56Ч4.57
Decreasing sequence 3.4 3.6
Dedekind 4H App.
Dense sets 4.4Ч4.11
Derivative 8.5
Derivative, inverse function 8.39Ч8.42
Derivative, second 8.35Ч8.37 9.22 9.29
Derived function 8.26Ч8.28
Descartes 8H
| Differentiable function are continuous 8.12
Differentiable function at one point 8.24
Differentiable function, chain rule for 8.18
Differentiable function, products of 8.13
Differentiable function, quotients of 8.17
Differentiable function, sums of 8.10
Dini 9H
Dirichlet 3H 5H 6.20 6H 7H 10.11 10H 12.27
Discontinuity 6.16 6.18Ч6.20 12.3Ч12.35
Discontinuity, jump 6.18 6.79Ч6.80
Discontinuity, removable 6.79 6.82
Divergent series 5.11 5.28Ч5.30 5.38 5.47 5.75
Domain of function 6.1 6.3
du Bois Ч Reymond 4.34 4.57 4H
e 2.43Ч2.49 2H 4.36 5.23 11.27Ч11.28 11.32
Equivalent propositions 4.32 4.46 4.56 4.79
Euclid 2.32 2H 3H 4H
Euler 2H 5.27 5.56 5H 6H 8H 11.32 11H
Euler's constant 5.56
Eventually, in sequence 3.13 3.17 3.18 3.28
Exponential function 5.110 9.38 11.28 11.32Ч11.33 11.37
Extending of continuous function on  7.46Ч7.48 7.46Ч7.48
Fermat 1H 8H 10.1 10.3 10H
Field App. 1
Field, Archimedean ordered App. 1
Field, complete Archimedean ordered App. 1
Field, ordered 2.1Ч2.29 App.
First comparison test for series 5.26
Fixed point 7.20 9.18
Floor term 3.15Ч3.16
FOURIER 5H 6H 10H
Function 6.1 6H
Function, arrow diagram of 6.2
Function, bounded 7.31Ч7.32
Function, composite 6.38 6.40
Function, constant 6.21 8.6 9.17
Function, continuous 6.18
Function, continuous, bounded 6.69 7.31Ч7.32 7.34
Function, continuous, positive 6.70
Function, differentiable 8.4
Function, Dirichlet's 6.20
Function, domain of 6.1
Function, fixed points 7.20 9.18
Function, identity 6.22
Function, integer 3.19 6.12
Function, integrable 5.57
Function, inverse 6. 7.22Ч7.27
Function, is continuous 8.12
Function, monotonic 5.57 7.1Ч7.7 7.22Ч7.27
Function, one to one 6.3
Function, onto 6.2
Function, point wise limits 6.9Ч6.11 12.5
Function, range of 6.1 6.2 6.3
Function, real 6.1
Function, ruler 6.72 10.36
Function, step 10.12Ч10.15
Function, sum of 6.23 8.10
Function, uniform limits 12.15Ч12.46
Function, waterfall App. 3.22
Fundamental Theorem of Arithmetic 4.1 3.18
Fundamental theorem of calculus 10.54 10H
Galileo 4H 10H
Gauss 2.38 4H 5H
Geometric mean 2.30Ч2.39
Geometric progression 3.21 2.39 2.42 5.2 5.5Ч5.9 5.12 5.32 5.40 5.86
Grassmann 1H
Greatest lower bound 4.71Ч4.78 4.81
Gregory of St Vincent 10.6 10.9 10H 11H
Gregory, James 5H 9H
hadamard 5H
Hardy 3H 8H 11.1 11.39
Harmonic series 5.30 5H
Harnack 2H 4H 11H
Heine 4.53 4H 6H 7H
Helmholtz 1H
Heron 2.37
Herschel 6H
hilbert 3H
Hopilal see Уde l'HopitalФ
HORNER 4.37
Improper integrals 10.61Ч10.68
Increasing function 7.1
Increasing sequence 3.4
Indefinite integrals 10.49Ч10.51
Indices, laws of 11.1 11.6 11.8 11.20
Induction, Principle of Mathematica 1.1Ч1.8
Infimum 4.71Ч4.78 4.81Ч4.84 7.5Ч7.7
Infinite decimal 3.51 4.11Ч4.17 4.31
infinity 4.22Ч4.30
Infinity, countable 4.24Ч4.27
Infinity, function tends to 6.100Ч6.101
Infinity, sequence tends to 3.17 3.20Ч3.23
Infinity, uncountable 4.23 4.28Ч4.30
Injection 6.3
INTEGER function 3.19 3.51 4.6
Integrable functions, continuous functions 10.39
Integrable functions, modulus of 10.34Ч10.35
Integrable functions, monotonic functions 10.7Ч10.8
Integrable functions, scalar multiple of 10.26
Integrable functions, step functions 10.12Ч10.15
Integrable functions, sum of two 10.10 10.29
Integral test for convergence 5.57 5H
Integral, improper 10.61Ч10.68
Integral, indefinite 10.50Ч10.51
Integral, lower 10.16Ч10.17 10.20Ч10.22
Integral, of continuous function 10.43 10.45
Integral, of step function 10.12Ч10.15
Integral, Riemann 10.23Ч10.24
Integral, upper 10.18Ч10.22
Integration by parts 10.57Ч10.59
Integration by substitution 10.60
Intermediate Value Theorem 7.12Ч7.21 App.
Intermediate Value Theorem for derivatives 9.12
Intervals 4.8 7.8Ч7.10 7.21
Intervals, closed 3.78 7.9 7.12Ч7.20 7.29Ч7.34
Intervals, end points of 6.91Ч6.92 8.46
Intervals, nested 4.42
Intervals, open 3.78 7.8
Inverse functions 6.6
Inverse of continuous functions 7.22Ч7.27
Irrational numbers 4.18Ч4.21 5.24
Iterative formula 9.18
JORDAN 2H
Jump discontinuity 6.18 6.79 6.80 10.56
Klein 11H App.
Koch 3.82
Koerner 8.42
L'Hopital see Уde l'HopitalФ
l'Huilier 3H 6H
Lacroix 3H
Lagrange 8H 9H
Laurent scries App. 1 App.
Least upper bound 4.59Ч4.66 4.80Ч4.82
Leathern 3H 6H
Leibniz 5H 8H 10.43 10.57 10.60 11H
Less than 2.1
Lim sup and lim inf 4.83Ч4.84 5.102
Limit of composite function 6.99
Limit of function as 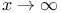 6.100 6.100
Limit of sequence 3.48 3.60
Limit of, modulus of 6.93
Limit of, one-sided 6.73 6.76 7.5Ч7.6
Limit of, products of 6.93
Limit of, reciprocal of 6.93
Limit of, sequence definition equivalent to neighbourhood definition 6.84Ч6.85 6.87Ч6.88
Limit of, sums of 6.93
Limit of, two-sided 6.86
Liouvill 4H
Lipschitz condition 7.42 9.18 10.50 11.18
Littlewood App. 2
|
|
 |
| –еклама |
 |
|
|
 |
|
ќ проекте
|
|
ќ проекте



 , countably infinite
, countably infinite