|
|
 |
| јвторизаци€ |
|
|
 |
| ѕоиск по указател€м |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Rockmore D. Ч Stalking the Riemann Hypothesis: The Quest to Find the Hidden Law of Prime Numbers |
|
|
 |
| ѕредметный указатель |
Moses 93
Mosteller, Fred 224 225
Mount Holyoke 244Ч245
Multivalued function 147Ч148
Nachlass (Riemann) 140
Nahin, Paul 72
Napoleon 81Ч82
Nash, John, Jr. 152Ч153
Natal University 211
Natural logarithm 34Ч35
Natural numbers 7Ч20
Natural numbers as "God given" 7 70 111 137
Natural numbers, composites 12Ч13
Natural numbers, defined 7
Natural numbers, primes see prime numbers
negative numbers 71
Neudecker, Werner 152
Nevanlinna, R. 145
New York Times 148 149
New York University 3Ч6 241 243 261Ч263
Newlands, John 108
Newman, Charlie 262 263
Newton, Isaac 38 48 50 52 82 236
Newton, Isaac, described 46Ч47
Nobel prize 102 150 152 155 163 171 174 257
Nobel Prize, mathematics and 141Ч142
Nobel, Alfred 141Ч142
Non-Euclidean geometry 39 123 201 203Ч205
Noncommutative geometry 230
Noncommutative multiplication 169n 230
Nonconstructive proof 265
Nonlinear differential equations 238
Nonorientable surface 97
Nontrivial zeta zeros 89Ч92
Nonzero 114n
Nuclear weapons 249Ч250 255
NUMBER 259
Number theorists 10
Number theory 10 53 265
Numerical methods 250
Numerosity, pattern of 8Ч10
Occam's razor 221
Odlyzko, Andrew 179n 198 199 218 258 263
Odlyzko, Andrew, described 180Ч181
Odlyzko, Andrew, Dyson Ч Montgomery Ч Odlyzko Law 180Ч185
On the Propagation of Heat in Solid Bodies (Fourier) 81
Operator 177
Operator theory 234
Operator, integral 178Ч179
Operator, zeta zeros and 180 220
Origin of Species, The (Darwin) 63
orthogonal matrices 219
Overfitting data 38 40
p-adic numbers 229Ч230
Pacific Institute of Mathematical Sciences 265
Painleve equations 237 238
Painleve, Paul 237Ч240 246
Painleve, Paul, described 238Ч239
Painleve: transcendants 237Ч240 256
Pair correlations 157Ч160 164
Parallel postulate 203Ч204
Partial differential equation 82
Patience sorting 253Ч254
Patterns 8Ч14
Patterns, figurate numbers and 10Ч11
Patterns, numerosity 8Ч10
Patterns, patterns within 11Ч14
Pavlovskii, V.V. 228
Peer review 148
Perfect number 12
Perfect shuffles 249
Periodic functions 83
Periodic orbit 191 192
permutations 245Ч259
Permutations, computer science and 247Ч249
Petain, Marshal 238
Philosophy 10
Physics 171
Physics, bridge between quantum mechanics and see semiclassical limit
Physics, physicists 217
Physics, statistical 233
Pi 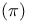 58 70Ч71 58 70Ч71
Planar domains 196
Planck's constant 187Ч188
Planck, Max 187
Plato 13 63
Poe, Edgar Allan 24 236
Poincare conjecture 202
Poincare disk 200Ч209
Poincare disk, chaos in 207Ч209
Poincare, Henri 102
Poincare, Henri, described 202
Point 204n
Poisson process 158Ч160
Poisson, Simeon-Denis 158
Polya Prize 260
Polya Ч Hilbert approach 179Ч180 185 198Ч199 227 232
Polya, George 177Ч180 259Ч260
Polya, George, described 177Ч178
Polya, George, integral operators and 178Ч179
Polynomial 72
Polytechnical School of Delft 95Ч96
Pons, Jean-Louis 40
Population density 41
Poraerance, Carl 264
Poussin, Nicholas 107 119 120
Preprocessing 248
Primal curve 28Ч29 32
Primal waves 86Ч87
Primality tests 15Ч16 264
Prime distribution analyzer (PDA) 76Ч77
Prime distribution analyzer (PDA), Dirichlet's 77 81
Prime distribution analyzer (PDA), Euler s 77 81
Prime distribution analyzer (PDA), Riemannian 77Ч81
Prime factorization 14 20
Prime number theorem 63 91Ч94 95
Prime Number Theorem, Cramer primes and 138Ч139
Prime Number Theorem, Gauss and 42Ч44 69 106 119 120 126 132Ч133 141 185
Prime Number Theorem, Legendre and 35Ч38 69
Prime Number Theorem, mathematically written 36n
Prime Number Theorem, proof of 105 106Ч107 116 118 119 129 139
Prime Number Theorem, statement of 35
Prime numbers, algorithms for checking 264
Prime numbers, as the integral atoms 14Ч15
Prime numbers, asymptotic study of 23Ч30 51 118Ч119
Prime numbers, cardinality of 22Ч23
Prime numbers, composites and 12Ч13
Prime numbers, Cramer 137Ч139 151
Prime numbers, defined 4
Prime numbers, digital cryptography and 17Ч18
Prime numbers, error correction and 16Ч17
Prime numbers, Euclid's proof of the infinitude of 18Ч20 21 24 28 50 51
Prime numbers, first cartographers of 30Ч45
Prime numbers, Gauss and see Gauss Carl
Prime numbers, Gaussian 112Ч113
Prime numbers, graphs of occurrence of 25Ч29
Prime numbers, harmonic series and 55Ч61
Prime numbers, Hawkins 151Ч152
Prime numbers, infinity of 18Ч20 21 51 59Ч62
Prime numbers, irregular appearance of 23Ч29
Prime numbers, Legendre and see Legendre Adrie-Marie
Prime numbers, music of 81
Prime numbers, pattern within the pattern 11Ч14
Prime numbers, periodic tables for 59Ч61 107Ч110
Prime numbers, Riemann hypothesis and see Riemann hypothesis
Prime numbers, search for 14Ч16
Prime numbers, series of reciprocals of 51
Prime numbers, shape of 21Ч29
Prime numbers, slowing occurrence of 41Ч42
Prime numbers, speaking in 16Ч18
| Prime numbers, twin 23Ч24
Prime numbers, zeta zeros and see zeta zeros
Princeton University 154 155 174 195 214 218 219 242Ч243
Probabilistic number theory 137
Probability theory 223
Proof (Auburn) 50
Proof of Riemann hypothesis, search for 131
Proof of Riemann hypothesis, search for, code breaking and 149Ч152
Proof of Riemann hypothesis, search for, Cramer and 136Ч139
Proof of Riemann hypothesis, search for, de la Vallee-Poussin and 107Ч110
Proof of Riemann hypothesis, search for, early twentieth century pursuit of 120Ч127
Proof of Riemann hypothesis, search for, eigenvalues and see eigenvalues
Proof of Riemann hypothesis, search for, epilogue 263Ч266
Proof of Riemann hypothesis, search for, first steps in 128Ч153
Proof of Riemann hypothesis, search for, Hadamardand 107Ч110
Proof of Riemann hypothesis, search for, Hamiltonian matrix and 227Ч228
Proof of Riemann hypothesis, search for, limits of computation 132Ч134
Proof of Riemann hypothesis, search for, Millennium meeting and 3Ч6 261Ч263
Proof of Riemann hypothesis, search for, Nash and 152Ч153
Proof of Riemann hypothesis, search for, Siegel and 139Ч140
Proof of Riemann hypothesis, search for, Stieltjes and 95Ч99 104Ч105
Proof of Riemann hypothesis, search for, true, false, or neither 134Ч136
Proof of Riemann hypothesis, search for, two-pronged assault 129
Pseudosphere 207
Ptolemy I 13 50
Purdue University 262 265
Putnam Exam 234
Pythagoras (Pythagoreans) 10Ч13 54
Pythagoras (Pythagoreans), theorem of 113n 114 115
Quadratic formula 72
Quantization 196Ч200
Quantum chaos 187Ч190
Quantum chaos, basic conjecture of 197Ч200
Quantum chaos, Berry and 187 188Ч189 197Ч198
Quantum chaos, billiard table analogy see billiard tables for physicists
Quantum chaos, comparison of distributions 222
Quantum chaos, making order out of 213Ч231
Quantum chaos, Sarnak and 217Ч221
Quantum chaos, zeta zeros and 199
Quantum chromodynamics (QCD) 237
Quantum electrodynamics (QED) 163 171 237
Quantum gravity 236Ч237
quantum mechanics 115 136Ч137
Quantum mechanics, classical physics and 171
Quantum mechanics, Planck's constant and 187Ч188
Quantum mechanics, spectral lines and 172Ч173
Quantum mechanics, uncertainty principle and 135 187Ч188
Quantum mechanics, wave function and 171Ч172 176Ч179 see
Rademacher, Hans 147Ч149
RAF Bomber Command 162Ч163
Rains, Eric 258
Random matrices 173Ч175 177Ч180 218
Random Matrices (Mehta) 218 239 244 245
Random matrices, Deift and 244Ч246
Random matrices, RSKand 258Ч259
Random matrices, Tracy Ч Widom distributions and 234Ч241
Random walk 100Ч104 177 178
Random Walk Down Wall Street, A (Malkiel) 102
Randomness 157Ч160
Randomness, understanding through 173Ч175
Rational integers 114
Rational numbers 70 116
Real axis 74
Real numbers 116
Real part of complex numbers 73 74
Reciprocals of logarithms 41Ч42
Reciprocals of Riemann's zeta function 96Ч99
Reeds, Jim 248
Reid, Constance 179 260n
Relative (percentage) error 126Ч127
Relativity theory, general 136 236Ч237
Relativity theory, special 202
Repulsion 160
Rescaling data 158Ч160
Rhind papyrus 246Ч247
Rhind, Henry 246
Rhodes University 242
Richter scale 33Ч34
Riemann hypothesis 88Ч94 125
Riemann hypothesis as "very likely" 91Ч94
Riemann hypothesis as possibly undecidable 134Ч136
Riemann hypothesis for function fields 145Ч147
Riemann hypothesis for L-series 110
Riemann hypothesis, attempts to prove see proof of Riemann hypothesis search for
Riemann hypothesis, claimed proofs (de Branges) 262Ч263 265Ч266
Riemann hypothesis, complex numbers and 70Ч73
Riemann hypothesis, complex plane and 73Ч76
Riemann hypothesis, defined 4Ч5 47 88Ч91
Riemann hypothesis, equivalence to eigenvalue properties of matrix 179 185
Riemann hypothesis, extended 219
Riemann hypothesis, function zeta zeros and 4 5 88Ч91
Riemann hypothesis, generalized 120Ч121
Riemann hypothesis, incorrect refutation (Rademache) 147Ч149
Riemann hypothesis, Polya Ч Hilbert approach 179Ч180
Riemann hypothesis, publication of 63Ч64
Riemann hypothesis, quest to settle 4Ч6
Riemann hypothesis, raw material for 53
Riemann hypothesis, road to 64Ч69
Riemann hypothesis, web of connections to 259Ч260
Riemann hypothesis, zeta function and see Ricmann's zeta
Riemann surfaces 147
Riemann Ч Hilbert problems 243Ч244
Riemann Ч Siegel formula 139Ч140 150Ч151
Riemann, Bernhard 4Ч6 63Ч94
Riemann, Bernhard, academic mentors of 45 59 61Ч62 65Ч69 76Ч78
Riemann, Bernhard, death of 65 92Ч93
Riemann, Bernhard, early life 65Ч66
Riemann, Bernhard, formulation of zeta function 80
Riemann, Bernhard, notes of 64Ч65
Riemann, Bernhard, reinvention of space 66Ч69
Riemann, Bernhard, statement of Riemann hypothesis 88Ч91
Riemannian geometry 66Ч69
Riemannian manifold 68
Riemannian prime distribution analyzer 77Ч81
Riemanns zeta function 5
Riemanns Zeta Function (Edwards) 140
Riemanns zeta function, "zoo" of zeta functions and 142Ч143 218 see search
Riemanns zeta function, creation of 76Ч81
Riemanns zeta function, Fourier analysis and 81Ч85
Riemanns zeta function, integral form of 80Ч81 129
Riemanns zeta function, logarithm of 81
Riemanns zeta function, music of the prime powers and 81Ч85
Riemanns zeta function, reciprocal of 96Ч99
Riemanns zeta function, Riemann hypothesis and 88Ч94
Riemanns zeta function, zeros of 85Ч92 119 125Ч126
Riffle shuffling 248
Rising sequence 252Ч259
Robinson Ч Schensted Ч Knuth (RSK) construction 257Ч259
Robinson, Gilbert de Beauregard 257
Rosser, John Barkely 151
Royal Danish Academy 75
Royal Society 150
Rubinstein, Michael 219
Rudnick, Zeev 218Ч219
Rutgers University 233
Saddle point 207
Sarnak, Peter 143 185 227 231 244 260 262
Sarnak, Peter, Cohen and 214Ч216
Sarnak, Peter, described 214 263
Sarnak, Peter, Katz and 219Ч221
Sarnak, Peter, quantum chaos and 217Ч221
Sarnak, Peter, Rudnick and 218Ч219
Sato, M. 237 239Ч240
Saxena, Nitin 264
Scattering theory 242Ч243
Schensted, Craige 257
Schmit, Charles 198
Schoenfeld, Lowell 151
Scholes, Myron 102
Schroedinger, Erwin 179
|
|
 |
| –еклама |
 |
|
|
 |
|
ќ проекте
|
|
ќ проекте