|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Street R., Murray M. (Ed), Broadbridge Ph. (Ed) — Quantum Groups: A Path to Current Algebra |
|
|
 |
| Предметный указатель |
2-category 100 102
2-cell 97
2-cell, 2-category 100
2-cell, 2-functor 101 102
2-cell, identity 98
2-cell, pasting 97
2-functor 101 102
A-module 101
A-module action 101
A-module arrow 101
A-module, left 101
A-point 55
action 59 101 111
Action of an A-module 101
Adjoining an antipode 117
Adjoining an inverse for the determinant 56
Adjoining, left-dual objects 117
Adjoint, left 111
Adjoint, left 2-adjoint 111
Adjoint, Lie algebra 33
Algebra, exterior 31
Algebra, Hopf 51
Algebra, morphism 27
Algebra, of endomorphisms 28
Algebra, of formal power series 42
Algebra, opposite 30
Algebra, over R 21
Algebra, polynomial algebra 45
Algebra, polynomial R-algebra 46
Algebra, R-algebra 27
Algebra, symmetric 45
Algebra, tensor 28
Algebra, universal 87
Algebra, universal enveloping 40
Algebraic geometry 5
Algebraic varieties 5
Antipode 7 51 105 117
Appropriate representation 11)3
Arrow between monoid arrows 97
Arrow composition 78
Arrow monoid 97
Arrow of category 6
Associativity 2 27 67
Autonomous 82
Autonomous, left 81
Autonomous, left/right 82
Balanced 106
Balanced bialgebra 76
Balanced YB-operator 88
Banach spaces 79
Banach spaces, example of closed symmetric tensor category 79
Bialgebra, balanced 76
Bialgebra, braided 72
Bialgebra, commutative over k 7
Bialgebra, matrix bialgcbra 46
Bialgebra, morphia in 44
Bialgebra, quasitriangular 72
Bialgebra, R-bialgebra 42
Bialgebra, symmetric 72
Bialgebra, triangular 72
Biclosed 78
Bilinear, R-bilinear 15
Bilinear, universal bilinear function 15
Bimodule, left R-fright s-bimodule 16
Bimonoid 105
Bimonoid in 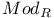 105 105
Bimonoid in V 105
Bimonoid with a braiding 106
Bimonoid with a twist 106
Bimonoid, balanced 106
Bimonoid, cobalanced 108
Bimonoid, cobraided 108
Bimonoid, cotortile 108
Bimonoid, strict quasi-bimonoid 105
Bimonoid, tortile 108 114
Bisection, canonical 1
Bradshaws xvii
Bradshaws, Age of String xviii
Bradshaws, Bradshaw Foundation xvii
Bradshaws, Edition Limitee xvii
Bradshaws, Grahame Walsh xvii
Bradshaws, silhouette viii 8 20 26 36 46 58 66 92 116 118 132 141
Braid category 69
Braid composition 69
Braid group, of Artin 69
Braid, tensor product 69
Braided 114
Braided bialgebra 72
Braided tensor category 68
Braiding 68 83 105
Braiding element 72
Braiding for a bimonoid 105
Braiding for a tensor category 68
Brown, Ronald xviii
Byrne, Simon xvi
C*-algebra, commutative 5
Cartan, Eli 33
Cartesian product x 1
Cartesian product of no sets 1
Category xi
Category of braids 69
Category, dual xii
Category, functor category xiv
Category, functors xiii
Category, monoidal 67
Category, morphisms xi
Category, objects xi
Category, of monoids in V 100
Category, opposite xii
Category, strict, tensor 104
Category, tensor 67
Cauchy H-comoduIe 65
Cauchy moduler 21
Closed functor 86
Closed tensor category 78
Closed, left-closed 77
Co-element, co-T-elements x
Coaction 62
Coalgebra over a ring 37
Coalgebra, morphism 38
Coalgebra, primitive element 41
Coalgebra, set-like element 40
Cobraiding 108
CoCauchy 47
Cocommutative coalgebra 38
Codomain target xii
Coend 115
Coherence theorem of Mac Lane 68 109
Commutative 3 30
Commutative Lie algebra 35
Commutative monoid 3
Commutative R-algebra 30
Commutative rig 4
Commutativity 3
Commutator defines a Lie bracket 32
Commute with diagonals 45
Comodule 59 62
Comodule morphism 62
Comodule, left 62
Composable morphisms xii
Composite morphism xii
Composite, pasted 100
Composition arrow 78
Composition of braids 69
Composition, vertical 98
Comuttiplication 7- 8 37
Constraint, associativity 67
Constraint, left unit 67
Constraint, right unit 67
Convergent 24
| Convolution product 39
Convolution structure 38
Coordinate algebra 9
Coordinate algebra, quantum matrices 0
Coordinate k-algebra 8
Coordinate k-algebra of general linear group 8
Coproduct xiii
Coprojection 8
Coset 14
Coset, H-coset 14
Coterminal object xiii
Cotwist 108
Counit 7 37 81
Cramer Rule quantum 120
Derivation 32
Diagonal 1
Diagonal in category X 7
Diagonal, formal 37
Diagonal, ternary 1
Direct sum of Lie algebras 34
Direct sum of modules 24
Disjoint union, X +Y x
Distributive 4
Domain source xii
Drinfel'd, Vladimir G. 105
Drinfel'd, Vladimir G., International Congress of Mathematicians, 1986 ix
Dual 21
Dual category xii
Dual, left dual 21
Dual, opposite xii
Dual, right dual 81
Duality principle x
Dualizing object 80
Dualizing object, left 79
Dualizing object, right 80
END 109 110
End is a limit 113
Endomorphism algebra 28
enrich 18
Enrich, abelian group with module structure 18
Equalizer 109
Essentially 104
Evaluation 77
Evaluation functor 95
Evaluation morphism 19
Exponential series 30
Exterior algebra 31
Faddc'ev, Ludwig D. 10
Field 4
Finitely generated 13
Forgetful functor 31 101 115
Formal diagonal 37
Formal power series 42
Formal Tannaka Duality 111
free 14
Free constructions 31
Free module 14
Free module from R to S, generated by X 16
Free module, functor 86
Freyd, Peter 82
Function identity 1
Function, complex-valued 5
Function, continuous 5
Function, diagonal 1
functor xiii
Functor category xiv
Functor, essentially weak tensor 103
Functor, evaluation 95
Functor, forgetful 101
Functor, natural transformation xiv
Functor, represent able xiv
Functor, represented by T xiii
Functor, self-adjoint 54
Functor, tensor 85
Functor, weak tensor functor 101
Fundamental theorem on coalgebras 115
Funny superscripts 10
Gelfand duality 5
General linear group 8
General linear group, commutative Hopf algebra 8
General linear group, coordinate k-algebra 8
Generalised co-element x
Generate 13
Generic point 10
Geometric series 28
Grassmannian algebra 10
Group 2 7
Group, affine over k 7
Group, diagrammatic definition 7
Group, Lie group 7
Group, R-algebra 29
Group, topological group 7
Homomorphism, generalised co-element x
Homothety 96
Hopf algebra 7 51
Hopf algebra, commutative 7 53
Hopf monoid 113
Hopf monoid, quasi 105
Hurwitz polynomials 45
Ideal 29
Ideal in an algebra 29
Identity 1 2 27 84
Identity morphism xii
Identity, 2-cell 98
Incidence, projective plane xi
Indeterminate 42
Initial object xiii
Injective 24
Injective morphism 24
Internal horn 77 110
Internal horn, left 77
Internal horn, right 78
Inverse, left- and right-inverses agree xiii
Invertibility 2
Invertible 2
Invertible isomorphism xiii
Invertible morphism xiii
Isomorphic xiii
Isomorphism xiii. 4
Isomorphism, invertible xiii
Isomorphism, isomorphic xiii
Isomorphism, natural xiv
Jacobi identity 32
Joyal, Andre xvi 91 117
k-algebra 4
k-algebra, coordinate algebra 8
k-algebra, morphism 4
Katis, Piergiulio 117
Kobyzev, Yu. 10
Kronecker delta 57
Left 2-adjoint 111
Left adjoint 111
Left dual 81
Left dual as a functor 81
Left dual of a module 21
Left dual of a signed set 83
Left inverse xiii
Left R-linear 14
Left-closed 77
Leibniz rule 32
Lie algebra 32 33
Lie algebra, adjoint 33
Lie algebra, commutative 35
Lie algebra, direct sum 34
Lie algebra, Lie bracket 32
Lie algebra, morphism 33
Lie algebra, universal enveloping 33
Lie bracket 32 33
Lie group 33
Lie. Sophus 33
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте